,2), 黎亮南京理工大学理学院, 南京 210094DYNAMIC ANALYSIS OF SANDWICH TAPERED POROUS MICRO-BEAMS CONSIDERING SIZE EFFECT 1)
Pu Gang, Zhang Dingguo,2), Li LiangSchool of Science, Nanjing University of Science and Technology, Nanjing 210094, China通讯作者: 2) 章定国, 教授, 主要研究方向: 多体系统动力学与控制. E-mail:zhangdg419@mail.njust.edu.cn
收稿日期:2019-06-25接受日期:2019-09-19网络出版日期:2019-09-20
| 基金资助: |
Received:2019-06-25Accepted:2019-09-19Online:2019-09-20
作者简介 About authors
摘要
基于修正偶应力理论, 研究了具有大范围旋转中心刚体-功能梯度夹层Euler-Bernoulli楔形多孔柔性微梁系统的动力学特性.楔形梁是中间层为不完全功能梯度层, 两表层为均质材料的功能梯度夹层结构, 它可以减小传统夹层结构由于层与层之间材料属性的不同导致脱粘类型损伤的影响.采用假设模态法描述变形, 考虑具有捕捉动力刚化效应的非线性耦合项, 计及von Kármán几何非线性应变, 运用第二类Lagrange方程, 导出了适用于较大变形的高次刚柔耦合动力学方程.对在平面内做大范围运动的中心刚体-功能梯度夹层Euler-Bernoulli楔形多孔微梁的动力学特性进行了详细研究.研究表明: 功能梯度夹层楔形梁表层结构高度、旋转角速度、功能梯度幂指数、尺度参数、孔隙度以及各层结构的体积分数对系统的动力学特性都有很大的影响; 功能梯度夹层楔形梁综合了功能梯度直梁和楔形梁的特性, 其相对于功能梯度直梁的固有频率增大, 同时使得孔隙度对结构固有频率变化趋势的影响不再与功能梯度直梁相同; 由于柔性梁变形能中具有横向与轴向的耦合势能, 系统在稳态下的平衡位置发生了迁移现象; 系统随着尺度参数的变化发生了频率转向与振型转换.
关键词：
Abstract
Based on the modified couple stress theory, a size-dependent dynamic model of large overall rotating hub-flexible sandwich tapered Euler-Bernoulli micro-beams made from functionally graded materials with porosities is developed to study their dynamic characteristics. The functionally graded sandwich tapered micro-beams are composed of a core with inperfect functionally graded materials sandwiched between two homogeneous face sheets, which can decline the influence of the traditional sandwich structure debonding damage caused by the mismatch of stiffness properties between their core and face sheets. The high-order rigid-flexible coupled dynamic equations of the system applied to large deformation are derived by considering the von Kármán geometric nonlinear strain and adopting Lagrange's equation of the second kind. The method of assumed modes is used to describe the chordwise and axial deformations of micro-beams. The dynamic stiffening effect is captured the nonlinear coupling term obtained by longitudinal shortening caused by the transverse bending deformation of the micro-beams. Then, under the effects of different parameters, such as the width of face sheets of the tapered micro-beams, rotating angular velocity, material gradient index, size-dependency, porosities and volume fractions of each layer structure, the dynamic characteristics of functionally graded sandwich micro-beams in the plane are investigated. The functionally graded sandwich tapered micro-beams combine the characteristics of the functionally graded rectangular micro-beams and the tapered micro-beams. Compared with the functionally graded rectangular micro-beams, these characteristics make the natural frequency of the functionally graded sandwich tapered micro-beams increase and lead to different influences of porosities on the natural frequencies of the structures. Moreover, since the coupling potential energy of the chordwise and axial motions is involved in the strain energy of the flexible micro-beams, the equilibrium position of the system migrates in the steady state. It is observed that interesting frequency veering and mode shift phenomena of the system are observed when the size-dependency changes.
Keywords：
PDF (3561KB)元数据多维度评价相关文章导出EndNote|Ris|Bibtex收藏本文
本文引用格式
蒲刚, 章定国, 黎亮. 考虑尺度效应的夹层楔形多孔梁动力学分析 1). 力学学报[J], 2019, 51(6): 1882-1896 DOI:10.6052/0459-1879-19-164
Pu Gang, Zhang Dingguo, Li Liang.
引言
随着航空航天以及微/纳米机械领域快速发展, 传统材料在超高温、大温度梯度等恶劣环境下可能无法适应. 为此, 最早在1984年由日本材料科学家提出的功能梯度材料(functionally graded materials, FGMs)[1]作为一种新型的复合材料被众多****广泛研究. FGMs是一种由两种或者多种各向同性材料(如陶瓷和金属)沿着某一路径按着一定体积分数光滑连续分布而形成的新型复合材料, 其中陶瓷侧提供耐热性, 金属侧提供机械强度和热导率, 并且与层合复合材料相比, 可以消除结构体系中应力集中现象, 具备了耐高温、轻质、高强度、高韧性等特点, 使结构更加容易适应复杂多变的工作环境[2-3]. FGMs由于其力学性能的优越性而被广泛应用于航空航天结构、涡轮叶片、火箭发动机部件、聚变能源装置、国防工业等领域. 此外, FGMs在不同的环境下其材料属性会发生很大的改变. 在烧结过程中, FGMs会在其内部形成气孔[4-5], 这对梯度(FG)结构的力学性能有很大影响. 因此, 有必要对含孔隙率的FGMs梁的动力学特性进行研究.近年来, 夹层结构在各种工程中被广泛应用, 但这种结构的主要缺点是它们的中心层和表层之间的刚度特性不匹配, 当夹层梁或板受到冲击载荷时, 极易导致脱粘类型损伤. 为了减少该种损伤对夹层结构的破坏, ****们将FGMs的概念与夹层结构结合起来, 产生了一种新型的功能梯度夹层(functionally graded sandwich, FGS)[6]结构. FGS结构主要有两种类型, 第一种类型是夹在两个均质表层之间为FG层的夹层结构, 第二种类型是均质中心层夹在两个FG表层之间的夹层结构. Correia等[7]通过考虑重量和成本最小化、固有频率最大化和应力降低等几个相互矛盾的目标函数, 对FG中心层的FGS板进行了优化. Al-shujairi等[8]在非局部应变梯度理论下, 研究了考虑热效应的中心层为均质层的FGS微梁的动力稳定性. Tomar等[9]研究了材料不确定性对具有倾斜角的FGS板的振动和弯曲性能的影响. Hao等[10]基于一种新的位移场分析了几何非线性FGS扁壳的稳定性. Loja[11]通过粒子群算法对FGS梁结构抗弯刚度进行了优化.
如今, 随着微动开关、微型无人机、纳米机器人、纳米发动机、纳米传感器、原子力显微镜等微型机械的不断出现, 微/纳米材料和结构在许多领域中都成为了研究热点, 微梁则是在微/纳米电子机械系统中普遍存在且研究众多的结构[12]. 因此, 微梁与宏观梁结构相对应的静力学、动力学以及屈曲等特性的问题引起了众多****的关注[13]. ****们通过大量的实验表明, 经典的连续介质理论由于未考虑微结构本身的材料特征长度参数的影响而不能正确描述其力学特性, 这是因为粒子之间的间隙在微尺度结构中扮演着重要的作用[14]. 为了能够通过本构方程来表示微结构的尺寸依耐性, 最常用的几种考虑了材料特征长度参数的非经典连续介质理论主要有: 偶应力理论、应变梯度理论和第二应变梯度理论[15]. 其中, 偶应力理论是在考虑物体变形时, 同时考虑了物质粒子的平移和旋转特性, 物质粒子的旋转可以通过用一个有代表性的体积单元包裹物质粒子来实现, 旋转特性的存在使得微观结构拥有了材料特征长度参数的影响. 偶应力理论由于只考虑了高阶应变张量的旋转曲率张量, 使得材料特征长度参数的个数由应变梯度理论的3个减少为1个, 为计算和实验都带来了方便. Ghadiri等[16]基于修正偶应力理论对旋转功能梯度Timoshenko梁在不同温度分布下进行了振动分析. Mirjavadi等[17]对在修正偶应力下的二维FG锥形多孔梁的热屈曲行为进行了分析. Ansari等[18]基于应变梯度Reddy梁理论分析了功能梯度微梁的自由振动. Karparvarfard等[19]研究了基于第二应变梯度理论的几何非线性梁模型.
众多****对在大尺度下做大范围运动的刚柔耦合模型的动力学问题有着深入的研究, 分析了柔性梁的二阶非线性耦合项对系统响应和固有频率的影响, 该耦合项使大范围运动的旋转结构能够适应更高的转速而不至于快速发散[20-24]. 但考虑耦合项的旋转动力学问题在微尺度下的研究屈指可数[25], 其中, 对含FGS楔形多孔柔性微梁这类新型复合材料结构的刚柔耦合动力学研究更是鲜见报道. 黎亮等[26]对中心刚体旋转功能梯度梁的刚柔耦合动力学模型进行了横向振动和纵向振动的耦合分析, 用模态振型对梁的频率转向进行了解释. 方建士等[27]研究了三维旋转功能梯度Euler - Bernoulli梁的不同材料参数对模态特性和响应的影响. 陈渊钊等[28]用绝对节点坐标法分析了具有安装角的旋转柔性板和曲梁的动力学特性. Zhao等[29]研究了旋转三维悬臂梁的耦合振动问题. 章定国等[30-31]在尺度效应下, 研究了微梁刚柔耦合动力学特性, 发现尺度效应使微梁的振幅减小, 频率变大.
由前面可知, FGS直梁的屈曲、多物理场、结构优化设计、旋转动力学等问题都研究了很多. 然而, FGS楔形微梁的研究一直处于空白, FGS楔形微梁结合了FGMs直梁和纯楔形梁的的特性, 使得自身具有更好的耐热性和强度特性, 不仅如此, 其在大范围旋转的过程中, 可以通过设计和调节表层结构的高度来适应不同的转速和热环境, 相对于FGS直梁可以节省一定的材料. 再者, FGMS材料在一定温度下会出现孔隙现象, 很多****只是研究了孔隙度对FGMs梁的影响, 但对层合结构特别是FGS楔形微梁中考虑孔隙度的大范围旋转结构研究甚少.
本文基于修正偶应力理论, 对做大范围旋转运动的中心刚体-FGS楔形多孔微梁系统的动力学问题进行了研究. 首先采用假设模态法描述变形, 考虑柔性梁横向变形引起纵向缩短的二阶非线性耦合项, 运用第二类Lagrange方程, 得到系统的高次耦合动力学方程. 通过数值仿真算例分析了刚柔耦合系统的表层结构高度、旋转角速度、功能梯度幂指数、尺度参数、孔隙度以及各层结构体积分数等对FGS楔形梁动力学特性的影响.
1 系统动力学建模
1.1 物理描述
研究如图1所示的中心刚体-FGS楔形多孔微梁系统, 在力矩$\tau$的作用下中心刚体做定轴转动, 在定轴上$O$点建立惯性坐标系$OXY$,柔性梁一端与中心刚体固结于$o$处, 在$o$点建立浮动坐标系$oxy$,$x$轴与梁未变形时的通过FG层几何质心的轴线重合, $ox$轴与$OX$轴的夹角为$\theta$. 中心刚体绕定轴的转动惯量为$J_{\rm ch}$, 半径为$R$.图1
新窗口打开|下载原图ZIP|生成PPT图1中心刚体-柔性梁变形示意图
Fig.1The deformation of the hub-flexible beam
图2为柔性梁的中性层剖面图, 柔性梁是由陶瓷、FGMs和金属三层材料组成的FGS楔形梁. 首先定义柔性梁靠近$y$轴正向的表面为上表面, 靠近$y$轴负向的表面为下表面. 再者, 柔性梁两表层陶瓷和金属层为梯形结构, 梁的长度为$L$, 宽度为$b$, 与中心刚体接触的梁高为近端高度$h_4+ h_1 $, 距离中心刚体最远的梁高为远端高度$h_6 + h_5$, FG层上表面到$x$轴的距离为$h_3$,下表面到$x$轴的距离为$h_2$, 柔性梁两表层高度满足关系$h_1 > h_5 $, $h_4 > h_6 $, 假设$h_3 =h_2 $.
图2
新窗口打开|下载原图ZIP|生成PPT图2柔性梁中性层剖面图
Fig.2The neutral layer of the flexible beam
在本文中, 假设柔性梁的材料分布满足幂函数关系如下
其中, $V_{\rm m}(y)$和$V_{\rm c}(y)$分别表示柔性梁沿着高度方向金属和陶瓷材料的体积分数, $N$表示材料沿高度方向梯度分布的非负幂指数. 在$-h_1$到$-h_2$之间为纯陶瓷层, 在$h_3$到$h_4$之间为纯金属层, 在$-h_2$到$h_3$之间为FG层, FG层的材料属性沿着高度从纯陶瓷到纯金属连续变化.
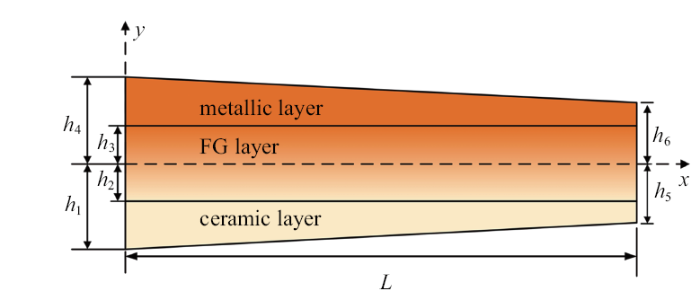
图3为孔隙度非均匀分布的FGS楔形梁的FG层横截面, 梁的材料属性表示为[32]
图3
新窗口打开|下载原图ZIP|生成PPT图3孔隙度非均匀分布的FGS楔形梁FG层横截面
Fig.3Cross section of FG layer of FGS tapered beam having uneven distribution of porosities
这里, $P_{\rm or}$表示孔隙度非均匀分布的函数为
其中, 下标m和c分别表示金属和陶瓷材料, $n$表示孔隙度, $P(y)$代表柔性梁上某一点的杨氏模量$E(y)$、密度$\rho(y)$和泊松比$\upsilon(y)$.
1.2 修正偶应力理论
由于微观材料在结构上表现出强烈的尺寸依赖特性, 传统的经典理论不能正确地描述微观结构的力学性能, 因此, 本文根据修正偶应力理论[33], 微尺度下柔性梁的变形能可表示为其中, $i,j = x,y,z$, 符号$V$代表柔性梁的体积, $\varepsilon _{ij} $和$\chi_{ij}^s$分别表示经典应变张量和转动梯度张量的分量, $\sigma_{ij}$和$m_{ij}^s$分别是与应变张量和转动梯度张量共轭的应力张量分量.
转动梯度张量$\chi_{ij}^s$的产生, 主要是由于当材料尺寸减小时, 长程原子间的相互作用和分子间凝聚力的影响会变得显著, 此时, 考虑材料变形时材料状态变化过程中的平移和旋转, 同时消除应变梯度的对称部分, 即膨胀梯度张量和拉伸梯度张量被忽略, 高阶应变张量因此只有转动梯度张量, 最后, 只取转动梯度张量的对称部分即得修正偶应力理论. $\varepsilon _{ij} $和$\chi_{ij}^s$表示为
式中, 符号","表示对坐标$x,y,z$求导, $u_i $和$\varphi _i$分别表示柔性梁上任意一点变形矢量的分量和无穷小旋转矢量的分量, $\varphi _i$与$u_i $满足如下关系
应力张量分量与相应应变张量分量之间的本构关系如下
式中, $l$是高阶应力张量分量的材料长度尺度参数, $\lambda (y)$和$\mu(y)$表示由于功能梯度作用随着$y$变化而变化的拉梅系数, $\delta_{ij}$表示$3\times3$的单位矩阵, 拉梅系数$\lambda (y)$和材料泊松比$\upsilon(y)$与弹性模量$E(y)$存在如下关系
1.3 系统势能
基于Euler - Bernoulli梁理论, 同时假设FGS楔形梁同一横截面上的不同点在浮动坐标系方向上的变形差别忽略不计, 柔性梁任意一点的变形场可表示为其中, $w_1 (x,t)$和$w_2(x,t)$分别为$y=0$时柔性梁的轴向变形量和横向弯曲变形量, $w_{\rm d}$是由梁的横向弯曲变形引起纵向缩短的二阶非线性耦合量, $k_z$表示梁绕着浮动坐标系$z$轴的旋转角度. 传统的零次耦合模型由于忽略了二阶非线性耦合量$w_{\rm d}$, 使其不能正确描述系统在高速运动时的真实情况. 二阶非线性耦合量$w_{\rm d}$和旋转角度$k_z $表示如下
考虑到柔性梁只具有轴向应变, 且能够适应较大变形环境, 在此, 将式(6)中$\varepsilon _{ij}$用非线性von K\'{a}rm\'{a}n张量的分量代替如下[34]
将式(10)代入式(7)可以得到无穷小旋转分量为
将式(6)、式(10)和式(13)代入式(8), 应力张量分量$\sigma _{ij}$和高阶应力张量分量$m_{ij}^s$的非零部分分量可以表示为
再将式(6)和式(14)代入式(5)中得到变形能表达式为
其中
式中, $f_{\rm c} (x)$表示柔性梁陶瓷层下表面的边界函数, $f_{\rm m} (x)$表示柔性梁金属层上表面的边界函数.
本文忽略重力势能对系统的影响, 系统中的势能只由梁的变形能组成.
1.4 系统动能
柔性梁上任意一点的位置矢量可以表示为将上式对时间求导得柔性梁任意一点的速度矢量$\dot{ r}$为
系统的动能表示如下
1.5 系统的刚柔耦合动力学方程
通过假设模态法, 轴向变形$w_1 (x,t)$和横向变形$w_2 (x,t)$可以离散为式中, $ \phi_x (x) \in R^{1\times K}$和$ \phi _y (x) \in R^{1\times K}$分别为柔性梁的纵向振动和横向振动的模态函数的行矢量, $ A(t) \in R^{K\times 1}$和$ B(t) \in R^{K\times 1}$分别为纵向振动和横向振动的模态坐标列矢量, $K$为模态截断数. 将离散变量代入二阶非线性耦合量$w_{\rm d}$中得到表达式
其中, $ H(x) \in R^{K\times K}$为耦合形函数,其表达式为
式中, $ \phi _y' $表示$ \phi _y $对$x$的偏导.
取$ q = (\theta , A^{\rm T}, B^{\rm T})^{\rm T}$作为广义坐标, 运用第二类Lagrange方程
式中, $ F_q = (\tau , 0, 0)^{\rm T}$是外主动力对应的广义力, 将式(15)和式(19)代入式(23), 即可得到系统的刚柔耦合动力学方程
式中
相关的常系数矩阵表达式如下
式(24) $\sim\!$式(52)表示旋转中心刚体-FGS楔形多孔微梁的高阶刚柔耦合动力学方程. 在动力学方程中, 单下划线的部分是由二阶非线性耦合量$w_{\rm d}$产生的附加耦合项, 双下划线的部分是与$w_{\rm d} $, $w_1 $, $w_2 $以及它们对时间的偏导数相关的三阶或更高阶的耦合项. 去掉双下划线部分, 该动力学方程就是一次近似耦合模型, 适用于高速小变形情形; 去掉双下划线和单下划线部分, 方程进一步退化为零次近似耦合模型, 适用于低速小变形情形; 而本文获得的是包含了单下划线和双下划线的高次耦合模型, 适用于高速较大变形情形; 较零次和一次近似耦合模型有更大的适用性[35]. 从动力学方程中还可以注意到, 具有尺度效应的材料长度尺度参数$l$包含在式(42)中, 孔隙度$n$由于其对杨氏模量、密度和泊松比的影响在整个动力学方程中得以体现.
2 数值仿真
首先, 为了研究中心刚体-FGS楔形多孔微梁表层结构对系统动力学的影响, 对表层结构高度做出如下两种假设:情形1 FGS 楔形梁近端高度不变, 远端高度$h_5 - h_2 $、$h_6 - h_3 $随着高度系数$R_{\rm c}$和$R_{\rm m} $的变化而变化, 表层结构的总体积发生改变.
定义陶瓷层的高度变化关系为
金属层的高度变化关系为
在该种情形下, 令$h = h_4 + h_1 $, 表示柔性梁的近端高度. 再者, 为了研究柔性梁中各层结构体积占比对系统的影响, 令
其中, $w_{\rm c} $和$w_{\rm m} $分别表示陶瓷层和金属层近端高度与FG层高度比值.
情形2 分别令梁表层的远端高度与近端高度比为, $S_{\rm c} = ( h_5 - h_2)/(h_1 - h_2)$, $S_{\rm m} = ( h_6 - h_3) / (h_4 - h_3)$, 且 $S_{\rm c}$和$S_{\rm m}$分别满足如下关系
其中, Constc和Constm分别表示两表层的近端高度与远端高度之和为定值, 即无论$S_{\rm c} $与$S_{\rm m} $怎么改变, 表层结构的总体积不变. 令$h$为
在这种情形下, $h$表示两表层的近端高度、远端高度与FG层的近端高度之和. 同样, 为了研究柔性梁中各层结构体积占比对系统的影响, 令
这里, $h_{\rm c} $和$h_{\rm m} $分别表示陶瓷层和金属层近端高度与远端高度的平均值(即平均高度)跟FG层近端高度的比值.
为了研究的普适性, 定义如下无量纲变量
其中, $T = (\rho _{\rm m}A_0L^4 / E_{\rm m} I_0 )^{1 / 2}$为广义时间参量, $A_0 = hb$, $I_0 = bh^3 / 12$, $\delta $为刚柔耦合系统径长比, $\sigma $为宽度与长度比值, $\zeta $为不同情形下的$h$与宽度的比值, $\beta $为无量纲材料长度尺度参数, $\gamma $为无量纲角速度.
2.1 系统的响应分析
首先, 为了验证该建模理论能够适用于较大变形, 将该建模理论应用于文献[36]中的柔性单摆, 其参数同文献[36]一样, 可知为$L=1.8$ m, $b=3.125$ cm$^{2}$, $h_3=h_2=0.4$ cm$^{2}$, $I_z =0.13$ cm$^{4}$, $\rho =2766.67$ kg/m$^{3}$, $E=6.895$~GPa, $w_{\rm c} = w_{\rm m}=0$. 通过图4发现, 用本文高次耦合理论对柔性单摆大变形进行仿真计算, 仿真结果与绝对节点坐标法的结果基本吻合.图4
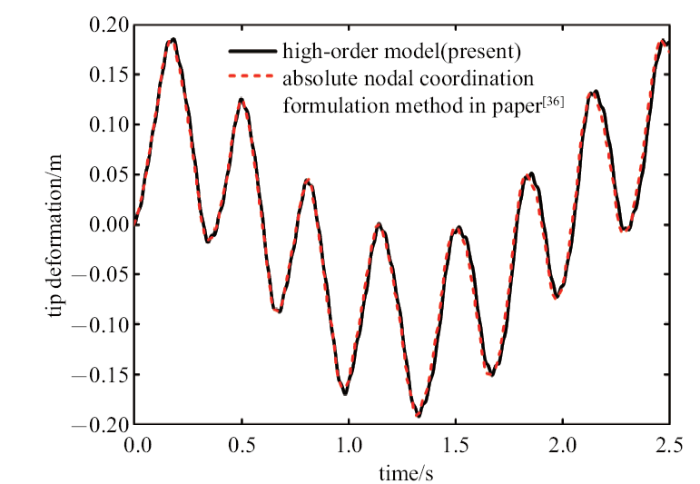新窗口打开|下载原图ZIP|生成PPT图4柔性单摆的末端变形
Fig.4The tip deformation of the pendulum
接着, 对本文系统进行响应分析. 我们知道在研究非惯性系下的动力学问题时, 系统运动往往为大范围运动已知或者未知. 本小节主要对旋转中心刚体-FGS楔形多孔微梁模型在大范围运动已知时的动力学特性进行仿真. 设系统旋转角速度变化规律为
其中, 无量纲角速度加速时间为$T^ * =6$, 角速度达到稳定后为$\gamma _0=5$.
本文柔性梁材料参数取自于文献[38], 陶瓷材料(Al$_2$O$_3$)的弹性模量为$E_{\rm c} = 380$~GPa, 密度为$\rho _{\rm c} = 3800$ kg/m$^{3}$, 泊松比为$v_{\rm c} = 0.3$, 金属材料(Al)的弹性模量为$E_{\rm m} = 70$~GPa, 密度为$\rho _{\rm m} = 2700$ kg/m$^{3}$, 泊松比为$v_{\rm m} = 0.3$.
图5是在柔性梁参数为$\sigma = 1/20$, $\delta = 0$, $\zeta = 1$, $n=0$, $S_{\rm c} = S_{\rm m}=1/2$, $h_{\rm c} = h_{\rm m}=1/12$时, 旋转中心刚体-柔性梁横向弯曲变形时的尖端响应. 从图中可以发现, 随着梯度指数$N$增大或者无量纲材料长度尺度参数$\beta$增大时, 柔性梁的横向变形减小, 平衡位置逐渐靠近初始状态, 稳态振幅也在随之减小. 这是因为式(16)中包含了横向弯曲变形与轴向拉伸变形的耦合势能, 从而导致了如图所示的随着参数$N$或者$\beta$改变, 平衡位置发现偏移的现象.
图5
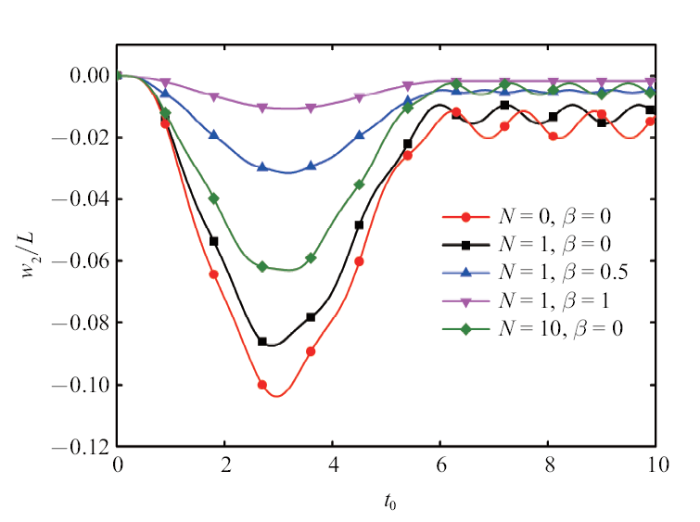新窗口打开|下载原图ZIP|生成PPT图5柔性梁在不同$N$和$\beta $下的尖端响应
Fig.5Tip responses of flexible beams for several values of $N$ and $\beta$
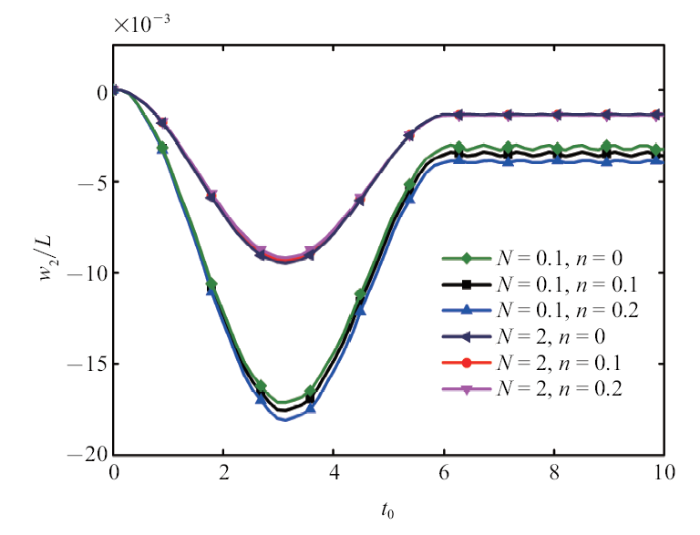
图6是在柔性梁参数为$\sigma = 1/20$, $\delta = 0$, $\zeta = 1$, $\beta =1$, $S_{\rm c} = S_{\rm m} =1/2$, $h_{\rm c} = h_{\rm m}=1/12$时, 旋转中心刚体-柔性梁在不同孔隙度$n$和幂指数$N$下横向弯曲变形时的尖端响应. 从图中可以看出, 当梯度指数$N$较大时, 孔隙度$n$对系统尖端响应的影响很小,当$N$较小时, $n$对系统尖端响应的影响较大, 同时对稳态平衡位置以及稳态振幅都有一定的影响. 这是因为当$N$较大时, 陶瓷在结构中占主要成分, 一定范围内的孔隙度造成弹性模量的损失量相对于陶瓷自身较大的弹性模量来说很小, 当$n$增大到较大值时才会对结构有比较明显的影响,此时, 结构可能发生了破坏. 相反, 当$N$较小时, 金属在结构中占主要成分, 由于金属弹性模量较小, 较小的孔隙度造成弹性模量的损失量都会对结构有较大的影响.
图6
新窗口打开|下载原图ZIP|生成PPT图6柔性梁在不同$N$和$n$下的尖端响应
Fig.6Tip responses of flexible beams for several values of $N$ and $n$
图7是在柔性梁参数为$\sigma = 1/20$, $\delta = 0$, $\zeta = 1$, $N=1$, $n=0$, $\beta=1$的横向弯曲变形时的尖端响应. 其中图7(a)是在 $h_{\rm c}=h_{\rm m}=1/12$时与 $S_{\rm c}$, $S_{\rm m}$有关的变化曲线, 图7(b)是在 $w_{\rm c}=w_{\rm m}=1/2$时与$R_{\rm c}$, $R_{\rm m}$有关的变化曲线. 从图7(a)中可以发现, 当柔性梁两表层体积不变时, $S_{\rm c}$, $S_{\rm m}$对结构响应影响很小, 主要是由于表层结构体积在柔性梁总体积中的占比较小. 从图7(b)中可以发现, $R_{\rm c}$, $R_{\rm m}$对结构响应有较大影响, 当 $R_{\rm c}=R_{\rm m}=1$时, 柔性梁为直梁, 结构由直梁变为楔形梁时, 柔性梁的变形减小.
图7
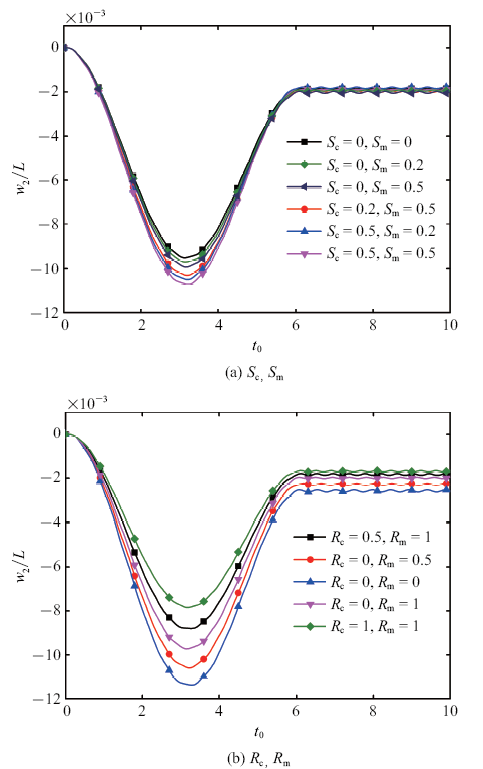新窗口打开|下载原图ZIP|生成PPT图7柔性梁的尖端响应
Fig.7Tip responses of flexible beams
2.2 系统的自由振动分析
式(26)为柔性梁做大范围运动未知的动力学方程, 本小节主要研究中心刚体-FGS楔形梁在做大范围运动已知时的自由振动特性. 为了研究方便, 假设中心刚体的大范围运动角速度为定值, 则系统横向弯曲与纵向拉伸相互耦合的自由振动方程可以写为如下式中
其中, 横向振动刚度$K_{33}$包含了二阶非线性耦合项和材料长度尺度参数.
通过式(62)可以得到系统的第$k^ * $阶固有频率$\lambda _{k^ * }(k^ * = 1,2,3\cdots)$, 进而可以得到无量纲固有频率为
式中, $\varpi _{k^ * }$表示第$k^ * $阶无量纲固有频率.
表1给出了在参数$\gamma = 10$, $\delta = 0.1$, $\sigma = 1/20$, $\zeta = 1$, $n=0.05$, $w_{\rm c} = w_{\rm m}=1/6$, $R_{\rm c}=R_{\rm m}=0.5$时旋转hub-FGS楔形梁(之后可直接称为柔性梁)在不同阶模态下的前三阶无量纲固有频率, 从表中可以看出, hub-柔性梁的固有频率随着$K$增大很快收敛, 本文取$K=7$作为模态截断数.
Table 1
表1
表1无量纲频率的收敛性
Table 1
 |
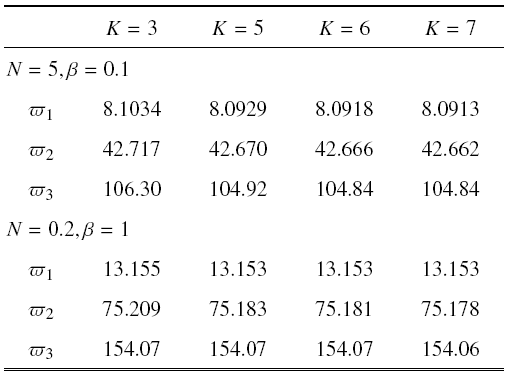新窗口打开|下载CSV
表2给出了在参数$\gamma = 10$, $\delta = 0$, $\sigma = \sqrt{12}/70$, $\zeta = 1$, $\beta = 0$, $N = 0$, $n=0$, $w_{\rm c} = w_{\rm m}=0$时旋转柔性梁的前四阶无量纲固有频率, 通过与文献[37,38]进行对比可知本文模型的正确性.
Table 2
表2
表2旋转柔性梁的前四阶无量纲固有频率
Table 2
 |
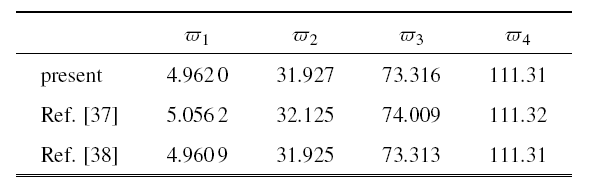新窗口打开|下载CSV
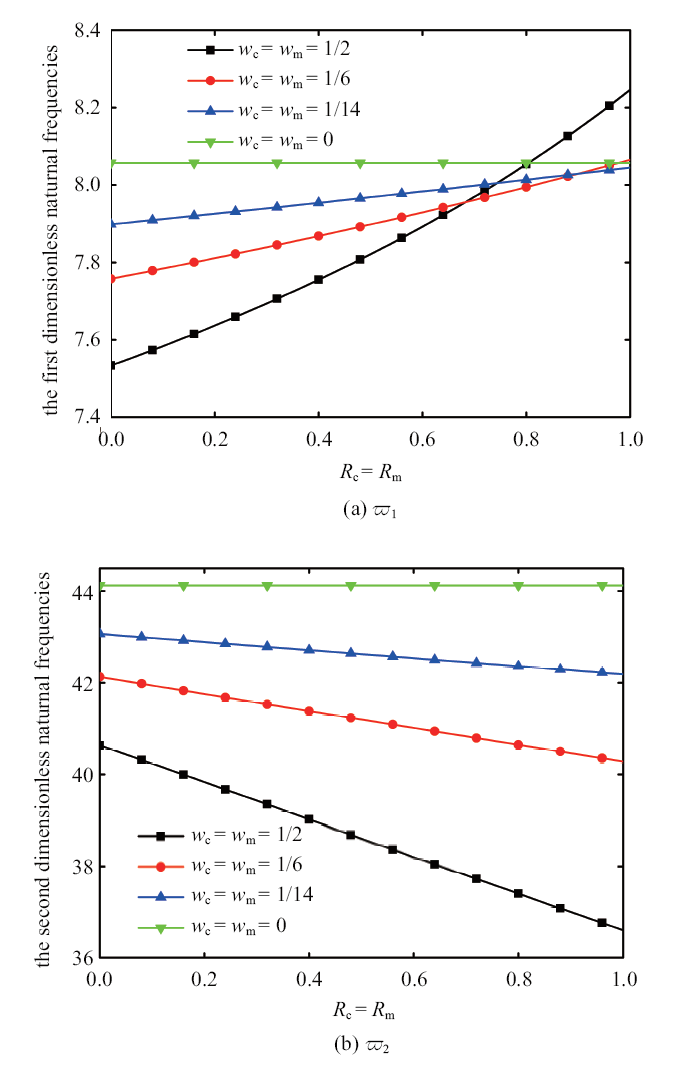
图8是在柔性梁参数为$\sigma = 1 / 100$, $\delta = 0.1$, $\zeta = 1$, $\beta = 0.1$, $\gamma = 10$, $N = 2$, $n = 0$时, 对于不同$w_{\rm c}$, $w_{\rm m}$的无量纲固有频率$\varpi$随着高度系数$R_{\rm c}$, $R_{\rm m}$的变化曲线. 从图中可以看出, 第一阶无量纲固有频率$\varpi _1 $随着$R_{\rm c} $, $R_{\rm m} $的增大而增大, 第二阶无量纲固有频率$\varpi _2 $随着$R_{\rm c} $, $R_{\rm m} $的增大而减小. 当$w_{\rm c}=w_{\rm m}=0$时, 柔性梁变为单层FGMs直梁, 柔性梁由直梁变为楔形梁时, $\varpi _2 $变小. 在图8(a)中还可以发现, 随着$w_{\rm c} $, $w_{\rm m} $越小, 曲线的斜率越小.再者, 楔形梁在不同$w_{\rm c} $, $w_{\rm m} $时的$\varpi _1 $与直梁的$\varpi _1 $出现了交叉点, 随着$w_{\rm c} $, $w_{\rm m} $减小, 该交叉点向右平移.
图8
新窗口打开|下载原图ZIP|生成PPT图8无量纲固有频率与$R_{\rm c}$, $R_{\rm m} $的关系
Fig.8Dimensionless natural frequencies versus $R_{\rm c} $, $R_{\rm m} $}
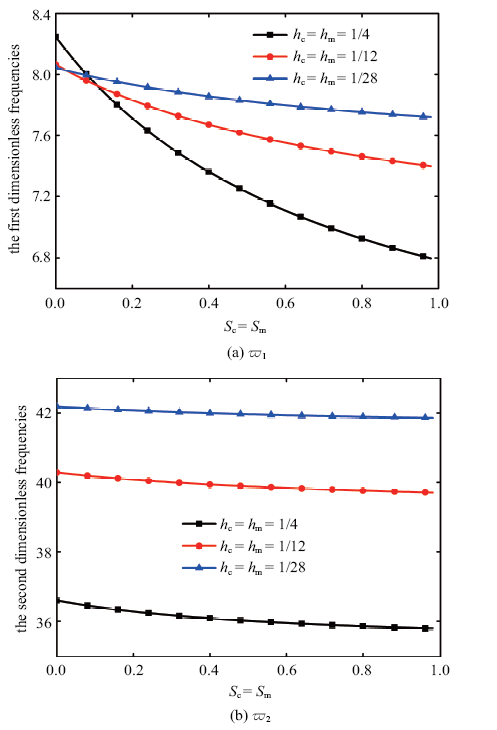
图9是在柔性梁参数为$\sigma = 1 / 100$, $\delta = 0.1$, $\zeta = 1$, $\beta = 0.1$, $\gamma = 10$, $N = 2$, $n = 0$时, 对于不同$h_{\rm c} $, $h_{\rm m} $的$\varpi $随着$S_{\rm c} $, $S_{\rm m} $的变化曲线. 图中显示, $\varpi _1 $和$\varpi _2 $均随着$S_{\rm c} $, $S_{\rm m} $的增大而减小. 不仅如此, $\varpi _1$和$\varpi _2$也随着$h_{\rm c}$,$h_{\rm m}$的增大而减小, 曲线的斜率也越小. 在图9(b)中还可以发现, 随着$h_{\rm c} $, $h_{\rm m} $减小, $\varpi _2 $增大.在图9(a)中可以发现, $\varpi _1 $在不同$h_{\rm c} $, $h_{\rm m} $时同样出现了交叉现象.
图9
新窗口打开|下载原图ZIP|生成PPT图9无量纲固有频率与$S_{\rm c} $, $S_{\rm m} $的关系
Fig.9Dimensionless natural frequencies versus $S_{\rm c} $, $S_{\rm m} $
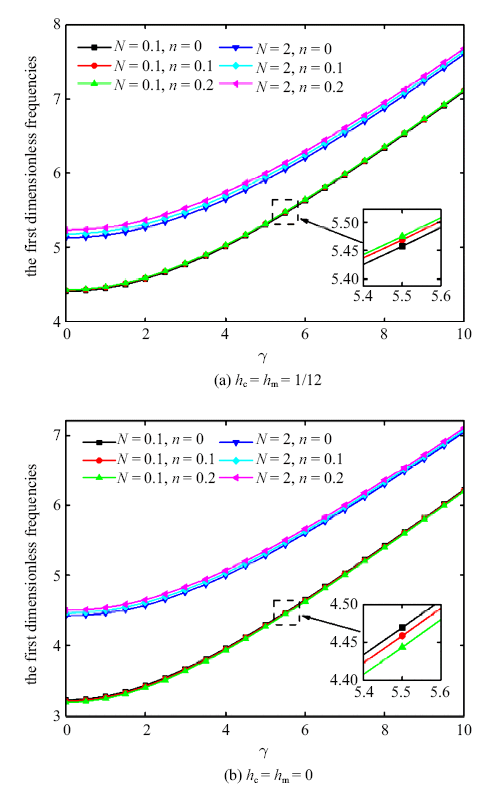
图10是在柔性梁参数为$\sigma = 1 / 100$, $\delta = 0.1$, $\zeta = 1$, $\beta = 0.1$, $S_{\rm c} = S_{\rm m}=1/2$时, 对于不同幂指数和孔隙度的$\varpi $随着无量纲角速度$\gamma $的变化曲线, 图10(a)表示FGS楔形梁, 图10(b)表示FGMs梁. 从图中可以发现, $\varpi _1 $随着$\gamma $的增大而增大, 当$\gamma $和$n$一定时, $\varpi _1 $随着幂指数$N$的增大而增大. 再者, 图10(a)中, 当$\gamma $和$N$一定时, $\varpi _1 $随着$n$的增大而增大; 图10(b)中, 当$\gamma $一定, $N=0.1$时, $\varpi _1 $随着$n$的增大而减小, $N=2$时, $\varpi _1 $随着$n$的增大而增大, 图10(a)与图10(b)变化趋势的差别, 也正是FGS楔形多孔梁与FGMs多孔梁的区别所在. 还可以发现, 曲线斜率随着$\gamma $的增大先增大后达到一个稳定值, 这是因为$\gamma $较小时, 刚度矩阵中的静刚度矩阵起着主导作用, 当$\gamma $较大时, 静刚度矩阵相对于动力刚度矩阵很小, 使频率的变化几乎不受静刚度矩阵的影响.
图10
新窗口打开|下载原图ZIP|生成PPT图10无量纲固有频率与无量纲角速度的关系
Fig.10Dimensionless natural frequencies versus dimensionless angular velocity
图11是在柔性梁参数为$\sigma = 1 / 100$, $\delta = 0.1$, $\zeta = 1$, $\beta = 0.1$, $\gamma = 10$, $S_{\rm c} = S_{\rm m} = 1/2$时, 对于不同幂指数$N$的$\varpi $随着孔隙度$n$的变化曲线, 图11(a)表示FGS楔形梁, 图11(b)表示FGMs梁. 从图中可以看出, $\varpi _1 $随着$N$的增大而增大. 再者, 图11(a)中, 当$N$一定时, $\varpi _1$随着$n$的增大而增大; 图11(b)中, 当$N=0.2$时, $\varpi _1$随着$n$的增大而减小, 随着$N$逐渐增大, $\varpi _1 $随着$n$的变化趋势也在改变, 最后变为随着$n$的增大而增大, 该种变化趋势与文献[4]中的相同. 可以发现, 该种现象与图(10)的现象息息相关.
图11
新窗口打开|下载原图ZIP|生成PPT图11无量纲固有频率与孔隙度的关系
Fig.11Dimensionless natural frequencies versus porosities
图12是在柔性梁参数为$\sigma = 1/100$, $\delta = 0.1$, $\zeta = 1$, $N = 2$, $n = 0$, $h_{\rm c} = h_{\rm m}=1/12$, $S_{\rm c} = S_{\rm m} = 1/2$时, 对于不同$\beta $的$\varpi $随着$\gamma $的变化曲线. 从图中可以看出, 用修正偶应力理论得出的固有频率与经典连续介质理论得出的固有频率有着显著的差异, 当柔性梁长度不断增大时, $\beta$不断变小, $\varpi $也在不断变小, $\beta $减小到对结构影响很小时, 修正偶应力理论可以退化为经典理论. 还可以发现, $\varpi_1 $和$\varpi _2 $均随着$\gamma $的增大而增大, $\beta $越小时, $\gamma $对$\varpi $的影响更为显著, 即曲线斜率随着$\gamma $的变化更为明显.
图12
新窗口打开|下载原图ZIP|生成PPT图12无量纲固有频率在不同$\beta $时与无量纲角速度的关系
Fig.12Dimensionless natural frequencies versus the dimensionless angular velocity for several values of $\beta $
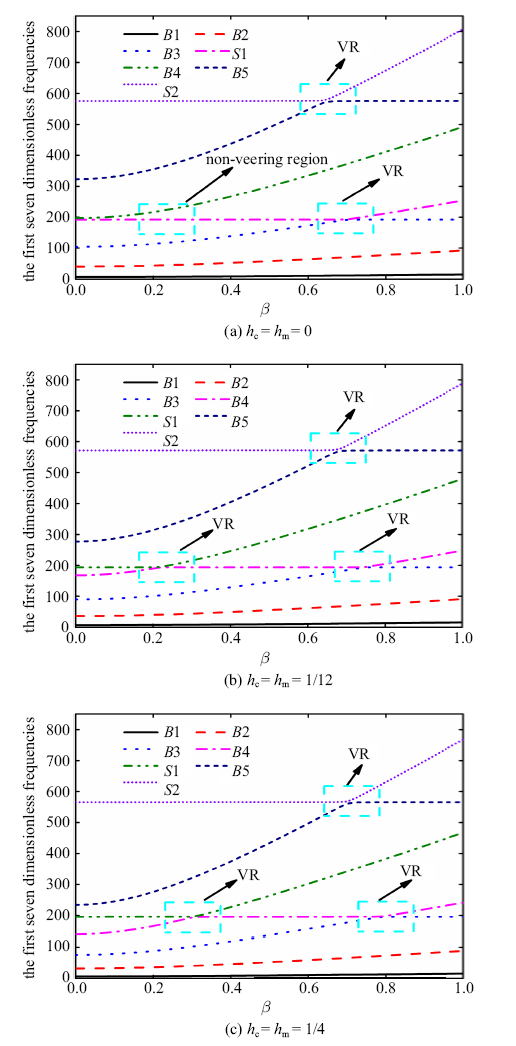
图13是在柔性梁参数为$\sigma =1/20$, $\delta = 0.1$, $\zeta = 1$, $\gamma = 8$, $N = 2$, $n = 0$, $S_{\rm c} = S_{\rm m} =1/2$时,前七阶无量纲固有频率随着$\beta $的变化曲线. 首先, 用$Be$表示第$e$阶横向弯曲振动频率, $e = 1,2,3,4,5$, $S_1 $和$S_2 $表示第一和第二阶拉伸振动频率. 从图中可以发现, 无量纲材料长度尺度参数$\beta $对弯曲主导频率尤其是高阶频率有更显著的影响, 但对拉伸主导频率几乎没有影响. 这是因为$\beta$只包含在弯曲刚度项$K_{33} $中, 在拉伸刚度项$K_{22} $中没有体现, 且弯曲刚度随着$\beta $的增大而增大. 此外, 当相邻频率轨迹接近时, 出现了图中所示的轨迹转向区域(veering region, VR), 由动力学方程可知, 轨迹转向现象是由轴向拉伸和横向弯曲运动的耦合作用引起的. 还可以发现, 图中轨迹转向现象不仅发生在相邻两阶不同模态频率之间(如$B$4与$S$1, $B$6与$S$2),在相邻两阶相同模态频率之间(如$B$3与$B$4)也会发生. 再者, 图13(a)中拉伸频率轨迹$S$1与横向弯曲频率轨迹$B$5之间没有出现轨迹转向区域, 随着$h_{\rm c}$, $h_{\rm m} $的变大(即柔性梁的近端高度$h$减小, 同时, FG层在总体积中的体积分数不断变小), 在图13(b)和图13(c)中, 系统的无量纲横向弯曲频率变小, 无量纲拉伸频率几乎不变, 导致S1与B5之间出现了轨迹转向区域, 且轨迹转向区域随着$h_{\rm c}$, $h_{\rm m} $的变大继续向右平移.
图13
新窗口打开|下载原图ZIP|生成PPT图13前七阶无量纲固有频率与无量纲尺度参数的关系
Fig.13The first seven dimensionless natural frequencies versus the dimensionless scale parameter
图14分别是对图13(b)中在无量纲材料长度尺度参数$\beta \approx 0.22$时的$B$4和$S$1频率轨迹转向处以及$\beta \approx 0.75$时的$B$3和$B$4频率轨迹转向处的局部放大图. 再分别对图14(a)中$\beta = 0.18$和$\beta = 0.26$处,以及图14(b)中$\beta = 0.7$和$\beta = 0.8$处的主导模态振型归一化如图15(a)和图15(b)所示. 从图中可以看出,当$0 \leqslant \beta < 0.22$时, $B$4和$S$1的模态振型分别由横向弯曲振动和拉伸振动主导, 当$0.22 \leqslant \beta <0.75$时, $B$4和$S$1对应的主导模态振型相互交换, 分别由拉伸振动和横向弯曲振动主导. 由于$B$4存在两次与相邻模态频率之间的转换, 因此, 当$\beta> 0.75$时, $B$4和$B$3分别由$0.22 < \beta < 0.75$的拉伸振动和横向弯曲振动主导模态振型转换成为由横向弯曲振动和拉伸振动主导模态振型, 此现象称为振型转换. 该现象进一步证明了旋转中心刚体-FGS楔形微梁的横向弯曲振动模态和纵向拉伸振动模态的耦合效应是导致频率轨迹转向的主要原因.
图14
新窗口打开|下载原图ZIP|生成PPT图14相邻两阶频率轨迹转向区域放大图
Fig.14Enlarged view of adjacent two-order frequency track veering regions
图15
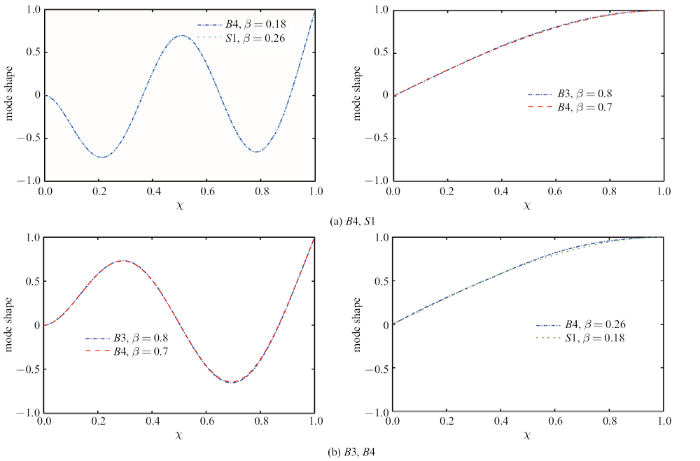新窗口打开|下载原图ZIP|生成PPT图15柔性梁的振型转换图
Fig.15Transforming diagram of the mode shapes of flexible beams
3 结论
本文基于修正偶应力理论, 建立了中心刚体-FGS楔形多孔微梁系统在大范围运动下的动力学方程, 研究了尺度参数、孔隙度等对系统的动力学特性的影响, 得到以下结论:(1) FGS楔形微梁相对于单一的功能梯度层结构的频率变大, 再者, 由于表层结构的存在, 使得FG层纯金属表面的强度更大, 不易被破坏, FG层纯陶瓷表面的隔热效果更好. 系统由于考虑了非线性应变和二阶非线性耦合项, 在较高速下较零次模型更加精确, 能够适用较大变形.
(2) FGS楔形微梁由于材料属性的不对称性, 导致应变能中横向与轴向的耦合势能不再为零, 使得系统在达到稳态后的平衡位置发生了偏移. FGS楔形微梁的表层结构高度变化中的情形1对动力学响应的影响较为明显, 情形2对动力学响应的影响较小.
(3) 当FGS楔形微梁的幂指数从零向正无穷变大时, 孔隙度对系统固有频率和动力学响应的影响也在变大. FGS楔形微梁相对于单一的功能梯度层结构, 当幂指数较小时, 系统固有频率由随着孔隙度的增大而减小转变为了随着孔隙度的增大而增大.
(4) 中心刚体-FGS楔形梁模型随着尺度参数的变化出现了频率转向现象, 随着楔形梁近端高度变大, 以及FG层在总体积中体积分数的变大, 频率转向区域会出现平移的现象, 使得楔形梁拉伸变形占主导的模态振型会提前出现.
本文研究结果对于在探索宏观领域到微观领域的过渡, 孔隙度对FGMs的影响程度, 以及如叶片和叶盘这类微观结构的振动测试点布置、故障分析、振型确定、平衡位置预测和稳态振幅激烈程度的判断都有一定的价值.
参考文献 原文顺序
文献年度倒序
文中引用次数倒序
被引期刊影响因子
DOIURLPMID [本文引用: 1]
Female genital mutilation or circumcision (FGM/C) is a perilous social and cultural practice that affects the physical, mental, and psychological health of affected women. It is widespread around the world, affecting 200 million women and girls. This study aimed to explore the relation of FGM/C to mental and physical conditions in Somali refugees displaced in a low-resource setting, applying the concept of poly-victimization to reveal multifaceted trauma sequelae. Data for this cross-sectional study with 143 female Somali youth living in Eastleigh, Kenya were collected between April and May in 2013. FGM/C was strongly associated with negative physical and mental health outcomes, including post-traumatic stress disorder and depressive, anxiety, and somatic symptoms. Logistic regression analysis revealed that separation from a parent and poly-victimization experiences were significantly associated with FGM/C experience. The results also showed that FGM/C and other traumas did not occur singly but were indicative of cumulative adversities, especially for women who were socially vulnerable and marginalized. The results of this study highlight the practice of FGM/C in the context of other adverse living conditions of refugees and the importance of attending to other co-occurring risk factors that prevail with FGM/C practice in the ecological system of refugee forced migration.
DOIURLPMID [本文引用: 1]
This work focuses on a titanium alloy implants incorporating a gradient of porosity, from the inner core to the outer surface, obtained by laser sintering of metal powder. Surface appearance, microstructure, composition, mechanical properties and fractography were evaluated.
[本文引用: 1]
[本文引用: 1]
DOIURL [本文引用: 2]
[本文引用: 1]
[本文引用: 1]
DOIURL [本文引用: 1]
DOIURL [本文引用: 1]
DOIURL [本文引用: 1]
[本文引用: 1]
DOIURL [本文引用: 1]
DOIURL [本文引用: 1]
Functionally graded materials are a type of composite materials which are tailored to provide continuously varying properties, according to specific constituent's mixing distributions. These materials are known to provide superior thermal and mechanical performances when compared to the traditional laminated composites, because of this continuous properties variation characteristic, which enables among other advantages, smoother stresses distribution profiles. Therefore the growing trend on the use of these materials brings together the interest and the need for getting optimum configurations concerning to each specific application.
In this work it is studied the use of particle swarm optimization technique for the maximization of a functionally graded sandwich beam bending stiffness. For this purpose, a set of case studies is analyzed, in order to enable to understand in a detailed way, how the different optimization parameters tuning can influence the whole process.
It is also considered a re-initialization strategy, which is not a common approach in particle swarm optimization as far as it was possible to conclude from the published research works. As it will be shown, this strategy can provide good results and also present some advantages in some conditions.
This work was developed and programmed on symbolic computation platform Maple 14. (C) 2013 Elsevier B.V.
[本文引用: 1]
[本文引用: 1]
DOIURL [本文引用: 1]
Buckling analysis of composite laminated beams is developed based on modified couple stress theory. By applying principle of minimum potential energy and considering two different beam theories, i.e., Euler-Bernoulli and Timoshenko beam theories, governing equations, boundary and initial conditions are derive for micro composite laminated beams. By using the new curvature tensor and modified couple stress theory, the size effects are captured unlike the classical continuum theories. A model of simply supported composite laminated beams is considered. These models can cover the classical composite beam theories and isotropic beam theories. Governing equations are solved by utilizing the Fourier series expansions. Comparison between results obtained by present study and those obtained by literature reveals that they are in good agreement. Some numerical results are presented to study the effect of material length scale parameter, beam thickness and length of beam on the micro composite laminated beam behavior. In addition, to investigate the effect of lamination on the buckling analysis of micro composite laminated beams, two types of lamination, i.e., [0, 90,0] and [90,0,90] are considered. (C) 2013 Elsevier Ltd.
DOIURLPMID [本文引用: 1]
In this paper we develop a theory for calculating the chemical energy liberation and heat production of a skeletal muscle subjected to an arbitrary history of stimulation, loading, and length variation. This theory is based on and complements the distribution-moment (DM) model of muscle [Zahalak and Ma, J. biomech. Engng 112, 52-62 (1990)]. The DM model is a mathematical approximation of the A. F. Huxley cross-bridge theory and represents a muscle in terms of five (normalized) state variables: A, the muscle length, c, the sarcoplasmic free calcium concentration, and Q0, Q1, Q2, the first three moments of the actin-myosin bond-distribution function (which, respectively, have macroscopic interpretations as the muscle stiffness, force, and elastic energy stored in the contractile tissue). From this model are derived two equations which predict the chemical energy liberation and heat production rates in terms of the five DM state variables, and which take account of the following factors: (1) phosphocreatine hydrolysis associated with cross-bridge cycling; (2) phosphocreatine hydrolysis associated with sarcoplasmic-reticulum pumping of calcium; (3) passive calcium flux across the sarcoplasmic-reticulum membrane; (4) calcium-troponin bonding; (5) cross-bridge bonding at zero strain; (6) cross-bridge strain energy; (7) tendon strain energy; and (8) external work. Using estimated parameters appropriate for a frog sartorius at 0 degree C, the energy rates are calculated for several experiments reported in the literature, and reasonable agreement is found between our model and the measurements. (The selected experiments are confined to the plateau of the isometric length-tension curve, although our theory admits arbitrary length variations.) The two most important contributions to the energy rates are phosphocreatine hydrolysis associated with cross-bridge cycling and with sarcoplasmic-reticulum calcium pumping, and these two contributions are approximately equal under tetanic, isometric, steady-state conditions. The contribution of the calcium flux across the electrochemical potential gradient at the sarcoplasmic-reticulum membrane was found to be small under all conditions examined, and can be neglected. Long-term fatigue and oxidative recovery effects are not included in this theory. Also not included is the so-called 'unexplained energy' presumably associated with reactions which have not yet been identified. Within these limitations our model defines clear quantitative interrelations between the activation, mechanics, and energetics in muscle, and permits rational estimates of the energy production to be calculated for arbitrary programs of muscular work.
[本文引用: 1]
[本文引用: 1]
DOIURL [本文引用: 1]
DOIURL [本文引用: 1]
DOIURL [本文引用: 1]
DOIURL [本文引用: 1]
[本文引用: 1]
[本文引用: 1]
[本文引用: 1]
[本文引用: 1]
[本文引用: 1]
DOIURL [本文引用: 1]
A comprehensive dynamic model of a rotating hub-functionally graded material (FGM) beam system is developed based on a rigid-flexible coupled dynamics theory to study its free vibration characteristics. The rigid-flexible coupled dynamic equations of the system are derived using the method of assumed modes and Lagrange's equations of the second kind. The dynamic stiffening effect of the rotating hub-FGM beam system is captured by a second-order coupling term that represents longitudinal shrinking of the beam caused by the transverse displacement. The natural frequencies and mode shapes of the system with the chordwise bending and stretching (B-S) coupling effect are calculated and compared with those with the coupling effect neglected. When the B-S coupling effect is included, interesting frequency veering and mode shift phenomena are observed. A two-mode model is introduced to accurately predict the most obvious frequency veering behavior between two adjacent modes associated with a chordwise bending and a stretching mode. The critical veering angular velocities of the FGM beam that are analytically determined from the two-mode model are in excellent agreement with those from the comprehensive dynamic model. The effects of material inhomogeneity and graded properties of FGM beams on their dynamic characteristics are investigated. The comprehensive dynamic model developed here can be used in graded material design of FGM beams for achieving specified dynamic characteristics. (C) 2013 Elsevier Ltd.
DOIURL [本文引用: 1]
DOIURL [本文引用: 1]
DOIURLPMID [本文引用: 1]
Active development of quantum informational components such as quantum computers and quantum key distribution systems requires parameter characterization of single photon detectors. A key property of the single photon detectors is detection efficiency. One of the methods of the detection efficiency measurement, as listed in the international standard ETSI, is the reference-free twin-photon-based Klyshko method. The signal-to-noise ratio (SNR) of this method depends on the combination of the pump wavelength, the nonlinear crystal's axis angle, and the type of detector's sensitive element. When the combination is difficult, one has to deal with the low SNR of the detector counts measurement. To gain the high SNR, one has to average the long record complicated with the &quot;random telegraph signal&quot; noise. This type of noise exhibits high spectral density at a zero frequency, where simple averaging works. The heterodyne based method we have proposed is to perform averaging at the higher frequency of the modulation introduced to the standard Klyshko measurement scheme. The method was numerically simulated and experimentally tested. The 14 times improvement in SNR for the proposed method relative to the simple averaging was demonstrated by the numerical simulation and confirmed experimentally.
[本文引用: 1]
[本文引用: 1]
[本文引用: 1]
[本文引用: 1]
DOIURL [本文引用: 1]
DOIURL [本文引用: 1]
Abstract
The deformation behavior of materials in the micron scale has been experimentally shown to be size dependent. In the absence of stretch and dilatation gradients, the size dependence can be explained using classical couple stress theory in which the full curvature tensor is used as deformation measures in addition to the conventional strain measures. In the couple stress theory formulation, only conventional equilibrium relations of forces and moments of forces are used. The couple's association with position is arbitrary. In this paper, an additional equilibrium relation is developed to govern the behavior of the couples. The relation constrained the couple stress tensor to be symmetric, and the symmetric curvature tensor became the only properly conjugated high order strain measures in the theory to have a real contribution to the total strain energy of the system. On the basis of this modification, a linear elastic model for isotropic materials is developed. The torsion of a cylindrical bar and the pure bending of a flat plate of infinite width are analyzed to illustrate the effect of the modification.DOIURL [本文引用: 1]
The geometrically nonlinear governing differential equations of motion and the corresponding boundary conditions are derived for the mechanical analysis of Timoshenko microbeams with large deflections, based on the strain gradient theory. The variational approach is employed to achieve the formulation. Hinged-hinged beams are considered as an important practical case, and their nonlinear static and free-vibration behaviors are investigated based on the derived formulation.
DOIURL [本文引用: 1]
对在平面内做大范围转动的中心刚体-柔性梁系统的刚柔耦合建模理论进行了深入研究,建立了系统的高次耦合动力学模型. 该动力学模型考虑了柔性梁横向弯曲变形和纵向伸长变形,且在纵向位移中计及由于横向变形而引起的纵向缩短项,即非线性耦合变形项,并保留了与非线性耦合项相关的一些高阶项,最终得到了系统的高次刚柔耦合动力学方程. 由此得到的动力学方程不仅能适用于柔性梁的小变形问题,也同样适用于大变形问题,弥补了一次近似耦合模型在处理柔性梁大变形问题上的不足. 通过与绝对节点坐标法以及一次近似耦合模型的对比验证了高次耦合模型的正确性.
DOIURL [本文引用: 1]
对在平面内做大范围转动的中心刚体-柔性梁系统的刚柔耦合建模理论进行了深入研究,建立了系统的高次耦合动力学模型. 该动力学模型考虑了柔性梁横向弯曲变形和纵向伸长变形,且在纵向位移中计及由于横向变形而引起的纵向缩短项,即非线性耦合变形项,并保留了与非线性耦合项相关的一些高阶项,最终得到了系统的高次刚柔耦合动力学方程. 由此得到的动力学方程不仅能适用于柔性梁的小变形问题,也同样适用于大变形问题,弥补了一次近似耦合模型在处理柔性梁大变形问题上的不足. 通过与绝对节点坐标法以及一次近似耦合模型的对比验证了高次耦合模型的正确性.
[本文引用: 2]
[本文引用: 2]
DOIURL [本文引用: 1]
DOIURL [本文引用: 2]
