浦俊, 卢东强^,2)上海大学 上海市应用数学和力学研究所, 上海 200072
上海市能源工程力学重点实验室, 上海 200072

HYDROELASTIC RESPONSE OF A SEMI-INFINITE PLATE DUE TO OBLIQUELY INCIDENT WAVES IN A THREE-LAYER FLUID¹⁾

Pu Jun, Lu Dongqiang^,2) Shanghai institute of applied mathematics and mechanics, Shanghai University, Shanghai 200072, China
Shanghai Key Laboratory of Mechanics in Energy Engineering, Shanghai 200072

通讯作者: 2) 卢东强, 研究员, 主要研究方向水波动力学. E-mail:dqlu@shu.edu.cn

收稿日期:2019-04-6接受日期:2019-05-9网络出版日期:2019-05-13

基金资助:

1) 国家自然科学基金资助项目.11872239

Received:2019-04-6Accepted:2019-05-9Online:2019-05-13
作者简介 About authors


摘要
解析地研究了在三层流体中斜入射波浪与半无限弹性板的相互作用引起的波散射和板的水弹性响应. 三层流体在界面处的密度发生阶跃, 各层为一常数. 假设流体不可压缩、无黏、流体运动无旋. 在线性势流理论框架下, 使用本征函数展开法和内积式给出波板相互作用的半解析解. 根据色散关系分析, 得到了表面波模态和界面波模态入射时的临界入射角. 随着物理参数的变化, 临界角将随之发生变化. 临界角决定了当由开阔水域向板覆盖水域传播的表面波或界面波的存在性: (1)板覆盖水域入射界面上, 透射波能否存在; (2)入射界面之上界面中, 板覆盖水域中的透射波以及开阔水域中的反射波能否存在. 当下界面波入射时并且入射角足够大时, 开阔水域中的下界面波模态是整个流体域中唯一存在的模态.
关键词： 三层流体;半无限弹性板;本征函数展开;斜入射

Abstract
Wave scattering and hydroelastic response of semi-infinite elastic plates due to obliquely incident waves in a three-layer fluid are studied analytically. The densities of the three layers of fluid are different with sharp interfaces, but are constant at each layer. The fluid is assumed to be inviscid and incompressible and the motion to be irrotational. Within the frame of the linear potential flow, semi-analytical solutions for wave-plate interaction are derived with the aid of the methods of matched eigenfunction expansions and the inner product of eigenfunction. The critical angles for the incident waves of the surface mode and the interfacial wave mode are deduced in terms of the dispersion relation. As the physical parameters change, the critical angle varies accordingly. The critical angle are closely related with the existence of the surface or interfacial wave modes that propagate from the open water region to the plate-covered one, by which two problems can be answered: (1) Is there a transmitted wave on the incident interface in the plate-covered region; (2) Is there, on the interfaces above the incident surface, a transmitted wave on the plate-covered region and a reflected wave in the open water region. When the low interfacial wave is incident and the incident angle is large enough, the low interfacial wave mode in the open-water region becomes the solo wave mode in the entire fluid domain.
Keywords：three-layer fluid;semi-infinite elastic plate;eigenfunction expansion;oblique incidence


PDF (5488KB)元数据多维度评价相关文章导出EndNote|Ris|Bibtex收藏本文
本文引用格式
浦俊, 卢东强. 三层流体中斜入射波作用下半无限板的水弹性响应¹⁾. 力学学报[J], 2019, 51(6): 1614-1629 DOI:10.6052/0459-1879-19-081
Pu Jun, Lu Dongqiang. HYDROELASTIC RESPONSE OF A SEMI-INFINITE PLATE DUE TO OBLIQUELY INCIDENT WAVES IN A THREE-LAYER FLUID¹⁾. Chinese Journal of Theoretical and Applied Mechanics[J], 2019, 51(6): 1614-1629 DOI:10.6052/0459-1879-19-081

引 言

超大型浮式结构物(very large floating structure, VLFS)作为充分利用海洋空间资源的新手段, 受到了众多****的关注, 并且逐渐开始向工程实用化发展. VLFS 的主要特点是它的水平尺度远大于垂直尺度, 使得它在波浪和移动载荷的影响下, 会出现较大的弹性形变. 因此流固耦合的水弹性响应是设计中不可或缺的要素^[1-4]. 为了简化, 一般将 VLFS 处理为板或梁模型进行分析. 此外, 极地区域超大块的浮冰在一定条件具有弹性力学性质, 也可视为一种 VLFS 模型. Squire ^[5] 建立了对冰区的水弹性响应的研究. 在国内有不少****研究VLFS的动力学行为, 例如闫红梅等 ^[6] 采用矩形平板的挠度格林函数进行超大型浮式结构物在波浪中的水弹性响应分析. 王志军等 ^[7] 利用三维线性水弹性理论以及 Bernoulli-Euler 梁解析解研究了箱式超大型浮体结构在正弦规则波中的动力响应. 刘超等 ^[8] 采用刚性模块柔性连接器模型, 比较了3种不同模型在7级海况作用下的连接器动力响应. 标识码卢东强^[9]借助Poincar$\acute{\textrm{e}}$-Lighthill-Kuo 方法, 研究了在薄冰层覆盖下不可压缩理想流体表面上传播两个非线性水弹性孤立波的迎面碰撞, 给出了三阶奇异摄动解的显式表达.

海洋中由于太阳辐射的影响, 在垂直结构上会出现密度、温度和盐度的分层, 其中强分层现象即为各层流体之间在界面处的物理量激烈变化, 对其进行建模可以使用密度均为常数的两层流体或三层流体模型, 上层流体密度小于下层流体. Linton 和Mclver ^[10] 基于线性水波理论的二维问题, 使用多极展开法求解单层和两层流体波浪与水平圆柱相互作用. Cadby 和Linton ^[11] 进一步研究了三维球体在单层和两层流体中与波浪的相互作用. Xu 和 Lu^[12] 根据两层流体中开阔水域垂向本征函数的正交性, 使用内积对方程进行简化, 研究了半无限弹性板和有限长度弹性板和水波的相互作用. Behera 等^[13] 使用边界元法研究两层流体中有限宽度的底部和表面穿孔多孔结构的倾斜表面重力波散射和俘获. Islam 和 Gayen ^[14] 基于线性理论使用超奇异积分方程研究了浸没在双层流体下层中的倾斜薄板引起的水波散射. Paul 和 De ^[15] 使用超奇异积分方程研究了双层流体中薄垂直渗透板对线性水波的二维散射. Rus$\mathring{a}$s 和 Grue ^[16]研究了界面对称孤立波在三层流体中的传播. 在三层流体中, 波振幅变得大于相应的两层流体, 其中忽略了密度的厚度, 而传播速度则相反. Das ^[17] 使用多级展开研究了三层流体中水平圆柱引起的波散射. Meng 和 Lu^[18] 使用本征函数展开研究了三层流体中有侧向内力的弹性板与波浪的相互作用.

水波与海洋结构物的相互作用中, 斜入射是相比正入射更为普遍. Fox 和 Squire ^[19] 使用本征函数展开和最小误差积分的方法, 分析了斜入射时海上浮冰对水波的反射和散射. Kim 等 ^[20] 得到了斜入射水波对半无限防波堤或中间带间隙防波堤模型的散射的解析解. Cho 和 Kim ^[21]研究了二维斜入射单色波和水下多孔板的相互作用,得到了不同的设计参数对浸没式水平多孔防波堤性能的影响. Lin 和 Lu^[22]使用本征函数展开法和 Xu 和 Lu ^[12] 提出的内积式, 研究了两层流体中斜入射水波对半无限弹性板的相互作用, 得到了不用参数对临界角的影响.

本文基于 Lin 和 Lu ^[22] 的工作, 将斜入射波浪与半无限弹性板的相互作用扩展到了三层流体的情形. 三层流体在表面和上下两个界面上都存在各自的一个行波模态, 它们的波数满足三层流体中的开阔水域和板覆盖水域的色散关系. 当入射频率给定, 由于板覆盖水域的波数小于自由水域的波数, 下层流体界面中的行进波波数大于上层流体界面中的行进波波数, 会出现斜入射波的临界入射角. 当入射角大于临界入射角, 会出现该行波模态的全反射现象.

1 数学模型

考虑有限深度三层流体中斜入射纯重力波与半无限长弹性板之间的水弹性相互作用. 选取三维直角坐标系, $z$ 轴垂直向上, $x$轴的正方向水平向右, $z=0$与静止时的自由水面重合, 如图1所示. $z=-h_1$, $z=-h_2$ 和 $z=-h_3$ 分别为三层流体之间的分界面和平坦刚性底面. 对于三层流体, 假设其不可压、无黏、运动无旋、各层密度为常数. 上层流体$(-h_1<z<0)$、中层流体$(-h_2<z<-h_1)$、下层流体$(-h_3<z<-h_2)$密度分别记为常数$\rho_1$, $\rho_2$, $\rho_3$. 弹性板是无间隙地漂浮在上层流体上面, 由$x=0$ 沿着$x$正方向至无穷远.整个流体域被板的边缘$x=0$ 分成两个半无限区域: 开阔水域$(-\infty<x<0)$和板覆盖水域$(0<x<+\infty)$.

图1

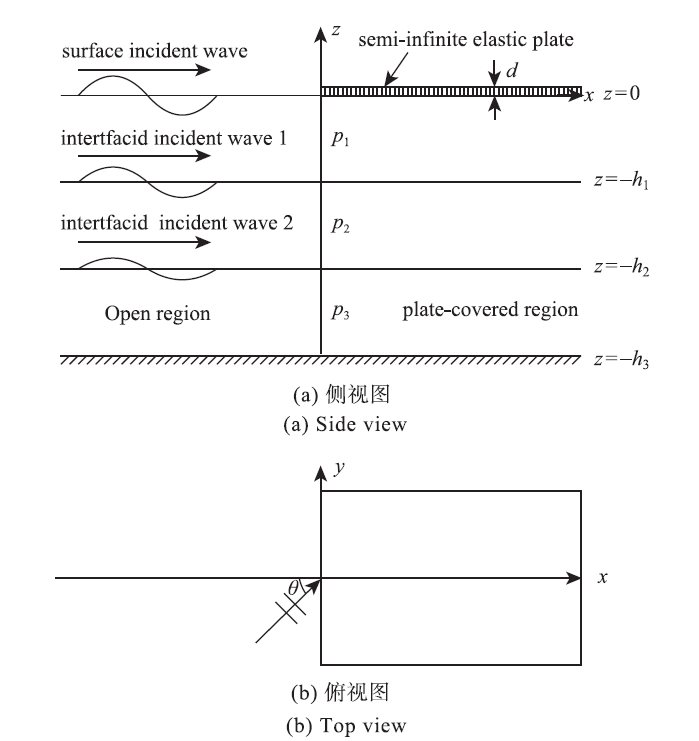新窗口打开|下载原图ZIP|生成PPT
图1三层流体中水波与半无限长弹性板相互作用的示意图

Fig.1Schematic diagram for the interaction between obliquely incident waves and a semi-infinite plate in a three-layer fluid



假设入射波仅从表面或者上下界面其中一个入射, 且系统处于稳恒周期态, 具有一个固定的频率$\omega$. 分离出速度势、表面和上下界面位移中的时间项, 得到速度势$\textrm{Re}[\phi_{m}(x,y,z) \textrm{e}^{-\textrm{i}\omega t }] (m=1,2,3)$, 表面位移$\textrm{Re}[\zeta_{1} (x,y) \textrm{e}^{-\textrm{i}\omega t }]$以及上下界面位移$\textrm{Re}[\zeta_{2} (x,y) \textrm{e}^{-\textrm{i}\omega t }]$和$\textrm{Re}[\zeta_{3} (x,y) \textrm{e}^{-\textrm{i}\omega t }]$, 其中$\phi_{m}(x,y,z)$是空间速度势; $m=1$, $m=2$和$m=3$分别表示上、中、下层流体的速度势;$\zeta_{m} (x,y)$分别是表面处($z=0$)以及上下两个界面处($z=-h_1$和$z=-h_2$) 的位移; $t$是时间.

空间速度势$\phi_m(x,y,z)$满足Laplace方程

(1) $\begin{align}\label{c_govening} & \left(\nabla^2+ \frac{\partial^2}{\partial z^2} \right) \phi_{m}=0\quad (-\infty<x<+\infty, -h_3<z<0)\end{align}$
在小振幅波稳态运动的假设下, 开阔水域中自由水面的线性运动学和动力学结合边界条件为

(2) $\begin{align} \label{c_surBC2} &-\omega^2\phi_{1}+g\frac{\partial\phi_{1}}{\partial z}=0\quad (-\infty<x<0, z=0) \end{align}$
其中$g$ 是重力加速度. 假设流体和弹性板是之间完全贴合没有间隙, 板覆盖水域的边界条件为

(3) $\begin{align} \label{c_surBC4} &-\rho_1\omega^2\phi_{1} + \left(D \nabla^{4} - M_{\textrm{e}}\omega^2+\rho_1 g\right)\cdot\notag\\ &\qquad \frac{\partial\phi_{1}}{\partial z}=0\quad (0<x<+\infty, z=0) \end{align}$
其中, $D=Ed^{3}/[12(1-\nu^{2})]$, $E$为杨氏模量, $\nu$为泊松比, 板的厚度$d$ , 板的单位面积上的质量$M_{\textrm{e}}=\rho_{\textrm{e}}d$, $\rho_{\textrm{e}}$为板的密度.

上中下层流体在界面上($z=-h_1$和$z=-h_2$)的法向速度和压强是连续的, 其线性的运动学和动力学条件可以写 为

(4) $\begin{align} \label{c_interBC1} &\frac{\partial\phi_{1}}{\partial z}=\frac{\partial\phi_{2}}{\partial z}=-\textrm{i} \omega \zeta_2\quad (z=-h_1)\\ \label{c_interBC2} \end{align}$
(5) $\begin{align}&\gamma_1 \left(\frac{\omega^2}{g}\phi_{1}-\frac{\partial\phi_{1}}{\partial z} \right)=\frac{\omega^2}{g}\phi_{2}-\frac{\partial\phi_{2}}{\partial z}\quad (z=-h_1)\\ \label{c_interBC3} \end{align}$
(6) $\begin{align}&\frac{\partial\phi_{2}}{\partial z}=\frac{\partial\phi_{3}}{\partial z}=-i \omega \zeta_{3}\quad (z=-h_2) \end{align}$
(7) $\begin{align}&\gamma_2 \left(\frac{\omega^2}{g}\phi_{2}-\frac{\partial\phi_{2}}{\partial z} \right)=\frac{\omega^2}{g}\phi_{3}-\frac{\partial\phi_{3}}{\partial z}\quad (z=-h_2) \end{align}$
其中$\gamma_n=\rho_n/\rho_{n+1},$ 并且 $0<\gamma_n<1 (n=1,2) $.

底部的非渗透边界条件为

(8) $\begin{align} \label{c_bottomBC} &\frac{\partial\phi_{3}}{\partial z}=0\quad (z=-h_3) \end{align}$
考虑到在$x=0$平面两侧水平速度和压力连续, 可以得到连续性条件为

(9) $\begin{align}&\phi_m(x,y,z)|_{x=0^-}=\phi_m(x,y,z)|_{x=0^+} \end{align}$
(10) $\begin{align}&\frac{\partial\phi_m(x,y,z)}{\partial x}\bigg|_{x=0^-}=\frac{\partial\phi_m(x,y,z)}{\partial x}\bigg|_{x=0^+} \end{align}$
这在求解过程中将作为匹配条件.

考虑在板的边缘($x=0, z=0$)有自由边界条件, 弯矩和等效剪力为零, 即

(11) $\begin{align} &\frac{\partial^2 \zeta}{\partial x^2}+\nu\frac{\partial^2 \zeta}{\partial y^2}=0 \end{align}$
(12) $\begin{align} &\frac{\partial^3 \zeta}{\partial x^3}+(2-\nu)\frac{\partial^3 \zeta}{\partial x\partial y^2}=0 \end{align}$

2 求解过程

从开阔水域倾斜入射的波动作用到弹性板边缘, 并透射到板覆盖水域, 水波的行波模态的波数发生变化, 在$x=0$ 处 发生折射现象. 假设开阔水域的波数和板覆盖水域的波数在$x=0$处沿着$y$ 方向上的分量相等.

在开阔水域, 行进波波数记为$k_{0_m} (m=1,2,3)$, 分别表示表面($m=1$)、上界面($m=2$)和下界面($m=3$)的波模态. 波动传播方向与$x$轴为$\theta_m$. 在$x$ 和$y$方向上的波数分量分别记为$\alpha_{0_m}=k_{0_m}\cos \theta_m$和$\beta_{0_m}=k_{0_m}\sin \theta_m$. 开阔水域中衰减波模态波数在$x$ 方向上的分量记为$\alpha_i$ $(i=1,2, \ldots)$.

板覆盖水域相应的各参数上方加“$\widetilde{ }$"表示, 以区别于开阔水域的参数. 在板覆盖水域, 行进透射波波数为为$\widetilde{k}_{0_m} (m=1,2,3)$. 模态传播方向为$\widetilde{\theta}_m$. 在$x$ 和$y$ 方向上的波数分量分别为$\widetilde{\alpha}_{0_m}=\widetilde{k}_{0_m}\cos \widetilde{\theta}_m$ 和 $\widetilde{\beta}_{0_m}=\widetilde{k}_{0_m}\sin \widetilde{\theta}_m$. 板覆盖水域中衰减波模态波数在$x$ 方向上的分量为$\widetilde{\alpha}_{j}$ $(j=\textrm{I},\textrm{II}, 1,2, \ldots)$.

Meng 和 Lu ^[18]导出了三层流体水弹性波动的色散关系的解析表达式. 对于一个给定频率$\omega$, 根据色散关系式可以得到$k_{0_m}$, $\widetilde{k}_{0_m}$, $\alpha_i$ $(i=1,2, \ldots)$ 和$\widetilde{\alpha}_{j}$ $(j=\textrm{I},\textrm{II}, 1,2, \ldots)$.

进一步, 我们假设表面波和界面波在$y$方向上具有相同的波数, 即

(13) $\begin{align} & \beta_{0_m}= \widetilde{\beta}_{0_m}= \beta \quad (m=1,2,3) \end{align}$
在开阔水域第$n$层面上$(n=1,2,3)$给定一入射波, 其入射角为$\theta_n$, 而$m=1,2,3$表示所要观测界面编号. 基于式(13), 可以通过入射波的波数和入射角, 确定其他界面和区域的波数和波向角. 即其他各层和各个区域的行波模态波数$k_{0_{m}}$和波向角$\theta_m$可以表示成

(14) $\begin{align} &\alpha_{0_m}=(k_{0_m}^2-\beta^2)^{1/2} \quad (m \neq n) \\ \end{align}$
(15) $\begin{align}&\widetilde{\alpha}_{0_m}=(\widetilde{k}_{0_m}^2-\beta^2)^{1/2} \quad (m=1,2,3) \end{align}$
(16) $\begin{align}&\tan \theta_m=\frac{\beta}{\alpha_{0_m}}=\frac{k_{0_n}\sin \theta_n} {(k_{0_m}^2-k_{0_n}^2\sin^2\theta_n)^\frac{1}{2}} \quad (m \neq n)\\ \end{align}$
(17) $\begin{align}&\tan \widetilde{\theta}_{m}=\frac{\beta}{\widetilde{\alpha}_{0_m}}= \frac{k_{0_n}\sin \theta_n}{(\widetilde{k}_{0_m}^2-\widetilde{k}_{0_n} ^2\sin^2\widetilde{\theta_n})^\frac{1}{2}} \quad (m=1,2,3) \end{align}$
假设在开阔水域第$n$层面上存在一入射波, 则入射势可以写作

(18) $\begin{align} &\phi^{\textrm{I}}(x,y,z)=I_{0_n}\textrm{e}^{\textrm{i}\alpha_{0_n}x} \textrm{e}^{\textrm{i}\beta y }Z_{0_n} \quad (-\infty<x<0, -h_3<z<0) \end{align}$
其中

(19) $\begin{align} &I_{0_n}=-\textrm{i}\omega \zeta_{0_n} \left[\frac{\partial V(k_{0_n},z) }{\partial z}\right]^{-1}_{z=0} \end{align}$
(20) $\begin{align} &Z_{0_{n}}=V(k_{0_{n}},z) \end{align}$
$V(k,z)$ 是垂向本征函数.

在三层流体中, $V(k,z)$ 为关于 $z$ 的分段函数^[18]. 对于 $-h_1<z<0$ 有

(21) $\begin{align} & V(k,z) = \dfrac{\cosh [k (h_2-h_1)] \cosh [k (h_3-h_2)] \cosh [k(z+h_1)]} {\gamma_1 \gamma_2 \cosh (k h_3)} \cdot\notag\\ &\qquad \left[ \varepsilon_1 ( \varepsilon_2 K t_3 - 1 ) K t_2 -\varepsilon_3 K t_3 + \gamma_2 t_2 t_3 +1 \right]+ \notag\\ &\qquad \dfrac{\cosh [k (h_2-h_1)] \cosh [k (h_3-h_2)] \sinh [k(z+h_1)]} {\gamma_2 \cosh (k h_3)}\cdot \notag\\ &\qquad (\gamma_2 t_3 + t_2 - \varepsilon_2 K t_2 t_3) \end{align}$
其中, $\varepsilon_1 = 1 - \gamma_1$, $\varepsilon_2 = 1 - \gamma_2$, $\varepsilon_3 = 1 - \gamma_1 \gamma_2$, $K=gk/\omega^2$, $t_m = \tanh k h_m$ 且$m=1,2,3$.

对于 $-h_2<z<-h_1$, 有

(22) $\begin{align} &V(k,z) = \dfrac{\cosh [k (h_3-h_2)]\cosh [k(z+h_2)]}{\gamma_2 \cosh (k h_3)} (1 - \varepsilon_2 K t_3)+\notag\\ &\qquad \dfrac{\sinh[ k (h_3-h_2)] \sinh[ k(z+h_2)]}{\cosh (k h_3)} \end{align}$
对于 $-h_3<z<-h_2$, 有

(23) $\begin{align} V(k,z) = &\dfrac{\cosh [k(z+h_3)]}{\cosh (k h_3)} \end{align}$
入射波的能量一部分透过了界面在板覆盖水域继续向$x$正方向传播, 一部分会在界面处反射回开阔水域向$x$ 负方向传播. 速度势在开阔水域$(-\infty<x<0)$由入射势$\phi^{\textrm{I}}$ 和反射势$\phi^{\textrm{R}}$组成, 在板覆盖水域$(0<x<+\infty)$ 只有透射势$\widetilde{\phi}$. 根据本征函数展开法, 速度势可以展开成

(24) $\begin{align} & \phi(x,y,z)=\phi^{\textrm{I}}(x,y,z)+\phi^{\textrm{R}}(x,y,z), \quad (-\infty<x<0) \end{align}$
(25) $\begin{align}&\phi^{\textrm{R}}(x,y,z) =\left( \sum_m R_{0_m}\textrm{e}^{-\textrm{i}\alpha_{0_m}x} Z_{0_m} +\sum_{i}R_{i} \textrm{e}^{\alpha_{i}x}Z_{i} \right) \textrm{e}^{\textrm{i}\beta y } \notag\\ &\qquad (-\infty<x<0) \end{align}$
(26) $\begin{align} &\widetilde{\phi}(x,y,z) =\left(\sum_m T_{0_m} \textrm{e}^{\textrm{i} \widetilde{\alpha}_{0_m}x}\widetilde{Z}_{0_m} + \sum_{j} T_{j} \textrm{e}^{ - \widetilde{\alpha}_{j }x} \widetilde{Z}_{j}\right) \textrm{e}^{\textrm{i}\beta y } \notag\\ &\qquad (0<x<+\infty) \end{align}$
其中$R_{0_m}$, $R_{i}$, $T_{0_m}$, $T_{j}$ $(m=1,2,3; i=1,2,\ldots; j=\textrm{I},\textrm{II}, 1,2, \ldots)$ 是待定展开系数, 且

(27) $\begin{align} \big\{Z_{0_{m}},Z_i,\widetilde{Z}_{0_{m}},\widetilde{Z}_j \big\} =\left\{ V(k_{0_{m}},z),V({i} k_i,z),V(\widetilde{k}_{0_{m}},z),V({i} \widetilde{k}_j,z) \right\} \end{align}$
将板覆盖水域速度势$\widetilde{\phi}(x,y,z)$代入板的自由边界条件(11)和(12)

(28) $\begin{align} &\sum_m \left(\widetilde{\alpha}_{0_m}^2 + \nu \beta^2 \right)T_{0_m} \frac{\partial \widetilde{Z}_{0_m}}{\partial z} + \sum_{j} \left(\nu \beta^2-\widetilde{\alpha}_{j}^2 \right)T_{j} \frac{\partial \widetilde{Z}_{j}}{\partial z} =0\\ \end{align}$
(29) $\begin{align} & \sum_m \widetilde{\alpha}_{0_m}\left[\widetilde{\alpha}_{0_m}^2 +(2-\nu) \beta^2 \right]T_{0_m} \frac{\partial \widetilde{Z}_{0_m}}{\partial z} +\notag\\ &\qquad \textrm{i} \sum_{j}\widetilde{\alpha}_{j}\left[(2-\nu) \beta^2- \widetilde{\alpha}_{j}^2 \right]T_{j} \frac{\partial \widetilde{Z}_{j}}{\partial z}=0 \end{align}$
假设表面波模态入射, 此时,入射速度势 $\phi^{\textrm{I}}$ 为式(18) 的形式.将速度势(24)$\sim$(26)代入到匹配条件 (9) 和(10)

(30) $\begin{align} & I_{0_1}Z_{0_1} + \sum_m R_{0_m} Z_{0_m} + \sum_{i}R_{i} Z_{i} = \sum_m T_{0_m} \widetilde{Z}_{0_m} + \sum_{j} T_{j} \widetilde{Z}_{j}\\ \end{align}$
(31) $\begin{align} &\alpha_{0_1} I_{0_1} Z_{0_1} - \sum_m \alpha_{0_m}R_{0_m}Z_{0_m} - \textrm{i}\sum_{i}\alpha_{i}R_{i} Z_{i} = \notag\\ &\qquad \sum_m \widetilde{\alpha}_{0_m}T_{0_m}\widetilde{Z}_{0_m} +\textrm{i}\sum_{j}\widetilde{\alpha}_{j} T_{j} \widetilde{Z}_{j} \end{align}$
参照 Meng 和 Lu ^[18] 的做法, 为了简化计算, 利用自由水域垂向本征函数作内积匹配关系. 对于自由水域, 内积具有如下定义形式

(32) $\begin{align} P_{il} = & \int_{-h_3}^{-h_2} Z_i \cdot Z_l {d}z + \gamma_2 \int_{-h_2}^{-h_1} Z_i \cdot Z_l {d}z + \notag\\ &\qquad \gamma_1 \gamma_2 \int_{-h_1}^0 Z_i \cdot Z_l {d}z \end{align}$
式中下标 $i,l=0_1,0_2,0_3,1,2,\cdots$. 容易验证, 在式 (32)定义下, 垂向本征函数族存在正交性, 即 $P_{il}=0$ (当 $i \ne l$ 时). 对板覆盖水域与开阔水域的垂向本征函数作内积, 内积具有如下形式

(33) $\begin{align} Q_{ql} =& \int_{-h_3}^{-h_2} \widetilde{Z}_q \cdot Z_l {d}z + \gamma_2 \int_{-h_2}^{-h_1} \widetilde{Z}_q \cdot Z_l {d}z +\notag\\ &\qquad \gamma_1 \gamma_2 \int_{-h_1}^0 \widetilde{Z}_q \cdot Z_l {d}z \end{align}$
其中 $q=0_1,0_2,0_3,\rm{I},\rm{II},1,2,\cdots$.

将开阔水域垂向本征函数$Z_{l}(z)(l=0_1,0_2,0_3,1,$ $2,\ldots)$ 对式(30) 的两端做内积

(34) $\begin{align} &(I_{0_1} + R_{0_1})P_{0_10_\textrm{1}} = \sum_m T_{0_m} Q_{0_m 0_1} + \sum_{j} T_{j} Q_{j 0_\textrm{1}}\\ \end{align}$
(35) $\begin{align} &R_{0_2}P_{0_20_\textrm{2}} = \sum_m T_{0_m} Q_{0_m 0_2} +\sum_{j} T_{j} Q_{j 0_\textrm{2}}\\ \end{align}$
(36) $\begin{align} &R_{0_3}P_{0_30_\textrm{3}} = \sum_m T_{0_m} Q_{0_m 0_3} +\sum_{j} T_{j} Q_{j 0_\textrm{3}}\\ \end{align}$
(37) $\begin{align} &R_iP_{i i} = \sum_m T_{0_m} Q_{0_m i} + \sum_{j} T_{j} Q_{j i}\quad (i=1,2,\ldots) \end{align}$
用本征函数$Z_{l}(z) (l=0_1,0_2,1,2,\ldots)$分别对式(31)作内积, 可得

(38) $\begin{align} &\alpha_{0_1}(I_{0_1} - R_{0_1})P_{0_10_\textrm{1}}= \sum_m \widetilde{\alpha}_{0_m}T_{0_m}Q_{0_m 0_1}+\textrm{i}\sum_{j}\widetilde{\alpha}_{j} T_{j} Q_{j 0_\textrm{1}} \end{align}$
(39) $\begin{align} &-\alpha_{0_2}R_{0_2}P_{0_20_\textrm{2}} =\sum_m \widetilde{\alpha}_{0_m}T_{0_m}Q_{0_m 0_2}+\textrm{i}\sum_{j}\widetilde{\alpha}_{j_2} T_{j_2} Q_{j 0_\textrm{2}} \end{align}$
(40) $\begin{align} &-\alpha_{0_3}R_{0_3}P_{0_30_\textrm{3}} =\sum_m \widetilde{\alpha}_{0_m}T_{0_m}Q_{0_m 0_3}+\textrm{i}\sum_{j}\widetilde{\alpha}_{j_3} T_{j_3} Q_{j 0_\textrm{3}}\\ \end{align}$
(41) $\begin{align} &-\textrm{i}\alpha_i R_i P_{ii} =\sum_m \widetilde{\alpha}_{0_m}T_{0_m}Q_{0_mi} +\textrm{i}\sum_{j}\widetilde{\alpha}_{j} T_{j} Q_{j i}\notag\\ &\qquad (i=1,2,\ldots) \end{align}$
令无限求和项$\sum_{i}$和$\sum_{j}$的项数分别为 $i=M$和 $j=M+2$, 即截断衰减模态项数为$M$. 联立板的边界条件(28) 和(29), 波板交界面的连续性条件(34)$\sim$(37) 和(38)$\sim$(41), 我们将得到$2M+6$为方程组, 对应于$2M+6$个未知数, $R_{0_m}$, $R_i$, $T_{0_m}$, $T_j$, 其中 $m=1,2,3$, $i=1,2,\ldots,M$, $j=\textrm{I},\textrm{II},1,2,\ldots,M$.

3 数值结果

3.1 无量纲化与验证能量守恒

以三层流体的总深度$h_3$, 重力加速度 $g$ 和上层流体的密度$\rho_1$为基本量, 对方程各个物理量进行无量纲化

(42) $\begin{align} &\left.{\begin{array}{l} \left\{\hat{x},\hat{y},\hat{z},\hat{\zeta_{0_1}},\hat{\zeta_{0_2}},\hat{\zeta_{0_3}},\hat{h},\hat{d}\right\}=\\\ \qquad \frac{1}{h_3} \left\{x,y,z,\zeta_{0_1},\zeta_{0_2},\zeta_{0_3},h,d \right\} \\\ \hat{k}=kh_3, \hat{\omega}=\omega\sqrt{\frac{h_3}{g}}, \hat{\rho}_\textrm{e}=\frac{\rho_\textrm{e}}{\rho_1}\\ \hat{D} =\frac{D}{\rho_1gh_3^4}, \hat \phi=\frac{\phi}{h_3\sqrt{g h_3}}\end{array}}\right\} \end{align}$
为了表达简洁, 本章的后续部分将省略符号上的帽子来表示无量纲量.

根据运动学边界条件, 在某一时刻的表面波$(n=1)$和界面波$(n=2,3)$的波形$\zeta_n$表示为

(43) $\begin{align} &\zeta_n(x,y)=\frac{\textrm{i}}{\omega}\frac{\partial\phi_n(x,y,-h_{n-1})}{\partial z} \end{align}$
其中$h_0=0$.表面波$(n=1)$和界面波$(n=2, 3)$的波幅$A_n$表示为

(44) $\begin{align} & A_n=\left\|\zeta_n(x,y) \right\| \end{align} $
为了验证方法的正确性和收敛性,需要验证开阔水域和板覆盖水域的交界处的波能流守恒. 波能流被定义为

(45) $\begin{align} \varepsilon_f=\overline{\int_{-h_3}^{\zeta_1}p u \textrm{d}z} \cong-\rho\int_{-h_3}^{\zeta_{0_1}}\overline{\frac{\partial\varPhi}{\partial t} \frac{\partial\varPhi}{\partial x} } \textrm{d}z \end{align}$
其中符号上横线表示对一个周期的时间求平均, $p$, $u$ 和 $\rho$ 分别是动压力、水平方向的速度和流体的密度. 三层流体的波能流守恒表示成

(46) $\begin{align} &E=\widetilde{E}\\ \end{align} $
(47) $\begin{align} &E=-\sum_{m=1}^3\rho_m\int_{-h_{m-1}}^{-h_m}\textrm{Re}\left[ \frac{\partial\varPhi_m}{\partial t}\left(\frac{\partial\varPhi_m}{\partial x}\right)^* + \left(\frac{\partial\varPhi_m}{\partial t}\right)^* \frac{\partial\varPhi_m}{\partial x} \right] \textrm{d}z \\ \end{align}$
(48) $\begin{align} &\widetilde{E}=-\sum_{m=1}^3\rho_m\int_{-h_{m-1}}^{-h_m}\textrm{Re}\left[ \frac{\partial\widetilde{\varPhi}_m}{\partial t}\left(\frac{\partial\widetilde{\varPhi}_m}{\partial x}\right)^* + \left(\frac{\partial\widetilde{\varPhi}_m}{\partial t}\right)^* \frac{\partial\widetilde{\varPhi} _m}{\partial x} \right] \textrm{d}z \end{align}$
其中$E$ 和 $\widetilde{E}$ 分别是开阔水域和板覆盖水域的波能流表达式.

3.2 表面波模态入射

以下计算中, 板的各参数取值为$\nu=0.3$, $\rho_\textrm{e}=0.9$, $E=1092$; 除非特别标示, 一般取$d =0.02$.

当表面波模态从开阔水域向板覆盖水域入射时, 参数选取为$M=7$, $2 h_1=h_2=0.4$, $\gamma_1=\gamma_2=0.9$, $\zeta_{0_1}=0.01$, $\omega=1.25$, $\theta_1=45^\circ$, 得到相对的能量误差为

(49) $\begin{align} \frac{|E - \widetilde{E}|}{\min[E,\widetilde{E}] } \approx 0.152\% \end{align}$
图2 显示了关于截断衰减模态项数$M$对计算结果收敛性的影响. 消散模态截断数从$M=1$ 到$M=7$, 3个界面的图形变化都很小, 高阶的衰减模态对计算结果的影响较小. 当$M\geq 5$, 曲线几乎不再变化.本文后续图形均是在 $M=7$ 的情形下得到的.

图2

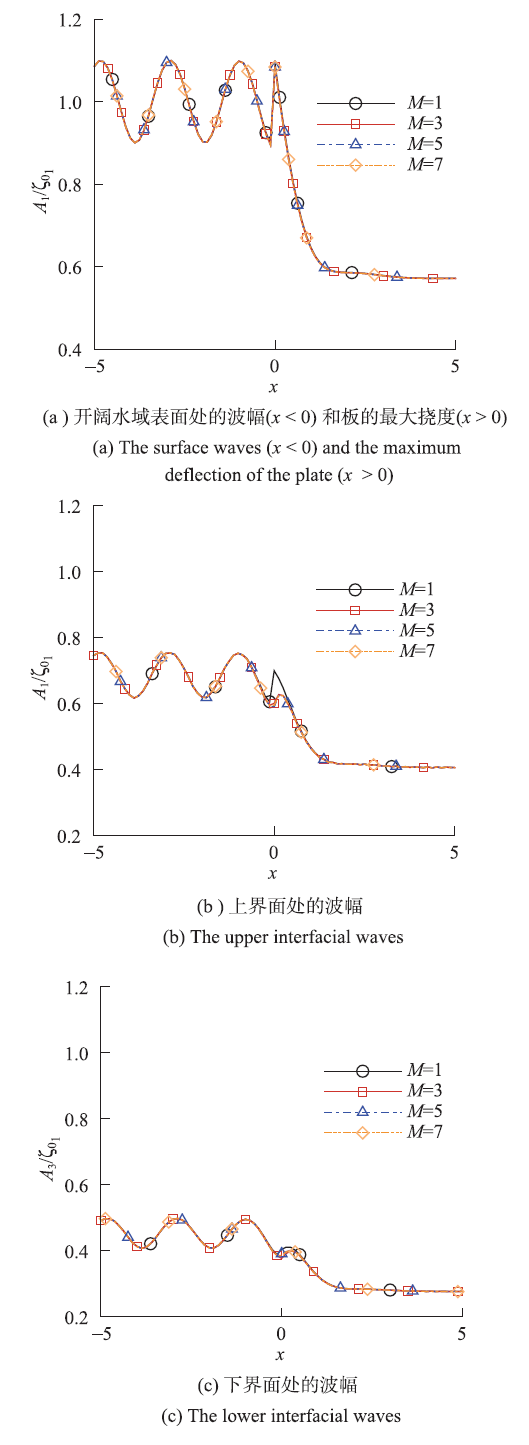新窗口打开|下载原图ZIP|生成PPT
图2当表面波模态入射时, 不同消散模态项数$M$下$A_{\textrm{1}}$, $A_{\textrm{2}}$和$A_{\textrm{3}}$随$x$的变化

Fig.2The amplitudes of the gravity waves and the hydroelastic deflection of the plate for different $M$ in the case of the incident waves of the surface wave mode



当表面波入射时, 折射角 $\widetilde{\theta}_1$ 将会随着入射角 $\theta_1$ 的增大而增大. 在$k_{0_1}>\widetilde{k}_{0_1}$的情况下, 当$\sin\widetilde{\theta}_1=1$ 时, 将会存在一个临界角$\theta_{1,1}$

(50) $\begin{align} \theta_{1,1}=\arcsin(\widetilde{k}_{0_1}/k_{0_1}) \end{align}$
当入射角$\theta_1>\theta_{1,1}$ 时, 板覆盖水域的表面波会在板边缘发生全反射, 在板覆盖区域将不出现透射的表面波模态. 入射角的变化特别是在临界角附近会显著影响弹性板在波浪载荷下的响应. 各物理参数会显著影响临界角的大小, 三维斜入射问题中需要着重考虑临界角的变化.

图3 显示了表面波入射时不同的入射角 $\theta_1$ 下的三维波形图, 图4 显示相应的波幅, 其中$\zeta_{0_1}=0.01$, $2 h_1=h_2=0.4$, $\gamma_1=\gamma_2=0.9$, 此时临界角$\theta_{1,1}\approx54.39^\circ$. 当$\theta_1<\theta_{1,1}$, 如图中$\theta_1=15^\circ, 30^\circ, 45^\circ$, 波浪从开阔水域到板覆盖水域的过程中, 波浪在两个区域的交界处发生折射, 在两个区域内波数 $k_{0_1}>\widetilde{k}_{0_1}$, 而波数在 $y$ 方向上的分量 $\beta$ 相同. 除了发生折射的透射波, 还有部分能量在交界处被反射, 与入射波发生干涉. 在交界面上可以发现, 板的挠度在边缘最大, 然后逐渐过渡到了水平状态. 而当$\theta_1>\theta_{1,1}$, 如图中$\theta_1=60^\circ$, 板覆盖水域各层的波幅都变为$0$. 此时发生了表面波的全反射, 在板覆盖水域各层的波幅在过渡区域之后都很小, 能量几乎被全部反射. 比较图4 中各界面处波幅, 可以发现表面波和界面波的波长接近相等, 说明了此时界面中传播的主要是表面波的模态, 能量转移到界面波模态的很少. 3个界面的波幅从上至下依次递减.

图3

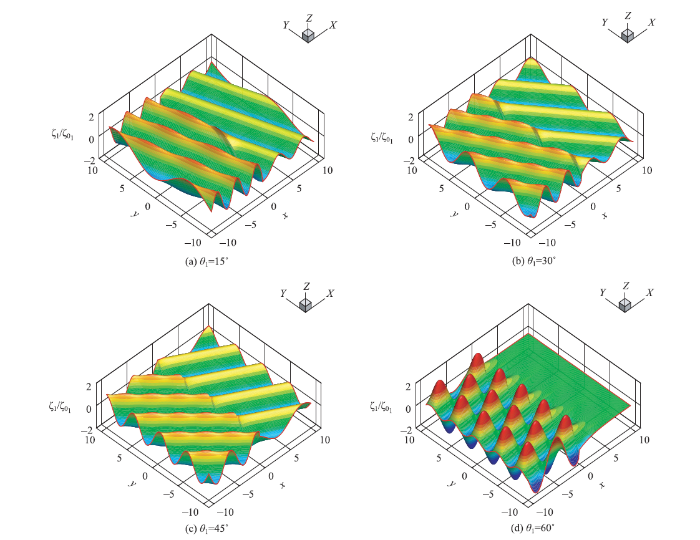新窗口打开|下载原图ZIP|生成PPT
图3当表面波模态入射时, 不同表面入射角$\theta_1$下表面处重力波的波形($x<0$)和板的挠度 ($x>0$)的三维图

Fig.3The three-dimensional profiles of the surface gravity waves ($x<0$) and the hydroelastic deflection of the plate ($x>0$) for different $\theta_1$ in the case of the incident waves of the surface wave mode

图4

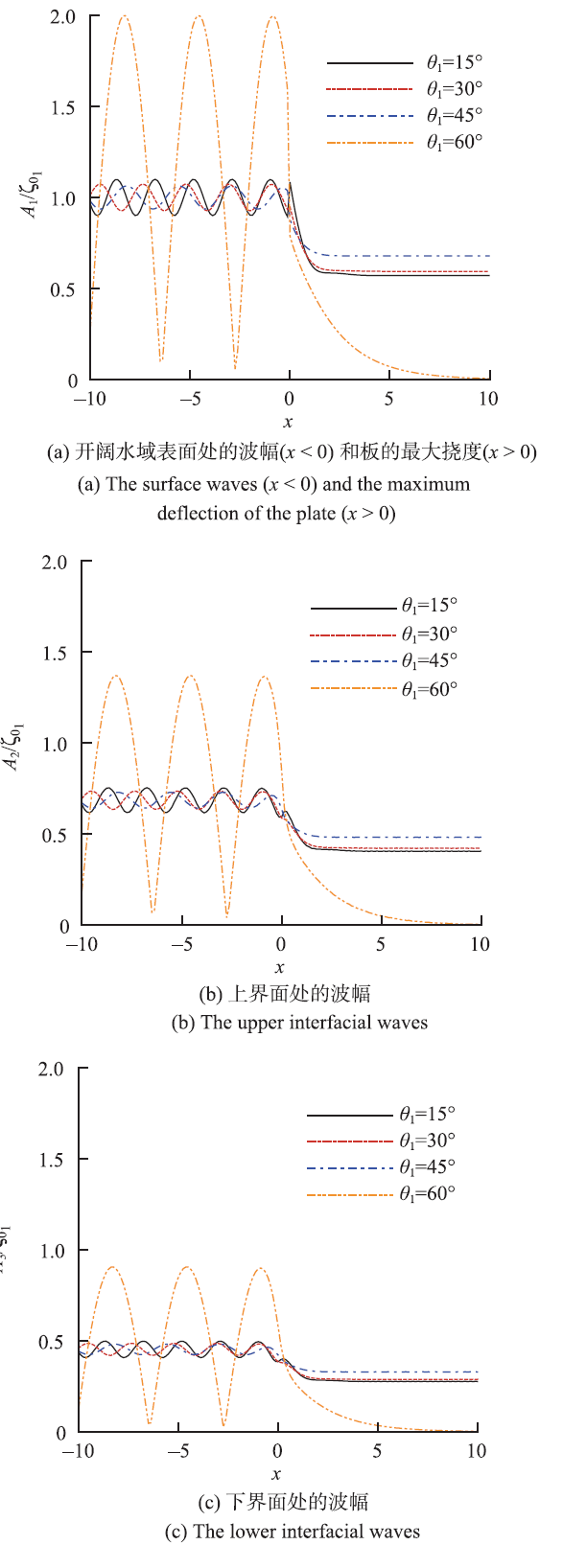新窗口打开|下载原图ZIP|生成PPT
图4当表面波模态入射时, 不同表面入射角$\theta_1$下$A_{\textrm{1}}$, $A_{\textrm{2}}$和$A_{\textrm{3}}$ 随$x$的变化

Fig.4The amplitudes of the gravity waves and the hydroelastic deflection of the plate for different $\theta_1$ in the case of the incident waves of the surface wave mode



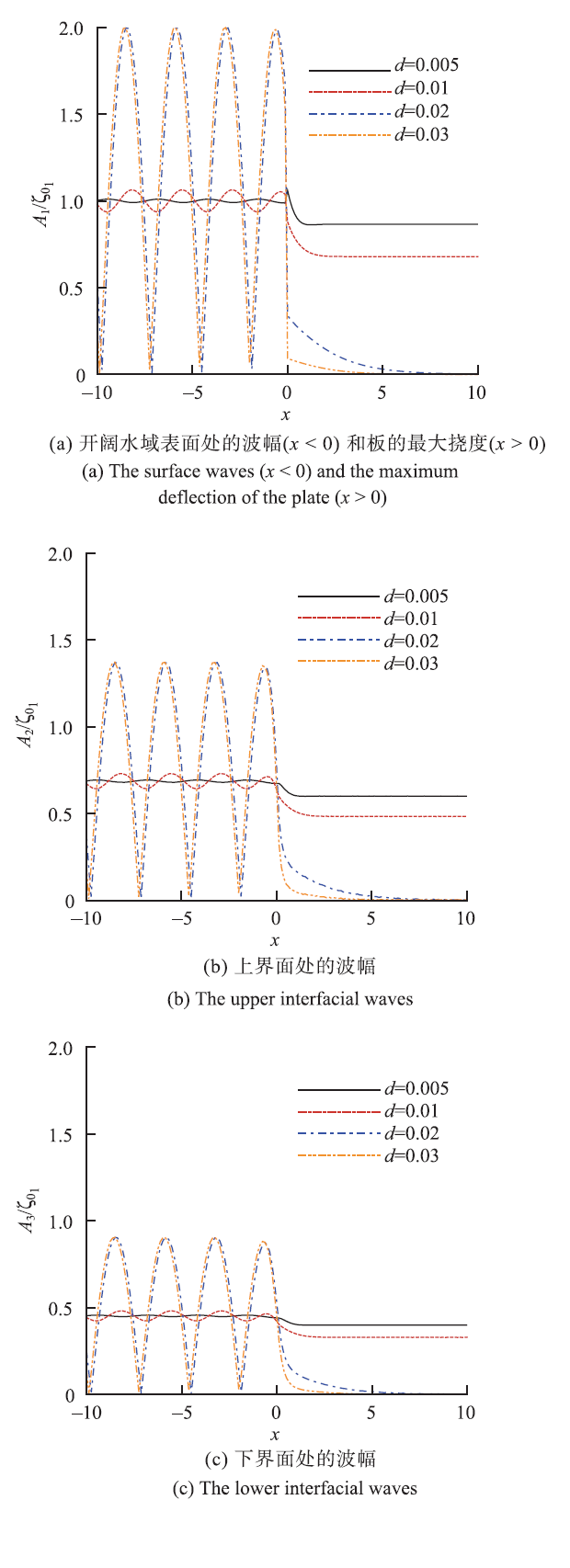
接着, 我们分析在表面波模态入射的情况下不同的物理量变化对水弹性响应的影响, 其中$\zeta_{0_1}=0.01$, $\theta_1=45^{\circ}$. 图5显示了当取板厚度 $d$不同时, 表面和界面处波形的变化, 其中$2 h_1=h_2=0.4$, $\gamma_1=\gamma_2=0.9$. 随着板的厚度增大, 板覆盖水域的各界面透射波波幅减小, 相比之下, 开阔水域的各界面反射波波幅增大. 由于板的厚度增大, 导致板覆盖水域的波数减小, 因而临界角$\theta_{1,1}$ 减小, 当$\theta_{1,1}<\theta_1$ 后, 如图中$d=0.02$和$d=0.03$时, 入射波的模态出现了全反射.

图5

新窗口打开|下载原图ZIP|生成PPT
图5当表面波模态入射时, 不同板厚度$d$下$A_{\textrm{1}}$, $A_{\textrm{2}}$和$A_{\textrm{3}}$ 随$x$ 的变化

Fig.5The amplitudes of the gravity waves and the hydroelastic deflection of the plate for different $d$ in the case of the incident waves of the surface wave mode



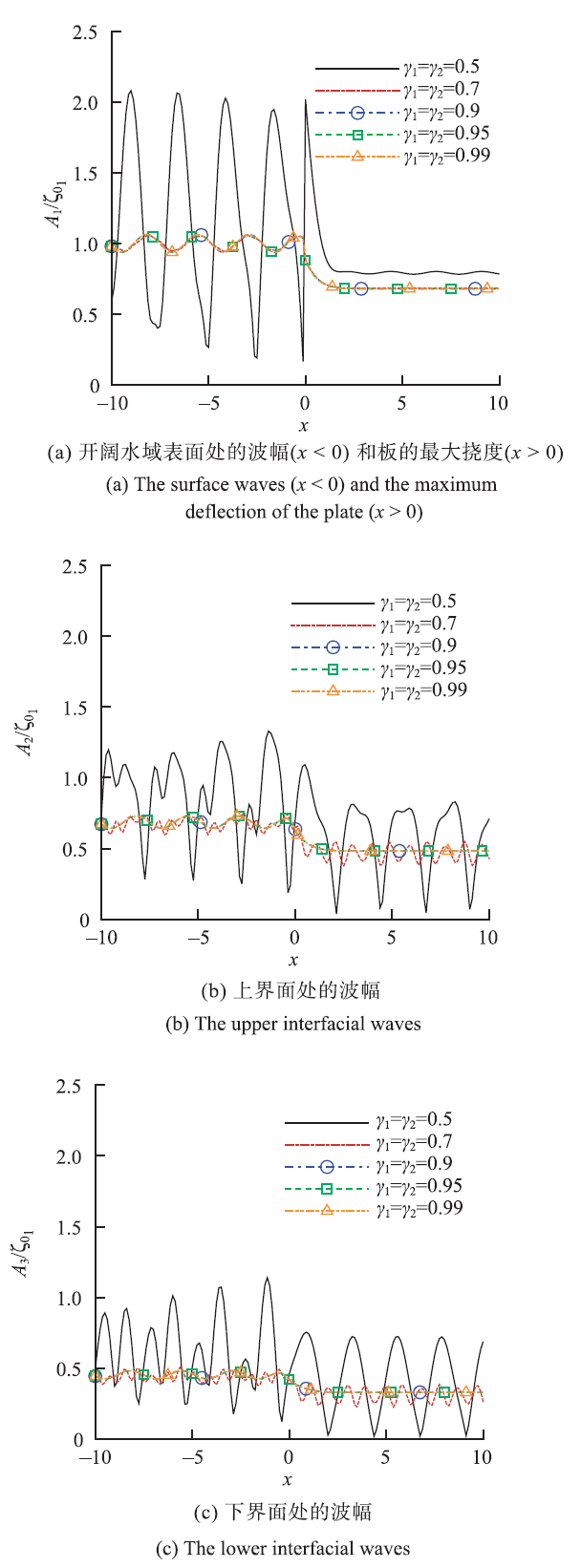
图6 显示了各层流体间密度比$\gamma=\gamma_1=\gamma_2$取不同值时, 表面和界面处波形的变化, 其中$2 h_1=h_2=$$0.4$. 如图中取$\gamma=0.7$ 时, $\gamma$的改变对表面波的影响非常小, 但是在两个界面上会激发出新的模态, 由于有界面波模态被激发, 界面波波形变得十分粗糙. 而$\gamma=0.5$ 时, 在表面和界面上都会明显激发出新的模态, 波长和波幅都有大幅变化. 当$\gamma=0.95$ 或$\gamma=0.99$时, 在表面和界面上的波幅与$\gamma=0.9$相比差距非常小, 与实际物理情况相符合.

图6

新窗口打开|下载原图ZIP|生成PPT
图6当表面波模态入射时, 不同密度比$\gamma$下$A_{\textrm{1}}$, $A_{\textrm{2}}$和$A_{\textrm{3}}$随$x$的变化

Fig.6The amplitudes of the gravity waves and the hydroelastic deflection of the plate for different $\gamma$ in the case of the incident waves of the surface wave mode



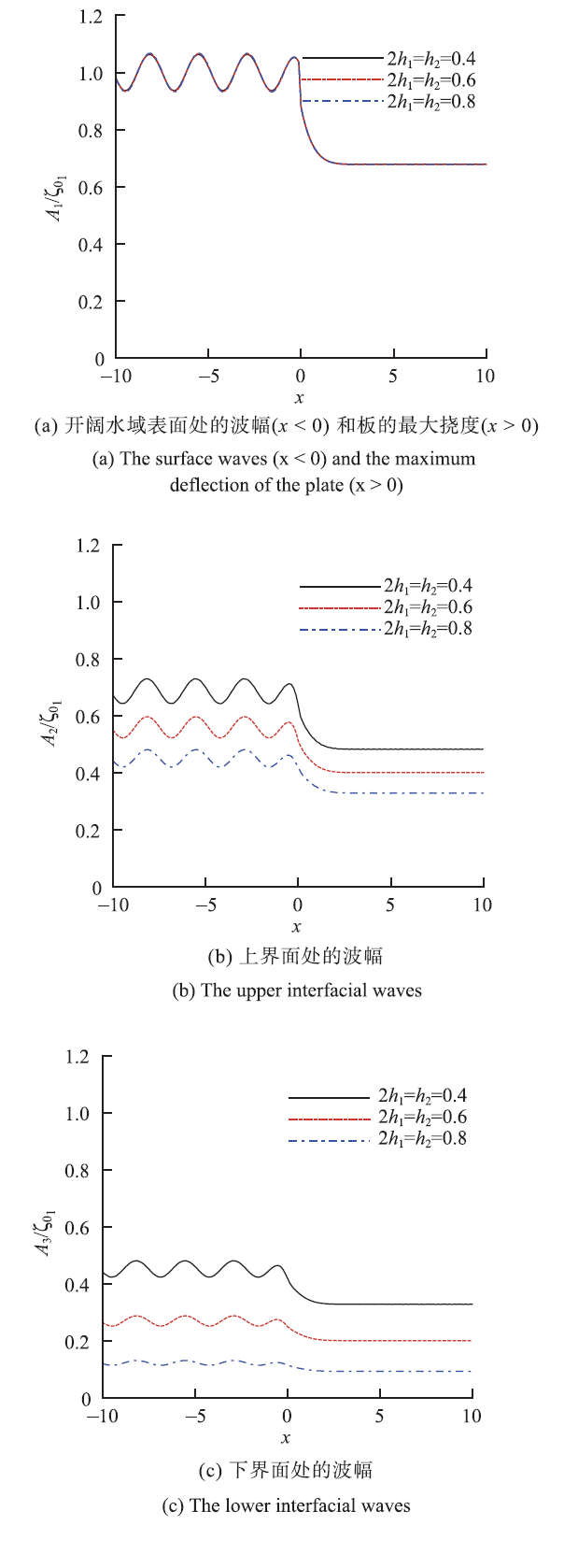
图7 显示了各层流体占据不同的深度比 $h_1$ 和 $h_2$ 下, 表面和界面处波形的变化, 其中$\gamma_1=\gamma_2=0.9$. 在图7 中, 上两层流体具有相同的深度比, 即$2 h_1=h_2$. $h$ 的变化并不显著影响表面和界面的波长, 且对表面波的波幅几乎没有影响, 仅仅会影响两个界面波的波幅. 随着上两层深度比的增大, 两个界面波的波幅减小. 也就是说, 当改变深度比后, 两个界面波幅仅与其所在的空间位置有关, 相互之间影响很小.

图7

新窗口打开|下载原图ZIP|生成PPT
图7当表面波模态入射时, 不同深度比$h$下$A_{\textrm{1}}$, $A_{\textrm{2}}$和$A_{\textrm{3}}$ 随$x$ 的变化

Fig.7The amplitudes of the gravity waves and the hydroelastic deflection of the plate for different $h$ in the case of the incident waves of the surface wave mode



使用薄板理论, 计算板的受力状态和应力状态

(51) $\begin{align} &M_x(x,y)=\left\|-D \left( \frac{\partial^2 \zeta_1}{\partial x^2}+\nu\frac{\partial^2 \zeta_1}{\partial y^2} \right) \right\|\\ \end{align}$
(52) $\begin{align} &M_{x y}(x,y)=\left\| -D(1-\nu)\frac{\partial^2 \zeta_1}{\partial x\partial y} \right\|\\ \end{align}$
(53) $\begin{align} &F_x(x,y)=\left\| -D \left(\frac{\partial^3 \zeta_1}{\partial x^3}+\frac{\partial^3 \zeta_1}{\partial x\partial y^2} \right) \right\|\\ \end{align}$
(54) $\begin{align} &\sigma_x(x,y)=\frac{6}{d^2} M_x(x,y)\\ \end{align}$
(55) $\begin{align} &\tau_{x y}(x,y)=\frac{6}{d^2}M_{x y}(x,y) \end{align}$
(56) $\begin{eqnarray} \tau_{x z}(x,y)=\frac{3}{2d} F_x(x,y) \end{eqnarray}$
其中, $M_x(x,y)$ 和$M_{x y}(x,y)$ 分别是截面单位长度上垂直于$x$轴的弯矩和扭矩; $F_x(x,y)$截面单位长度上垂直于$x$ 轴的平行于$z$ 轴的剪力; $\sigma_x(x,y)$是截面上平行于$x$轴的最大的法应力分量; $\tau_{x y}(x,y)$ 和$\tau_{x z}(x,y)$ 分别表示截面上的最大剪应力分量. 三维相比二维情况下, 多了扭矩$M_{x y}(x,y)$和对应的剪应力$\tau_{x y}(x,y)$.

图8 显示了板的应力状态随入射角 $\theta_1$的变化, 其中$2 h_1=h_2=0.4$, $\gamma_1=\gamma_2=0.9$. 在板和水的交界处, 由于是自由边界条件, $M_x$ 和$F_x$ 的值均为零. 当发生表面波模态的全反射时, 如图中入射角$\theta_1=60^\circ$ 时, 在表面波模态全反射的情况下, 在经过过渡区后, 各受力状态都会趋向于$0$ . 板的正应力$\sigma_x$ 和切应力$\tau_{x y}$的值远大于$\tau_{x z}$, 可以忽略$\tau_{x z}$的作用. 在三维情况下, 扭矩带来的剪应力$\tau_{x y}$的影响不可忽略.

图8

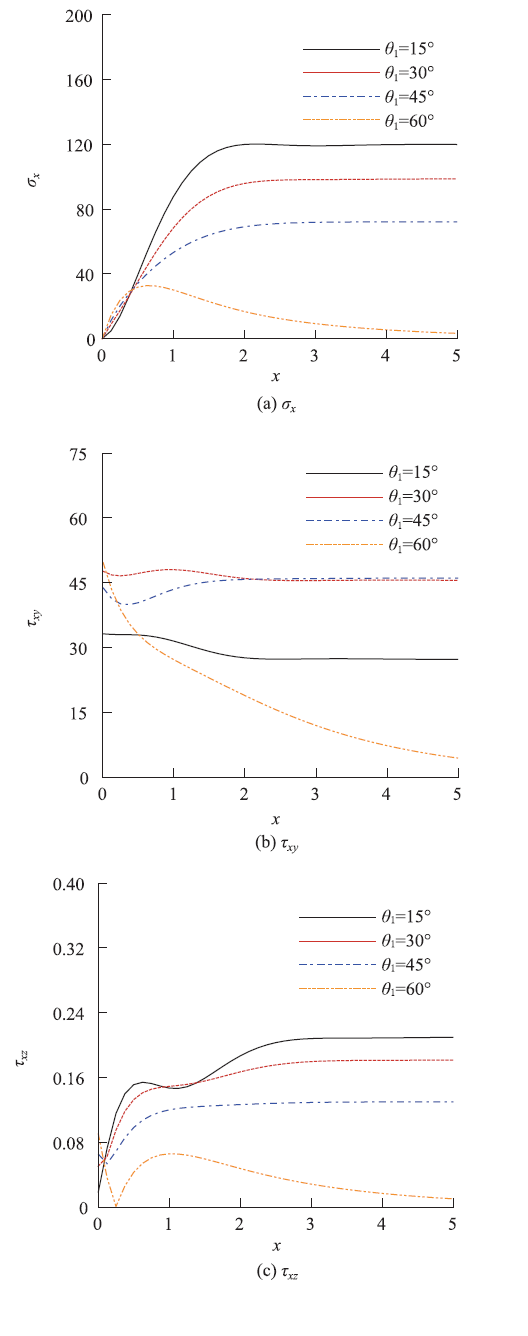新窗口打开|下载原图ZIP|生成PPT
图8当表面波模态入射时, 不同$\theta_1$下板的应力情况

Fig.8The stress state of the plate for different $\theta_1$ in the case of the incident waves of the surface wave mode

3.3 界面波模态入射

Lin 和 Lu ^[22]得到了两层流体斜入射半无限弹性板的水弹性问题中, 界面波模态入射下存在3个临界角. 在三层流体中, 能得到同样形式的临界角

(57) $\begin{align} &\theta_{n ,{2m-1}}=\arcsin(\widetilde{k}_{0_m}/k_{0_n})\quad (m=1,2,\ldots ,n) \\ \end{align}$
(58) $\begin{align} &\theta_{n ,2m}=\arcsin(k_{0_m}/k_{0_n})\quad (m=1,2,\ldots ,n) \end{align}$
式中$n$表示入射波所在界面($n=1,2,3)$, 且$\theta_{n,1}<\theta_{n,2}<\ldots<\theta_{n ,{2m-1}}$, 对于比入射波所在位置更上层的区域, 自表面开始, 当入射角越过奇数临界角, 将消去对应的第$m$界面处板覆盖水域模态; 入射角越过偶数临界角时, 将消去对应的第$m$界面处开阔水域模态, 只剩下开阔水域的界面波模态. 这一结论可推广到任意$n$层($n\geq 2$)的情形.

图9 显示了上界面波入射的波幅图, 其中$\zeta_{0_2}=0.01$, $2 h_1=h_2=0.4$, $\gamma_1=\gamma_2=0.9$. 在此参数下, 上界面波模态对应的临界角为 $\theta_{2,1} \approx 2.66^\circ$, $\theta_{2,2} \approx 3.27^\circ$. 当$\theta_2<\theta_{2,1}$, 如图中取入射角$\theta_2=1^\circ, 2^\circ$ 时, 此时各界面模态均存在. 当$\theta_{2,1}<\theta_2<\theta_{2,2}$, 例如取$\theta_2=3^\circ$ 时, 此时板覆盖水域的表面波模态消失. 当$\theta_{2,2}<\theta_2<\theta_{2,3}$, 例如取$\theta_2=4^\circ$, 此时开阔水域的表面波模态消失.

图9

新窗口打开|下载原图ZIP|生成PPT
图9当上界面波模态入射时, 不同入射角$\theta_2$ 下$A_{\textrm{1}}$, $A_{\textrm{2}}$和$A_{\textrm{3}}$ 随$x$ 的变化

Fig.9The amplitudes of the gravity waves and the hydroelastic deflection of the plate for different $\theta_2$ in the case of the incident waves of the upper interfacial wave mode



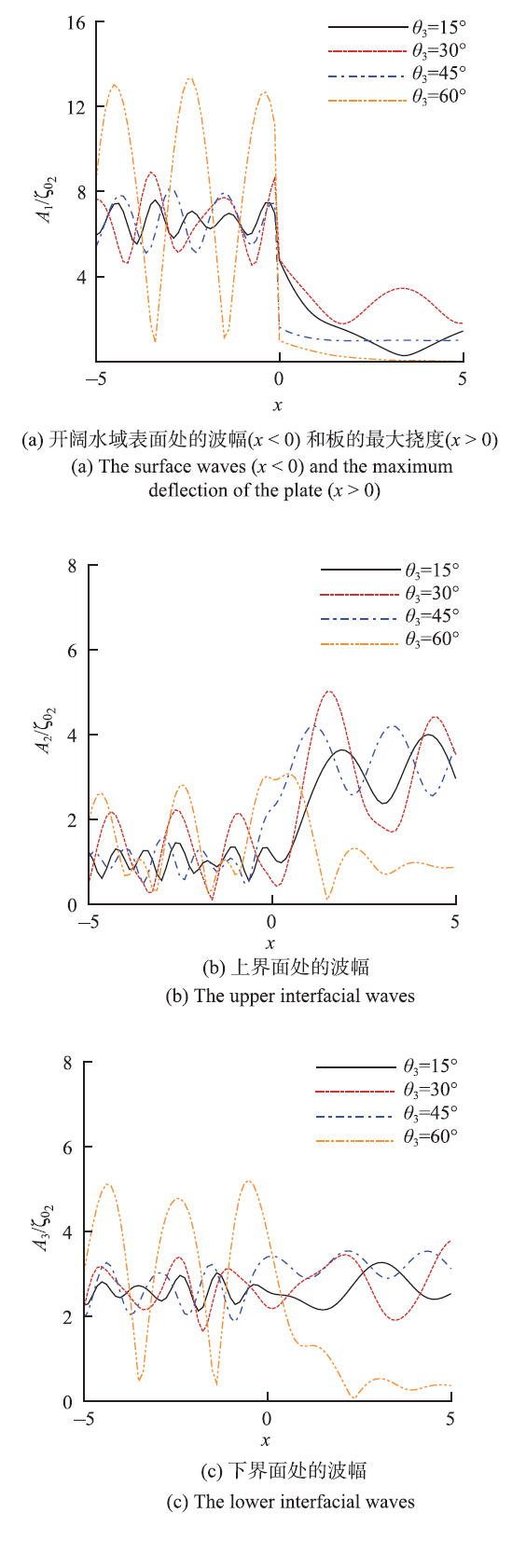
图10 显示了上界面波入射的波幅图, 其中$\zeta_{0_2}=0.01$, $2 h_1=h_2=0.4$, $\gamma_1=\gamma_2=0.3$. 在此参数下, 上界面波模态对应的临界角为 $\theta_{2,1} \approx 30.02^\circ$, $\theta_{2,2} \approx 34.97^\circ$ 和 $\theta_{2,3} \approx 54.11^\circ$. 当$\theta_2<\theta_{2,1}$, 如图中取入射角 $\theta_2=15^\circ$, 此时各界面模态均存在.

图10

新窗口打开|下载原图ZIP|生成PPT
图10当上界面波模态入射时, 不同入射角$\theta_2$ 下$A_{\textrm{1}}$, $A_{\textrm{2}}$和$A_{\textrm{3}}$随$x$的变化

Fig.10The amplitudes of the gravity waves and the hydroelastic deflection of the plate for different $\theta_2$ in the case of the incident waves of the upper interfacial wave mode



当$\theta_{2,1}<\theta_2<\theta_{2,2}$, 如图中取$\theta_2=30^\circ$, 此时板覆盖水域的表面波模态消失. 当$\theta_2>\theta_{2,3}$, 如图示取$\theta_2=60^\circ$, 此时各界面板覆盖水域的波幅都趋向于$0$.

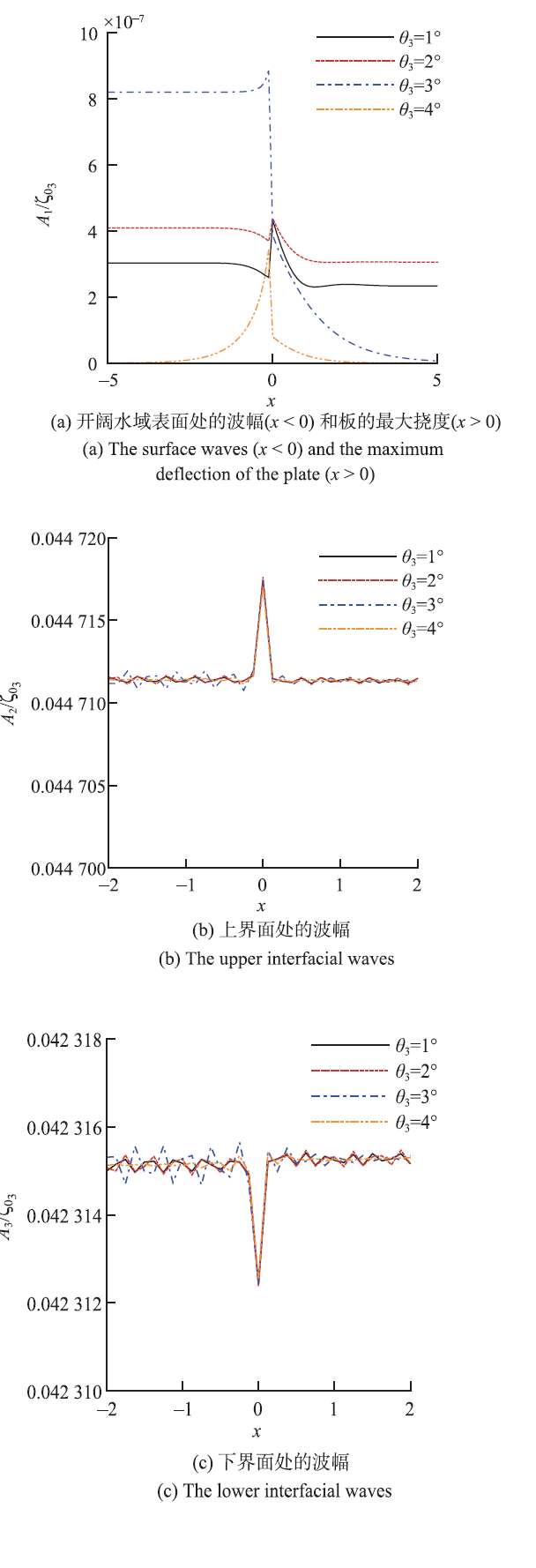
图11 显示了下界面波入射的波幅图, 其中$\zeta_{0_3}=0.01$, $2 h_1=h_2=0.4$, $\gamma_1=\gamma_2=0.9$. 在此参数下, 下界面波模态对应的临界角为 $\theta_{3,1} \approx 2.64^\circ$, $\theta_{3,2} \approx 3.25^\circ$.当$\theta_3<\theta_{3,1}$, 如图中取入射角$\theta_3=1^\circ,2^\circ$ 时, 此时各界面模态均存在. 当$\theta_{3,1}<\theta_3<\theta_{3,2}$, 如图示取入射角$\theta_3=3^\circ$时, 此时板覆盖水域的表面波模态消失. 当$\theta_{3,2}<\theta_3<\theta_{3,3}$, 如图中取入射角$\theta_3=4^\circ$, 此时开阔水域的表面波模态消失.

图11

新窗口打开|下载原图ZIP|生成PPT
图11当下界面波模态入射时, 不同入射角$\theta_3$ 下$A_{\textrm{1}}$, $A_{\textrm{2}}$和$A_{\textrm{3}}$随$x$ 的变化

Fig.11The amplitudes of the gravity waves and the hydroelastic deflection of the plate for different $\theta_3$ in the case of the incident waves of the lower interfacial wave mode



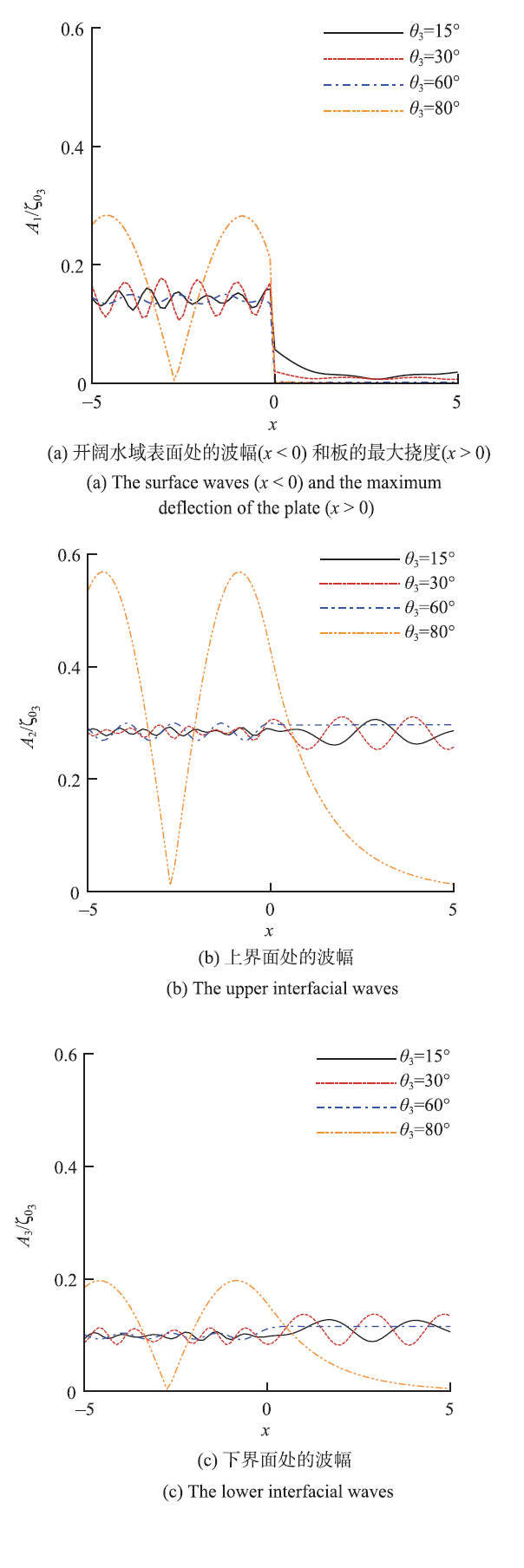
图12 显示了下界面波入射的波幅图, 其中$\zeta_{0_3}=0.01$, $2 h_1=h_2=0.4$, $\gamma_1=\gamma_2=0.3$. 在此参数下, 下界面波模态对应的临界角为 $\theta_{3,1} \approx 18.96^\circ$, $\theta_{3,2} \approx 21.85^\circ$, $\theta_{3,3} \approx 31.74^\circ$, $\theta_{3,4} \approx 40.50^\circ$ 和 $\theta_{3,5} \approx 77.06^\circ$. 当$\theta_3<\theta_{3,1}$, 如图中取入射角$\theta_3=15^\circ$, 此时各界面模态均存在. 当$\theta_{3,4}<\theta_3<\theta_{3,5}$, 如图中取入射角$\theta_3=60^\circ$, 此时板覆盖水域的表面和上界面波模态消失. 当$\theta_3>\theta_{3,5}$, 如图示取入射角$\theta_3=80^\circ$, 此时各界面板覆盖水域的波幅都趋向于$0$.

图12

新窗口打开|下载原图ZIP|生成PPT
图12当下界面波模态入射时, 不同入射角$\theta_3$ 下$A_{\textrm{1}}$, $A_{\textrm{2}}$和$A_{\textrm{3}}$随$x$ 的变化

Fig.12The amplitudes of the gravity waves and the hydroelastic deflection of the plate for different $\theta_3$ in the case of the incident waves of the lower interfacial wave mode



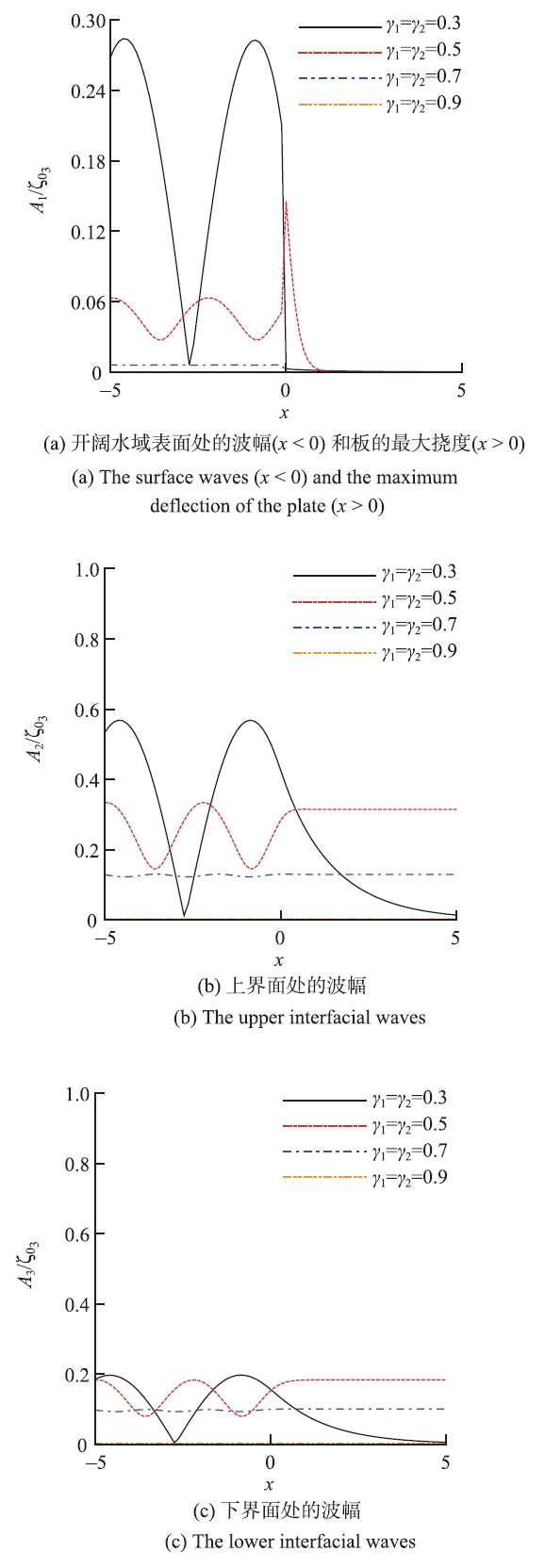
图13显示了下界面波模态入射下密度比$\gamma=\gamma_1=\gamma_2$对水弹性响应的影响, 其中$\zeta_{0_3}=0.01$, $2 h_1=h_2=0.4$, $\theta_3=80^\circ$. 随着$\gamma$ 的减小, 各临界角都会发生很大的变化. 如图14 所示, $\theta_{3,5}$随着$\gamma$的减小而减小. 当密度比减小, 使得$\theta_3>\theta_{3,5}$, 例如$\gamma = 0.3$, 此时的$\theta_{3,5}\approx77.06^{\circ}$, 下界面波模态全反射, 各界面板覆盖水域的波幅都趋向于$0$.

图13

新窗口打开|下载原图ZIP|生成PPT
图13当下界面波模态入射时, 不同密度比$\gamma$ 下$A_{\textrm{1}}$, $A_{\textrm{2}}$和$A_{\textrm{3}}$ 随$x$的变化

Fig.13The amplitudes of the gravity waves and the hydroelastic deflection of the plate for different $\gamma$ in the case of the incident waves of the lower interfacial wave mode

图14

新窗口打开|下载原图ZIP|生成PPT
图14$d, E$ 和 $\gamma$ 对不同临界角的影响

Fig.14The effects of $d, E$ and $\gamma$ on the different critical angles



图14 显示了物理参数对临界角的影响. 对于$\theta_{1,1}$, $\theta_{2,1}$ 和 $\theta_{3,1}$, 它们随着$d$ 和 $E$ 的增大而减小. 而其他临界角随$d$ 和 $E$ 的变化很小. 当改变密度比$\gamma=\gamma_1=\gamma_2$ 时, 所有临界角都会出现巨大变化. 当$E$足够小时, 挠曲重力波逐渐退化成有重力载荷的表面重力波. 此时板覆盖水域的波数将会出现大于开阔水域的波数的情况, 临界角$\theta_{1,1}$ 将不存在.

4 结 论

使用本征函数展开法, 研究了有限深三层流体中小振幅斜入射波与半无限弹性的水弹性相互作用, 使用内积得到一个封闭线性方程组. 并用波能流守恒进行验证, 数值结果显示此方法收敛性好.

当表面波入射时, 界面波以表面波模态传播, 而不是界面波模态. 存在临界角$\theta_{11}$, 当表面波入射角$\theta_1>\theta_{1,1}$ 时, 板覆盖水域各层的表面波模态消失.

在第$n$层界面波入射时, 存在临界角$\theta_{n,1}$, $\theta_{n,2},$ $\ldots$, $\theta_{n,{2m-1}}$, 对于比入射波更上层的区域, 自表面开始, 每当入射角越过奇数临界角将消去对应的第$m$ 界面处板覆盖水域模态, 入射角越过偶数临界角将消去对应的第$m$ 界面处开阔水域模态, 最后只剩下开阔水域的界面波模态.

与正入射二维问题相比, 斜入射三维问题中, 需要着重考量各个物理参数对临界角的影响, 板的厚度$d$和Young 模量$E$ 主要影响板覆盖水域的表面波模态和与之相关的临界角$\theta_{1,1}$, $\theta_{2,1}$ 和 $\theta_{3,1}$. 各层流体的密度比$\gamma$的改变会显著影响界面波响应.

参考文献 View Option 原文顺序
文献年度倒序
文中引用次数倒序
被引期刊影响因子

[1] Kashiwagi M . Research on hydroelastic responses of VLFS: Recent progress and future work
International Journal of Offshore and Polar Engineering, 2000,10(2):81-90
[本文引用: 1]

[2] Watanabe E, Utsunomiya T, Wang CM . Hydroelastic analysis of pontoon-type VLFS: A literature survey
Engineering Structures, 2004,26(2):245-256
DOIURL

Abstract

Presented herein is a literature survey of the research on hydroelastic analysis of pontoon-type very large floating structures (VLFS). After a brief introduction of VLFS, the reader is provided with the basic assumptions, equations and boundary conditions for a hydroelastic analysis of VLFS and the commonly used approaches for solving the problem. Based on a comprehensive search, research papers that contain significant contributions to the aforementioned topic are grouped under the following topics: wave forces, drift forces and other forces, VLFS models, VLFS shapes, mooring system, breakwaters, profiles of seabed, and anti-motion devices. More importantly, some future directions for VLFS research are articulated. In addition to providing a long list of papers, we also include a list of relevant conference proceedings, and websites containing valuable information on VLFSs.

[3] Ohkusu M, Namba Y . Hydroelastic analysis of a large floating structure
Journal of Fluids and Structures, 2004,19(4):543-555
DOIURL

Abstract

An analytical approach to predict the bending vibration of a very large floating structure of thin and elongated rectangular plate configuration, floating on water of shallow depth and under the action of a monochromatic head wave, is presented. The horizontal size of the plate is huge compared with the wavelength of the incident waves, yet the wavelength is much larger than the draft. The fluid–plate interaction is solved by considering that the draft of the plate is asymptotically zero and the plate bottom surface is located at the water surface. The boundary condition for the fluid flow at the plate bottom surface is derived from the hydroelastic behavior of the plate and is different from the condition at the real water surface. The solution is constructed by matching the wave in the water surface to the wave on the plate bottom surface, i.e., the transverse vibration of the plate. Solutions valid in three sub-regions of the plate bottom surface and two sub-regions of the water surface are found separately to be asymptotically matched. The plate vibration is obtained in an explicit form and its accuracy is confirmed against the results from more computationally involved approach.

[4] Wang CM, Tay ZY . Very large floating structures: Applications, research and development
Procedia Engineering, 2011,14:62-72
DOIURLPMID [本文引用: 1]
Polydimethylsiloxane (PDMS) membranes are used in various applications, such as microvalves, micropumps, microlenses, and cell culture substrates, with various thicknesses from microscale to nanoscale. In this study, we propose a simple fabrication method for PDMS membranes on a water surface, referred to as the floating-on-water (FoW) method. FoW can be used to easily fabricate PDMS membranes with thicknesses of a few micrometers (minimum 3 μm) without special equipment. In addition, as the membrane is fabricated on the water surface, it can be easily handled without damage. In addition, alternative membrane structures were demonstrated, such as membrane-on-pins and droplet-shaped membranes. FoW can be widely used in various applications that require PDMS membranes with microscale thicknesses.

[5] Squire VA . Of ocean waves and sea-ice revisited
Cold Regions Science and Technology, 2007,49(2):110-133
DOIURL [本文引用: 1]

[6] 闫红梅, 崔维成, 刘应中 . 超大型浮体水弹性分析的平板格林函数法
海洋工程, 2003,21(4):8-14
[本文引用: 1]

( Yan Hongmei, Cui Weicheng, Liu Yingzhong . Hydroelastic analysis of VLFS using plate Green function method
Ocean Engineering, 2003,21(4):8-14 (in Chinese))
[本文引用: 1]

[7] 王志军, 李润培, 舒志 . 箱式超大型浮体结构在不规则波中的水弹性响应
海洋工程, 2001,19(3):9-13
[本文引用: 1]

( Wang Zhijun, Li Runping, Shu Zhi . Study on hydroelastic response of box 2 shaped very large floating structure in regular waves
Ocean Engineering, 2001,19(3):9-13 (in Chinese))
[本文引用: 1]

[8] 刘超, 祁恩荣, 陆晔 . 浅吃水超大型浮体连接器动力响应
船舶力学, 2014,18(5):581-590
[本文引用: 1]

( Liu Chao, Qi Enrong, Lu Ye . Dynamic response of connectors of very large floating structures under shallow draft
Journal of Ship Mechanics, 2014,18(5):581-590 (in Chinese))
[本文引用: 1]

[9] 巴迪, 卢东强 . Plotnikov-Toland板模型中水弹性孤立波的迎撞
力学学报, 2018,50(6):1406-1417
[本文引用: 1]

( Bhatti MM, Lu Dongqiang . Head-on collision between two hydroelastic solitary waves with Plotnikov-Toland’s plate model
Chinese Journal of Theoretical and Applied Mechanics, 2018,50(6):1406-1417 (in Chinese))
[本文引用: 1]

[10] Linton CM, McIver M . The interaction of waves with horizontal cylinders in two-layer fluids
Journal of Fluid Mechanics, 1995,304(1):213-229
DOIURL [本文引用: 1]

[11] Cadby JR, Linton CM . Three-dimensional water-wave scattering in two-layer fluids
Journal of Fluid Mechanics, 2000,423(1):155-173
DOIURL [本文引用: 1]

[12] Xu F, Lu DQ . Wave scattering by a thin elastic plate floating on a two-layer fluid
International Journal of Engineering Science, 2010,48(9):809-819
DOIURL [本文引用: 2]

Abstract

The hydroelastic interaction between an incident gravity wave and a thin elastic plate floating on a two-layer fluid of finite depth is analyzed with the aid of the method of matched eigenfunction expansions. The fluid is assumed to be inviscid and incompressible. A two-dimensional problem is formulated within the framework of linear potential theory for small-amplitude waves. The fluid domain is divided into two and three regions for semi-infinite and finite plates, respectively, with the matching relations representing the continuities of the pressure and velocity. A new inner product involving two single integrals is proposed, in which the vertical eigenfunctions in the open water region of the two-layer fluid are orthogonal. Then the orthogonality of the eigenfunctions with respect to the newly defined inner product is used to obtain a set of simultaneous equations for the expansion coefficients of the velocity potentials, and the edge conditions are included as a part of the equation system. The effects of the fluid density ratio and the position of interface on the wave reflection and transmission are discussed. Numerical analysis shows that the method proposed herein is effective with a higher rate of convergence.

[13] Behera H, Koley S, Sahoo T . Wave transmission by partial porous structures in two-layer fluid
Engineering Analysis with Boundary Elements, 2015,58:58-78
DOIURL [本文引用: 1]

[14] Islam N, Gayen R . Scattering of water waves by an inclined plate in a two-layer fluid
Applied Ocean Research, 2018,80:136-147
DOIURL [本文引用: 1]

[15] Paul S, De S . Effects of vertical porous barrier on progressive waves in a two layered fluid
Ocean Engineering, 2018,156:153-166
DOIURL [本文引用: 1]

[16] Rus$\mathring{a}$ PO, Grue J . Solitary waves and conjugate flows in a three-layer fluid
European Journal of Mechanics B/Fluids, 2002,21(2):185-206
DOIURL [本文引用: 1]

[17] Das D . Wave scattering by a horizontal circular cylinder in a three-layer fluid
Journal of Ocean Engineering and Science, 2016,1(2):135-148
DOIURL [本文引用: 1]

[18] Meng QR, Lu DQ . Hydroelastic interaction between water waves and thin elastic plate floating on three-layer fluid
Applied Mathematics and Mechanics - English Edition, 2017,38(4):567-584
[本文引用: 4]

[19] Fox C, Squire VA . On the oblique reflexion and transmission of ocean waves at shore fast sea ice
Philosophical Transactions of the Royal Society of London. Series A: Physical and Engineering Sciences, 1994,347(1682):185-218
[本文引用: 1]

[20] Kim H, Do KD, Suh KD . Scattering of obliquely incident water waves by partially reflecting non-transmitting breakwaters
Ocean Engineering, 2011,38(1):148-158
DOIURL [本文引用: 1]

Abstract

In the present paper, analytic solutions are derived for scattering of water waves obliquely incident to a partially reflecting semi-infinite breakwater or breakwater gap. In order to examine the correctness of the derived solutions, they are compared with the solutions derived by McIver (1999) and Bowen and McIver (2002) for a semi-infinite breakwater and a breakwater gap, respectively, in the case of perfect reflection. The derived analytic solutions are used to investigate the effect of reflection coefficient of the breakwater and wave incident angle upon the tranquility at harbor entrance. The tranquility is deteriorated by the reflected waves as the reflection coefficient increases and as waves are incident more obliquely.

[21] Cho I, Kim M . Transmission of oblique incident waves by a submerged horizontal porous plate
Ocean Engineering, 2013,61:56-65
DOIURL [本文引用: 1]
The interaction of oblique monochromatic incident waves with a submerged horizontal porous plate has been investigated in the context of two-dimensional linear potential theory. The matched eigenfunction expansion method is applied to obtain the analytic solution. The present formulations, suggested by Molin and Nielsen (2004) and Liu and Li (2011), are compared with the independent solutions using complex dispersion relations and they are proved to be identical. The former approach, however, may be more straightforwardly extended to the case of multi-layer horizontal porous plates. Also, the present predictions are compared with a series of experiments conducted in a two-dimensional wave tank at Jeju National University. By using the empirical relationship between plate porosity and porous parameter obtained by authors (Cho and Kim, 2008), the measured reflection and transmission coefficients reasonably follow the trend of predicted values. The performance of the proposed submerged horizontal porous breakwaters can be significantly enhanced by selecting optimal design parameters, such as porosity, submergence depth, and plate width. The optimal cases are obtained through a systematic parametric study, which illustrates the usefulness of the presently developed analytic solutions. (C) 2013 Elsevier Ltd.

[22] Lin Q, Lu DQ . Hydroelastic interaction between obliquely incident waves and a semi-infinite elastic plate on a two-layer fluid
Applied Ocean Research, 2013,43(43):71-79
DOIURL [本文引用: 3]