王巍^,2), 唐滔, 卢盛鹏, 张庆典, 王晓放大连理工大学海洋能源利用与节能教育部重点实验室, 大连 116023

NUMERICAL SIMULATION AND ANALYSIS OF ACTIVE JET CONTROL OF HYDROFOIL CAVITATION¹⁾

Wang Wei^,2), Tang Tao, Lu Shengpeng, Zhang Qingdian, Wang XiaofangKey Laboratory of Ocean Energy Utilization and Energy Conservation of Ministry of Education, Dalian University of Technology, Dalian 116024, China

通讯作者: 2)王巍, 副教授, 主要研究方向: 先进动力装置及流体机械设计和优化. E-mail:wangw@dlut.edu.cn

收稿日期:2019-08-19接受日期:2019-10-17网络出版日期:2019-10-21

基金资助:

1) 国家自然科学基金.51876022 国家973计划资助.2015CB057301

Received:2019-08-19Accepted:2019-10-17Online:2019-10-21
作者简介 About authors


摘要
为了改善高速流动工况下水翼吸力面上流场的空化特性, 提出了水翼表面主动射流对绕水翼周围流动加以控制的方法. 基于密度分域滤波的FBDCM混合湍流模型联合Zwart-Gerber-Belamri空化模型, 分析了来流空化数为0.83, 来流攻角为8$^\circ$, 射流位置距水翼前缘为$x=0.19c$时, 主动射流对于水翼吸力面上流动的空化特性和水动力特性影响. 对回射流的强度进行了量化分析, 以探究回射流与流场空化特性的关系. 数值分析结果表明, 在射流水翼吸力面上的时均空泡体积为原始水翼的1/15, 使得流场内空化流动由云空化状态转变为较为稳定的片空化状态, 显著地削弱了云空化的发展. 此外, 射流极大地改善了水翼的水动力性能, 使得水翼的升阻比较原始水翼提高了22.9${\%}$, 空泡的脱落频率减少了26.2${\%}$, 空泡脱落所引起的振幅减小了9.1${\%}$. 射流大幅降低了水翼吸力面上低压区面积, 水翼吸力面上流体的逆向压力减小, 回射流强度降低; 同时, 射流使水翼吸力面上的边界层减薄, 增强了流动的抗逆压梯度能力, 一定程度上阻挡了回射流向水翼前缘的流动, 这也从机理上分析了主动射流抑制空化的原因.
关键词： 空化抑制;主动射流;回射流;水动力性能;流动控制

Abstract
In order to improve the cavitation characteristics of the flow field on the suction side of the hydrofoil under high-speed flow conditions, a method of active water jet arranged on the suction side is proposed to control the flow around the hydrofoil. Based on a filter-based density correction turbulence model combined with Zwart-Gerber-Belamri cavitation model, the influence of the water jet on the cavitation and hydrodynamic characteristics of the hydrofoil is analyzed when the cavitation number is 0.83, the angle of attack is 8$^\circ$ and the water jet is 0.19$c$ from the foil leading edge. The intensity of the re-entrant jet is analyzed quantitatively to explore the relationship between the re-entrant jet and the cavitation characteristics of the flow field. The numerical results show that the time-average cavity volume on the suction side of the hydrofoil with jet is 14/15 smaller than that of the original hydrofoil, which indicate that the water jet can significantly weaken the development of cavitation, and thus the cavitation pattern in the flow field transforms from cloud cavitation to sheet cavitation. Moreover, the water injection greatly improves the hydrodynamic performance of the hydrofoil. The lift to drag ratio of the hydrofoil increases by 22.9${\%}$ compared with that of the original hydrofoil, meanwhile, and the shedding frequency of the cavitation decreases by 26.2${\%}$, and the amplitude caused by the shedding of the cavitation decreases by 9.1${\%}$. The water jet shrinks low pressure area on the suction side sharply and reduces the reverse pressure difference of flow in the vicinity of the hydrofoil, as a result, intensity of the re-entrant jet declined. The water injection also thins the boundary layer which enhances the anti-reverse pressure gradient capability of the flow and then blocks the re-entrant jet. Those explain the mechanism of cavitation flow control by active water injection.
Keywords：cavitation suppression;active injection;re-entrant jet;hydrodynamic performance;flow control


PDF (6267KB)元数据多维度评价相关文章导出EndNote|Ris|Bibtex收藏本文
本文引用格式
王巍, 唐滔, 卢盛鹏, 张庆典, 王晓放. 主动射流控制水翼空化的数值模拟与分析¹⁾. 力学学报[J], 2019, 51(6): 1752-1760 DOI:10.6052/0459-1879-19-222
Wang Wei, Tang Tao, Lu Shengpeng, Zhang Qingdian, Wang Xiaofang. NUMERICAL SIMULATION AND ANALYSIS OF ACTIVE JET CONTROL OF HYDROFOIL CAVITATION¹⁾. Chinese Journal of Theoretical and Applied Mechanics[J], 2019, 51(6): 1752-1760 DOI:10.6052/0459-1879-19-222

引 言

空化是存在于液体中的相变现象, 它是指流体的局部压力下降到饱和蒸气压以下时, 流体会产生相变生成气泡的一种物理现象^[1]. 空化现象是导致一系列流动失稳的原因之一, 在核主泵、水轮机和船用螺旋桨中, 由于叶片表面产生空化而导致叶片或整机的运行失稳的情况也层出不穷^[2-3].

空化流是一种复杂的多相湍流^[4], 空化模型和湍流模型在空化流模拟中起着至关重要的作用^[5-6]. 但数值模型中总是存在着若干经验系数, 严重影响数值计算精度, 这是数值计算始终存在着不确定性或误差的重要原因^[7]. Huang等^[8]的研究发现湍流和空化之间的相互作用非常复杂, Ye等^[9]发现在汽相体积分数较高时, 湍流极大地抑制了液相的汽化, 这说明空化模型对于预测由气泡聚结引起的充满蒸汽的大空腔流动是不适用的. Anderlini等^[10]基于喷嘴内部流动利用响应面方法进行了Schnerr-Sauer空化模型中4个参数对于大涡模拟结果准确性的灵敏度分析, 发现参数选择的不同结果不同. Congedo等^[11]对湍流模型和空化模型所得的模拟结果的不确定性进行了量化分析. 黄彪等^[12]通过一个桥接函数将FBM^[13]和DCM^[14]结合起来, 提出了基于密度分域的混合湍流模型(FBDCM), 证实了FBDCM模型在捕捉湍流脱落细节以及空泡形态演化规律要优于FBM和DCM湍流模型. Yu等^[15]利用FBDCM模型研究了NACA66水翼空化特征, 其结果表明FBDCM能够成功预测空泡生长、破裂溃灭过程, 尤其在脱落频率上与实验吻合极好.

空化可通过主动控制和被动控制两种手段进行控制, 两者的本质区别是有无向体系中注入额外能量来控制空化^[16]. 不同的应用环境, 有效控制空化的方式也是不相同的. Chatterjee等^[17]通过超声波和电解来控制流场中的空化核数, 来达到抑制空化的目的. Timoshevskiy等^[16]观察了沿水翼表面不同强度的切向液体射流对空泡的影响, 并划分了不同射流速度区间, 研究对空化流的影响. Chahine等^[18]基于船用螺旋桨研究了注入水和甘油的混合物来达到抑制空化的效果. Chang等^[19]通过向尾涡核心注入水和聚合物溶液来达到延迟梢涡空化初生的目的. M$\ddot{a}$kiharju等^[20]将少量的不凝结气体注入空泡界面使空泡的发展提前终止, 以此来达到抑制空化震荡. Wang等^[21]在水翼吸力面布置射流水孔, 发现射流能够阻挡回射流向前缘发展, 从而达到降低空泡脱落频率的效果.

以上都是通过一些主动控制的方式来达到的抑制空化的目的, 除此之外, 被动控制也能实现对空化流动的控制. 被动控制是通过某种方式改变壁面特性来实现的, 不需要向流场提供能量. Leger等^[22]使用不同疏/亲水性的材料来研究材料性质对于空泡分离的影响, 证实固液之间的黏性力会对附着型空泡的形状产生强烈的影响. Kadivar等^[23]提出在水翼吸力面前缘布置汽泡发生器, 结果显示布置汽泡发生器能够显著减小水翼吸力面低压区大小, 有效抑制空化的发展. 王巍等^[24]在水翼表面设置凹坑, 结果显示在某些工况下, 设置凹坑能够有效改善水翼吸力面的边界层流动. Kawanami等^[25]揭示了回射流是引起云状空泡脱落的原因, 在片状空泡尾缘处设置障碍物能够有效阻挡回射流, 从而达到抑制云空化的目的. Wang等^[26]通过在水翼上开不同角度通孔, 利用水翼压力面与吸力面之间的压差使主流沿射流孔流入, 进而提高低压区压力和流速达到抑制空化的目的. Coutier-Delgosha等^[27]研究了水翼表面粗糙度对于空泡不稳定性的影响, 发现随着表面粗糙度的增加空泡长度减小、震荡频率增大. 并且粗糙度增大使得空化的周期演变更加混乱, 这有助于减小了压力波动的强度. 诸多主动或被动的方式用以来改变壁面压力分布方式, 进而改变空化特性.

值得注意的是, 对于被动控制, 一旦被动装置设置完成, 对于某类特定工况具有较好的空化控制效果, 但对于其他工况, 空化抑制效果并不明显. 所以从适应性角度来看, 主动控制方法的应用范围更为广泛. 论文基于主动射流抑制空化的实验研究基础^[28], 采用一种基于密度分域的滤波器湍流模型方法, 对主动射流控制空化流场开展了详细研究, 以期为空化抑制研究提供可行方案.

1 数值计算方法及验证

1.1 控制方程

气液均相流模型是目前研究者在数值求解空化流动计算中普遍采用的方法之一, 因此, 本文基于均相流模型建立空化流场的流动模型. 均相流模型将汽液混合物视为均一的介质, 汽液两相共享流场参数, 无须考虑单独两相间相互作用, 混合相参数取气液两相相应参数的加权平均, 两相间无滑移速度, 汽液两相的连续性方程和动量方程^[29]为

(1) $\begin{eqnarray} \frac{\partial \rho_{\rm m}}{\partial t}+\frac{\partial(\rho_{\rm m}u_j)}{\partial x_j}=0 \end{eqnarray}$
(2) $\begin{eqnarray} \frac{\partial(\rho_{\rm m}u_i)}{\partial t}+\frac{\partial(\rho_{\rm m}u_iu_j)}{\partial x_j}=-\frac{\partial p}{\partial x_i}+ \\ \qquad\frac{\partial}{\partial x_j}\lt[(u_{\rm m}+u_{\rm t})\lt(\frac{\partial u_i}{\partial x_j}+\frac{\partial u_j}{\partial x_i}-\frac23\frac{\partial u_i}{\partial x_j}\delta_{ij})] \end{eqnarray}$
混合相密度和黏度为

(3) $\begin{eqnarray} \rho_{\rm m}=\rho_{\rm l}\alpha_{\rm l}+\rho_{\rm v}\alpha_{\rm v} \end{eqnarray}$
(4) $\begin{eqnarray} \mu_{\rm m}=\mu_{\rm l}\alpha_{\rm l}+\mu_{\rm v}\alpha_{\rm v} \end{eqnarray}$
式中, 下标$i$和$j$代表坐标方向, $u$和$p$分别为混合相速度和压力, $\rho_{\rm m}$, $\mu_{\rm m}$和$\mu_{\rm t}$分别是混合相密度和混合相层流/湍流黏性系数, $\alpha$代表体积分数, 下标l和v分别表示液相和汽相.

1.2 基于密度分域滤波模型(FBDCM)

FBDCM湍流模型由黄彪等^[12]提出, 该模型基于标准$k$-$\varepsilon$湍流模型将DCM模型^[14]和FBM模型^[13]结合起来, 可以兼顾不同区域的流动特点, 充分考虑了空化过程的多尺度效应的湍流流动和多相流的特点, 在捕捉附着型空穴的生长发展过程的细节上有较高的预测精度. 标准$k$-$\varepsilon$湍流模型的湍流黏度为

(5) $\begin{eqnarray} \mu _{\rm t} = \rho _{\rm m} C_{\mu}\frac{k^2}{\varepsilon } \end{eqnarray}$
式中, $k$为湍动能, $\varepsilon$的为湍动能耗散率, 修正系数$C_{\mu}=0.09$.

基于标准$k$-$\varepsilon$模型, 对FBM和DCM的湍流黏性系数进行桥接, 进一步对湍流黏性$\mu_{\rm t}$进行密度分域修正. FBDCM模型引入混合函数$\chi$ ($\rho_{\rm m}/\rho_{\rm l})$对式(5)的湍流黏度$\mu_{\rm t}$进行修正, 其中$f_{\rm FBM}$和$f_{\rm DCM}$分别为滤波模型和密度修正模型的修正系数

(6) $\begin{eqnarray} f_{\rm FBM}={\rm Min}\lt[1, C_3\lambda\varepsilon/k^{3/2}],\ \ C_3=1.0 \end{eqnarray}$
(7) $\begin{eqnarray} f_{\rm DCM}=\frac{\rho_{\rm v}+(\alpha_{\rm l})^{\rm n}(\rho_{\rm l}-\rho_{\rm v})}{\rho_{\rm v}+(\alpha_{\rm l})(\rho_{\rm l}-\rho_{\rm v})} \end{eqnarray}$
(8) $\begin{eqnarray} \chi\lt(\frac{\rho_{\rm m}}{\rho_{\rm l}})=0.5+\tanh\lt[\frac{C_1(C_3\rho_{\rm m}/\rho_{\rm 1}-C_2)}{C_4(1-2C_2)+C_2}]\Bigg/ \\ \qquad(2\tanh C_1) \end{eqnarray}$
(9) $\begin{eqnarray} f_{\rm FBDCM}=\chi\lt(\frac{\rho_{\rm m}}{\rho_{\rm l}})f_{\rm FBM}+\lt[1-\chi\lt(\frac{\rho_{\rm m}}{\rho_{\rm l}})]f_{\rm DCM} \end{eqnarray}$
(10) $\begin{eqnarray} \mu_{\rm t}=\frac{\rho_{\rm m}C_{\mu}k^2}{\varepsilon}f_{\rm FBDCM} \end{eqnarray}$
式(8)中$C_{1}$, $C_{2}$, $C_{3}$及$C_{4}$四个模型参数均为常数, 本文采用文献^[12]中的推荐值$C_{1}=4$, $C_{2}=0.2$, $C_{3}=0.6$及$C_{4}=0.2$. 本文研究过程采用文献^[30]推荐的密度修正系数$n=10$, 滤波尺寸取$\lambda =0.015c$.

1.3 ZGB空化模型

空化模型采用由Zwart等^[31]开发出的基于质量输运方程(11)的ZGB空化模型, 如式(12)所示.

(11) $\begin{eqnarray} \frac{\partial\rho_{\rm m}\alpha_{\rm v}}{\partial t}+\frac{\partial(\rho_{\rm m}u_j\alpha_{\rm v})}{\partial x_j}=R_{\rm e}-R_{\rm c} \end{eqnarray}$
(12) $\left. \begin{array}{l@{\quad}l} P\leqslant P_{\rm v},& R_{\rm e}=F_{\rm vap}\dfrac{3\alpha_{\rm nuc}(1-\alpha_{\rm v})\rho_{\rm v}}{R_{\rm B}}\sqrt{\dfrac23\dfrac{P_{\rm v}-P}{\rho_{\rm l}}}\\\ P\geqslant P_{\rm v},& R_{\rm c}=F_{\rm cond}\dfrac{3\alpha_{\rm v}\rho_{\rm v}}{R_{\rm B}}\sqrt{\dfrac23\dfrac{P-P_{\rm v}}{\rho_{\rm l}}} \end{array} \right\}$
(13) $\begin{eqnarray} P_{\rm v}=P_{\rm sat}+\dfrac12(0.39\rho k) \end{eqnarray}$
其中$R_{\rm B}$为气泡半径, 参考值为1 $\mu$m; $\alpha_{\rm nuc}$为形核点的体积分数参考值为$5\times 10^{ - 4}$; $F_{\rm vap}$为蒸发系数, 参考值为50; $F_{\rm cond}$为凝结系数, 参考值为0.01. 式(12)中, 考虑到湍动能对空化的影响, 饱和蒸汽压$P_{\rm v}$将采用式(13)进行修正.

1.4 物理模型测试参数

本文针对FBDCM湍流模型结合ZGB空化模型对绕NACA66(MOD)水翼空化流动现象进行数值模拟. 无量纲翼型尺寸见参数文献^[32], 水翼弦长为70 mm, 展长为67 mm, 最大厚弦比为12${\%}$, 最大厚度在距水翼前缘$x=0.45c$位置, 最大弯度为2${\%}$, 距水翼前缘为$x=0.50c$. 在水翼吸力面上距水翼前缘$x=0.19c$布置单排射流水孔, 射流孔直径为1.4 mm, 射流孔数目为25, 相邻射流孔圆心相距2.35 mm, 射流水翼示意图如图1所示. 水翼安放攻角$\alpha =8^\circ$, 主流流速$U_{\infty}=7.832$ m/s, 与之相对应的雷诺数$Re=5.07\times 10^{5}$, 空化数$\sigma =(P_{\rm out}-P_{\rm v})/(0.5\rho_{\rm l}U_{\infty }^{2})=0.83$, 其中, 液相的密度$\rho _{\rm l}=998.7$ kg/m$^{3}$, 动力黏度$\mu_{\rm l}=1079.85$ $\mu$Pa$\cdot $s, 汽相密度$\rho_{\rm v}=0.01449$ kg/m$^{3}$, 动力黏度$\mu_{\rm v}=9.65$ $\mu$Pa$\cdot $s, 对应的水的温度为17${^\circ}$C, 该温度下的饱和蒸气压$p_{\rm v}=1940$ Pa. 对于射流水翼, 射流动量系数表示为射流动量与来流动量的比值, 即

(14) $\begin{eqnarray} C_{\mu}=m_{\rm inj}U_{\rm inj}/m_0U_{\infty}=Q_{\rm inj}^2/(nU_{\infty}hs\pi r^2)\approx \\ \qquad 3.497\times10^{-6}Q_{\rm inj}^2/U_{\infty}^2 \end{eqnarray}$

图1

新窗口打开|下载原图ZIP|生成PPT
图1射流水翼示意图

Fig.13D schematic of the hydrofoil with jet



式中各字母分别表示射流的质量流量$m_{\rm inj}$ (kg/s), 射流的体积流量$Q_{\rm inj}$ (L/h), 射流流速$U_{\rm inj}$ (m/s), 主流通过水翼沿主流方向的投影面积$S_{0}$ $(S_{0}=hs=5.7526$ cm$^{2})$的等效质量流量$m_{0}$ (kg/s), 水翼沿主流方向投影的最大高度即水翼的最大厚度$h$ $(h=8.218$ mm), 水翼展向长度$s$ $(s=67$ mm), 射流孔半径$r$ $(r=0.7$ mm), 射流孔数目$n$ $(n=25$). 利用上述公式计算$C_{\mu }$, 当$Q_{\rm inj}$单位为L/h, $U_{\infty }$单位为m/s时, 代入数值部分进行计算即可获得无量纲参数$C_{\mu }$的值$C_{\mu }=11.55\times 10^{ - 3}$.

1.5 计算域和边界条件设置

计算域如图2所示, 与实验过程参数保持一致, 入口来流为均匀速度条件, 雷诺数$Re=5.07\times 10^{5}$; 出口为压力出口边界条件, 空化数$\sigma =0.829$; 叶片前缘距上游速度入口为4$c$, 距下游压力出口为6$c$, 水翼表面及流场上下前后壁面均为速度无滑移绝热壁面条件; 对于原始水翼, 射流孔处为速度无滑移壁面边界条件, 对于含射流的水翼, 射流孔处为入口流量边界条件.

图2

新窗口打开|下载原图ZIP|生成PPT
图2计算域及边界条件设置

Fig.2Computational domain and boundary conditions setting



整个流场的网格采用结构化划分, 如图3所示, 弦长方向的横截面网格节点设置为$320\times 160$, 展向方向网格节点为260个, $y+$在0.5$\sim$10之间,时间步长的选取为$T_{\rm ref}$/100^[33]. 采用Ansys Fluent 17.0进行数值仿真. 基于有限体积法对动量方程和连续性方程进行离散, 采用SIMPLE格式的压力$\!-\!$速度耦合算法求解, 对流项采用一阶迎风格式计算初值, 稳定后采用二阶迎风格式来计算. 压力项采用PRESTO!格式. 非定常项的离散采用一阶隐式格式.

图3

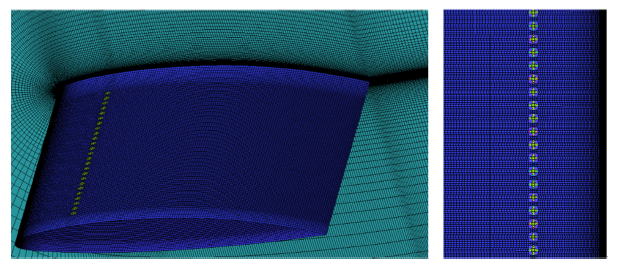新窗口打开|下载原图ZIP|生成PPT
图3水翼及射流孔周围网格划分

Fig.3Mesh generation around the hydrofoil and the jet holes

1.6 数值验证

为了验证本文所采用的物理模型和数值模型的准确性, 将数值模拟所得的结果和实验结果相比较. 在小型空化水洞进行了NACA66 (MOD)水翼的全流场流动测试, 使用5000 Hz的高速摄影相机对流场细节信息进行捕捉. 图4中给出一个典型周期内实验和数值结果的空化形态对比, 数值模拟所得的空化形态演变能够准确地呈现出空泡初生、发展、坍缩和溃灭的过程.

图4

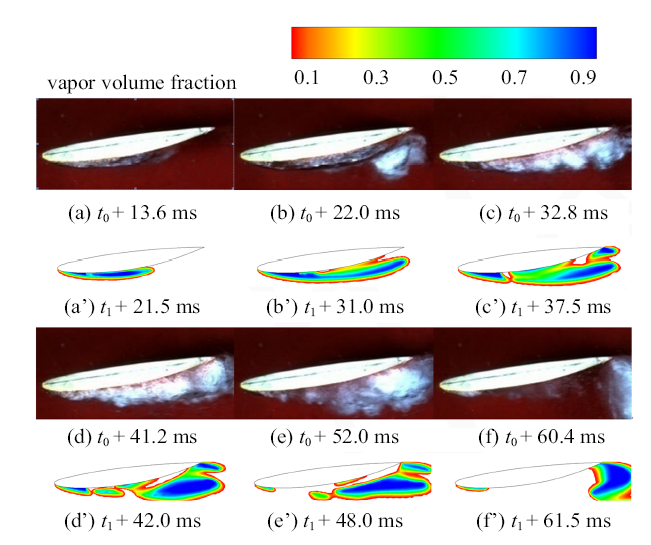新窗口打开|下载原图ZIP|生成PPT
图4一个典型周期内绕原始水翼空化脱落的侧视视角 (上: 实验结果, 下: 数值结果; $\sigma=0.83$, $\alpha=8^\circ$, $Re=5.07\times10^5$)

Fig.4Side view of cavity shedding pattern for $\sigma=0.83$, $\alpha=8^\circ$, $Re=5.07\times10^5$ during a typical cycle (up: experimental results, down: numerical results)



为进一步定量的分析数值结果中空泡的演变过程与实验现象的契合度, 使用灰度处理和图像二值法统计空泡的无量纲面积$S/S_{\rm C}$的变化. 结果如图5所示,使用水翼空化区域面积比$S/S_{\rm C}(S_{\rm C}$为图4 中水翼面积)和斯特劳哈尔数$St_{\rm c}$来比较与实验结果的差别, 结果如表1所示. 空泡的时均面积和$St_{\rm c}$与实验对比的误差分别为3.55${\%}$和0.15${\%}$, 说明数值模型中所涉及的参数选择符合数值精度的要求.

图5

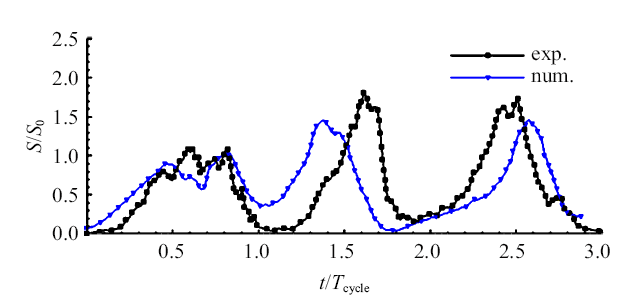新窗口打开|下载原图ZIP|生成PPT
图5三个典型周期内数值预测的无量纲空化区面积与实验结果对比($Re=5.01\times 10^{5}$, $\alpha =8^\circ$, $\sigma =0.83$)

Fig.5The comparison of dimensionless cavitation area obtained by numerical predictions with the experimental results in 3 typical periods ($Re=5.01\times 10^{5}$, $\alpha =8^\circ$ and $\sigma =0.83$)



Table 1
表1
表1实验测量和数值预测的空泡面积以及斯特劳哈尔数对比($Re=5.01\times 10^{5}$, $\alpha =8^\circ$, $\sigma =0.83$)
Table 1The comparison of cavitation area and $St_{\rm c}$ obtained by numerical predictions with the experimental results ($Re=5.01\times 10^{5}$, $\alpha =8^\circ$, $\sigma =0.83$)

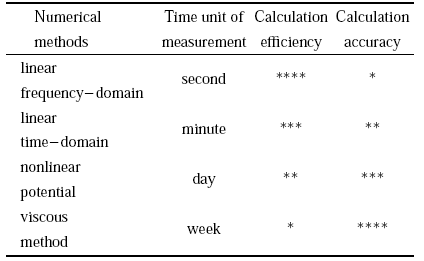新窗口打开|下载CSV

2 结果与分析

2.1 射流对空泡形态影响分析

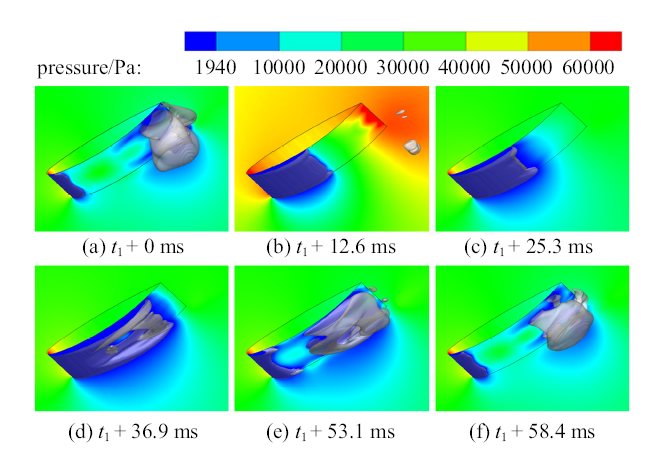
由图6所示, 对于原始水翼(a)时刻为空泡的初生阶段, 在空泡快速增长到$0.5c$后, 空泡长时间维持在$0.5c \sim 0.8c$. 整个周期内空化剧烈, 水翼尾缘空腔脱落现象显著, (c)时刻之后随着空化发展, 低压区覆盖整个水翼吸力面. 在射流动量系数为$C_{\mu }=0.0115$时, 与之对应工况的绕射流水翼云空化状态如图7所示, 其空泡演变过程与原始水翼空泡存在着显著的差别. (a’)时刻为水翼前缘空泡的生长初始阶段, (b’)时刻前缘空泡达到阶段性的最大空泡长度. 由(c’)时刻压力云图看出, 上一周期遗留的尾缘空泡的溃灭产生高压脉冲使得前缘空泡回缩. 在(d’)$\sim$(e’)阶段, 前缘空泡由极小值长度开始重新生长, 且射流孔附近也产生空泡, 然后前缘空泡与射流孔空泡融为一体, 并向尾缘生长. 由(d’)$\sim$(e’)为空泡的缓慢生长阶段, 在(e’)时刻达到前缘空泡的最大值之后空泡开始回缩. 尽管空化区回缩, 但这一阶段低压区依然由水翼前缘向尾缘发展, 至(f’)时刻, 由于低压区的存在, 使水翼尾缘出现了空腔, 即尾缘低压区的存在使空腔在尾缘形成了脱落现象, 但相比于原始水翼脱落空腔的体积已大幅减小.

图6

新窗口打开|下载原图ZIP|生成PPT
图6一个典型周期内绕原始水翼空化脱落的标识码角($\alpha_{\rm V}=0.9$等值面及压力云图分布) ($\sigma =0.829$, $\alpha =8^\circ$, $Re=5.07\times 10^{5}$)

Fig.6Angle-view of cavity shedding pattern (iso-surface of $\alpha_{\rm V}=0.9$) for $\sigma =0.829$, $\alpha =8^\circ$ and $Re=5.07\times 10^{5}$ during a typical cycle of original hydrofoil

图7

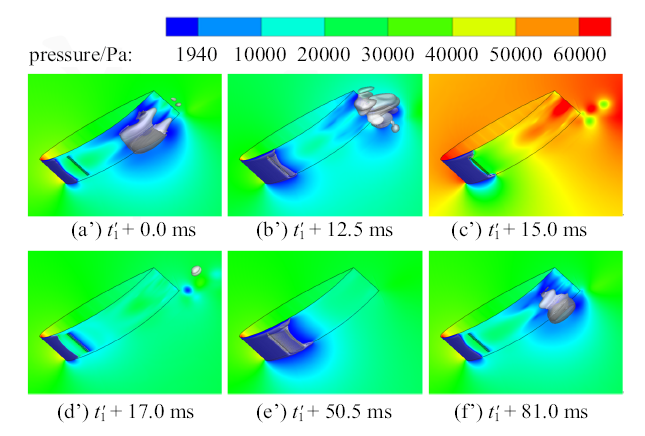新窗口打开|下载原图ZIP|生成PPT
图7一个典型周期内绕射流水翼的空化脱落的斜视视角($\alpha_{\rm V}=0.9$等值面及压力云图分布) ($\sigma =0.829$, $\alpha =8^\circ$, $Re=5.07\times 10^{5}$, $C_\mu=0.0115$)

Fig.7Angle-view of cavity shedding pattern (iso-surface of $\alpha_{\rm V}=0.9$) for $\sigma =0.829$, $\alpha =8^\circ$ and $Re=5.07\times 10^{5}$ during a typical cycle of hydrofoil with jet ($C_\mu=0.0115$)



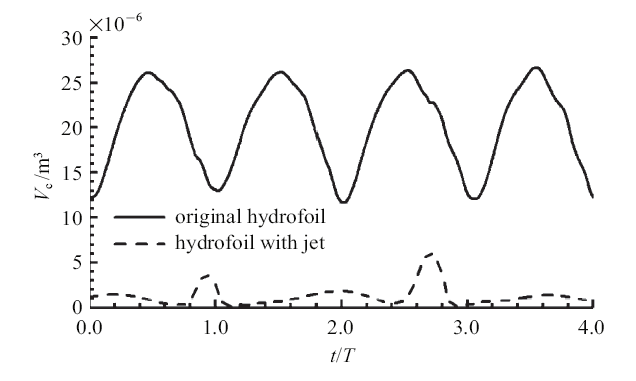
对比图6和图7发现射流改善了低压区的压力分布, 对空泡体积的大小有明显的抑制作用. 为定量分析射流对抑制空化的影响程度, 说明射流对空化发展具有明显的抑制作用. 如图8所示, 对流场中的汽相体积分数进行体积积分,最终发现原始水翼上空泡的时均体积为19.7 cm$^{3}$, 而相同工况下, 射流水翼上空泡的时均体积仅为1.28 cm$^{3}$, 射流水翼上的空泡时均体积为对应工况原始水翼的1/15.

图8

新窗口打开|下载原图ZIP|生成PPT
图8原始水翼与射流水翼的空泡体积对比($\sigma =0.829$, $\alpha =8^\circ$, $Re=5.07\times 10^{5}$)

Fig.8Comparison of vapor volume of original hydrofoil and hydrofoil with jet $\sigma =0.829$, $\alpha =8^\circ$ and $Re=5.07\times 10^{5}$

2.2 射流对水动力特性的影响

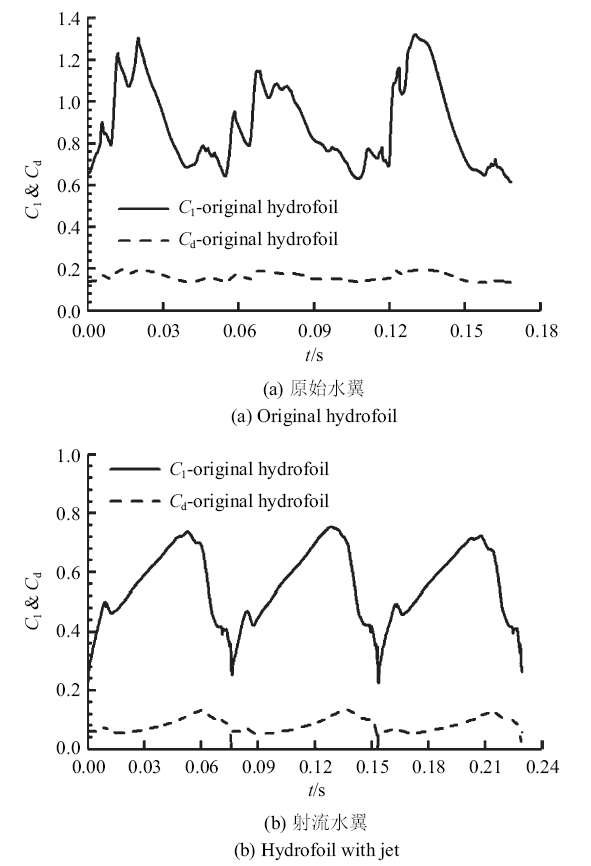
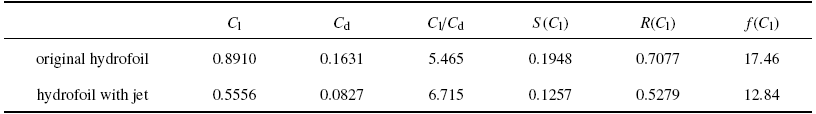
图9给出了不同水翼在实验工况下时序的升、阻力系数随时间的变化. 相比于原始水翼, 射流水翼的时均升、阻力系数均有减小, 且最大的升、阻力系数也有不同程度地降低, 且升力系数变化周期延长, 说明射流使水翼的水动力性能更加平稳. 结合表2得出, 相较于原始水翼, 射流水翼的时均升力系数减小37.6${\%}$, 时均阻力系数减小了49.3${\%}$, 时均阻力系数下降明显意味着射流有良好的减阻效果. 时均升阻比提高了22.9${\%}$, 极大地提高了水翼的水动力特性, 同时由射流水翼的升力系数波动的标准差和极差减小, 也能看出来射流能够使水翼周围的流动保持平稳的水动力性能.

图9

新窗口打开|下载原图ZIP|生成PPT
图9三个典型周期原始水翼和射流水翼的时均升阻力系数对比

Fig.9Comparison of predicted time evolution of the lift and drag coefficients of original hydrofoil and hydrofoil with jet in three typical cycles



Table 2
表2
表2原始水翼和射流水翼水动力性能对比
Table 2Comparison of hydrodynamic performance of original hydrofoil and hydrofoil with jet
Note: $C_{\rm l}$ is time-averaged lift coefficient; $C_{\rm d}$ is time-averaged drag coefficient; $C_{\rm l}$/$C_{\rm d}$ is time-averaged lift to drag ratios; $S(C_{\rm l})$ is standard deviation of lift coefficient; $R(C_{\rm l})$ is range of lift coefficient; $f(C_{\rm l})$ is frequency of lift coefficient.}

新窗口打开|下载CSV

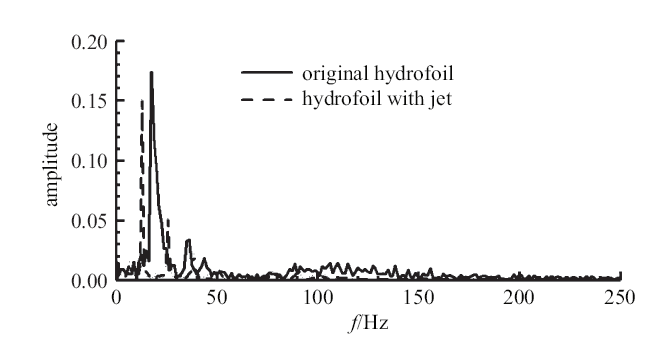
图10展示了原始水翼和射流水翼的快速傅里叶变换结果, 原始水翼的一阶空泡脱落频率为17.46 Hz, 而射流水翼的一阶空泡脱落频率为12.84 Hz. 频谱分析中的一阶频率即为云状脱落的主导频率, 由此可知: 在采用射流控制空化后, 水翼吸力面上的云空泡脱落频率和其引起的振幅都减小, 其中频率相较于原始水翼减小了26.2${\%}$, 由云状空泡脱落引起的振幅减小了9.1${\%}$. 在图10中, 原始水翼和射流水翼升力系数对应的二阶频率分别为36.16 Hz和25.69 Hz, 可以看出二阶频率大致为一阶频率的两倍, 说明空化引起的水翼的振动具有谐波性. 除主峰以外的其他较小的峰值与所观测到的除主空泡以外的小空泡脱落相关, 称为谐波频率. 以上分析可知射流水翼的二阶频率较原始水翼而言也减小28.9${\%}$, 证明射流的存在改变了水翼空化的周期性, 使得空化发展的速度减缓.

图10

新窗口打开|下载原图ZIP|生成PPT
图10原始水翼和射流水翼的升力系数快速傅里叶变换

Fig.10Fast Fourier transform (FFT) diagram of the lift coefficients fluctuations for original hydrofoil and hydrofoil with jet



空泡脱落频率的减小证实了射流抑制空泡脱落的有效性, 而回射流强度是影响空泡脱落的重要因素, 为此对回射流强度进行量化分析, 来分析射流对于回射流的影响^[34]. 采用无量纲数$C_{\rm re}$来定义回射流强度, 具体表达式如下

(15) $\begin{eqnarray} C_{\rm re}=\frac{P-P_{\rm v}}{P_{\rm v}}\frac{\rho_{\rm l}vL}{\mu_1}=\lt(\frac{P}{P_{\rm v}}-1)Re_1 \end{eqnarray}$
其中, $P$为回射流初始段当地压力, $v$取为回射流平均速度, $L$为回射流和空泡接触的弦向长度, $Re_{\rm l}$即为以回射流速度$v$为特征速度, 回射流和空泡接触长度$L$为特征长度的雷诺数.

$C_{\rm re}$的大小影响着空泡的形态, 当$C_{\rm re}$为10$^{4}$量级时, 流场中的空化形态为片空化; 当$C_{\rm re}$为10$^{5}$量级时, 流场中的空化形态为云空化. 经计算原始水翼吸力面上的$C_{\rm re}$为$5.75\times 10^{5}$, 而射流水翼吸力面上的$C_{\rm re}$仅为$1.18\times 10^{4}$. 故通过回射流强度分析的空化形态与图6和图7相符, 并证明了射流有效减弱了回射流强度, 抑制了空化的发展与脱落.

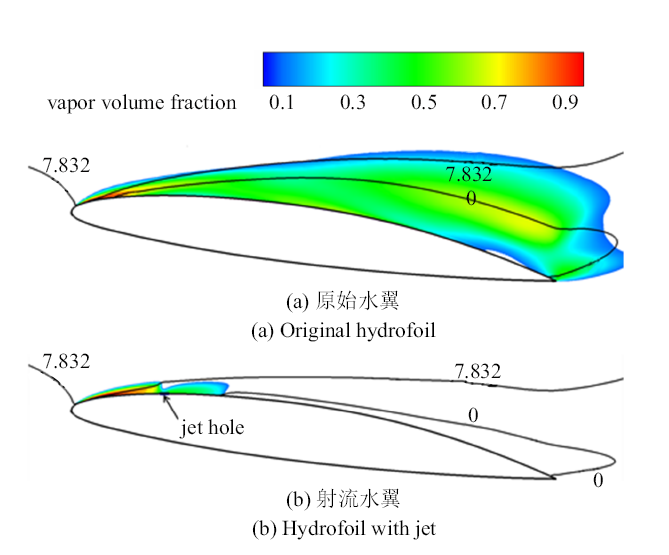
如图11所示选取水翼1/2展长处截面, 分析其速度分布主要特征, 图11分别给出了绕原始水翼和射流水翼的时均空化区形态和时均速度边界层(速度为7.832 m/s标识码线与水翼吸力面之间区域)及回射流区(速度为0的等值线和水翼吸力面之间区域)分布. 由速度边界层的分布可以看出, 在$x=0.19c$位置设置射流, 能有效减小边界层的厚度, 同时回射流区域相对原始水翼也大大减小. 如图11(b)所示, 在射流水翼吸力面上由于射流的存在使得时均边界层在$x=0.19c$处突然跃升了45${\%}$, 但是与图11(a)中的原始水翼相比较, 时均边界层在$x=0.19c$处的厚度仍减小30.5${\%}$. 水翼吸力面上的边界层厚度减小, 说明近壁面流动的抗逆压梯度的能力增加, 使得回射流更难以达到附着型空泡的前缘, 从而抑制了大空泡的脱落.

图11

新窗口打开|下载原图ZIP|生成PPT
图11时均空化形态和时均速度边界层分布对比

Fig.11Comparison of time-averaged cavitation patterns and distribution of time-averaged velocity boundary layer



如图12分别给出了绕原始水翼和射流水翼, 吸力面上沿弦长分布的压力脉动. 对于原始水翼, 结合图6可以看出, 一个周期内低压区覆盖整个吸力面距水翼前缘$x=0.19c$至$x=0.75c$的范围均为低压区, 水翼尾缘存在大量空泡脱落, 所以水翼尾缘压力脉动剧烈. 向流场注入射流后, 结合图7可以看出低压区面积大幅减小, 对应到吸力面上特征位置的压力脉动分布可以看出, 射流后监测点的压力显著提高. 尽管射流后尾缘依然存在空泡脱落现象, 但如图12所示, 水翼表面压力脉动频率明显较原始水翼的脉动频率更小, 即空泡脱落周期减小, 且最大压力脉动幅值也小于原始水翼. 射流水翼吸力面上的压力较原始水翼明显提高, 压力的提高极大地减缓了空化发展的速度. 原始水翼的尾缘与前缘存在着极大的压差, 导致水翼吸力面存在着极大的逆压梯度, 存在的逆压梯度是导致回射流产生的主要原因. 而在射流水翼吸力面上, 不同位置之间的压差减小, 所以这也使得射流水翼吸力面上的回射流衰弱, 进而抑制了空泡的脱落.

图12

新窗口打开|下载原图ZIP|生成PPT
图12原始水翼和射流水翼吸力面不同位置压力脉动对比

Fig.12Comparisons of pressure fluctuations at different positions on suction sides of original hydrofoil and hydrofoil with jet

3 结 论

本文利用数值手段分析了绕NACA66(MOD)水翼的云状空化流动特性, 对比了原始水翼和射流水翼在流动过程中的空化形态演化规律, 分析了射流对流场空化特性和水力学特性的影响, 通过对速度边界层以及回射流强度的对比, 揭示了主动射流对空化抑制的有效性和抑制机理.

(1)射流减小了水翼表面低压区范围, 使水翼吸力面上的空泡体积减小了14/15; 射流减小了水翼周围的逆压梯度, 使得回射流强度显著降低. 无量纲回射流强度, 较原始水翼明显减小, 对应的空化形态也由云空化转变为片空化, 显著抑制了空化的发展.

(2)向流场注入射流后, 相较于原始水翼, 水翼的升阻比提高了22.9${\%}$, 吸力面上的云空泡脱落频率减小了26.2${\%}$, 极大地改善了水翼动力学特性.

(3)射流使得水翼吸力面上的边界层厚度减薄, 近壁面流动抗逆压梯度的能力增强, 回射流更难以达到附着型空泡的前缘, 从而抑制了大空泡的脱落.

参考文献 View Option 原文顺序
文献年度倒序
文中引用次数倒序
被引期刊影响因子

[1] Pendar MR, Roohi E . Investigation of cavitation around 3D hemispherical head-form body and conical cavitators using different turbulence and cavitation models
Ocean Engineering, 2016,112:287-306
DOIURL [本文引用: 1]

[2] 王畅畅, 王国玉, 黄彪 . 空化可压缩流动空穴溃灭激波特性研究
力学学报, 2018,50(5):990-1002
[本文引用: 1]

( Wang Changchang, Wang Guoyu, Huang Biao . Numerical simulation of shock wave dynamics in transient turbulent cavitating flows
Chinese Journal of Theoretical and Applied Mechanics, 2018,50(5):990-1002 (in Chinese))
[本文引用: 1]

[3] 李晓俊 . 离心泵叶片前缘空化非定常流动机理及动力学特性研究
[博士论文]. 镇江: 江苏大学, 2013
[本文引用: 1]

( Li Xiaojun . Mechanism and unsteady dynamic characteristics of leading edge cavitation in a centrifugal pump
[PhD Thesis]. Zhenjiang: Jiangsu University, 2013 (in Chinese))
[本文引用: 1]

[4] Li CY, Ceccio SL . Interaction of single travelling bubbles with the boundary layer and attached cavitation
Journal of Fluid Mechanics, 1996,322:329-353
DOIURL [本文引用: 1]

[5] Chen YL, Heister SD . Modeling hydrodynamic nonequilibrium in cavitating flows
Journal of Fluids Engineering, 1996,118(1):172-178
DOIURL [本文引用: 1]

[6] Huang B, Wang GY . Experimental and numerical investigation of unsteady cavitating flows through a 2D hydrofoil
Science China Technological Science, 2011,54(7):1801-1812
[本文引用: 1]

[7] 张洋, 陈科, 尤云祥 等. 浮力气泡对水平壁面的回弹动力学特性
力学学报, 2019,51(5):1285-1295
[本文引用: 1]

( Zhang Yang, Chen Ke, You Yunxiang , et al. Bouncing behaviors of a buoyancy-driven bubble on a horizontal solid wall
Chinese Journal of Theoretical and Applied Mechanics, 2019,51(5):1285-1295 (in Chinese))
[本文引用: 1]

[8] Huang B, Zhao Y, Wang GY . Large eddy simulation of turbulent vortex-cavitation interactions in transient sheet/cloud cavitating flows
Computers & Fluids, 2014,92:113-124
DOIURLPMID [本文引用: 1]
Active development of quantum informational components such as quantum computers and quantum key distribution systems requires parameter characterization of single photon detectors. A key property of the single photon detectors is detection efficiency. One of the methods of the detection efficiency measurement, as listed in the international standard ETSI, is the reference-free twin-photon-based Klyshko method. The signal-to-noise ratio (SNR) of this method depends on the combination of the pump wavelength, the nonlinear crystal's axis angle, and the type of detector's sensitive element. When the combination is difficult, one has to deal with the low SNR of the detector counts measurement. To gain the high SNR, one has to average the long record complicated with the "random telegraph signal" noise. This type of noise exhibits high spectral density at a zero frequency, where simple averaging works. The heterodyne based method we have proposed is to perform averaging at the higher frequency of the modulation introduced to the standard Klyshko measurement scheme. The method was numerically simulated and experimentally tested. The 14 times improvement in SNR for the proposed method relative to the simple averaging was demonstrated by the numerical simulation and confirmed experimentally.

[9] Ye YH, Li GJ . Modeling of hydrodynamic cavitating flows considering the bubble- bubble interaction,
International Journal of Multiphase Flow, 2016,84:155-164
DOIURL [本文引用: 1]

[10] Anderlini A, Salvetti MV, Agresta A , et al. Stochastic sensitivity analysis of numerical simulations of injector internal flows to cavitation modeling parameters
Comput Fluids, 2019,183:130-147
DOIURL [本文引用: 1]

[11] Congedo PM, Goncalves E, Rodio MG . About the uncertainty quantification of turbulence and cavitation models in cavitating flows simulations
European Journal of Mechanics—B/Fluids, 2015,53:190-204
DOIURL [本文引用: 1]

[12] Huang B, Wang GY, Zhao Y . Numerical simulation unsteady cloud cavitating flow with a filter-based density correction model
Journal of Hydrodynamics, 2014,26(1):26-36
DOIURL [本文引用: 3]
In this paper, various turbulence closure models for unsteady cavitating flows are investigated. The filter-based model (FBM) and the density correction model (DCM) were proposed to reduce the turbulent eddy viscosities in d turbulent cavitating flow based on the local meshing resolution and the local fluid density, respectively. The effects of the resolution control parameters in the FBM and DCM models are discuised. It is shown that the eddy viscosity near the cavity closure region can significantly influence the cavity shapes and the unsteady shedding pattern of the cavitating flows. To improve the predictions, a Filter-Based Density Correction model (FBDCM) is proposed, which blends the FBM and DCM models according to the local fluid density. The new FBDCM model can effectively represent the eddy viscosity, according to the multi-phase characteristics of the unsteady cavitating flows. The experimental validations regarding the force analysis and the unsteady cavity visualization show that good agreements with experimental visualizations and measurements are obtained by the FBDCM model. For the FBDCM model, the attached cavity length and the resulting hydrodynamic characteristics are subsequently affected by the detail turbulence modeling parameters, and the model is shown to be effective in improving the overall predictive capability.

[13] Johansen ST, Wu JY, Shyy W . Filter-based unsteady RANS computations
International Journal of Heat and Fluid Flow, 2004,25(1):10-21
DOIURL [本文引用: 2]

Abstract

The Reynolds-averaged Navier–Stokes (RANS) approach has been popular for engineering turbulent flow computations. The most widely used ones, such as the k−ε two-equation model, have well-recognized deficiencies when treating time dependent flow fields. To identify ways to improve the predictive capability of the current RANS-based engineering turbulence closures, conditional averaging is adopted for the Navier–Stokes equation, and one more parameter, based on the filter size, is introduced into the k−ε model. The sub-filter stresses are constructed directly by using the filter size and the conventional turbulence closure. The filter is decoupled from the grid, making it possible to obtain grid independent solutions with a fixed filter scale. The model is assessed in transient, planar turbulent wake flow simulations over a square cylinder utilizing progressively refined grid. In comparison to the standard k−ε model, overall, the filter-based model is shown to improve the predictive capability considerably.

[14] Coutier-Delgosha O, Reboud JL, Delannoy Y . Numerical simulation of the unsteady behaviour of cavitating flows
International Journal for Numerical Methods in Fluids, 2003,42(5):527-548
[本文引用: 2]

[15] Yu A, Ji B, Huang RF , et al. Cavitation shedding dynamics around a hydrofoil simulated using a filter-based density corrected model
Science China Technological Sciences, 2015,58(5):864-869
DOIURL [本文引用: 1]

[16] Timoshevskiy MV, Zapryagaev II, Pervunin KS , et al. Manipulating cavitation by a wall jet: Experiments on a 2D hydrofoil
International Journal of Multiphase Flow, 2018,99:312-328
DOIURL [本文引用: 2]

[17] Chatterjee D, Arakeri VH . Towards the concept of hydrodynamic cavitation control
Journal of Fluid Mechanics, 1997,332:377-394
DOIURL [本文引用: 1]

[18] Chahine GL, Frederick GF, Bateman RD . Propeller tip vortex cavitation suppression using selective polymer injection
Journal of Fluids Engineering, 1993,115(3):497-503
DOIURL [本文引用: 1]

[19] Chang N, Ganesh H, Yakushiji R , et al. Tip vortex cavitation suppression by active mass injection
Journal of Fluids Engineering, 2011,133(11):111301
DOIURL [本文引用: 1]

[20] M$\ddot{a}$kiharju SA, Ganesh H, Ceccio SL . Effect of non-condensable gas injection on cavitation dynamics of partial cavities
//Farhat M, Müller A eds. Journal of Physics: Conference Series, the 9th International Symposium on Cavitation (CAV2015), Lausanne, 2015, Bristol: Ashton A, 2015. 012161
[本文引用: 1]

[21] Wang W, Yi Q, Wang YY , et al. Adaptability research of hydrofoil surface water injection on cavitation suppression
Journal of Drainage and Irrigation Machinery Engineering, 2017,35(6):461-466, 480
[本文引用: 1]

[22] Leger AT, Ceccio SL . Examination of the flow near the leading edge of attached cavitation. Part 1. Detachment of two-dimensional and axisymmetric cavities
Journal of Fluid Mechanics, 1998,376:61-90
DOIURL [本文引用: 1]

[23] Kadivar E, Moctar O E, Javadi K . Investigation of the effect of cavitation passive control on the dynamics of unsteady cloud cavitation
Applied Mathematical Modelling, 2018,64:333-356
DOIURL [本文引用: 1]

[24] 王巍, 唐滔, 卢盛鹏 等. 水翼吸力面布置凹槽抑制空化研究
农业工程学报, 2019,35(2):40-47
URL [本文引用: 1]
空化引起不同程度振动、冲击和噪声，加剧物体表面空蚀，使结构提早发生疲劳。为有效抑制和延缓空化发生和空泡脱落，该文提出了在水翼吸力面布置凹槽的方法，旨在通过水翼表面结构的改变来实现空化流动的调节。在数值模拟研究中，采用Realizable k-ε湍流模型和Schnerr-Sauer空化模型，围绕8°攻角下NACA66 (MOD)水翼，开展不同空化数、凹槽尺度和凹槽位置对二维水翼空化流场的动力学特性研究，并进一步分析了水翼表面特殊结构抑制空化的机理。结果表明：当片空化发生时，凹槽布置在距水翼前缘0.32弦长位置时，能降低空泡振荡频率，提高水翼水动力性能；当云空化发生时，适当的凹槽表面构型能够使水翼吸力面边界层变薄，边界层分离点滞后，水翼尾缘回流区减薄，吸力面低压区减小，证明了凹槽表面构型对空化抑制的适用性。然而，在水翼吸力面布置凹槽，虽然可以降低水翼表面边界层的厚度，增强抗逆压能力，但却触发了凹槽附近区域回射流的加速。因此，只有当抗逆压梯度能力大于回射流冲击时，才可以实现对空化流动的抑制。该研究成果扩大了空化流动的被动控制方法研究范围，为水力机械空化抑制技术提供了参考。
( Wang Wei, Tang Tao, Lu Shengpeng , et al. Investigation of cavitation suppression by arranging pits on hydrofoil suction side
Transactions of the Chinese Society of Agricultural Engineering, 2019,35(2):40-47 (in Chinese))
URL [本文引用: 1]
空化引起不同程度振动、冲击和噪声，加剧物体表面空蚀，使结构提早发生疲劳。为有效抑制和延缓空化发生和空泡脱落，该文提出了在水翼吸力面布置凹槽的方法，旨在通过水翼表面结构的改变来实现空化流动的调节。在数值模拟研究中，采用Realizable k-ε湍流模型和Schnerr-Sauer空化模型，围绕8°攻角下NACA66 (MOD)水翼，开展不同空化数、凹槽尺度和凹槽位置对二维水翼空化流场的动力学特性研究，并进一步分析了水翼表面特殊结构抑制空化的机理。结果表明：当片空化发生时，凹槽布置在距水翼前缘0.32弦长位置时，能降低空泡振荡频率，提高水翼水动力性能；当云空化发生时，适当的凹槽表面构型能够使水翼吸力面边界层变薄，边界层分离点滞后，水翼尾缘回流区减薄，吸力面低压区减小，证明了凹槽表面构型对空化抑制的适用性。然而，在水翼吸力面布置凹槽，虽然可以降低水翼表面边界层的厚度，增强抗逆压能力，但却触发了凹槽附近区域回射流的加速。因此，只有当抗逆压梯度能力大于回射流冲击时，才可以实现对空化流动的抑制。该研究成果扩大了空化流动的被动控制方法研究范围，为水力机械空化抑制技术提供了参考。

[25] Kawanami Y, Kato H, Yamaguchi H , et al. Mechanism and control of cloud cavitation
Journal of Fluids Engineering, 1997,119(4):788-794
DOIURLPMID [本文引用: 1]
Shock wave lithotripsy has generally been a first choice for kidney stone removal. The shock wave lithotripter uses an order of microsecond pulse durations and up to a 100 MPa pressure spike triggered at approximately 0.5-2 Hz to fragment kidney stones through mechanical mechanisms. One important mechanism is cavitation. We proposed an alternative type of lithotripsy method that maximizes cavitation activity to disintegrate kidney stones using high-intensity focused ultrasound (HIFU). Here we outline the method according to the previously published literature (Matsumoto et al., Dynamics of bubble cloud in focused ultrasound. Proceedings of the second international symposium on therapeutic ultrasound, pp 290-299, 2002; Ikeda et al., Ultrasound Med Biol 32:1383-1397, 2006; Yoshizawa et al., Med Biol Eng Comput 47:851-860, 2009; Koizumi et al., A control framework for the non-invasive ultrasound the ragnostic system. Proceedings of 2009 IEEE/RSJ International Conference on Intelligent Robotics and Systems (IROS), pp 4511-4516, 2009; Koizumi et al., IEEE Trans Robot 25:522-538, 2009). Cavitation activity is highly unpredictable; thus, a precise control system is needed. The proposed method comprises three steps of control in kidney stone treatment. The first step is control of localized high pressure fluctuation on the stone. The second step is monitoring of cavitation activity and giving feedback on the optimized ultrasound conditions. The third step is stone tracking and precise ultrasound focusing on the stone. For the high pressure control we designed a two-frequency wave (cavitation control (C-C) waveform); a high frequency ultrasound pulse (1-4 MHz) to create a cavitation cloud, and a low frequency trailing pulse (0.5 MHz) following the high frequency pulse to force the cloud into collapse. High speed photography showed cavitation collapse on a kidney stone and shock wave emission from the cloud. We also conducted in-vitro erosion tests of model and natural kidney stones. For the model stones, the erosion rate of the C-C waveform showed a distinct advantage with the combined high and low frequency waves over either wave alone. For optimization of the high frequency ultrasound intensity, we investigated the relationship between subharmonic emission from cavitation bubbles and stone erosion volume. For stone tracking we have also developed a non-invasive ultrasound theragnostic system (NIUTS) that compensates for kidney motion. Natural stones were eroded and most of the resulting fragments were less than 1 mm in diameter. The small fragments were small enough to pass through the urethra. The results demonstrate that, with the precise control of cavitation activity, focused ultrasound has the potential to be used to develop a less invasive and more controllable lithotripsy system.

[26] Wang W, Lu SP, Xu RD , et al. Numerical study of hydrofoil surface jet flow on cavitation suppression
Journal of Drainage and Irrigation Machinery Engineering, 2017,35(10):829-834
DOIURLPMID [本文引用: 1]
A method of water injection to flow field using distributed holes on the suction surface of hydrofoil is presented in this article to control cavitation flow. Modified renormalization group k-ε turbulence model is coupled with full-cavitation model to calculate periodical cavitation patterns and the dynamic characteristics of the NACA66(MOD) hydrofoil. Water injection is found to be highly effective for cavitation suppression. The cavitation suppression effect of distributed regulation of jet holes and porosities along three-dimensional spanwise hydrofoil is also investigated. The appropriate porosities of single row spanwise jet holes and optimal jet position of double row jet holes are revealed for both cavitation suppression and good hydrodynamic performance. Double row jet holes setting in forward trapezoidal arrangement shows great potential for cavitation suppression and hydrodynamic performance. This research provides a method of water injection to flow field to actively control cavitation, which will facilitate development of engineering designs.

[27] Coutier-Delgosha O, Devillers JF, Leriche M , et al. Effect of wall roughness on the dynamics of unsteady cavitation
Journal of fluids Engineering, 2005,127(4):726-733
DOIURL [本文引用: 1]

[28] Lu SP, Wang W, Hou TF , et al. Experiment research on cavitation control by active injection//Katz J. Proceedings of the 10th International Symposium on Cavitation(CAV2018), The 10th International Symposium on Cavitation(CAV2018), Baltimore, 2018, New York: ASME Press, 2018: 363-368
[本文引用: 1]

[29] 张佳悦, 李达钦, 吴钦 等. 航行体回收垂直入水空泡流场及水动力特性研究
力学学报, 2019,51(3):803-812
[本文引用: 1]

( Zhang Jiayue, Li Daqin, Wu Qin , et al. Numerical investigation on cavity structures and hyrodynamics of the vehicle during vertical water-entry
Chinese Journal of Theoretical and Applied Mechanics, 2019,51(3):803-812 (in Chinese))
[本文引用: 1]

[30] Coutier-Delgosha O, Fortes-patella R, Reboud JL . Evaluation of the turbulence model influence on the numerical simulations of unsteady cavitation
Journal of Fluids Engineering, 2003,125(1):38-45
DOIURL [本文引用: 1]

[31] Zwart PJ, Gerber AG, Belamri T . A two-phase flow model for predicting cavitation dynamics//Fifth International Conference on Multiphase Flow
Yokohama, Japan, 2004,152
[本文引用: 1]

[32] Leroux JB, Astolfi JA, Billard JY . An experimental study of unsteady partial cavitation
Journal of Fluids Engineering, 2004,126(1):94-101
DOIURL [本文引用: 1]

[33] 李聪洲, 张新曙, 胡晓峰 等. 高雷诺数下多柱绕流特性研究
力学学报, 2018,50(2):233-243
[本文引用: 1]

( Li Congzhou, Zhang Xinshu, Hu Xiaofeng , et al. The study of flow past multiple cylinders at high reynolds numbers
Chinese Journal of Theoretical and Applied Mechanics, 2018,50(2):233-243 (in Chinese))
[本文引用: 1]

[34] 王巍, 徐瑞铎, 羿琦 等. 回射流强度对水翼表面空化形态的影响
排灌机械工程学报, 2016,34(11):921-926, 940
[本文引用: 1]

( Wang Wei, Xu Ruiduo, Yi Qi , et al. Influence of re-entrant jet strength on cavitation characteristics of hydrofoil
Journal of Drainage and Irrigation Machinery Engineering, 2016,34(11):921-926, 940 (in Chinese))
[本文引用: 1]