滑轮绳索系统中动态节点绳索单元 1)

删除或更新信息，请邮件至freekaoyan#163.com(#换成@)

本站小编 Free考研考试/2022-01-01

齐朝晖^,²⁾, 国树东, 卓英鹏大连理工大学工程力学系, 大连 116024

ROPE ELEMENTS WITH MOVING NODES IN ROPE-PULLEY SYSTEMS ¹⁾

Qi Zhaohui^,²⁾, Guo Shudong, Zhuo YingpengDepartment of Engineering Mechanics, Dalian University of Technology, Dalian 116024, China

通讯作者: 2) 齐朝晖, 教授, 主要研究方向: 多体系统动力学. E-mail:zhaohuiq@dlut.edu.cn

收稿日期:2019-06-29接受日期:2019-09-20网络出版日期:2019-09-20

基金资助:

1) 国家自然科学基金资助项目.11872137
1) 国家自然科学基金资助项目.91748203

Received:2019-06-29Accepted:2019-09-20Online:2019-09-20
作者简介 About authors

摘要
解除了传统有限元方法中单元节点与物质点固结的假设, 建立了空间点的速度和加速度与相应物质点的速度和加速度之间的数学关系, 强调了虚功率原理中出现的速度和加速度皆为物质速度和物质加速度. 在此基础上构造了单元节点既不与空间坐标固定也不与物质坐标固定的动态节点绳索单元. 根据滑轮绳索系统的特点, 选取绳索出入绳点的弧长坐标、出入绳角、面外摆角以及拉伸应变等空间参数描述了滑轮绳索系统的运动. 将绳索与滑轮以及绳索与卷筒之间的相互作用合理简化为物质速度间的约束条件, 避免了传统方法中接触力计算不收敛、效率低等缺点. 所提方法可精细求解绳索与滑轮间接触边界点位置和卷筒入绳点在卷筒上的运动、滑轮的中心和其连体基的运动、绳索出入滑轮和卷筒时空间方位的变化以及绳索上任意点的拉力变化等细节. 可为含绳索机械系统的力学分析提供新的理论基础. 所用的解除单元节点与物质点绑定的理论具有一定的普适性, 可为有限元方法的理论和应用提供参考.
关键词： 多柔体系统动力学;有限元方法;滑轮绳索系统;动态节点;空间描述

Abstract
In traditional finite element methods, a common hypothesis is the nodes of an element being fixed with material points, which place restrictions on the description of a system. In this paper, this hypothesis is released and a new kind of rope element with nodes being no longer fixed with either spatial coordinates or material coordinates is presented. The theory of obtaining material velocity and acceleration of a point from the corresponding spatial velocity and acceleration of the point is established. The velocities and accelerations in the principle of virtual power should being material velocities and accelerations respectively is emphasized and applied. According to the feature of a rope-pulley system, its movement can be described by a new group of variables, such as arc-lengths, azimuthal angles and strains of ropes at entrance and exit contacting points. Instead of traditional methods, conditions of contact between rope and pulley are modeled as the material velocity of a point on the contacting rope being equal to the corresponding point on the pulley. By means of the presented methods, some obstacles, such as frequently divergence and high time consuming that resulting from traditional finite element methods can be removed. Motion equation of the rope-pulley system is derived by the principle of virtual power. Arc-length coordinates, positions of contact boundary points, the movement of pulley centers and their body-fixed reference coordinate systems, shape and positions changing of ropes as well as the tension forces in every point of a rope, can be obtained high precisely. The presented method can provide a new theoretical basis for analysis of mechanical systems with ropes and pulleys. The presented theory of using spatial points as describing variables to replace traditional material points is of applicable widely. It can be a reference for theory and application of finite element methods.
Keywords：dynamics of multi-flexible bodies;finite element methods;rope-pulley system;moving node;spatial description

PDF (981KB)元数据多维度评价相关文章导出EndNote|Ris|Bibtex 收藏本文
本文引用格式
齐朝晖, 国树东, 卓英鹏. 滑轮绳索系统中动态节点绳索单元 ¹⁾. 力学学报[J], 2019, 51(6): 1856-1871 DOI:10.6052/0459-1879-19-168
Qi Zhaohui, Guo Shudong, Zhuo Yingpeng. ROPE ELEMENTS WITH MOVING NODES IN ROPE-PULLEY SYSTEMS ¹⁾. Chinese Journal of Theoretical and Applied Mechanics[J], 2019, 51(6): 1856-1871 DOI:10.6052/0459-1879-19-168

引言

建立复杂系统的力学模型需要解决以下两个核心问题: 选取系统描述变量以及依据物理定律建立描述变量演化方程. 如果系统中含有可变形物体, 如何用有限个参数描述这类物体的运动则是选取系统变量时无法回避的共性问题.

有限元方法是解决这一共性问题的最普遍方法^[1-3]. 它的基本思想是: 将连续体划分为有限个单元的集合, 用单元节点参数近似单元域内的场函数. 节点参数所应满足的方程可由相应的变分原理导出^[4]. 然而, 在一个区域内的积分等于组成该区域的各个子区域内积分之和是有条件的, 单元形函数必须使被积函数中出现的最高阶导数在单元边界处的函数值为有限值. 很多情况下, 满足这种连续性条件的单元形函数是很难构造的. 此时, 将应力场也作为独立场函数可使被积函数中出现的导数阶次降低^[5]. 有限元法自问世以来, 经过半个多世纪的发展, 在理论和应用两个方面都已日臻成熟^[6-13], 但有一个基本假设却仍然如初: 在流体力学中单元节点与空间点绑定, 在固体力学中单元节点与物质点固结^[14]. 众所周知, 描述一个系统的运动通常可归结为描述系统中有限个关键点的运动, 如果限定这些关键点必须与物质点或空间点绑定, 有时可在很大程度上限制了系统变量的选取方式, 增加问题的求解难度.

滑轮绳索系统由于具有质量轻, 动力传输范围广, 传输过程中能耗低等优点, 被广泛应用于起重机、机器人、航空航天等机械系统中^[15-22], 对这类机械系统的精确性和可靠性要求也越来越高. 例如, 用于核反应堆搭建和操作的核环吊系统, 必须在提升几百吨重物的过程中使重物的水平位移偏差控制在每10 m小于5 mm的范围内^[23]. 这些机械系统若要达到精确可靠的要求, 必须对滑轮绳索系统进行精确可靠的计算分析. 早期的绳索力学模型主要是采用集中质量模型, 它将绳索的分布质量凝聚到一系列离散质点上, 将绳索的弹性和阻尼效应模拟为连接相邻质点的弹簧和阻尼器^[24]. Kamman与Huston^[25]利用集中质量模型对船舶拖曳设备中的变长度绳索进行建模分析. Danilin等^[26]运用集中质量模型来对航天器绳索进行仿真. Russell和Anderson^[27]将绳索看作集中质量模型, 利用该模型对绳索系统的动态不稳定进行了研究. 绳索的有限段模型将绳索离散为由多个刚体组成的链状结构, 刚体之间采用球铰和弹簧进行连接^[28-30]. 通过弱化抗弯扭模量, 绳索也可看作为一种特殊的梁^[31].

现有文献尽管在细节上有所差异, 但因受制于单元节点固结于物质点的假定, 都采用了类似的求解策略: 将系统中所有的绳索离散为多个绳索单元; 从初始时刻起跟踪每个单元节点的运动以判断绳索是否与滑轮接触; 对处于接触状态的单元, 用弹簧阻尼接触力模型计算对应的接触力. 由于单元形函数不能高精度描述圆弧曲线, 只能通过缩小单元尺寸逼近滑轮上的一段圆弧, 往往需要很多单元才能模拟滑轮绳索的运动. 其次, 接触力计算需要实时判断绳索滑轮间接触条件, 单元形函数稍稍复杂一点就会大幅度地增加这一过程的难度,也经常发生不收敛现象.

2011年, 文献^[32]为研究物体在一维介质中的运动提出了任意欧拉$\!-\!$拉格朗日绝对节点坐标单元(ALE-ANCF). 这类单元解除了单元节点与物质点的绑定, 并很快在求解一些工程问题中得到了应用^[33-35]. 充分说明了采用空间点描述系统运动的必要性和可能性.

与纯粹的物质描述不同的是: 空间点的选取需要结合所研究问题的特征. 本文针对滑轮绳索系统的特点, 提出了一种节点不固结于绳索上物质点的绳索单元, 从而可依据绳索所处的空间位置划分绳索单元. 这种单元具备传统单元所没有的处理形状约束能力, 不仅更加精细地描述了滑轮绳索的运动, 也在很大程度上简化了滑轮绳索系统的分析过程.

1 物质速度和空间速度

绳索中一点在空间中的矢径${{ r}}$可看作为物质坐标$s$ (原始弧长)和时间$t$的函数,其速度可以描述为

(1)$\begin{eqnarray}{{\dot{ r}}} = \frac{\partial {{ r}}}{\partial t} +\frac{\partial {{ r}}}{\partial s}\dot{s}\end{eqnarray}$

如果物质坐标不随时间变化($\dot{s} = 0$), 则该点称为物质点, 否则称该点为空间点. 空间点是为更好地描述系统运动而采用的具有明确几何意义的点. 例如, 图~1所示的简单滑轮绳索系统中, 只要知道绳索与滑轮间的两个边界点(入绳点和出绳点)的运动, 就可了解系统运动的主要特征. 但这两个点的弧长坐标是随时间变化的, 因而是空间点.

与物质点不同的是: 空间点与系统描述方式相关, 因而带有一定的主观性. 例如, 图1所示系统中, 为描述滑轮两边绳索的形状, 需在这两段绳索上选取一些点. 为确保这些点在运动过程中始终位于滑轮两侧, 其弧长坐标也必须是变化的, 因而是空间点. 如果入绳点物质坐标为$s_1$, 出绳点物质坐标为$s_2 $, 固定端到入绳点间的绳索形状可通过$n + 1$个物质坐标分别为0, ${s_1 }/n$, ${2s_1 }/n$, $\ldots$, $s_1 $的空间点描述. 理论上这些点还可以采取其他方式选取. 为明确空间点的定义, 空间点的物质坐标要么本身就是描述变量一部分, 要么就是描述变量的明确函数.

图1

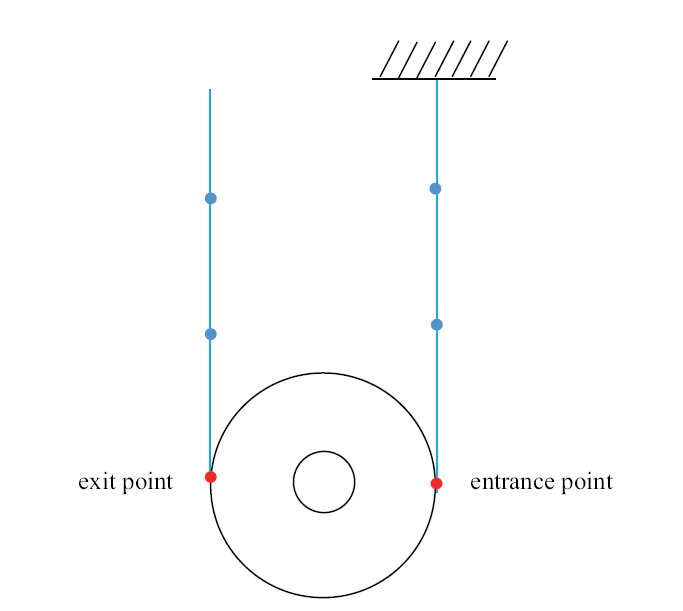新窗口打开|下载原图ZIP|生成PPT
图1空间点实例: 绳索与滑轮间的边界点

Fig.1An example of spatial points: Boundary points of a rope-pulley system

按定义, 方程(1)左端为空间点的速度$\!-\!$空间速度, 右端第一项${\partial {{ r}}}/{\partial t}$是固定$s$时矢径${{ r}}$的一阶变化率, 也就是坐标为$s$的物质点的速度$\!-\!$物质速度. 对方程求时间导数可得: 空间加速度

(2)$\begin{eqnarray}{{\ddot{ r}}} = \frac{\partial ^2{{ r}}}{\partial t^2} +2\frac{\partial ^2{{ r}}}{\partial s\partial t}\dot{s} +\frac{\partial ^2{{ r}}}{\partial s^2}\dot{s}^2 + \frac{\partial{{ r}}}{\partial s}\ddot{s}\end{eqnarray}$

等号右端第一项${\partial ^2{{ r}}}/{\partial t^2}$是固定$s$时矢径${{ r}}$的二阶变化率, 也就是坐标为$s$的物质点的加速度$\!-\!$物质加速度.

方程(1)和(2)建立了物质速度(加速度)和空间速度(加速度)之间的联系. 同时也表明了两者之间的显著差异. 为了从空间速度得到物质速度, 除了需要明确给出弧长坐标的变化率之外, 还需给出矢径对弧长的导数${{ {r}'}}$, 而为使物质速度在节点处连续, ${{ {r}'}}$必须连续. 同理, 为使物质加速度在节点处连续, ${{ {r}''}}$必须连续.

2 虚功率原理

虚功率原理是建立系统离散方程的物理基础. 值得强调的是: 由于虚功率原理源于能量守恒定律和以质点为对象的牛顿定律, 虚功率原理中的虚速度、加速度应是物质速度和物质加速度^[36-37], 其数学表达式可以写作

(3)$\begin{eqnarray}&&\int_0^L {\delta \left( {{\partial {{ r}}}/{\partial t}} \right)^{\rm T}{\partial ^2{{ r}}}/{\partial t^2}\rho {\rm d}s} + \\&&\qquad\int_0^L{\delta \left( {{\partial {{ \varepsilon }}}/{\partial t}} \right)^{\rm T}{{ \sigma }}{\rm d}s} =\delta p_f\end{eqnarray}$

其中, 等式右端为系统外力虚功率, 左端第一项为惯性力虚功率, 第二项为变形虚功率, ${{ \varepsilon }}$和${{ \sigma }}$分别为广义应变和广义应力.

如果忽略微小的抗弯扭效应, 绳索的广义应变

(4)$\begin{eqnarray}\varepsilon = \| {{ {r}'}} \| - 1\end{eqnarray}$

为简化符号, 除非另有说明, 上式及下文中变量上标``$'$''表示变量对$s$的偏导数. 与广义应变相应的广义应力可由抗拉模量$E_A $表示为

(5)$\begin{eqnarray}\sigma = E_A \varepsilon\end{eqnarray}$

为在模型中滤除高频分量, 可将式(3)中的应力替换为一小段时间内的平均应力^[38]

(6)$\begin{eqnarray}&& \bar{\sigma } = \frac{1}{h}\int_0^h {\left( {\sigma + \tau{\partial \sigma }/{\partial t} + \frac12\tau ^2{\partial ^2\sigma }/{\partial t^2}}\right){\rm d}\tau } =\\&&\qquad E_A \left( {\varepsilon +\frac12h{\partial\varepsilon }/{\partial t} +\frac16h^2{\partial ^2\varepsilon }/{\partial t^2}} \right)\end{eqnarray}$

其中, 常数$h$为光滑因子, 应变的物质速度和物质加速度分别为

(7)$\begin{eqnarray}&&{\partial \varepsilon }/{\partial t} = \dot{\varepsilon } - {\varepsilon }'\dot{s} \end{eqnarray}$

(8)$\begin{eqnarray}&&{\partial ^2\varepsilon }/{\partial t^2} = \ddot{\varepsilon } + {\varepsilon }''\dot{s}^2 - {\varepsilon }'\ddot{s} - 2{\dot{\varepsilon }}'\dot{s}\end{eqnarray}$

其中

(9)$\begin{eqnarray}&&\dot{\varepsilon } = ( {1 + \varepsilon } )^{ - 1}( {{ {r}'}} )^{\rm T}{{\dot{ {r}'}}}\end{eqnarray}$

(10)$\begin{eqnarray}&&\ddot{\varepsilon } = ( {1 + \varepsilon } )^{ - 1}\lt[{( {{ {r}'}} )^{\rm T}{{\ddot{ {r}'}}} + ( {{\dot{ {r}'}}} )^{\rm T}{{\dot{ {r}'}}} - \dot{\varepsilon }^2}]\end{eqnarray}$

$\varepsilon$的物质速度虚变分

(11)$\begin{eqnarray}\delta \left( {{\partial \varepsilon }/{\partial t}} \right) = \left( {1 + \varepsilon } \right)^{ - 1}\left( {{ {r}'}} \right)^{\rm T}\delta {{\dot{ {r}'}}} - {\varepsilon }'\delta \dot{s}\end{eqnarray}$

由方程(1)可知: 节点矢径物质速度的虚变分

(12)$\begin{eqnarray}\delta \left( {{\partial {{ r}}}/{\partial t}} \right) = \delta {{\dot{ r}}} - {{ {r}'}}\delta \dot{s}\end{eqnarray}$

3 滑轮间绳索单元

如图2所示, 将两滑轮间一段绳索按弧长坐标等分为$n$段, 各节点的矢径分别为${{ r}}_0 $, ${{ r}}_1 $, $\ldots$, ${{ r}}_n $, 两端点的弧长坐标分别为$s_0 $和$s_n $, 两端点矢径对弧长坐标的导数分别为${{ {r}'}}_0 $和${{ {r}'}}_n $. 这些节点参数组成的列阵${{ q}}_r = \left[ {{{ r}}_0 ;{{ r}}_1 ; \cdots ;{{ r}}_n ;{{ {r}'}}_0 ;{{ {r}'}}_n;s_0 ;s_n } \right]$.

图2

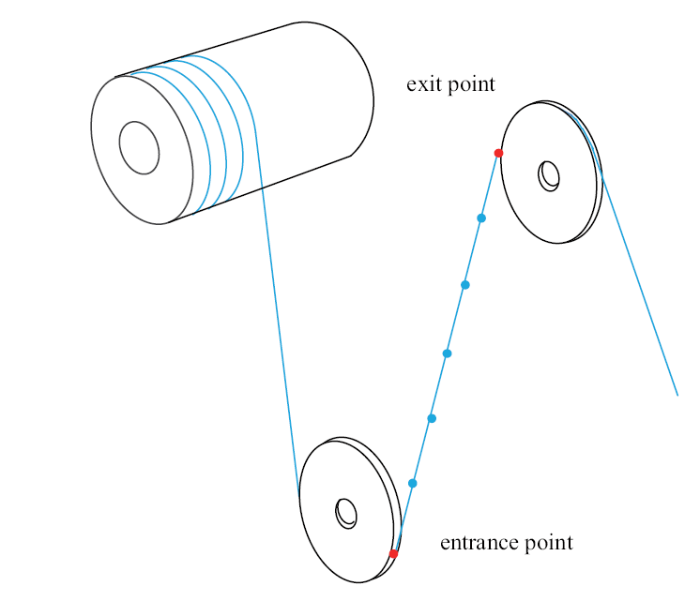新窗口打开|下载原图ZIP|生成PPT
图2滑轮间绳索单元节点$i$的弧长坐标

Fig.2A rope element between two pulleys

(13)$\begin{eqnarray}s_i = n^{ - 1}\left( {n - i} \right)s_0 + n^{ - 1}is_n\end{eqnarray}$

处于两节点间绳索上一点的矢径可用Hermite插值函数近似为

(14)$\begin{eqnarray}{{ r}} = N_1 {{ r}}_i + N_2 {{ r}}_{i + 1} + L(N_3 {{ {r}'}}_i + N_4 {{ {r}'}}_{i + 1} )\end{eqnarray}$

其中, $L = {\left( {s_n - s_0 } \right)}/n$, 形函数

(15)$\begin{eqnarray}&&N_1 = 1 - 3\xi ^2 + 2\xi ^3 \end{eqnarray}$

(16)$\begin{eqnarray}&&N_2 = 3\xi ^2 - 2\xi ^3 \end{eqnarray}$

(17)$\begin{eqnarray}&&N_3 = \xi - 2\xi ^2 + \xi ^3 \end{eqnarray}$

(18)$\begin{eqnarray}&&N_4 = \xi ^3 - \xi ^2 \end{eqnarray}$

归一化参数$\xi$定义为

(19)$\begin{eqnarray}\xi = L^{ - 1}( {s - s_i })\end{eqnarray}$

与传统形函数不同的是: 单元运动过程中, 参数$\xi $是不变的, $L$却是可变的, 其变化率

(20)$\begin{eqnarray}\dot{L} = n^{ - 1}\left( {\dot{s}_n - \dot{s}_0 } \right) \buildrel \Delta \over = {{ T}}_L {{\dot{ q}}}_r \end{eqnarray}$

由式(13)可知

(21)$\begin{eqnarray}\dot{s}_i = n^{ - 1}\left( {n - i} \right)\dot{s}_0 + n^{ - 1}i\dot{s}_n\end{eqnarray}$

两节点间绳索上的点, 其弧长坐标也是变化的, 由式(19)可知: 其变化率为

(22)$\begin{eqnarray}\dot{s} = \dot{L}\xi + \dot{s}_i \buildrel \Delta \over = {{ T}}_s{{\dot{ q}}}_r\end{eqnarray}$

虽然式(14)中出现了节点矢径对弧长坐标的导数, 但内部节点矢径对弧长坐标的导数${{ {r}'}}_k $($k \ne 0,k \ne n$)并不是单元的节点参数, 它们是通过一、二阶导数在节点处的连续性方程

(23)$\begin{eqnarray}[ {{ {r}'}} ]{{ A}} = a[ {{ r}}]{{ B}} + [ {{{ {r}'}}_0 } \ \ {{ {r}'}}_n]{{ C}}\end{eqnarray}$

求得(参见三次样条插值理论). 式中, $a = L^{ - 1}$; 列向量为节点矢径对弧长坐标一阶导数的矩阵$[ {{ {r}'}} ] =[ {{\begin{array} {{{ {r}'}}_0 } \ \ {{{ {r}'}}_1 } \ \ \cdots \ \ {{{ {r}'}}_n } \\ \end{array} }} ]$; 列向量为节点矢径的矩阵$[r]=[r_{0} r_{1} \cdots r_{n}]$; 系数矩阵$ A$, $ B$都是$( {n + 1} )\times ( {n + 1} )$维三对角矩阵. $ A$的第一列中, 第一个元素为1其余为0, 最后一列中, 最后一个元素为1其余为0, 除此之外的其它列中, 对角线附近的3个元素皆为$[{2;8;2} ]$; $ B$的第一和最后一列元素皆为0, 除此之外的其他列中, 对角线附近的3个元素皆为$[{ - 6;0;6} ]$. 系数矩阵$ C$为$2\times ( {n+1} )$维常数矩阵, 它只有两个非零元素$C_{1,1} = C_{2,n + 1} = 1$. 从式(23)中可得

(24)$\begin{eqnarray}&&[ {{\dot{ {r}'}}} ] = ( {a[ {{\dot{ r}}} ] + \dot{a}[ {{ r}} ]} ){{ B}}_a+ [ {{\begin{array}{*{20}c} {{{\dot{ {r}'}}}_0 } \ \ {{{\dot{ {r}'}}}_n } {{ C}}_a \end{array} }} ]\end{eqnarray}$

(25)$\begin{eqnarray}&&[ {{\ddot{ {r}'}}} ] = ( {a[ {{\ddot{ r}}} ] + 2\dot{a}[ {{\dot{ r}}} ] + \ddot{a}[ {{ r}} ]} ){{ B}}_a + [{{ {{{\ddot{ {r}'}}}_0 } \ \ {{{\ddot{ {r}'}}}_n } \\ }} ]{{ C}}_a\end{eqnarray}$

式中, ${{ B}}_a = {{ B A}}^{ - 1}$, ${{ C}}_a = {{ C A}}^{ - 1}$. 这两个矩阵的$i$行$j$列元素分别记为$b_{i,j} $和$c_{i,j} $. 在此基础上, 可以将式(24)改写为

(26)$\begin{eqnarray}{{\dot{ {r}'}}}_i = ( {[ {{\begin{array}{*{20}c} {a{{ Y}}_i } \ \ {{{ \varGamma }}_i } \ \ { 0_{3\times2} } \\\end{array} }} ] - a^2[ {{ r}} ]{{ B}}_a {{ T}}_L } ){{\dot{ q}}}_r \buildrel \Delta \over = {{ \varPhi }}_i {{\dot{ q}}}_r\end{eqnarray}$

其中, ${{ Y}}_i $和${{ \varGamma }}_i $可用$b_{i,j} $和$c_{i,j} $分别表示为

(27)${{ Y}}_i = [ {{{b_{1,i} {{ E}}} \ \ {b_{2,i} {{ E}}} \ \ \cdots\ \ {b_{n + 1,i} {{ E}}} }} ]$

(28)${{\varGamma}}_i = [ {c_{1,i} {{ E}}} {c_{2,i} {{ E}}}] $

$ E$为$3\times 3$维单位矩阵. 式(25)可以改写为

(29)$\begin{eqnarray}{{\ddot{ {r}'}}}_i = {{ \varPhi }}_i {{\ddot{ q}}}_r +{{ \gamma }}_i\end{eqnarray}$

其中, 加速度余量

(30)$\begin{eqnarray}{{ \gamma }}_i = - 2a^2\dot{L}\sum_{k = 0}^n {b_{k + 1,i}{{\dot{ r}}}_k } + 2a^3\dot{L}^2\sum_{k = 0}^n {b_{k + 1,i}{{ r}}_k }\end{eqnarray}$

对式(14)求弧长坐标导数可得

(31)$\begin{eqnarray}&&{{ {r}'}} = a( {{N}'_1 {{ r}}_i + {N}'_2 {{ r}}_{i + 1} } ) + {N}'_3 {{ {r}'}}_i + {N}'_4 {{ {r}'}}_{i + 1}\\\end{eqnarray}$

(32)$\begin{eqnarray}&&{{ {r}''}} = a^2({N}''_1 \,{{ r}}_i + {N}''_2 \,{{ r}}_{i + 1} ) + a( {{N}''_3 \,{{ {r}'}}_i + {N}''_4 \,{{ {r}'}}_{i + 1} } )\\\end{eqnarray}$

(33)$\begin{eqnarray}&&{{ {r}'''}} = a^3( {{N}'''_1 \,{{ r}}_i + {N}'''_2 \,{{ r}}_{i + 1} } ) + a^2({N}'''_3 \,{{ {r}'}}_i + {N}'''_4\,{{ {r}'}}_{i + 1} )\qquad\end{eqnarray}$

式中, $N_i $对$\xi $的1$\sim$3阶导数分别为: ${N}'_i \buildrel \Delta \over = {\partial N_i }/{\partial \xi }$, ${N}''_i \buildrel \Delta \over = {\partial ^2N_i }/{\partial \xi }^2$, ${N}'''_i \buildrel \Delta \over = {\partial ^3N_i }/{\partial \xi }^3$. 对式(14)和式(31)求时间导数可得

(34)$\begin{eqnarray} {{\dot{ {r}}}} = {{\bar{ T}}}_{r} {{\dot{ q}}}_r,\ \ {{\ddot{ {r}}}} = {{\bar{ T}}}_{r} {{\ddot{ q}}}_r+ {{\bar{ \alpha }}}_{r} \end{eqnarray}$

(35)$\begin{eqnarray} {{\dot{ {r}'}}} = {{\bar{ T}}}_{r}' {{\dot{ q}}}_r,\ \ {{\ddot{ {r}'}}} = {{\bar{ T}}}_{r}' {{\ddot{ q}}}_r+ {{\bar{ \alpha }}}_{r}' \end{eqnarray}$

其中, 转换矩阵

(36)$\begin{eqnarray}&&{\bar{ T}}_r = N_1 {{ G}}_i + N_2 {{ G}}_{i + 1} + L(N_3{{ \varPhi }}_i + N_4 {{ \varPhi }}_{i + 1} )+\\&&\qquad(N_3 {{ {r}'}}_i + N_4 {{ {r}'}}_{i + 1} ){{ T}}_L \end{eqnarray}$

(37)$\begin{eqnarray}&&{\bar{ T}}_{r}' = a( {{N}'_1 {{ G}}_i + {N}'_2 {{ G}}_{i + 1} } ) + {N}'_3 {{ \varPhi }}_i + {N}'_4 {{ \varPhi}}_{i + 1} -\\&&\qquad a^2( {{N}'_1 {{ r}}_i + {N}'_2 {{ r}}_{i + 1} } ){{ T}}_L\end{eqnarray}$

上式中矩阵${{ G}}_i $中的元素除了$1$至$3$行和$3(i - 1) + 1$至$3(i - 1) + 3$列的元素为单位矩阵外, 其余元素皆为0. 式中的加速度余量分别为

(38)$\begin{eqnarray}&&{\bar{ \alpha }}_r = L(N_3 {{ \gamma }}_i + N_4 {{ \gamma}}_{i + 1} ) + 2\dot{L}(N_3 {{\dot{ {r}'}}}_i + N_4 {{\dot{ {r}'}}}_{i + 1} ) \end{eqnarray}$

(39)$\begin{eqnarray}&&{\bar{ \alpha }}_{r}' = {N}'_3 {{ \gamma }}_i + {N}'_4 {{ \gamma }}_{i + 1} \mbox{ + 2}\dot{a}( {{N}'_1 {{\dot{ r}}}_i +{N}'_2 {{\dot{ r}}}_{i + 1} } ) +\\&&\qquad 2a^3\dot{L}^2({{N}'_1 {{ r}}_i + {N}'_2 {{ r}}_{i + 1} } )\end{eqnarray}$

式(14)描述的是空间点的运动, 其时间变化率式(34)和式(35)是空间速度和空间加速度(文中用变量上方加``.''表示), 为建立系统动力学方程, 需要求解相应的物质速度和物质加速度. 根据式(1)和式(2), $r$的物质速度和加速度分别为

(40)$\begin{eqnarray}&&{\partial {{ r}}}/{\partial t} = {{\dot{ r}}} - {{ {r}'}}\dot{s} \buildrel \Delta \over = {{ T}}_r {{\dot{ q}}}_r\end{eqnarray}$

(41)$\begin{eqnarray}&&{\partial ^2{{ r}}}/{\partial t^2} ={{\ddot{ r}}} - {{ {r}'}}\ddot{s} - 2{{\dot{ {r}'}}}\dot{s} + {{ {r}''}}\dot{s}^2 = {{ T}}_r {{\ddot{ q}}}_r + {{ a}}_r\end{eqnarray}$

其中, 转换矩阵

(42)$\begin{eqnarray}{{ T}}_r = {{\bar{ T}}}_r - {{ {r}'T}}_s\end{eqnarray}$

加速度余量

(43)$\begin{eqnarray}{{ a}}_r = {{\bar{ a}}}_r + {{ {r}''}}\dot{s}^2 -2{{\dot{ {r}'}}}\dot{s}\end{eqnarray}$

由式(3)可知: 惯性力虚功率

(44)$\begin{eqnarray}\delta p_m = \int_0^L {\delta ( {{\partial {{ r}}}/{\partial t}} )^{\rm T}{\partial ^2{{ r}}}/{\partial t^2}\rho {\rm d}s} = \delta {{\dot{ q}}}^{\rm T}_r ( {{{ M}}_r {{\ddot{ q}}}_r + {{ f}}_r } )\quad \end{eqnarray}$

其中, 质量矩阵

(45)$\begin{eqnarray}{{ M}}_r = \rho L\int_0^1 {{{ T}}_r^{\rm T} {{ T}}_r{\rm d}\xi }\end{eqnarray}$

广义力列阵

(46)$\begin{eqnarray}{{ f}}_r = \rho L\int_0^1 {{{ T}}_r^{\rm T} {{ a}}_r{\rm d}\xi }\end{eqnarray}$

根据式(4)和式(31)可以求得应变$\varepsilon $, 它的一、二阶变化率分别为

(47)$\begin{eqnarray}\dot{\varepsilon } = {{\bar{ T}}}_\varepsilon {{\dot{ q}}}_r,\ddot{\varepsilon } = {{\bar{ T}}}_\varepsilon {{\ddot{ q}}}_r + \bar{\alpha }_\varepsilon \end{eqnarray}$

其中, 转换矩阵

(48)$\begin{eqnarray}{{\bar{ T}}}_\varepsilon = ( {1 + \varepsilon } )^{ -1}( {{ {r}'}} )^{\rm T}{{\bar{ T}}}_{r}'\end{eqnarray}$

加速度余量

(49)$\begin{eqnarray}\bar{\alpha }_\varepsilon = ( {1 + \varepsilon } )^{ - 1}\lt[{( {{\dot{ {r}'}}} )^{\rm T}{{\dot{ {r}'}}} + ({{ {r}'}} )^{\rm T}{{\bar{ \alpha }}}_{r}' - \dot{\varepsilon }^2} ]\end{eqnarray}$

应变对弧长坐标的导数

(50)$\begin{eqnarray}&&{\varepsilon }' = ( {1 + \varepsilon } )^{ - 1}( {{ {r}'}} )^{\rm T}{{ {r}''}}\end{eqnarray}$

(51)$\begin{eqnarray}&&{\varepsilon }'' = ( {1 + \varepsilon } )^{ - 1}[ {({{ {r}''}} )^{\rm T}{{ {r}''}} + ( {{ {r}'}})^{\rm T}{{ {r}'''}} - ( {\varepsilon }' )^2}]\end{eqnarray}$

对式(50)求时间导数可得

(52)$\begin{eqnarray}\dot{{\varepsilon }'} = ( {1 + \varepsilon } )^{ - 1}[{( {{\dot{ {r}'}}} )^{\rm T}{{ {r}''}} + ( {{ {r}'}} )^{\rm T}{{\dot{ {r}''}}} - \dot{\varepsilon}{\varepsilon }'}]\end{eqnarray}$

应变的物质速度和加速度分别为

(53)$\begin{eqnarray}&&{\partial \varepsilon }/{\partial t} = {{ T}}_\varepsilon {{\dot{ q}}}_r \end{eqnarray}$

(54)$\begin{eqnarray} &&{\partial ^2\varepsilon }/{\partial t^2} = {{ T}}_\varepsilon {{\ddot{ q}}}_r + \alpha_\varepsilon\end{eqnarray}$

其中, 转换矩阵

(55)$\begin{eqnarray}{{ T}}_\varepsilon = {{\bar{ T}}}_\varepsilon - {\varepsilon}'{{ T}}_s\end{eqnarray}$

加速度余量

(56)$\begin{eqnarray}\alpha _\varepsilon = \bar{\alpha }_\varepsilon + {\varepsilon }''\dot{s}^2 - 2\dot{{\varepsilon }'}\dot{s}\end{eqnarray}$

根据式(3), 变形虚功率

(57)$\begin{eqnarray}\delta p_\varepsilon = \int_0^L {\delta ( {{\partial \varepsilon}/{\partial t}} )^{\rm T}\bar{\sigma }{\rm d}s} = \delta{{\dot{ q}}}^{\rm T}_r ( {{{ M}}_\varepsilon {{\ddot{ q}}}_r + {{ f}}_\varepsilon } )\end{eqnarray}$

其中, 质量矩阵

(58)$\begin{eqnarray}{{ M}}_\varepsilon = \frac16h^2E_A L\int_0^1{{{ T}}_\varepsilon ^{\rm T} {{ T}}_\varepsilon {\rm d}\xi }\end{eqnarray}$

广义力列阵

(59)$\begin{eqnarray}{{ f}}_\varepsilon = E_A L\int_0^1 {{{ T}}_\varepsilon^{\rm T} \lt( {\varepsilon + \frac12h{\partial \varepsilon }/{\partial t} + \frac16h^2\alpha_\varepsilon } ){\rm d}\xi }\end{eqnarray}$

4 滑轮单元

滑轮相对基矢量为$( {{{ g}}_1,{{ g}}_2,{{ g}}_3 })$的总体参照系转动, 可用3个卡尔丹角描述: $\beta _1 $为绕${{ g}}_1 $的转角, $\beta _2 $为绕${{\bar{ e}}}_y $的转角, $\beta _3 $为绕滑轮转轴${{ e}}_z $的转角. 滑轮运动参数组成的列向量${{ q}}_p = [ {{{ p}}_0;\beta _1 ;\beta _2 ;\beta _3 } ]$.

滑轮参照系基矢量可用卡尔丹角描述为

(60)$\begin{eqnarray}&&{{\bar{ e}}}_x = \cos \beta _2 {{ g}}_1 + \sin \beta _1 \sin \beta _2 {{ g}}_2 - \cos \beta _1 \sin \beta _2 {{ g}}_3\quad\end{eqnarray}$

(61)$\begin{eqnarray}&&{{\bar{ e}}}_y = \cos \beta _1 {{ g}}_2 + \sin \beta _1 {{ g}}_3\end{eqnarray}$

(62)$\begin{eqnarray}&&{{\bar{ e}}}_z = \sin \beta _2 {{ g}}_1 - \sin \beta _1 \cos \beta _2 {{ g}}_2 + \cos \beta _1 \cos \beta _2 {{ g}}_3\end{eqnarray}$

如图3所示, 滑轮连体基的3个基矢量分别为

(63)$\begin{eqnarray}&&{{ e}}_x = \cos \beta _3 {{\bar{ e}}}_x + \sin\beta _3{{\bar{ e}}}_y\end{eqnarray}$

(64)$\begin{eqnarray}&&{{ e}}_y = \cos \beta _3 {{\bar{ e}}}_y - \sin \beta _3 {{\bar{ e}}}_x\end{eqnarray}$

(65)$\begin{eqnarray}&&{{ e}}_z = {{\bar{ e}}}_z\end{eqnarray}$

图3

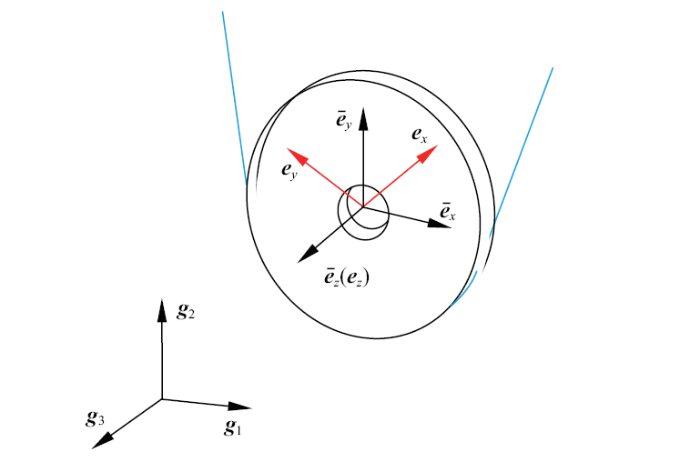新窗口打开|下载原图ZIP|生成PPT
图3滑轮参照系与滑轮连体基滑轮参照系的角速度和角加速度分别为

Fig.3The reference and body-fixed coordinate system of a pulley

(66)$\begin{eqnarray}&&{{\bar{ \omega }}}_p = \dot{\beta }_1 {{ g}}_1 + \dot{\beta}_2 {{\bar{ e}}}_y \end{eqnarray}$

(67)$\begin{eqnarray}&&{{\dot{\bar{ \omega }}}}_p = \ddot{\beta }_1 {{ g}}_1 +\ddot{\beta }_2 {{\bar{ e}}}_y +\dot{\beta }_2 \dot{\beta }_1 {{ g}}_1 \times {{\bar{ e}}}_y\end{eqnarray}$

滑轮的角速度和角加速度分别为

(68)$\begin{eqnarray}&&{{ \omega }}_p = {{\bar{ \omega }}}_p + \dot{\beta }_3 {{ e}}_z \buildrel \Delta \over = {{ T}}_\omega {{\dot{ q}}}_p\end{eqnarray}$

(69)$\begin{eqnarray}&&{{\dot{ \omega }}}_p = {{\dot{\bar{ \omega }}}}_p + \ddot{\beta }_3 {{ e}}_z + \dot{\beta }_3 {{\bar{ \omega }}}_p\times {{ e}}_z \buildrel \Delta \over = {{ T}}_\omega {{\ddot{ q}}}_p + {{ \alpha }}_\omega \end{eqnarray}$

其中, 转换矩阵

(70)$\begin{eqnarray}&&{{ T}}_\omega = [ {{\begin{array}{*{20}c} { 0_{3\times 3} } \ \ {{{ g}}_1 } \ \ {{{\bar{ e}}}_y } \ \ {{{ e}}_z } \\\end{array} }} ]\end{eqnarray}$

角加速度余量

(71)$\begin{eqnarray}{{ \alpha }}_\omega = \dot{\beta }_2 \dot{\beta }_1 {{ g}}_1\times {{\bar{ e}}}_y + \dot{\beta }_3 {{\bar{ \omega }}}_p\times {{ e}}_z\end{eqnarray}$

滑轮转动惯量矩阵

(72)$\begin{eqnarray}{{ J}}_p = J_p {{ e}}_x {{ e}}_x^{\rm T} + J_p {{ e}}_y {{ e}}_y^{\rm T} + 2J_p {{ e}}_z {{ e}}_z^{\rm T}\end{eqnarray}$

其中, $J_p $为滑轮绕中截面直径的转动惯量. 滑轮对系统惯性力虚功率的贡献为

(73)$\begin{eqnarray}\delta w_p = \delta {{\dot{ q}}}^{\rm T}_p ( {{{ M}}_p {{\ddot{ q}}}_p + {{ f}}_p } )\end{eqnarray}$

其中, 质量矩阵

(74)$\begin{eqnarray}{{ M}}_p = {{ T}}_\omega ^{\rm T} {{ J}}_p {{ T}}_\omega +\lt[ {{\begin{array}{*{20}c} {m{{ E}}_{3\times 3} } \ \ { 0_{3\times 3} } \\ { 0_{3\times 3} } \ \ { 0_{3\times 3} } \\\end{array} }} ]\end{eqnarray}$

广义力列阵

(75)$\begin{eqnarray}{{ f}}_p = {{ T}}_\omega ^{\rm T} ( {{{ J}}_p {{ \alpha }}_\omega + {{ \tilde {\omega }}}_p {{ J}}_p {{ \omega }}_p } )\end{eqnarray}$

5 滑轮内绳索单元

如图4所示, 在半径为$r$的滑轮中心面内建立滑轮参照系, 它随滑轮一起摆动, 但不随滑轮一起绕法向转动. 其原点位于滑轮中截面圆心${{ p}}_0 $, 基矢量${{\bar{ e}}}_z $指向滑轮中心面的法向, 基矢量${{\bar{ e}}}_x $和${{\bar{ e}}}_y $在滑轮的中心面内.

图4

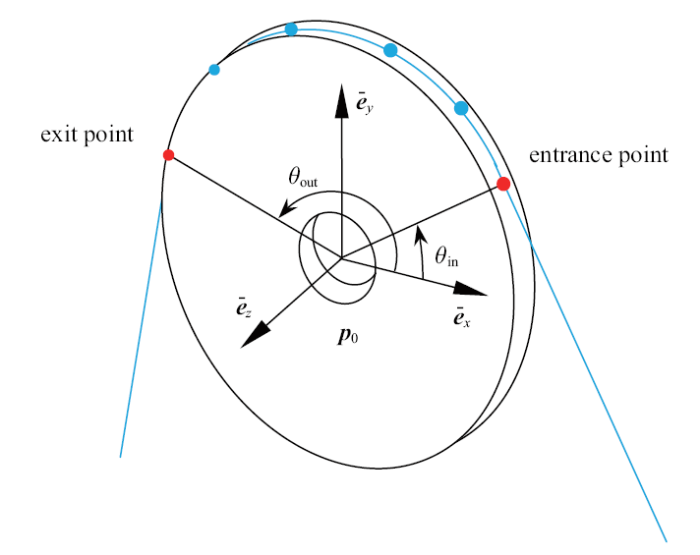新窗口打开|下载原图ZIP|生成PPT
图4滑轮内绳索单元

Fig.4A rope element within a pulley

由于绳索抗弯模量很低, 与滑轮相接触的一段绳索被整形为一段圆弧, 弧长坐标分别为$s_{\rm in}$和$s_{\rm out} $, 其入绳点和出绳点对应的方位角分别为$\theta _{\rm in} $和$\theta _{\rm out} $(分别为$\bar{ e}_x $轴逆时针转到入绳点和出绳点的角度, 并且$s_{\rm out} > s_{\rm in} $), 包角$\varphi \buildrel \Delta \over = \theta _{\rm out} - \theta _{\rm in} $与滑轮接触段绳索上的弧长坐标是方位角$\theta $和时间的函数. 方位角为$\theta \in ( {\theta _{\rm in},\theta _{\rm out} })$一点的绳索矢径

(76)$\begin{eqnarray}{{ r}}_\theta = {{ p}}_0 + r( {\cos \theta {{\bar{ e}}}_x + \sin \theta {{\bar{ e}}}_y } ) \buildrel \Delta\over = {{ p}}_0 + r{{ e}}_n\end{eqnarray}$

该点的空间速度和物质速度分别为

(77)$\begin{eqnarray}&&{{\dot{ r}}}_\theta = {{\dot{ p}}}_0 + r{{\bar{ \omega }}}_p \times {{ e}}_n\end{eqnarray}$

(78)$\begin{eqnarray}&&{\partial {{ r}}_\theta }/{\partial t} = {{\dot{ r}}} - (1 + \varepsilon )( {\cos \theta{{\bar{ e}}}_y - \sin \theta {{\bar{ e}}}_x }){\partial s}/{\partial t}\end{eqnarray}$

其中, ${{\bar{ \omega }}}_p $为滑轮参照系的角速度, 绳索在$\theta $处的应变为

(79)$\begin{eqnarray}\varepsilon _\theta = r( {s}' )^{ - 1} - 1\end{eqnarray}$

其中, ${s}' \buildrel \Delta \over = {\partial s}/{\partial \theta }$. 理想状态下, 进入滑轮中的绳索与滑轮无相对滑动. 绳索的物质速度与滑轮上相应点的物质速度相同. 由此可得

(80)$\begin{eqnarray}&&{\partial s}/{\partial t} = - {s}'\dot{\beta }_3\end{eqnarray}$

(81)$\begin{eqnarray}&&{\partial {s}'}/{\partial t} = - {s}''\dot{\beta }_3\end{eqnarray}$

其中, ${s}'' \buildrel \Delta \over = {\partial ^2s}/{\partial \theta ^2}$, $\dot{\beta }_3 $为滑轮自转角速度. 对式(79)分别求时间导数和弧长导数可得

(82)$\begin{eqnarray}&&\dot{\varepsilon }_\theta = r( {s}' )^{ - 2}{s}''\dot{\beta }_3\end{eqnarray}$

(83)$\begin{eqnarray}&&&{\varepsilon }'_\theta = - r( {s}' )^{ - 3}{s}''\end{eqnarray}$

方位角为$\theta $处的绳索应变物质导数

(84)$\begin{eqnarray}{\partial \varepsilon _\theta }/{\partial t = \dot{\varepsilon }_\theta -}{\varepsilon }'_\theta ( {{\partial s}/{\partial t}} ) = 0\end{eqnarray}$

上式说明: 滑轮内部的绳索其变形虚功率为零. 对式(77)求时间导数可得

(85)$\begin{eqnarray}{{\ddot{ r}}}_\theta = {{\ddot{ p}}}_0 + r{{\dot{\bar{ \omega }}}}_p \times {{ e}}_n +r{{\bar{ \omega }}}_p \times ( {{{\bar{ \omega }}}_p \times {{ e}}_n } )\end{eqnarray}$

矢径对弧长的导数

(86)$\begin{eqnarray}&&{{ {r}'}}_\theta = r( {s}' )^{ - 1}{{ e}}_z \times{{ e}}_n\end{eqnarray}$

(87)$\begin{eqnarray}&&{{ {r}''}}_\theta = - r( {s}' )^{ - 2}{{ e}}_n -r( {s}' )^{ - 3}{s}''{{ e}}_z \times {{ e}}_n\end{eqnarray}$

式中利用了关系式$\varepsilon _\theta = r{\theta }' - 1$. 根据式(2)可得该点的物质加速度

(88)$\begin{eqnarray}{\partial ^2{{ r}}_\theta }/{\partial t^2} = {{\ddot{ r}}}_\theta - 2{{\dot{ {r}'}}}_\theta \dot{s} + {{ {r}''}}_\theta {\kern 1pt} \dot{s}^2 -{{ {r}'}}_\theta {\kern 1pt} \ddot{s}\end{eqnarray}$

其中

(89)$\begin{eqnarray}&&{{\dot{ {r}'}}}_\theta = r( {s}' )^{ - 1}{{\bar{ \omega }}}_p \times ( {{{ e}}_z \times {{ e}}_n })+r( {s}' )^{ - 2}{s}''\dot{\beta }_3 {{ e}}_z \times {{ e}}_n\end{eqnarray}$

(90)$\begin{eqnarray}&&\ddot{s} = {s}''\dot{\beta }_3^2 - {s}'\ddot{\beta }_3\end{eqnarray}$

将式(89)和式(90)代入式(88)中可得

(91)$\begin{eqnarray}{\partial ^2{{ r}}_\theta }/{\partial t^2} = {{\ddot{ p}}}_0 + r{{\dot{ \omega }}}_p\times {{ e}}_n +r{{ \omega }}_p \times ( {{{ \omega }}_p \times {{ e}}_n } )\end{eqnarray}$

利用${{ q}}_p $可将物质速度写作

(92)$\begin{eqnarray}{\partial {{ r}}_\theta }/{\partial t} = [ {{{ E}}_{3\times 3}, - r{{ e}}_n \times[ {{{ g}}_1,{{\bar{ e}}}_y,{{ e}}_z } ]}]{{\dot{ q}}}_p \buildrel \Delta \over = {{ \varGamma }}_r{{\dot{ q}}}_p\end{eqnarray}$

惯性力虚功率

(93)$\begin{eqnarray}\delta p_m = \delta {{\dot{ q}}}_p^{\rm T} ( {{{ M}}_m {{\ddot{ q}}}_p + {{ f}}_m } )\end{eqnarray}$

其中, 质量矩阵

(94)$\begin{eqnarray}{{ M}}_m = \varphi \int_0^1 {{{ \varGamma }}_r^{\rm T} {{ \varGamma }}_r \rho {s}'{\rm d}\xi }\end{eqnarray}$

广义力列阵

(95)$\begin{eqnarray}{{ f}}_m = \varphi \int_0^1 {{{ \varGamma }}_r^{\rm T} {{\dot{ \varGamma }}}_r {{\dot{ q}}}_p \rho {s}'{\rm d}\xi }\end{eqnarray}$

弧长坐标$s$与$\theta $之间的关系可由插值函数

(96)$\begin{eqnarray}&&s = N_1 s_{\rm in} + N_2 s_{\rm out} + r\varphi [N_3 (1 + \varepsilon _{\rm in} )^{ - 1} +\\&&\qquad N_3 (1 + \varepsilon _{\rm out} )^{ - 1}]\end{eqnarray}$

近似, 它对$\theta $的导数

(97)$\begin{eqnarray}&&{s}' = \varphi ^{-1}({N}'_1 s_{\rm in} + {N}'_2 s_{\rm out} ) + r[{N}'_3 (1 + \varepsilon _{\rm in} )^{ - 1} +\\&&\qquad {N}'_4 (1 + \varepsilon _{\rm out} )^{ - 1}]\end{eqnarray}$

其中的形函数与式(15)$\sim\!$式(18)形式相同, 只需将自变量改变为$\xi = \varphi ^{ - 1}( {\theta - \theta_{\rm in} } )$.

6 滑轮绳索间边界条件

入绳点绳索矢径

(98)$\begin{eqnarray}{{ r}}_{\rm in} = {{ p}}_0 + r{{ n}}_{\rm in}\end{eqnarray}$

入绳点的径向矢量和切线矢量分别为

(99)$\begin{eqnarray}&&{{ n}}_{\rm in} = \cos \theta _{\rm in} {{\bar{ e}}}_x + \sin \theta_{\rm in} {{\bar{ e}}}_y\end{eqnarray}$

(100)$\begin{eqnarray}&&{{ t}}_{\rm in} = \cos \theta _{\rm in} {{\bar{ e}}}_y - \sin \theta_{\rm in} {{\bar{ e}}}_x\end{eqnarray}$

入绳点处绳索矢径对弧长坐标的导数

(101)$\begin{eqnarray}{{ {r}'}}_{\rm in} = ( {1 + \varepsilon _{\rm in} } )( {\cos\phi _{\rm in} {{ t}}_{\rm in} + \sin \phi _{\rm in} {{\bar{ e}}}_z } )\end{eqnarray}$

其中, $\phi _{\rm in} $为${{ {r}'}}_{\rm in} $与${{ t}}_{\rm in} $间的夹角. 入绳点处绳索的物质速度

(102)$\begin{eqnarray}{\partial {{ r}}_{\rm in} }/{\partial t = }{{\dot{ p}}}_0 + r{{\bar{ \omega }}}_p \times{{ n}}_{\rm in} + r\dot{\theta }_{\rm in} {{ t}}_{\rm in} - {{ {r}'}}_{\rm in} \dot{s}_{\rm in}\end{eqnarray}$

出绳点处相应矢量可依同理给出. 理想状态下, 滑轮与绳索沿滑轮切向没有相对滑动, 由此可得

(103)$\begin{eqnarray}&&r( {\dot{\theta }_{\rm in} - \dot{\beta }_3 } ) = \cos \phi_{\rm in} ( {1 + \varepsilon _{\rm in} } )\dot{s}_{\rm in}\end{eqnarray}$

(104)$\begin{eqnarray}&&r( {\dot{\theta }_{\rm out} - \dot{\beta }_3 } ) = \cos \phi_{\rm out} ( {1 + \varepsilon _{\rm out} } )\dot{s}_{\rm out}\end{eqnarray}$

7 卷筒绳索间边界条件

如图5所示, 在卷筒端部建立不随卷筒转动的参照系, 其原点为${{ w}}_0$, 基矢量${{ g}}_x $和${{ g}}_y $位于卷筒横截面内, ${{ g}}_z $沿卷筒轴向. 记卷筒半径为$\bar{r}$, 角速度为$\omega _w$. 卷筒上入绳点处绳索的矢径

(105)$\begin{eqnarray}{{ r}}_{\rm in} = {{ w}}_0 + z_{\rm in} {{ g}}_z + \bar{r}{{ n}}_{\rm in}\end{eqnarray}$

其中

(106)$\begin{eqnarray}{{ n}}_{\rm in} = \cos \theta _{\rm in} {{ g}}_x + \sin \theta _{\rm in}{{ g}}_y\end{eqnarray}$

该点矢径对弧长的导数

(107)$\begin{eqnarray}{{ {r}'}}_{\rm in} = ( {1 + \varepsilon _{\rm in} } )( {\cos \phi _{\rm in} {{ g}}_z \times {{ n}}_{\rm in} + \sin \phi _{\rm in}{{ g}}_z } )\end{eqnarray}$

其中, $\phi _{\rm in} $为${{ {r}'}}_{\rm in} $与${{ g}}_z \times{{ n}}_{\rm in} $间的夹角.

图5

新窗口打开|下载原图ZIP|生成PPT
图5卷筒上的入绳点

Fig.5The entrance point on a winch

7.1 卷筒上无刻槽时

理想状态下, 绳索与卷筒表面无相对滑动, 绳索和卷筒在接触点处的物质速度相同, 此时

(108)$\begin{eqnarray}{\partial {{ r}}_{\rm in} }/ {\partial t} = {{\dot{ r}}}_{\rm in} - \dot{s}_{\rm in} {{ {r}'}}_{\rm in} =\omega _w \bar{r}{{ g}}_z \times {{ n}}_{\rm in}\end{eqnarray}$

由此可得

(109)$\begin{eqnarray}&&\bar{r}( {\dot{\theta }_{\rm in} - \omega _w } ) = \dot{s}_{\rm in}( {1 + \varepsilon _{\rm in} } )\cos \phi _{\rm in}\end{eqnarray}$

(110)$\begin{eqnarray}&&\dot{z}_{\rm in} = \dot{s}_{\rm in} ( {1 + \varepsilon _{\rm in} } )\sin\phi _{\rm in}\end{eqnarray}$

7.2 卷筒有刻槽时

刻槽螺距为$d$(正螺旋时$d > 0$, 反螺旋时$d < 0$). 刻槽上一点由螺旋角$\gamma_{\rm in} $描述. 入绳点的位置和速度可用$\gamma _{\rm in} $和$\dot{\gamma }_{\rm in}$表示为

(111)$\begin{eqnarray}&&{{ r}}_{\rm in} = \bar{r}\cos ( {\gamma _{\rm in} + \vartheta }){{ g}}_x + \bar{r}\sin ( {\gamma _{\rm in} + \vartheta }){{ g}}_y +\\&&\qquad \lambda _z \gamma _{\rm in} {{ g}}_z\end{eqnarray}$

(112)$\begin{eqnarray}&&{{\dot{ r}}}_{\rm in} = {{ g}}_z \times ( {{{ r}}_{\rm in} - \lambda _z \gamma _{\rm in} {{ g}}_z } )( {\dot{\gamma }_{\rm in} + \omega _w } ) + \lambda _z \dot{\gamma }_{\rm in} {{ g}}_z\quad\end{eqnarray}$

其中, $\lambda _z = d\pi ^{ - 1}/2$; $\omega _w$为卷筒的自转角速度; $\vartheta $为卷筒在$t$时刻转过的角度, 即

(113)$\begin{eqnarray}\vartheta = \int_0^t {\omega _w {\rm d}t}\end{eqnarray}$

然而, 入绳点的位置也可用$z_{\rm in} $和$\theta _{\rm in}$表示(参见式(105)). 由此可得

(114)$\begin{eqnarray}&&z_{\rm in} = \lambda _z \gamma _{\rm in}\end{eqnarray}$

(115)$\begin{eqnarray}&&\dot{\theta }_{\rm in} = \omega _w + \dot{\gamma }_{\rm in}\end{eqnarray}$

入绳点处刻槽的切向

(116)$\begin{eqnarray}{{\bar{ t}}}_{{\rm in}} = \lambda _w^{ - 1} ( {\bar{r}\cos\theta _{\rm in} {{ g}}_y - \bar{r}\sin \theta _{\rm in} {{ g}}_x +\lambda _z {{ g}}_z } )\end{eqnarray}$

其中, $\lambda _w = ( {\bar{r}^2 + \lambda _{{\kern 1pt} z}^2 })^{1 / 2}$. 理想状态下, 绳索与卷筒沿刻槽无相对滑动, 由此可得

(117)$\begin{eqnarray}\lambda _w^2 \,\dot{\gamma }_{\rm in} =(1+ \varepsilon _{\rm in} )\dot{s}_{\rm in}( {\bar{r}\cos \varphi _{\rm in} + \lambda _z \sin \phi _{\rm in} })\end{eqnarray}$

8 算例

算例1: 如图1所示的系统中, 假设绳索不可伸长. 滑轮两侧绳索均沿竖直方向; 左侧绳索顶点以速度$v$收绳.

左侧绳索顶点的空间速度为零, 按方程(1)该点的物质速度方向为竖直向上, 大小为

$$\begin{eqnarray*}v_T = v\end{eqnarray*}$$

由于绳索不可伸长, 出绳点处物质速度

$$\begin{eqnarray*}v_{\rm out} = v_T = v\end{eqnarray*}$$

入绳点处的物质速度

$$\begin{eqnarray*}v_{{\rm in}} = 0\end{eqnarray*}$$

理想状态下, 滑轮与绳索间无相对滑动, 滑轮上与入绳点和出绳点对应的直径左右端点的物质速度分别为$v_{\rm out}$ 和$v_{\rm in} $. 说明滑轮以入绳点为瞬心转动, 从而滑轮的速度

$$\begin{eqnarray*}v_p = \frac12v\end{eqnarray*}$$

滑轮的角速度

$$\begin{eqnarray*}\omega =\frac{v}{2r}\end{eqnarray*}$$

这些结果与用其他理论所得结果相符.

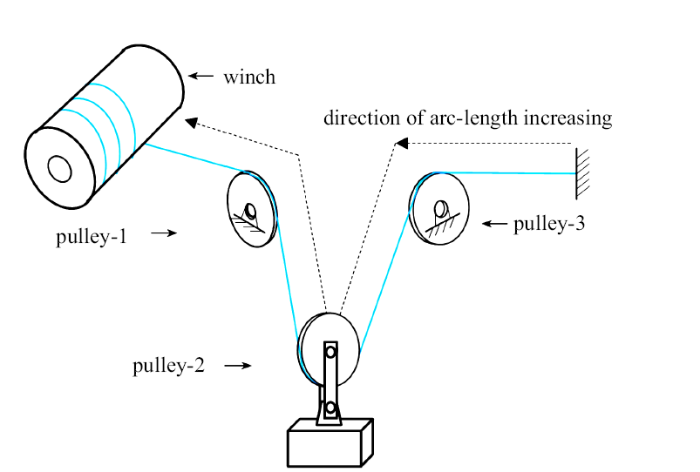
算例2: 如图6所示的滑轮绳索系统由1个卷筒、2个定滑轮和1个动滑轮组成. 总体坐标系的原点位于最右端固定点处, $x$轴沿水平方向, $y$轴沿竖直向上方向.

图6

新窗口打开|下载原图ZIP|生成PPT
图6空间滑轮绳索系统

Fig.6A spatial rope-pulley system

卷筒半径为63 cm, 其表面上有螺距为34 mm的正螺旋刻槽. 卷筒首先用1 s时间使其角速度从零加速到$\pi$/3, 再以此角速度匀速转动2 s后, 用1 s时间将角速度降至为零. 在加减速段与匀速段的过渡时刻, 卷筒的角加速度均为零. 加减速段内的角速度可依据上述条件用时间的多项式拟合. 3个滑轮的半径均为27 cm, 质量均为35 kg, 轴向转动惯量均为1.4 kg$\cdot$m$^{2}$. 各滑轮参照系位于滑轮中面, 卷筒和各滑轮参照系的坐标轴均与总体坐标系相应坐标轴平行, 其原点坐标如表1所示. 质量为5~t的重物与动滑轮通过一个简单机构连接. 连接机构使重物随动滑轮一起摆动, 但不随动滑轮绕轴向转动. 绳索直径为30 mm, 抗拉模量为$4.85\times10^6$ KN, 质量线密度为3.1 kg/m. 卷筒与定滑轮以及两滑轮间绳索均划分为5段.

Table 1
表1
表1卷筒和滑轮参照系原点
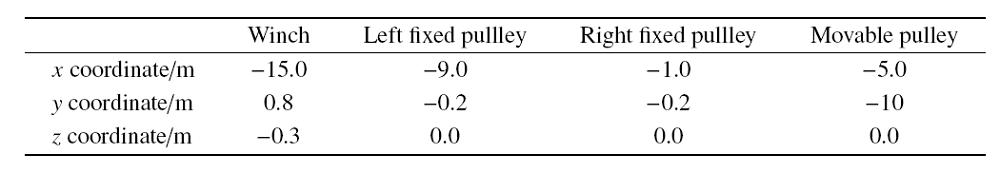Table 1Origins of coordinate systems for the winch and pulleys

新窗口打开|下载CSV

初始时刻, 卷筒入绳点在卷筒坐标系中的轴向坐标为0.345 m. 系统弧长坐标的原点为右端固定点. 出入绳点处的绳索预拉力均为26.6 KN, 相应的弧长坐标以及方位角和摆角如表2所示. 这些初值是通过求解系统非线性静平衡方程得到的. 求解非线性方程的初始值可通过求滑轮公切线获得.

Table 2
表2
表2初始时刻出入绳点处弧长以及方位角和摆角
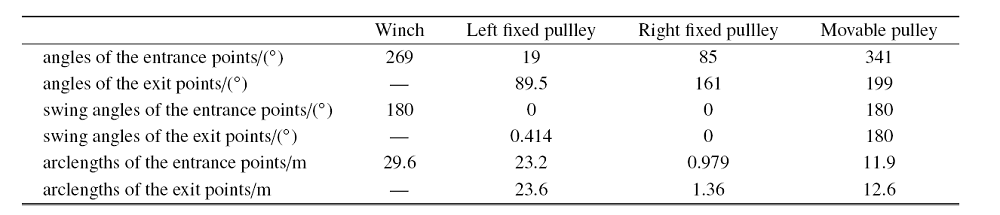Table 2Initially arclengths along with azimuth and swing angles of the rope at entrance and exit points

新窗口打开|下载CSV

利用本文所述方法(求解器: ODE45, 相对精度: $1.0\times10^{-3}$, 绝对精度: $1.0\times10^{-7}$, 磨光因子: 0.1)可以求得任意时刻卷筒上入绳点的位置、弧长坐标、绳索方位、系统中各滑轮的弧长坐标、出入绳角、绳索摆角、绳索拉力、滑轮的自转角、动滑轮形心矢径以及滑轮间绳索上4个空间点的矢径.

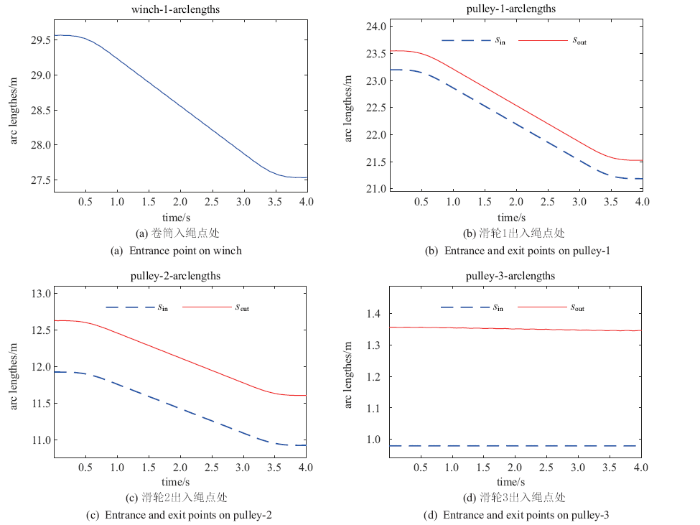
卷筒及其各滑轮的弧长坐标如图7(a)$\sim\!$图7(d)所示. 其中, $s_{\rm in} $和$s_{\rm out}$分别表示滑轮入绳点和出绳点弧长坐标. 从图中可见: 在卷筒加速和减速阶段, 卷筒的最大角速度不超过匀速段角速度, 弧长坐标的变化也相对平缓;pulley-3通过绳索与固定点相连, 其入绳点和出绳点的弧长坐标基本不变.

图7

新窗口打开|下载原图ZIP|生成PPT
图7弧长坐标

Fig.7Arclengths

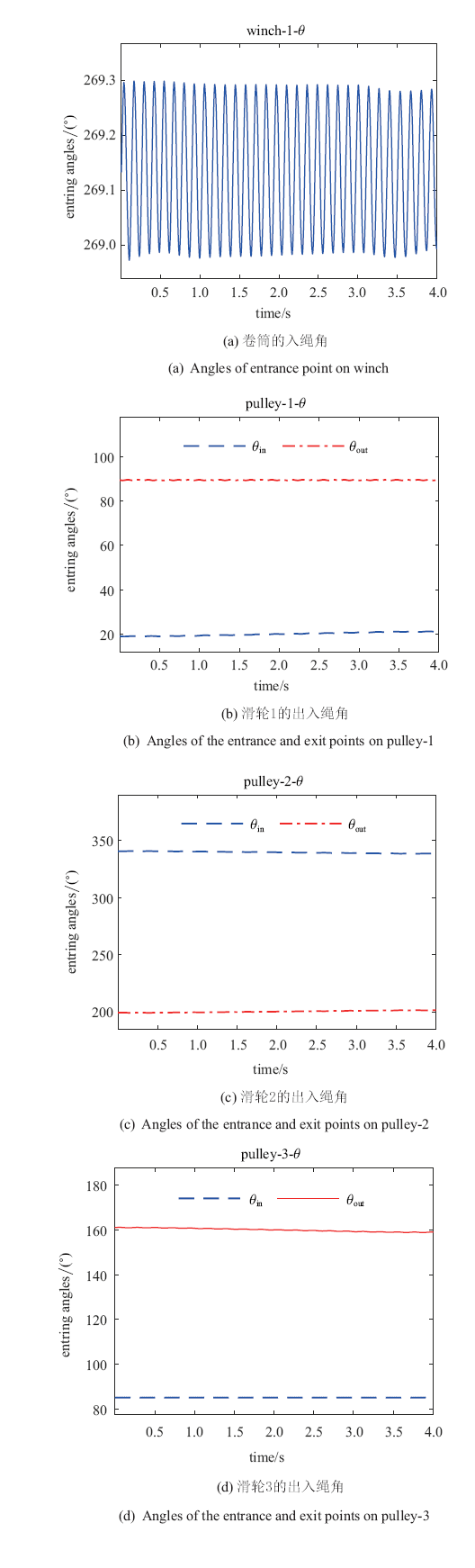
卷筒及其各滑轮的出入绳角如图8(a) $\sim\!$图8(d)所示. 其中, $\theta _{\rm in} $和$\theta_{\rm out} $分别表示滑轮的入绳角和出绳角.

图8

新窗口打开|下载原图ZIP|生成PPT
图8出入绳

Fig.8Angles of the entrance and exit points

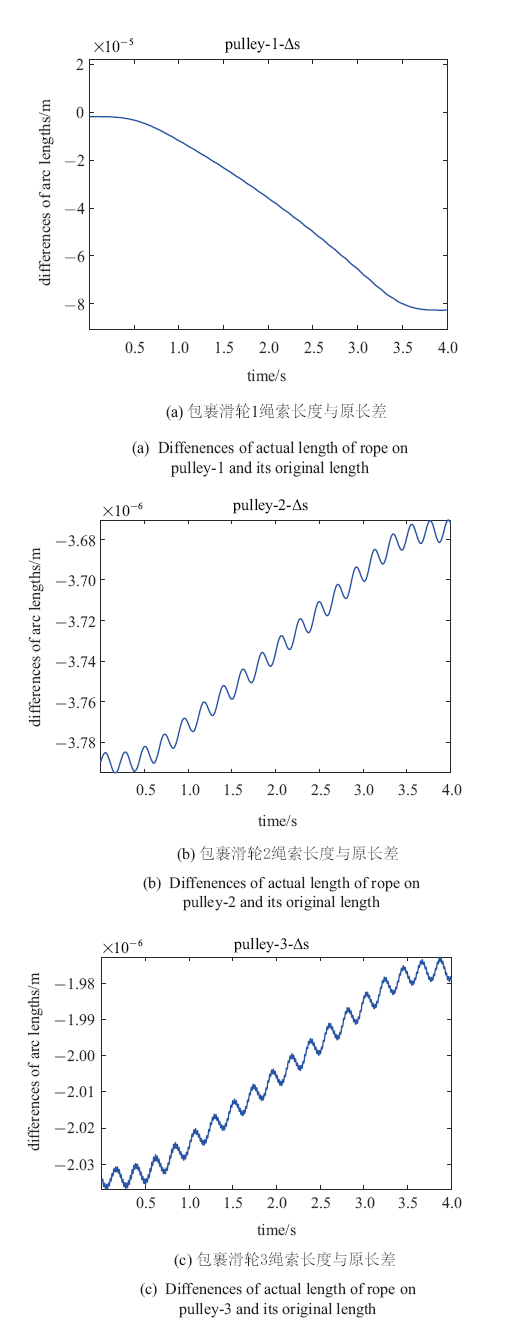
角包裹滑轮的绳索长度为$r( {\theta _{\rm out} - \theta _{\rm in} })$, 其中$r = 0.27$ m为滑轮的半径. 这段绳索的原长为$s_{\rm out} -s_{\rm in}$, 两者之差如图9(a)$\sim\!$图9(c)所示. 从中可见: 包裹滑轮的绳索长度与其原长相差十分微小. 两者相差的原因是由绳索中的拉力造成的.

图9

新窗口打开|下载原图ZIP|生成PPT
图9包裹滑轮绳索长度与原长差

Fig.9Differences of actual length of rope and its original length

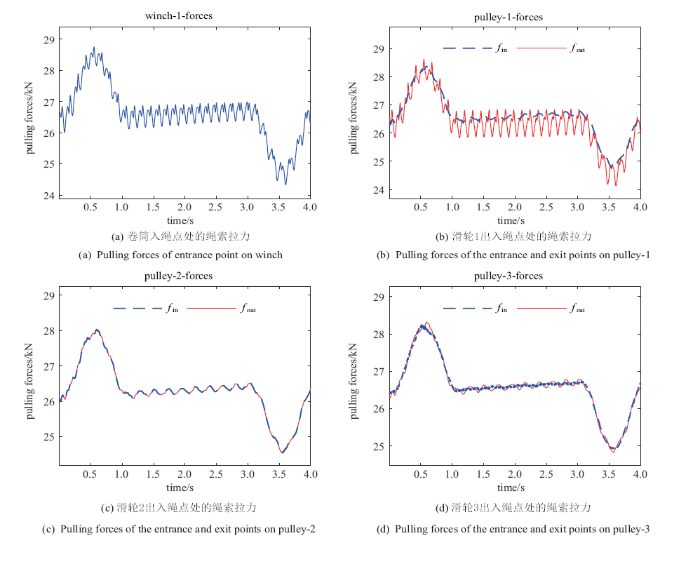
卷筒及其各滑轮出入绳点处绳索拉力如图10(a) $\sim\!$图10(d)所示. 其中, $f_{\rm in}$和$f_{\rm out} $分别表示滑轮入绳点和出绳点处的绳索拉力.

图10

新窗口打开|下载原图ZIP|生成PPT
图10绳索拉力从图中可见: 当卷筒转速增大和减小时, 卷筒和滑轮边界点处的拉力也随之出现波峰和波谷;当卷筒匀速转动时, $f_{\rm in}$和$f_{\rm out} $在相应的均值26$\sim$27 KN附近微幅振荡. 该均值与吊重49 KN的一半相当. 由于各滑轮的转动惯量很小, 滑轮两边的绳索拉力基本相等. 造成两者之间微小差异的原因除滑轮的转动惯量外, 滑轮的出入绳角以及摆角的变化也会使$f_{\rm in}$与$f_{\rm out} $有微小偏差.

Fig.10Pulling forces

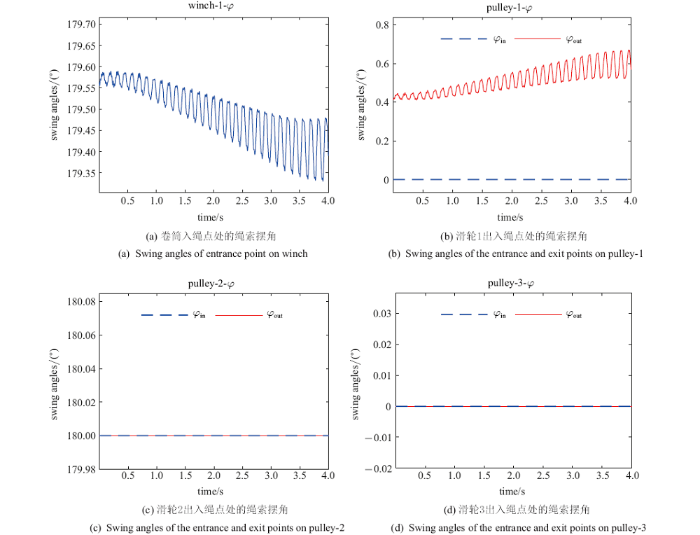
卷筒及其各滑轮的出入绳点处绳索摆角如图11(a)$\sim\!$图11(d)所示. 其中, $\varphi _{\rm in}$和$\varphi _{\rm out}$分别表示绳索在滑轮入绳点和出绳点处的摆角. 它表示在滑轮轴向和入绳点(出绳点)处的切线组成的平面内, 绳索沿弧长增加方向与切线间的夹角. 由于3个滑轮的中截面彼此共面, 从左端定滑轮入绳点到固定点间的绳索也几乎处于一个平面内, 这段绳索的行进方向无滑轮轴向分量, 相应的摆角接近于0或$\pi$. 卷筒转动过程中, 卷筒上绳索沿卷筒轴向移动(如图12所示), 造成卷筒入绳点和左端定滑轮出绳点处的摆角也随之变化, 正如图11(a)和图11(b)所示.

图11

新窗口打开|下载原图ZIP|生成PPT
图11绳索摆角

Fig.11Swing angles of the entrance and exit points

图12

新窗口打开|下载原图ZIP|生成PPT
图12卷筒上入绳点的轴向坐标

Fig.12Axial coordinates of entrance points on winch

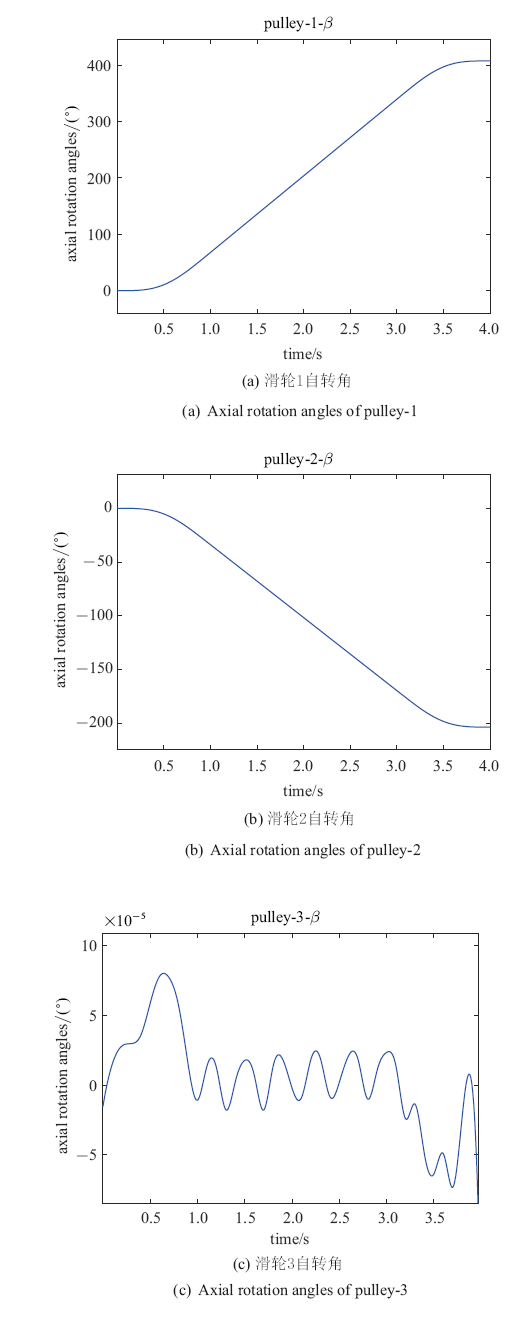
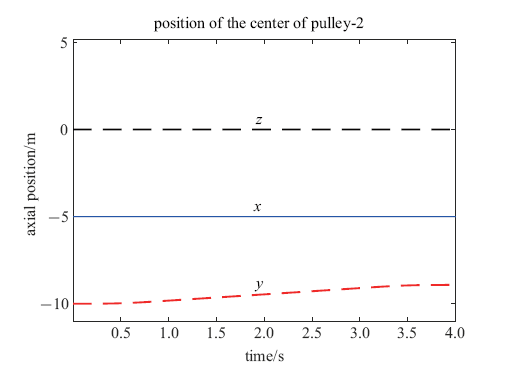
如图13(a) $\sim\!$图13(c)所示, 绳索的流动带动各滑轮也随之转动. 从滑轮1流出的绳索其原长共计2.026 m,相应地滑轮1的自转角应共计为2.0206/0.27弧度(430$^\circ$);从滑轮2流出的绳索其原长共计1.0243 m, 相应地滑轮2的自转角应共计为1.0243/0.27弧度(217$^\circ$);滑轮3与固定点相连, 其自转角接近于零. 从动滑轮流出的绳索长度之所以比从左端定滑轮流出的绳索长度小, 是由于动滑轮的形心向上提升造成的(如图14所示).

图13

新窗口打开|下载原图ZIP|生成PPT
图13自转角

Fig.13Axial rotation angles

图14

新窗口打开|下载原图ZIP|生成PPT
图14动滑轮轮心位置

Fig.14Position of the center of the movable pulley

9 结论

复杂系统动力学分析包含两个核心步骤: 选取系统描述参数和建立这些参数的演化方程.

(1)将不与物质点固定但又有明确物理意义的点作为描述参数, 可有效克服传统方法所引起的困难.

(2)依据虚功率原理可建立复杂系统参数演化方程. 值得特别引起注意的是: 虚功率方程中出现的虚速度是质点物质速度的虚速度, 相应的加速度也应是物质加速度.

(3)空间点的物质速度和加速度可由其空间速度和加速度推演得到, 其表达式中分别含有矢径对弧长的一、二阶导数. 建立节点为空间点的单元时应考虑由此派生出的连续性要求.

(4)按上述原理建立的``动态节点绳索单元''适应滑轮绳索系统的特点, 克服了传统方法中滑轮半径严重限制单元尺寸、必须将滑轮绳索间接触力引入系统动力学方程等困难, 能够对滑轮绳索系统进行深入精细的分析.

参考文献原文顺序
文献年度倒序
文中引用次数倒序
被引期刊影响因子

[1]

Zienkiewicz

, Taylor

, Zhu

. The Finite Element Method: Its Basis and Fundamentals
Elsevier Science, 2013

DOI URL PMID [本文引用: 1]

The reduction of esters and carboxylic acids to alcohols is a highly relevant conversion for the pharmaceutical and fine-chemical industries and for biomass conversion. It is commonly performed using stoichiometric reagents, and the catalytic hydrogenation of the acids previously required precious metals. Here we report the homogeneously catalyzed hydrogenation of carboxylic acids to alcohols using earth-abundant cobalt. This system, which pairs Co(BF4)2·6H2O with a tridentate phosphine ligand, can reduce a wide range of esters and carboxylic acids under relatively mild conditions (100°C, 80 bar H2) and reaches turnover numbers of up to 8000.

[2]

Zienkiewicz

, Taylor

. The Finite Element Method for Solid and Structural Mechanics
Elsevier Science, 2013

DOI URL PMID

Zienkiewicz

, Taylor

, Nithiarasu

. The Finite Element Method for Fluid Dynamics
Elsevier Science, 2013

DOI URL PMID [本文引用: 1]

钱伟长 . 广义变分原理. 上海: 知识出版社, 1985

[本文引用: 1]

( Qian

Weichang

. Generalized Variational Principle. Shanghai: Knowledge Publishing House, 1985 (in Chinese))

[本文引用: 1]

[5]

龙驭球, 龙志飞, 岑松 . 新型有限元论. 北京: 清华大学出版社, 2003

[本文引用: 1]

( Long

Yuqiu

, Long

Zhifei

, Cen

Song

. New Finite Element Theory. Beijing: Tsinghua University Press, 2003 (in Chinese))

[本文引用: 1]

[6]

Saritas

, Soydas

. Variational base and solution strategies for non-linear force-based beam finite elements
International Journal of Non-Linear Mechanics, 2012,47(3):54-64

DOI URL [本文引用: 1]

This paper presents the variational bases for the non-linear force-based beam elements. The element state determination of these elements is obtained exactly from a two-field functional with independent stress and strain fields. The variational base of the non-linear force-based beam elements implemented in a general purpose displacement-based finite element program requires the inclusion of independent displacement field in the formulation. For this purpose, a three-field functional is considered with independent displacement, stress, and strain fields. Various local and global solution strategies come out from the mixed formulation of the beam element, and these are shown to yield the algorithms presented for non-linear force formulation beam elements in literature; thus removing any doubts on their variational bases. The presented numerical examples demonstrate the accuracy and robustness of the solution algorithms adapted for mixed formulation elements over popularly used displacement-based beam finite elements even for large structural systems. (C) 2012 Elsevier Ltd.

[7]

Lee

, Angela

, Hale

, et al. Strain smoothing for compressible and nearly-incompressible finite elasticity
Computers & Structures, 2017,182(1):540-555

DOI URL PMID

Active development of quantum informational components such as quantum computers and quantum key distribution systems requires parameter characterization of single photon detectors. A key property of the single photon detectors is detection efficiency. One of the methods of the detection efficiency measurement, as listed in the international standard ETSI, is the reference-free twin-photon-based Klyshko method. The signal-to-noise ratio (SNR) of this method depends on the combination of the pump wavelength, the nonlinear crystal's axis angle, and the type of detector's sensitive element. When the combination is difficult, one has to deal with the low SNR of the detector counts measurement. To gain the high SNR, one has to average the long record complicated with the &quot;random telegraph signal&quot; noise. This type of noise exhibits high spectral density at a zero frequency, where simple averaging works. The heterodyne based method we have proposed is to perform averaging at the higher frequency of the modulation introduced to the standard Klyshko measurement scheme. The method was numerically simulated and experimentally tested. The 14 times improvement in SNR for the proposed method relative to the simple averaging was demonstrated by the numerical simulation and confirmed experimentally.

[8]

Antonietti

, Verani

, Vergara

, et al. Numerical solution of fluid-structure interaction problems by means of a high order Discontinuous Galerkin method on polygonal grids
Finite Elements in Analysis & Design, 2019,159(1):1-14

DOI URL PMID

?The objectives of this study were to validate a finite element model of the equine distal limb transfixation cast and to determine the effect of six transcortical pin parameters on bone-pin interface (BPI) stresses in the third metacarpal bone.

[9]

Sun

, Chen

, Yang

. The families of nonconforming mixed finite elements for linear elasticity on simplex grids
Applied Mathematics and Computation, 2019,358(1):348-362

DOI URL

[10]

, Niu

, Liu

. Highly accurate smoothed finite element methods based on simplified eight-noded hexahedron elements
Engineering Analysis with Boundary Elements, 2019,105(1):165-177

DOI URL

[11]

Durand

, Pantoja-Rosero

, Oliveira

. A general mesh smoothing method for finite elements
Finite Elements in Analysis & Design, 2019,158(1):17-30

DOI URL PMID

何涛 . 基于ALE有限元法的流固耦合强耦合数值模拟
力学学报, 2018,50(2):395-404

( He

Tao

, A partitioned strong coupling algorithm for fluid-structure interaction using arbitrary lagrangian-eulerian finite element formulation
Chinese Journal of Theoretical and Applied Mechanics, 2018,50(2):395-404 (in Chinese))

[13]

江守燕, 李云, 杜成斌 . 改进型扩展比例边界有限元法
力学学报, 2019,51(1):278-288

[本文引用: 1]

( Jiang

Shouyan

, Li

Yun

, Du

Chengbin

, Improved extended scaled boundary finite element methods
Chinese Journal of Theoretical and Applied Mechanics, 2019,51(1):278-288 (in Chinese))

[本文引用: 1]

[14]

Murdoch

. Physical Foundations of Continuum Mechanics. Cambridge: Cambridge University Press, 2012

[本文引用: 1]

[15]

金栋平, 文浩, 胡海岩 . 绳索系统的建模、动力学和控制
力学进展, 2004,34(3):304-313

URL [本文引用: 1]

绳索系统具有无限自由度,当计入非线性因素的作用时,其面内和面外的振动相互耦合,呈现非常丰富的非线性动力学行为.另外,绳索系统经常工作在风、流体、微重力、电磁力等作用下,进一步加剧了其动力学的复杂性.绳索系统的动力学现象引起了工程界和力学界的关注.本文对绳索在重要工程系统中的应用及相应的动力学现象进行概述,给出了柔索的动力学建模过程,对绳索系统的动力学和控制研究进行了总结,并指出了值得进一步关注的若干问题.

( Jin

Dongping

, Wen

Hao

, Hu

Haiyan

, Modeling,dynamics and control of cable systems
Advances in Mechanics, 2004,34(3):304-313 (in Chinese))

URL [本文引用: 1]

胡海岩, 田强, 张伟等. 大型网架式可展开空间结构的非线性动力学与控制
力学进展, 2013,43(4):390-414

DOI URL

我国航天工业迫切需要掌握可入轨后展开的大型网架式空间结构技术，以便研制口径十几米、乃至数十米的大型星载天线。该技术的主要科学基础是这类空间结构展开和服役过程的非线性动力学建模、分析和控制。本文综述了与上述科学基础相关的研究进展，提出应重点关注的三个科学问题：一是大型网架式空间结构展开过程的多柔体系统动力学，尤其是如何对微重力环境下索网的接触和缠绕、运动副内碰撞、结构展开与航天器本体间的耦合等导致的非线性动力学进行建模和分析；二是大型网架式空间结构展开锁定后服役的动力学分析，尤其是如何揭示结构柔性、众多运动副间隙、交变热载荷等因素引起的复杂非线性振动机理；三是大型网架式空间结构展开锁定后服役的动力学控制，尤其是如何在欠驱动、低能耗条件下对非线性振动和波动传播提出有效的控制方法。

( Hu

Haiyan

, Tian

Qiang

, Zhang

Wei

, et al. Nonlinear dynamics and control of large deployable space structures composed of trusses and meshes
Advances in Mechanics, 2013,43(4):390-414 (in Chinese))

DOI URL

浦汉军, 谢小鹏, 牛高产等. 钢丝绳吊重提升系统的动载分析
中国机械工程, 2012,23(24):2926-2929

URL

建立了钢丝绳吊重提升系统的动力学模型,将精细积分法用于该模型的动载分析,研究了索具刚度对起升动载系数的影响,较真实地反映了钢丝绳吊重离地起升过程中的动态特性。实验表明,该方法计算量小,精度高,易于分析系统在不同起升速度下的动载荷。


( Pu

Hanjun

, Xie

Xiaopeng

, Niu

Gaochan

, et al. Study on dynamic load in load-lifting system of wire rope
China Mechanical Engineering, 2012,23(24):2926-2929 (in Chinese))

URL

建立了钢丝绳吊重提升系统的动力学模型,将精细积分法用于该模型的动载分析,研究了索具刚度对起升动载系数的影响,较真实地反映了钢丝绳吊重离地起升过程中的动态特性。实验表明,该方法计算量小,精度高,易于分析系统在不同起升速度下的动载荷。


[18]

沈文厚, 赵治华, 任革学等. 拦阻索冲击的多体动力学仿真研究
振动与冲击, 2015,34(5):73-77

URL

舰载机着舰时，尾钩冲击拦阻索导致索内应力动态向甲板两端传播。为研究拦阻索应力波传播规律及峰值决定因素，本文在多体动力学框架下，发展了基于绝对节点坐标法的具有接触碰撞功能的大位移索单元，在此基础上建立了包含缓冲装置的拦阻系统多体动力学模型，仿真揭示了拦阻索受冲击后应力波在传播过程中，分别在甲板两侧的导向轮和尾钩处，因横波的反射与叠加均造成了应力尖峰，而滑轮缓冲装置与钢索末端缓冲装置均能有效的降低索内应力峰值。拦阻系统中的钢索用轻质材料代替，亦能降低索内应力峰值。此外，本文建立的全尺寸舰载机拦阻系统多体动力学模型为系统的设计与优化提供了一种计算手段。

( Shen

Wenhou

, Zhao

Zhihua

, Ren

Gexue

, et al. Multi-body dynamic simulation of impact on cross deck pendant
Journal of Vibration and Shock, 2015,34(5):73-77 (in Chinese))

URL

Wang

, Cao

, Wang

, et al. A novel driving strategy for dynamic simulation of hoisting rope with timeivarying length
International Journal of Modeling Simulation & Scientific Computing, 2013,4(3):135009-1350025

DOI URL PMID

One of two empagliflozin phase 3 trials in type 1 diabetes (EASE-3 but not EASE-2) included a lower 2.5 mg dose. A placebo-corrected HbA1c reduction 0.28% was demonstrated without the increased risk of diabetic ketoacidosis observed at higher doses (10 mg and 25 mg). Since only one trial included the lower dose, we aimed to confirm the observed reduction in HbA1c for the 2.5 mg dose by modeling and simulation analyses.

[20]

陈伟海, 陈泉柱, 刘荣强等. 绳驱动拟人臂机器人回零算法分析
浙江大学学报(工学版), 2013,47(2):345-352

DOI URL

为解决绳驱动拟人臂机器人在运动过程中产生的误差累积问题以及确定断电后重启的位姿，介绍一种不依靠外部设备的回零方法，即仅依靠自身检测的传感信息和相应的算法实现自回零.针对三自由度的并联球关节的自动回零问题，结合绳驱动并联机器人的结构特点，系统采用增量式编码器与限位开关相结合作为回零检测装置|在此基础上，对机构的运动耦合性进行了分析，提出分三步实现机器人动平台的原点定位与自动回零方法，并通过解耦控制，实现每步回零运动只需主动控制一条驱动绳索|仿真与实验验证这种三步自动回零算法的可行性，能够灵活准确的使机器人回到初始状态.

( Chen

Weihai

, Chen

Quanzhu

, Liu

Rongqiang

, et al. Homing algorithm analysis of a cable-driven humanoid-arm manipulator
Journal of Zhejiang University (Engineering Science), 2013,47(2):345-352 (in Chinese))

DOI URL

谭春林, 魏承 . 绳索动力学基础研究. 北京: 科学出版社, 2018

( Tan

Chunlin

, Wei

Cheng

. Basic Research of Rope Dynamics. Beijing: Science Press, 2018 (in Chinese))

[22]

王郡, 朱永宁, 徐鉴 . 自主泳动弹性绳的轨迹模拟
力学学报, 2019,51(1):198-208

[本文引用: 1]

( Wang

Jun

, Zhu

Yongning

, Xu

Jian

, Trajectory simulation of self-propelled elastic rods in fluid
Chinese Journal of Theoretical and Applied Mechanics, 2019,51(1):198-208 (in Chinese))

[本文引用: 1]

[23]

齐朝晖, 宋慧涛, 张志刚 . 核环吊平衡路径与吊重轨迹偏差
工程力学, 2014,31(1):209-217

[本文引用: 1]

( Qi

Zhaohui

, Song

Huitao

, Zhang

Zhigang

, Equilibrium paths and load trajectory deflections of nuclear ring cranes
Engineering Mechanics, 2014,31(1):209-217 (in Chinese))

[本文引用: 1]

[24]

Fritzkowski

, Kaminski

. Dynamics of a rope modeled as a multi-body system with elastic joints
Computational Mechanics, 2010,46(6):901-909

DOI URL [本文引用: 1]

A discrete model of a rope with spiral springs in joints is considered, the aim being to include transverse elasticity of the rope. Elastic characteristic of the springs is derived on the basis of simple geometrical formulas and the classical curvature-bending moment relationship for beams. Lagrange’s equations of motion are presented and their complexity is discussed from the computational point of view. Numerical experiments are performed for a system with both scleronomic and rheonomic constraints. The influence of the elasticity on behaviour of the model is analyzed. Results validity is examined in terms of basic energy principles.

[25]

Kamman

, Huston

. Multibody dynamics modeling of variable length cable systems
Multibody Syst Dyn, 2001,5(3):211-221

DOI URL [本文引用: 1]

This paper presents a procedure for studying the dynamics ofvariable length cable systems. Such systems commonly occur in deploymentand retrieval (pay-out and reel-in) in cable towing systems such as inship and marine applications.The cable is modeled as a chainand treated as a multibody system. The chain links in turn are modeledas lumped masses. The pay-out/reel-in process is modeled with variablelength links near the towing point.

[26]

Danilin

, Grishanina

, Shklyarchuk

, et al. Dynamics of a space vehicle with elastic deploying tether
Computers & Structures, 1999,72(1-3):141-147

DOI URL PMID [本文引用: 1]

Russell

, Anderson

. Equilibrium and stability of a whirling rod-mass system
International Journal of Non-Linear Mechanics, 1977,12(2):91-101

DOI URL [本文引用: 1]

[28]

Kamman

, Huston

. Dynamics of constrained multibody systems
Journal of Applied Mechanics, 1984,51(4):899-903

DOI URL PMID [本文引用: 1]

Inverse dynamics is a technique in which measured kinematics and, possibly, external forces are used to calculate net joint torques in a rigid body linked segment model. However, kinematics and forces are usually not consistent due to incorrect modelling assumptions and measurement errors. This is commonly resolved by introducing 'residual forces and torques' which compensate for this problem, but do not exist in reality. In this study a constrained optimization algorithm is proposed that finds the kinematics that are mechanically consistent with measured external forces and mimic the measured kinematics as closely as possible. The algorithm was tested on datasets containing planar kinematics and ground reaction forces obtained during human walking at three velocities (0.8 m/s, 1.25 and 1.8 m/s). Before optimization, the residual force and torque were calculated for a typical example. Both showed substantial values, indicating the necessity of developing a mechanically consistent algorithm. The proposed optimization algorithm converged to a solution in which the residual forces and torques were zero, without changing the ground reaction forces and with only minor changes to the measured kinematics. When using a rigid body approach, our algorithm ensures a consistent description of forces and kinematics, thereby improving the validity of calculated net joint torque and power values.

[29]

谭益松, 刘伊威, 刘宏等. 空间大型末端执行器柔性钢丝绳的建模与捕获动力学
机器人, 2011,33(2):156-160

URL

针对大容差软捕获这一空间捕获操作的重要准则，研制了基于钢丝绳的末端执行器大容差柔性捕获机构.针对钢丝绳具有较大柔性，在空间应用环境下难于快速连续建模控制的问题，提出了柔性钢丝绳的离散控制模型，解决了钢丝绳的建模问题.通过钢丝绳的离散模型，分析了末端执行器钢丝绳柔性机构捕获空间自由飞行载荷舱的动力学问题.实验证明钢丝绳模型建立正确，钢丝绳捕获动力学推导合理.

( Tan

Yisong

, Liu

Yiwei

, Liu

Hong

, et al. Modeling and capture dynamics of flexible cables used in large-scale space end-effector
Robot, 2011,33(2):156-160 (in Chinese))

URL

Wittbrodt

, Adamiec-Wójcik

, Wojciech

. Dynamics of Flexible Multibody Systems: Rigid Finite Element Method
Berlin: Springer, 2006

[本文引用: 1]

[31]

, Choo

. Super element approach to cable passing through multiple pulleys
International Journal of Solids & Structures, 2005,42(11-12):3533-3547

DOI URL PMID [本文引用: 1]

Although a resistivity saturation (minimum conductivity) is often observed in disordered metallic solids, such phenomena in the corresponding liquids are not known. Here we report a saturation of the electrical resistivity in Zr_{64}Ni_{36} and Cu_{50}Zr_{50} liquids above a dynamical crossover temperature for the viscosity (T_{A}). The measurements were made for the levitated liquids under the microgravity conditions of the International Space Station. Based on recent molecular dynamics simulations, the saturation is likely due to the ineffectiveness of electron-phonon scattering above T_{A} when the phonon lifetime becomes too short compared to the electron relaxation time. This is different from the conventional resistivity saturation mechanisms in solids.

[32]

Hong

, Ren

. A modeling of sliding joint on one dimensional flexible medium
Multibody Syst Dyn, 2011,26:91-106.

DOI URL [本文引用: 1]

The dynamic modeling of a sliding joint on a one-dimensional medium, such as a cable or a beam, is studied in this paper. The sliding joint is implemented by positioning it at a moving node on the one-dimensional medium, which is realized by variable-length elements at either side of the joint. The variable-length element is established with an absolute nodal coordinate formulation (ANCF) in the framework of the Arbitrary Lagrange-Euler (ALE) description. The sliding of the joint is described by the increasing of the length on one side of the one-dimensional medium and a corresponding decreasing of the other side. In order to capture the discontinuity of the slopes at the position of the sliding joint, the moving node has two slopes as generalized coordinates which are equal to each other in the case of a beam but not in the case of a cable, and in order to avoid the addition-deletion constraint, the node adjacent to the moving node is added or deleted if the element is too long or too short. The governing equations for the coupled system are derived in terms of D'Alembert's principle and the resulting equations of motion are formulated in the standard form of differential algebraic equations of multibody systems. Numerical examples are presented to validate the method proposed by comparing with analytical results which are available or are made possible by simplifying the model.

[33]

Escalona

JoséL

. An arbitrary Lagrangian--Eulerian discretization method for modeling and simulation of reeving systems in multibody dynamics
Mechanism and Machine Theory, 2017,112:1-21

DOI URL [本文引用: 1]

[34]

Sun

, Tian

, Hu

, et al. Axially variable-length solid element of absolute nodal coordinate formulation
Acta Mechanica Sinica, 2019,35(3):653-663

DOI URL

[35]

, Bao

, Cui

, et al. Dynamic analysis of cable-driven parallel manipulators with time-varying cable lengths
Finite Elements in Analysis and Design, 2012,48(1):1392-1399

DOI URL [本文引用: 1]

In conventional researches, cables of cable-driven parallel manipulators are treated as simple linear elements that can only work in tension. This results in the fact that the effect of cable dynamics on the positioning precision of the end-effector is not adequately taken into account. To overcome this shortcoming, a dynamic model for cable-driven parallel manipulators with cables of slowly time-varying length is presented in this paper. The partial differential equation characterizing the dynamics of a cable with varying-length is deduced, and converted into ordinary differential equations through spatial discretization by finite difference approximation. Then, the dynamic model for cable-driven parallel manipulators is achieved considering the relationship between the motion of the end-effector and the cable end force, in which the degrees of freedom of cables and the end-effector are all involved. Two numerical examples are demonstrated to validate the dynamic model, and also show that it is necessary to take into consideration the cable dynamics for manipulators of long-span cables. (C) 2011 Elsevier B.V.

[36]

Banfi

, Marzocchi

, Musesti

. On the principle of virtual powers in continuum mechanics
Ricerche Di Matematica, 2006,55(2):139-150

DOI URL [本文引用: 1]

We give a general formulation of the Principle of virtual powers in Continuum Mechanics from a distributional point of view, and study some of its relevant consequences in the field of balance equations. Keywords: Virtual Powers, Contact Interactions, Balance Equations

[37]

Maugin

. The principle of virtual power: from eliminating metaphysical forces to providing an efficient modelling tool
Continuum Mechanics & Thermodynamics, 2013,25(2-4):127-146

DOI URL PMID [本文引用: 1]

A new therapeutic option to treat osteoporosis is focused on Wnt signaling and its inhibitor sclerostin, a product of the Sost gene. In this work, we study the effect of sclerostin deficiency on trabecular bone formation and resorption in male and female mice and whether it affects mechano-responsiveness. Male and female 10- and 26-week-old Sost knockout (KO) and littermate controls (LCs) were subjected to in vivo mechanical loading of the left tibia for 2?weeks. The right tibia served as internal control. The mice were imaged using in vivo micro-computed tomography at days 0, 5, 10, and 15 and tibiae were collected for histomorphometric analyses after euthanasia. Histomorphometry and micro-CT-based 3D time-lapse morphometry revealed an anabolic and anti-catabolic effect of Sost deficiency although increased trabecular bone resorption accompanied by diminished trabecular bone formation occurred with age. Loading led to diminished resorption in adult female but not in male mice. A net gain in bone volume could be achieved with mechanical loading in Sost KO adult female mice, which occurred through a further reduction in resorbed bone volume. Our data show that sclerostin deficiency has a particularly positive effect in adult female mice. Sclerostin antibodies are approved to treat postmenopausal women with high risk of osteoporotic fractures. Further studies are required to clarify whether both sexes benefit equally from sclerostin inhibition.

[38]

齐朝晖, 曹艳, 王刚 . 多柔体系统数值分析的模型降噪方法
力学学报, 2018,50(4):863-870

[本文引用: 1]

( Qi

Zhaohui

, Cao

Yan

, Wang

Gang

, Model smoothing methods in numerical analysis of flexible multibody systems
Chinese Journal of Theoretical and Applied Mechanics, 2018,50(4):863-870 (in Chinese))

[本文引用: 1]