复合柔性结构全局模态函数提取与状态空间模型构建 1)

删除或更新信息，请邮件至freekaoyan#163.com(#换成@)

本站小编 Free考研考试/2022-01-01

魏进^*, 曹登庆^??^,²⁾, 于涛^*

* 烟台大学机电汽车工程学院,山东烟台 264005

?? 哈尔滨工业大学航天学院,哈尔滨 150001

EXTRACTION OF GLOBAL MODE FUNCTIONS AND CONSTRUCTION OF STATE SPACE MODEL FOR A COMPOSITE FLEXIBLE STRUCTURE ¹⁾

Wei Jin^*, Cao Dengqing^??^,²⁾, Yu Tao^*

* School of Electromechanical and Automotive Engineering, Yantai University, Yantai 264005, Shandong, China

? School of Astronautics, Harbin Institute of Technology, Harbin 150001, China

收稿日期:2018-10-28接受日期:2019-02-1网络出版日期:2019-03-18

基金资助:

国家自然科学基金重点项目资助.11732005

Received:2018-10-28Accepted:2019-02-1Online:2019-03-18
作者简介 About authors
2)曹登庆,教授,主要研究方向：结构动力学与振动控制.E-mail:dqcao@hit.edu.cn

摘要
随着航空航天等领域中实际工程结构的大型化和柔性化,结构的非线性振动和主动振动控制问题越来越凸显.分析和处理此类结构出现的复杂振动问题的关键在于建立系统的非线性动力学模型与状态空间模型.对于由柔性部件、刚体、连接部件构成的复合柔性结构,由于各部件之间的振动耦合效应,单个柔性部件在悬臂、简支和自由等静定边界下的模态与结构的真实模态有较大差异.为此,本文提出复合柔性结构全局模态的解析提取方法,通过全局模态离散得到系统非线性动力学模型,从而构建状态空间模型.该方法采用笛卡尔坐标描述系统的运动,建立系统的运动方程;结合描述柔性部件的偏微分方程、刚体的常微分运动方程、连接界面处力、力矩、位移和转角的匹配条件以及系统的边界条件,利用分离变量法给出统一形式的频率方程,获取系统的固有频率和解析函数表征的全局模态.这里提出的全局模态提取方法不仅便于复合柔性结构固有频率和全局模态的参数化分析,而且为建立复合柔性结构低维非线性动力学模型和状态空间模型提供了有效的途径,对于推进这类结构的非线性动力学分析与主动振动控制研究具有重要意义.
关键词： 柔性结构;全局模态;解析方法;非线性动力学;振动控制

Abstract
With the scale enlarging and flexibility of the actual engineering structures utilized in aerospace and other fields, the issues on the study of nonlinear vibration and active vibration control of the structure become more and more important. The key process of dealing with the vibration and control for such a kind of structure is to establish the nonlinear dynamic model and formulate the state space model of the system. For composite flexible structures composed of flexible components, rigid bodies and flexible joints, because of the vibration coupling between each part of the structure, the modes of an individual flexible component with the cantilever, simply supported and free stationary boundary are different from the real mode of the structure. In this paper, an analytic extraction method of global modes of composite flexible structures is presented, and the nonlinear dynamic model and the state-space model of the system can be obtained by the global mode discretization. Adopting the Cartesian coordinates to describe the motion of the system, establishing the motion equations of the system, and combining with the partial differential equation of the flexible part, the ordinary differential motion equation of the rigid body, the matching condition of force, moment, slope of the deflection curve and displacement at the interface, and the boundary condition of the system, the frequency equation of uniform form is given by using the separating variable method. Consequently, the natural frequencies and the global mode representation of the analytic function of the system are obtained. The global mode extraction method presented here not only facilitates the parametric analysis of the natural frequencies and global modes of composite flexible structures, but also provides an effective way to establish the low dimensional nonlinear dynamic model and the state space model of the composite flexible structure, which is of great significance for the study of nonlinear dynamic responses and the design of active vibration control of this kind of structures.
Keywords：flexible structures;global modes;analytic method;nonlinear dynamics;vibration control

PDF (2593KB)元数据多维度评价相关文章导出EndNote|Ris|Bibtex 收藏本文
本文引用格式
魏进, 曹登庆, 于涛. 复合柔性结构全局模态函数提取与状态空间模型构建 ¹⁾. 力学学报[J], 2019, 51(2): 341-353 DOI:10.6052/0459-1879-18-356
Wei Jin, Cao Dengqing, Yu Tao. EXTRACTION OF GLOBAL MODE FUNCTIONS AND CONSTRUCTION OF STATE SPACE MODEL FOR A COMPOSITE FLEXIBLE STRUCTURE ¹⁾. Chinese Journal of Theoretical and Applied Mechanics[J], 2019, 51(2): 341-353 DOI:10.6052/0459-1879-18-356

引言

复合柔性结构是由多个柔性部件(包括细长杆、薄板、薄壳等)、刚体以某种方式相联接而构成的多体系统. 在航空航天^[1]、机械^[2]、土木^[3]等工程领域中,诸如带太阳能帆板的空间飞行器、带外挂(飞机发动机)的飞机机翼、带细长叶片的大型旋转机械、大跨度斜拉桥等都可以归结为复合柔性结构. 随着空间科学与技术的不断进步与发展,柔性部件的大型化和柔性化不可避免,整个柔性结构的动力学建模与振动响应分析、结构振动控制等问题变得愈加复杂且难以处理^[4].

对于复合柔性结构,构成部件多为具有无限自由度的柔性体,描述其运动的动力学方程为偏微分方程. 无论是对系统的动力学特性进行分析,还是基于其动力学模型的状态空间进行控制器设计,都要建立具有有限自由度的动力学模型. 实际工程应用中,通常采用有限元法对复杂结构进行离散化处理,只有在结构相对简单(包括单一的索、杆、梁、板、壳等)时才采用解析方法建模.

有限元法可以离散具有复杂形状的复合柔性结构,在单元数量足够多的情况下,有限元模型所得到的结果可以达到较高的精度.因此,许多****^[5-9]采用有限元法,建立系统的有限元模型,进行动力学特性的分析和研究.例如,Al-Bedoor等^[7]建立了含有柔性关节的柔性机械臂的有限元模型,并开展了柔性关节的扭转变形对于系统动力学的影响研究.采用有限元商业软件(如Ansys、Abaqus、Nastran等)建模,也是分析复合柔性结构固有特性的有效途径^[10-13]. 例如,代颖等^[12]采用软件Ansys建立了车用电机的有限元模型,分析了各结构部件对电机固有特性的影响.然而,有限元模型为了保证其精度,需要较多的单元数,导致系统的离散动力学模型出现维数过高的弊端,这为系统的控制器设计或非线性动态响应分析带来了困难;并且由有限元商业软件得到的系统全局模态是以数值形式给出的,对于存在非线性环节的复合结构,很难建立解析形式的非线性动力学模型.

相比于有限元离散方法,采用解析形式的模态函数导出复合柔性结构的模态方程,利用Galerkin截断可以有效地降低系统的维数^[14],从而明显地提高求解系统动力学模型的计算效率. 因此,解析方法在多刚柔体系统动力学中得到了广泛的应用,尤其是在柔性航天器^[15-18]的动力学建模与控制器设计. 解析方法的关键在于将柔性部件的位移表示为部件模态与模态坐标的线性组合,所建立的动力学模型的准确性依赖于所选模态的精确性和数量,也即所选模态越能准确地描述柔性部件的真实变形,其需要的模态数量就越少,同时所推导出的动力学模型的准确性也越高. Pan和Liu^[19]通过钟摆和曲柄连杆机构的数例仿真结果,表明了边界为两端自由、简支或悬臂的振型函数适合于柔性多体系统的建模,能得到较好的结果. 但是,采用两端固支或具有超静定边界的振型函数可能导致系统动力学方程的计算结果出现较为严重的误差. Schwertassek等^[20]通过结合柔性附件的静变形和特征函数,构造出合适的振型函数用于描述柔性体的变形,从而提高系统动力学模型的准确性和收敛性.

对于由多个柔性部件组成的复合结构,各柔性部件之间的弹性振动耦合效应不可忽略. 系统中单一柔性部件在悬臂、简支和自由等静定边界下的模态与其在整体结构中的真实模态有较大差异. 采用柔性部件的假设模态建模,可能会导致其动力学模型精度下降,甚至出现较大误差. 为此,针对由中心刚体和柔性附件组成的航天器,Hablani^[21]指出,如果选取相同数量的模态,全局模态所得到的动力学模型比假设模态得到的模型具有更高的精度. 这是因为全局模态包含了系统中刚体的运动和全部柔性部件弹性振动的模态,选取少量的全局模态就能较好地描述系统的运动. 针对空间柔性机械臂系统,魏进等^[22]分别采用假设模态和全局模态建立了系统的动力学模型,并对两种模型进行了对比研究. 将有限元结果作为参考值,对比分析的结果表明全局模态方法所得结果的精度远高于假设模态方法给出的结果.

鉴于采用全局模态进行离散具有优越性,已有一些****通过全局模态离散,得到复合柔性结构的动力学离散模型.针对索——梁组合结构,Gattulli等^[23-24]、赵跃宇等^[25-26]和王志搴等^[27-28]利用边界条件和连接点的匹配条件,获取系统的固有频率和全局模态,通过全局模态进行离散,建立了系统的动力学离散模型.Hablani等^[29-31]采用系统的全局模态,建立了柔性航天器的动力学模型,并在此基础上对其动力学特性分析以及控制作了比较全面的研究.曲广吉^[32]和繆炳祺等^[33-34]通过求解系统的特征值问题或模态综合等方法,得到系统的全局模态,离散柔性部件的位移,由此基于伪坐标的拉格朗日方程导出了柔性航天器的动力学方程.

由此可见,建立系统低维高精度动力学模型的关键在于如何获取系统的全局模态. 近年来,曹登庆等针对微机电系统中的静电梳^[35]、大跨度斜拉桥^[36]、带非线性铰链的多梁结构^[37]、带柔性附件的航天器^[38]等细长柔性组合结构,建立了系统的动力学模型,获取了系统的全局模态.在此基础上,开展了密频与重频分析以及模态跃迁、系统动态响应分析等一系列研究.这些工作对于如何获取系统的全局模态,据此推导出系统的低维高精度动力学模型具有一定的借鉴和指导意义.

基于作者近期的工作^[35-38],本文研究多个刚体和柔体组成的复合柔性结构,建立了提取这类结构的解析形式的全局模态函数的一般方法. 采用解析形式的模态函数导出复合柔性结构的模态方程,利用Galerkin截断可以有效地降低系统的维数,得到系统的低维高精度非线性动力学模型. 基于该模型可以方便地构建系统的低维状态空间模型,有利于主动振动控制器的设计.

1 复合柔性结构全局模态解析提取方法

1.1 定义坐标系

采用笛卡尔坐标描述系统的运动,一般以系统中每一个物体为单元,在刚体、柔性部件和连接部件上建立坐标系,其上每个部件的位形都相对于一个公共参考系进行定义,其位形坐标统一为笛卡尔坐标.如图1所示,在系统中第i个物体和第j个物体的质心上分别建立各自的坐标系为$o_i xyz$和$o_j xyz$,坐标系$OXYZ$为公共参考系. 这样,物体上任意一点$P_i$和$P_j$的位置矢量分别为$\gamma _i$和$\gamma _j$.

图1

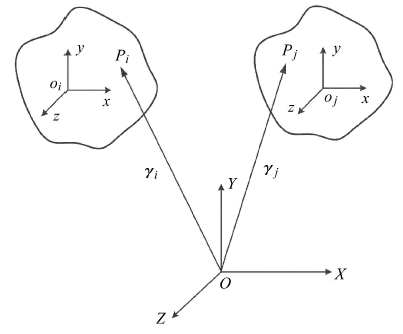新窗口打开|下载原图ZIP|生成PPT
图1基于笛卡尔坐标的运动描述

Fig.1Motion description based on Descartes coordinates

1.2 系统的运动方程

对于由多个刚体和柔体组成的复合柔性结构中的刚体,采用牛顿-欧拉法建立动力学方程. 在系统的g个刚体中取刚体$ A_i \ (i = 1,2, \cdots ,g)$为研究对象,它不仅受到系统构件相互之间的作用力和力矩,也可能受到外部的作用力和力矩.

如图2所示,只考虑系统的平面运动,在刚体$A_i$的质心上建立坐标系,并命名为$o_i^r xy$. 在连接点$S_{i,i-1}$处,相邻物体作用在刚体$A_i$的力$f_{x,{\rm int}}^{i,i-1}$和$f_{y, {\rm int}}^{i,i-1}$分别沿坐标系$o_i^r xy$的x方向和y方向,力矩为$M_{\rm int}^{i,i-1}$. 在连接点$ S_{i + 1,i}$处,相邻物体作用在刚体$A_i$的力$f_{x, {\rm int}}^{i + 1,i}$和$f_{y, {\rm int}}^{i+1,i}$分别沿坐标系$o_i^r xy$的x方向和y方向,力矩为$M_{\rm int}^{i + 1,i}$. 刚体$A_i$受到的外部作用力$f_{x, {\rm ext}}^i$和$f_{y, {\rm ext}}^i$分别沿坐标系$o_i^r xy$的x方向和y方向,外部力矩为$M_{{\rm ext}}^i$.因此,在连接点$S_{i,i-1}$和$S_{i + 1,i}$上,相邻物体作用在刚体$A_i$的总力矩为

(1)$ M_{i, {\rm int}} \; = M_{\rm int}^{i,i-1} + M_{\rm int}^{i + 1,i} + {\pmb d}_{i,i-1} \cdot \left( {f_{x, {\rm int}}^{i,i-1} {\pmb i} + f_{y, {\rm int}}^{i,i-1} {\pmb j}} \right) + \qquad {\pmb d}_{i +1,i} \cdot \left( {f_{x, {\rm int}}^{i + 1,i} {\pmb i} + f_{y, {\rm int}}^{i + 1,i} {\pmb j}} \right)$

图2

新窗口打开|下载原图ZIP|生成PPT
图2刚体所承受的作用力和力矩

Fig.2Forces and moments acted on the rigid body

其中,${\pmb d}_{i,i-1}$和${\pmb d}_{i+ 1,i}$分别是连接点$S_{i,i-1}$和$S_{i + 1,i}$的作用力到刚体$A_i$的质量中心的矢量,${\pmb i}$和${\pmb j}$分别为沿x方向和y方向的单位矢量. 刚体$A_i$受到外部作用的总力矩为

(2)$$ M_{i,{\rm ext}} = M_{{\rm ext}}^i + {\pmb d}_i \cdot \left( {f_{x, {\rm ext}}^i {\pmb i} + f_{y, {\rm ext}}^i {\pmb j} } \right)$$

其中,${\pmb d}_i$为刚体$ {A}_i$受到的外部作用力到其质量中心的矢量.

通过牛顿-欧拉法,可以写出单个刚体$A_i$的动力学方程

(3)$$ \left. \begin{array}{l} m_i \ddot {x}_i^r + f_{x, {\rm int}}^{i,i-1} + f_{x, {\rm int}}^{i+ 1,i} = f_{x, {\rm ext}}^i m_i \ddot {y}_i^r + f_{y, {\rm int}}^{i,i-1} + f_{y, {\rm int}}^{i + 1,i} = f_{y, {\rm ext}}^i J_i \ddot {\theta }_i^r + M_{i,{\rm int}} = M_{i, {\rm ext}} \end{array} \right\}$$

其中,$m_i$和$J_i$分别为刚体$A_i$的质量和关于质心$o_i^r$的转动惯量,$x_i^r$和$y_i^r$分别为刚体$A_i$沿坐标系$o_i^r xy$的x方向和y方向的坐标,$\theta _i^r$为刚体$A_i$的绕质心$o_i^r$转动角位移.因此,刚体$A_i$的平动和转动可以用向量${\pmb\gamma}_i^r = \left[ {x_i^r ,y_i^r ,\theta _i^r } \right]^{\rm T}$来描述.

在复合柔性结构中,柔体具有连续分布的质量和弹性,且有无限多个自由度,其动力学方程为偏微分方程. 为了求解系统的固有频率和全局模态,需要确定系统的边界条件和相邻物体之间的力、力矩、位移和转角的匹配条件.由图3描述的相邻两个柔体$B_i$和$B_{i + 1}$,它们通过${\pmb\gamma}_i^{\rm d}$向量连接在一起. 在柔体$B_i$和$B_{i + 1}$上分别建立浮动坐标系,并命名为$o_i^{\rm f} xy$和$o_{i + 1}^{\rm f} xy$. 在连接点$S_{i,i-1}$处,柔体$B_{i + 1}$作用在$B_i$的力和力矩分别为$f_{i,i-1}$和$M_{i,i-1}$. 同样地,在连接点$S_{i + 1,i}$处,柔体$B_i$作用在$B_{i + 1}$的力和力矩分别为$f_{i + 1,i}$和$M_{i + 1,i}$. 在柔体的连接点$S_{i,i-1}$和$S_{i + 1,i}$处,柔体的矢量${\pmb\gamma}_i^{\rm f}$和${\pmb\gamma}_{i + 1}^{\rm f}$必须满足约束条件

(4)$$ {\pmb\gamma}_i^{\rm f} + {\pmb\gamma}_i^{\rm d} ={\pmb\gamma}_{i + 1}^{\rm f}$$

图3

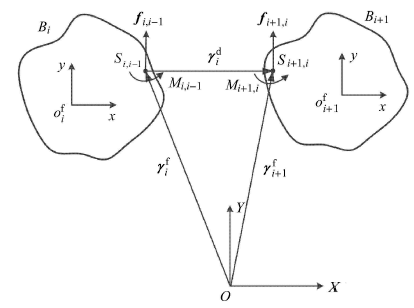新窗口打开|下载原图ZIP|生成PPT
图3刚性连接相邻柔体的约束关系

Fig.3Constraint relationship of rigid connecting deformable bodies

如图3所示,当两个柔体$B_i$和$B_{i + 1}$为刚性连接时,连接向量满足

(5)$$ {\pmb\gamma}_i^{\rm d} = {\bf 0}$$

亦即

(6)$$ {\pmb\gamma}_i^{\rm f} = {\pmb\gamma}_{i + 1}^{\rm f}$$

两个柔体$B_i$和$B_{i + 1}$相互之间的力和力矩也应满足关系式

(7)$$ {\pmb f}_{i,i-1} = {\pmb f}_{i + 1,i} , \ \ \ \ M_{i,i-1} = M_{i+ 1,i}$$

如图4所示,当两个柔体$B_i$和$B_{i + 1}$为弹性连接时,连接向量${\pmb\gamma}_i^{\rm d} \ne {\bf 0}$,且连接向量${\pmb\gamma}_i^{\rm d}$为弹性连接结构$C_i$的变形向量. 此时,弹性连接结构所受到的作用力$f_i$和力矩$M_i$可由自身的弹簧和线性阻尼构成的无质量系统来表达,即

(8)$$ {\pmb f}_i = \left[ \begin{array}{l} k_{i,1} x_i^{\rm d} + c_{i,1} \dot {x}_i^{\rm d} k_{i,2} y_i^{\rm d} + c_{i,2} \dot {y}_i^{\rm d} \end{array} \right] , \ \ M_i = k_{i,3} \theta _i^{\rm d} + c_{i,d} \dot {\theta }_i^{\rm d}$$

图4

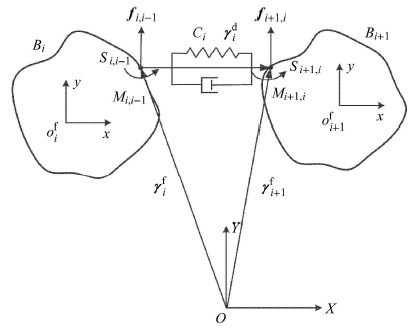新窗口打开|下载原图ZIP|生成PPT
图4弹性连接相邻柔体的约束关系

Fig.4Constraint relationship of elastic connection deformable bodies

其中,$x_i^{\rm d} ,y_i^{\rm d}$和$\theta _i^{\rm d}$分别为连接结构变形向量${\pmb\gamma}_i^{\rm d}$的水平位移,垂直位移和转动角位移,其相对应方向的弹簧刚度系数为$k_{i,1} ,k_{i,2}$和$k_{i,3}$,阻尼系数为$c_{i,1} ,c_{i,2}$和$c_{i,3}$. 连接结构的变形向量${\pmb\gamma}_i^{\rm d}$可以表示为${\pmb\gamma} _i^{\rm d} =\left[ {x_i^{\rm d} , y_i^{\rm d} ,\theta _i^{\rm d} } \right]^{\rm T}$.

两个柔体在连接点$S_{i,i-1}$和$S_{i + 1,i}$的矢量${\pmb\gamma} _i^{\rm f}$和${\pmb\gamma }_{i + 1}^{\rm f}$满足的约束条件为

(9)$$ {\pmb\gamma }_i^{\rm d} = {\pmb\gamma }_{i + 1}^{\rm f}-{\pmb\gamma }_i^{\rm f}$$

两个柔体$B_i$和$B_{i + 1}$相互之间的力和力矩也应满足关系式

(10)$$ {\pmb f}_{i,i-1} = {\pmb f}_i = {\pmb f}_{i + 1,i} , \ \ M_{i,i-1} =M_i = M_{i + 1,i}$$

为了便于理解,以欧拉——伯努利梁作为典型的柔体,推导复合柔性结构中单个柔体的动力学方程.如图5所示,在欧拉梁$B_i$的质心建立坐标系$o_i^f xy$,其点$o_i^f$固结在未变形前的梁$B_i$的中心,$B_i$的长度为$2l$. 梁$B_i$上任一点的变形向量为${\pmb u}_i$. 由于只考虑梁$B_i$在y方向上的运动,变形向量为${\pmb u}_i = \left[ {0, w_i } \right]$,$w_i$表示梁$B_i$上任一点在y方向上的位移. $B_i$上任一点的位置向量为自身的变形向量,可表示为

(11)$$ {\pmb\gamma}_i^{\rm f} = w_i (x,t) , \ \ \ x \in \left[ {-l,l} \right]$$

图5

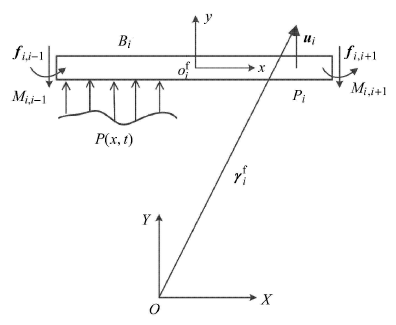新窗口打开|下载原图ZIP|生成PPT
图5欧拉——伯努利梁的运动描述

Fig.5Motion description of the Euler-Bernoulli beam

根据欧拉——伯努利梁理论,可以得到其运动微分方程为

(12)$$ EI{{w}"'}'_i + \rho \ddot {w}_i = p(x,t)$$

式中,$(')$表示对x求导数,梁的弹性模量为E,截面惯性矩为$I$,单位长度的质量为$\rho$,梁上各点的运动位移为, 作用在梁上沿长度方向的分布载荷为$p(x,t)$.

由图5所示,在$B_i$的连接点$S_{i,i-1}$和$S_{i,i + 1}$的位移分别为$w_i (-l,t)$和$w_i (l,t)$. 同样地,在$B_i$的连接点$S_{i,i-1}$和$S_{i,i + 1}$的转角分别为${w}'_i (-l,t)$和${w}'_i (l,t)$. 在$B_i$的连接点$S_{i,i-1}$和$S_{i,i + 1}$的剪力可表示为

(13)$$ f_{i,i-1} = EI{w}"'_i (-l,t) , \ \ \ \ f_{i,i + 1} = EI{w}"'_i (l,t)$$

在$B_i$的连接点$S_{i,i-1}$和$S_{i,i + 1}$的弯矩可表示为

(14)$$ M_{i,i-1} = EI{w}"_i (-l,t) , \ \ \ \ M_{i,i +1} = EI{w}"_i (l,t) $$

1.3 系统的固有频率和全局模态

对于由多个刚体、柔体和弹性连接结构组成的复合柔性结构,可以简化为g个刚体,$h$个柔体以及M个弹性连接结构.仅考虑系统的平面运动,由1.2节给出的系统中各物体的动力学方程,刚体$A_i (i = 1,2, \cdots ,g)$的平动和转动用向量${\pmb\gamma} _i^r$描述,弹性连接结构$C_j (j = 1,2, \cdots ,h)$在平动和转动方向上的变形用向量${\pmb\gamma}_j^{\rm d}$描述,柔体$B_k (k = 1,2, \cdots ,m)$上任一点的位移用向量${\pmb\gamma} _k^{\rm f}$ (即弹性变形向量${\pmb u}_k$)描述.考虑系统的自由振动,根据系统具有与时间无关的确定振型的特性,可用分离变量法推导系统的特征方程.同时定义系统的各广义坐标以同一频率作谐振动. 系统中刚体$A_i$,弹性连接结构$C_j$和柔体$B_k$的广义坐标特解形式为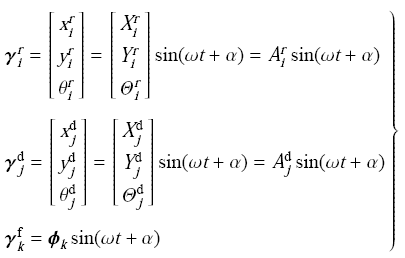

(15)

式中,$\omega$为系统的固有频率.

因为柔体是连续体,其变形是用偏微分方程来描述的,所以柔体$B_k$的振型矢量${\pmb\phi}_k$,是与空间位置有关的函数,根据柔体的类型不同,有其不同的形式.

如图5所示,当欧拉梁$B_k$在y方向上有位移时,其描述变形的偏微分方程如式(12)所示,那么描述欧拉梁$B_k$任一点的位置向量${\pmb\gamma} _k^{\rm f}$为自身的位移$w_k (x,t)$,其特解表示为

(16)$$ {\pmb\gamma} _k^{\rm f} = w_k (x,t) = \varphi _k (x)\sin (\omega t + \alpha )$$

将式(16)代入方程(12)的自由振动形式,可得

(17)$$ EI{\varphi }""_k (x)-\omega ^2\rho \varphi _k (x) = 0$$

式(17)的通解形式可写为

(18)$$\varphi _k (x) = A_k cos (\beta x) + B_k sin (\beta x) + C_k cosh (\beta x) + D_k sinh (\beta x) x \in [-l,l]$$

其中,$\beta = \left( {\frac{\rho \omega ^{2}}{EI}} \right)^{1 / 4}$. 令

(19)$$ {\pmb\psi}_k = \left[ {A_k ,B_k ,C_k ,D_k } \right]^{\rm T}$$

将式(18)代入梁$B_k$在连接点$S_{i,i-1}$和$S_{i,i + 1}$的位移、转角、剪力和弯矩的匹配条件,将式(15)表示刚体运动和连接结构变形的广义坐标特解分别代入刚体的动力学方程(3)和弹性连接结构的匹配条件(10)可得

(20)$$ {\pmb H}(\omega ){\pmb A} ={\bf 0}$$

为了求解系统的固有频率,需保证方程(20)有非零解,即矩阵${\pmb H}(\omega )$的行列式必须等于零.通过行列式等于零可以解出系统的固有频率,进而确定${\pmb A}$中各项系数.将关于描述柔体弹性变形的振型函数系数$\psi _1 ,\psi _2 , \cdots ,\psi _m$代入到各自的振型中,可以得到系统的全局模态.

1.4 系统动力学模型

与多自由度系统的振型叠加法类似,系统的各个物体的位移响应可以用$N$个模态叠加的形式近似表示. 系统中各个物体的第$s$阶模态振型可表示为

(21)$$ \left. \begin{array}{l} {\pmb A}_{i,s}^r = \left[ {X_{i,s}^r ,Y_{i,s}^r ,\varTheta _{i,s}^r } \right] , \ {\pmb A}_{j,s}^{\rm d} = \left[ {X_{j,s}^{\rm d} ,Y_{j,s}^{\rm d} ,\varTheta _{j,s}^{\rm d} } \right] \\ {\pmb \psi }_s = \left[ {A_{1,s}^r , \cdots ,A_{g,s}^r ,A_{1,s}^{\rm d} , \cdots ,A_{h,s}^{\rm d} ,\phi _{1,s} , \cdots ,\phi _{ m,s} } \right] \\ s = 1,2, \cdots ,N \end{array} \right\}$$

式中,${\pmb A}_{i,s}^r$和${\pmb A}_{j,s}^{\rm d}$分别为刚体$A_i$,弹性连接结构$C_j$的第$s$阶模态振型,${\pmb\phi} _{ k,s}$为柔体$B_k$的第$s$阶模态振型,${\pmb\psi}_s$为系统第$s$阶全局模态振型.

系统中各个物体的位移可以表示为

(22)$$ \left. \begin{array}{l} {\pmb \gamma}_1^r =\sum_{s = 1}^N {\pmb A}_{1,s}^r q_s (t) , \ {\pmb \gamma}_2^r =\sum _{s = 1}^N {\pmb A}_{2,s}^r q_s (t) , \cdots , \qquad {\pmb \gamma}_g^r =\sum _{s = 1}^N {\pmb A}_{g,s}^r q_s (t) {\pmb \gamma}_1^{\rm d}=\sum _{s = 1}^N {\pmb A}_{1,s}^{\rm d} q_s (t) , \ {\pmb \gamma}_2^{\rm d} =\sum _{s = 1}^N {\pmb A}_{2,s}^{\rm d} q_s (t) , \cdots , \qquad {\pmb \gamma}_h^{\rm d} =\sum _{s = 1}^N {\pmb A}_{h,s}^{\rm d} q_s (t) {\pmb u}_1 =\sum _{s = 1}^N {\phi _{1,s} q_s (t)} , \ {\pmb u}_2 =\sum _{s = 1}^N {\pmb\phi} _{2,s} q_s (t) , \cdots , \qquad {\pmb u}_m =\sum _{s = 1}^N {\pmb\phi} _{m,s} q_s (t) \end{array} \right\}$$

将上式代入刚体的运动微分方程(3),弹性连接结构的变形表达式(8),描述柔体弹性变形的偏微分方程(例如式(12)),然后将刚体的运动微分方程,描述柔体弹性变形的偏微分方程分别乘以自身的第$n$阶模态,再将描述柔体弹性变形的偏微分方程进行空间积分,并利用系统全局模态的正交性关系,可以得到系统的模态方程为

(23)$$ M_n \ddot {q}_n (t) + K_n q_n (t) = F_n (t) , \ \ \ n = 1,2, \cdots ,N$$

式中,$M_n$为第$n$阶模态质量,$K_n$为第$n$阶模态刚度,$F_n(t)$为系统外激励.

2 应用算例

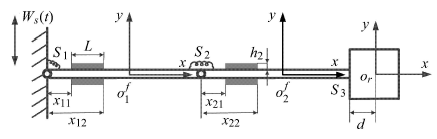
将图6所示的铰链连接双梁结构作为应用算例,图中的结构由两个欧拉——伯努利梁通过铰链连接而成,右端梁的自由端与刚体刚性连接.在该应用实例中,由于支撑平台移动,系统受到外部激励$w_s (t)$. 假定各个梁有着相同的截面和材料特性.同样地,各个铰链也是有着相同的几何参数和材料特性. 梁的长度为$2l$,宽度为$b$,厚度为$h_1$,单位长度的质量为$\rho$,弹性模量为E,截面惯性矩为$I$.分别在第i个梁上下表面粘贴一对压电片作为作动器,压电片的长度为$L$,厚度为$h_2$,宽度与梁相同,压电致动器在厚度方向(即图6所示y方向)分别施加输入电压为$U_i (t)$而产生的作动力矩$\hat {M}_i(x,t)$.$\theta _i$为描述第i个铰链的扭转变形的角位移.$m_r$和$J_r$分别为端部刚体的质量和关于质心$o_r$的转动惯量.d为连接点$S_{3}$到刚体质心$o_r$的距离. 令$o_i^{\rm f} xy$为第i个梁的坐标系,其坐标原点为梁的中心. 令$o_r xy$为端部刚体的坐标系,其坐标原点为刚体的质心.

图6

新窗口打开|下载原图ZIP|生成PPT
图6铰链连接的压电双梁结构

Fig.6Piezoelectric multi-beam structure connected with joints

2.1 系统的运动方程

压电作动器在输入电压的作用下对第i个梁产生的力矩为^[39]

(24)$$ \hat {M}_i (x,t) = CU_i (t)\left[ {H(x-x_{i1} )-H(x-x_{i2} )} \right] , \ \ i = 1,2$$

式中,$C = bpE(h_1 + h_2 )$,$x_{i2} = x_{i1} + L$,$p$为压电常数,$H(x)$为Heaviside阶跃函数.

由欧拉——伯努利梁理论,图6所示的第i个梁的运动微分方程为

(25)$$ \rho \ddot {w}_i + \xi _a \dot {w}_i + EI{{w}"'}'_i + \eta I \dot {{{w}"'}'}_i = \rho \ddot {w}_s (t) + \hat {{M}"}_i (x,t)$$

其中,$w_i$为第i个梁的垂向位移,$w_s (t)$为支撑平台的运动位移.$\xi _a$和$\eta$分别为梁的外阻尼和内阻尼系数,并且假定它们分别与其质量和刚度成比例关系,可以表示为

(26)$$ \xi _a = a\rho , \ \ \ \ \eta = bE$$

将式(24)代入方程(25)可得

(27)$$ \rho \ddot {w}_i + \xi _a \dot {w}_i + EI{{w}"'}'_i + \eta I \dot {{{w}"'}'}_i = \rho \ddot {w}_s (t) + \qquad CU_i (t)\left[ {H(x-x_{i1} )-H(x-x_{i2} )} \right] "$$

又因$H(x)$的广义导数为${H}'(x) = \delta (x)$,${H}"(x) = {\delta }'(x)$,由此可得

(28)$$ \rho \ddot {w}_i + \xi _a \dot {w}_i + EI{{w}"'}'_i + \eta I \dot {{{w}"'}'}_i = \rho \ddot {w}_s (t) + \qquad CU_i (t)\left[ {{\delta }'(x-x_{i1} )-{\delta }'(x-x_{i2} )} \right]$$

如图7所示,铰链作为弹性连接结构,可以简化为由非线性弹簧和线性阻尼构成的无质量的单自由度系统.基于参数识别方法,铰链传递的扭矩可由其状态来描述^[40]. 此时,第i个铰链传递扭矩的非线性表达式如下

(29)$$ M_i^T = c\dot {\theta }_i + k_L \theta _i + k_N \theta _i ^3 + \mu {\rm sign} \dot {\theta }_i$$

式中,$c,k_L ,k_N$和$\mu$分别为铰链的线性阻尼系数,线性刚度系数,三次非线性刚度系数和摩擦力矩系数.

图7

新窗口打开|下载原图ZIP|生成PPT
图7铰链模型

Fig.7The model of the joint

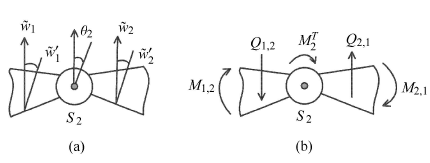
由图8(a)所示的铰链${S}_{2}$的几何条件,相对应的位移和转角可以表示为

(30)$$ \left. \begin{array}{l} \tilde {w}_1 = w_1 (l,t) , \ \ \tilde {w}_2 = w_2 (-l,t) {\tilde {w}}'_1 = {w}'_1 (l,t) , \ \ {\tilde {w}}'_2 = {w}'_2 (-l,t) \end{array} \right\}$$

图8

新窗口打开|下载原图ZIP|生成PPT
图8铰链${S}_{2}$的(a) 几何条件 (b) 自然条件

Fig.8(a) Geometric and (b) natural matching conditions at the joint${S}_{2}$

如图8(b)所示,$Q_{1,2}$和$Q_{2,1}$分别为铰链${S}_{2}$两边的剪力,$M_{1,2}$和$M_{2,1}$分别为铰链${S}_{2}$两边的弯矩,它们可以表示为

(31)$$ \left. \begin{array}{l} Q_{1,2} = EI{w}"'_1 (l,t) , \ \ Q_{2,1} = EI{w}"'_2 (-l,t) M_2^T = M_{1,2} = EI{w}"_1 (l,t) , \ \ M_2^T = M_{2,1} = EI{w}"_2 (-l,t) \end{array} \right\}$$

由图8所示的铰链匹配关系,根据柔体之间满足的位移和转角的匹配条件(9),力和力矩的匹配条件(10),由式(30)和式(31),可以得到铰链${S}_{2}$的匹配条件为

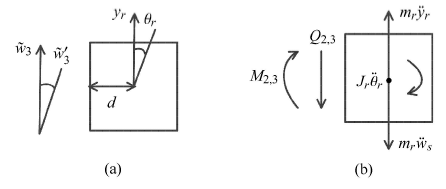
(32)$$ \left. \begin{array}{l} w_1 (l,t) = w_2 (-l,t) {w}'_1 (l,t) + \theta _2 = {w}'_2 (-l,t) EI{w}"'_1 (l,t) = EI{w}"'_2 (-l,t) EI{w}"_1 (l,t) = M_2^T = EI{w}"_2 (-l,t) \end{array} \right\}$$

如图9(b)所示,端部刚体左边受到连接处${S}_{3}$的力$Q_{2,3}$和力矩$M_{2,3}$,它们可以表示为

(33)$$ Q_{2,3} = EI{w}"'_2 (l,t) , \ \ M_{2,3} = EI{w}"_2 (l,t) $$

图9

新窗口打开|下载原图ZIP|生成PPT
图9刚体的(a) 几何条件 (b) 自然条件

Fig.9(a) Geometric and (b) natural matching conditions on the rigid body

由式(33),根据牛顿-欧拉法得到的刚体动力学方程(3),基于图9(b)所示的力和力矩的平衡关系,可以得到端部刚体平移和转动的运动方程为

(34)$m_r \ddot {y}_r + \xi _b \dot {y}_r-EI{w}"'_2 (l,t) = m_r \ddot {w}_s$

(35)$J_r \ddot {\theta }_r + \xi _c \dot {\theta }_r + EI{w}"_2 (l,t)-d EI{w}"'_2 (l,t) = 0$

式中,$\xi _b$和$\xi _c$分别为中心刚体在y方向上平移和绕质心$o_r$转动的外阻尼系数,它们分别表示为

(36)$$ \xi _b = a m_r , \ \ \ \ \xi _c = a J_r$$

如图9(a)所示,端部刚体所对应的位移和转角可以表示为

(37)$$ \tilde {w}_3 = w_2 (l,t) , \ \ \ \ {\tilde {w}}'_3 = {w}'_2 (l,t)$$

由式(37),通过它们之间的位移和转角的匹配关系,可以得到端部刚体的几何匹配条件

(38)$$ y_r = w_2 (l,t)-d\theta _r , \ \ \ \ \theta _r = {w}'_2 (l,t)$$

在铰链${S}_1$,系统的边界条件为

(39)$$ w_1 (-l,t) = 0 , \ \ {w}'_1 (-l,t) = \theta _1 , \ \ EI{w}"_1 (-l,t) = M_1^T$$

2.2 系统的固有频率和全局模态

采用分离变量法,求解系统特征值问题. 将系统的位移表示为

(40)$$ \left. \begin{array}{l} w_i (x,t) = \varphi _i (x)\sin (\omega t + \alpha ) \theta _i = \varTheta _i \sin (\omega t + \alpha ) y_r = Y_r \sin (\omega t + \alpha ) \theta _r = \varTheta _r \sin (\omega t + \alpha ) \end{array} \right\}$$

式中,$\omega$为系统的固有频率. 将式(40)代入梁的无阻尼自由振动方程(25)可得

(41)$$ EI{{\varphi }"'}'_i (x)-\omega ^2\rho \varphi _i (x) = 0 , \ \ i = 1,2$$

同样地,将式(40)代入系统中铰链${S}_{2}$的匹配条件(32)的线性表达式,得到

(42)$$ \left. \begin{array}{l} \varphi _1 (l) = \varphi _2 (-l) {\varphi }'_1 (l) + \varTheta _2 = {\varphi }'_2 (-l) EI{\varphi }"'_1 (l) = EI{\varphi }"'_2 (-l) EI{\varphi }"_1 (l) = k_L \varTheta _2 = EI{\varphi }"_2 (-l) \end{array} \right\}$$

将式(40)代入端部刚体平移和转动的无阻尼自由振动方程,得到

(43)$m_r \omega ^2Y_r + EI{\varphi }"'_2 (l) = 0$

(44)$J_r \omega ^2\varTheta _r-EI{\varphi }"_2 (l) + d EI{\varphi }"'_2 (l) = 0$

将式(40)代入端部刚体的几何匹配条件(38),得到

(45)$$ Y_r = \varphi _2 (l)-d\varTheta _r , \ \ \ \ \varTheta _r = {\varphi }'_2 (l)$$

将式(40)代入铰链$ {S}_1$的边界条件,得到

(46)$$ \varphi _1 (-l) = 0 , \ {\varphi }'_1 (-l) = \varTheta _1 , \ EI{\varphi }"_1 (-l) = k_L \varTheta_1$$

方程(41)的通解可以写为

(47)$$ \varphi _i (x) = A_i \cos (\beta x) + B_i \sin (\beta x) + C_i \cosh (\beta x) + \qquad D_i \sinh (\beta x) , \ \ x \in [-l,l]$$

其中$\beta = \left( {\frac{\rho \omega ^{2}}{EI}} \right)^{1 /4}$. 令

(48)$$ \left. \begin{array}{l} {\pmb \psi}_i = \left[ {A_i } \ \ {B_i } \ \ {C_i } \ \ {D_i } \right] {\pmb A}_r = \left[ {Y_r } \ \ {\Theta _r } \right] {\pmb A}_d = \left[ {\varTheta _1 } \ \ {\varTheta _2 } \right] {\pmb A} = \left[ {A_r } \ \ {A_d } \ \ {\psi _1 } \ \ {\psi _2 } \right]^{\rm T} \end{array} \right\}$$

将式(47)代入铰链${S}_{2}$的匹配条件(42),刚体平移和转动的无阻尼自由振动方程(43)和(44),端部刚体的几何匹配条件(45)和铰链${S}_1$的边界条件(46),可以得到

(49)$$ {\pmb H}(\omega ){\pmb A} = 0$$

其中,${\pmb H}(\omega ) \in {\bf R}^{12\times 12}$.为了求解系统的固有频率,需保证方程(49)具有非零解,即矩阵${\pmb H}(\omega )$的行列式必须等于零.通过行列式等于零可以解出系统的固有频率,进而确定A中各项系数. 将这些系数代入式(47),可以得到系统的全局模态.

2.3 系统全局模态的正交性

在一定的边界条件下,系统对应于任意两个不同的固有频率$\omega _j$和$\omega _k$的模态函数分别为${\pmb\phi} _{\;j} (x)$和${\pmb\phi}_{\;k} (x)$,即

(50)$$ {\pmb\phi}_{\;j} (x) = \left[ {Y_r^j } \ \ {\varTheta _r^j } \ \ {\varTheta _1^j } \ \ {\varTheta _2^j } \ \ {\varphi _1^j } \ \ {\varphi _2^j } \right]^{\rm T} , \ \ j = 1,2, \cdots$$

根据方程(41),(43)和(44),可以有

(51)$EI{{\varphi _i^j }^{\prime \prime \prime }}^\prime (x) = \omega _j^{ 2} \rho \varphi _i^j (x)$

(52)$-EI{\varphi _2^j }^{\prime \prime \prime }(l,t) = m_r \omega _j^2 Y_r^j$

(53)$EI{\varphi _2^j }^{\prime \prime }(l,t)-d EI{\varphi _2^j }^{\prime \prime \prime } (l,t) = J_r \omega _j^2 \varTheta _r^j$

方程(51)两边分别乘以$\varphi _i^k (x)$,加上所有方程,得到

(54)$$∫_{-l}^{l} EI \varphi _{1}^{j^{\prime \prime \prime \prime}} (x) \varphi _1^k (x) dx + ∫_{-l}^l {{EI\varphi _2^j}^{\prime \prime \prime }}^\prime (x)\varphi _2^k (x) d x = \qquad \omega _j^2 \left[ {∫_{-l}^l {\rho \varphi _1^j (x)\varphi _1^k } (x) d x + ∫_{-l}^l {\rho \varphi _2^j (x)\varphi _2^k (x)} d x} \right]$$

方程(52)和(53)两边分别乘以$Y_r^k$和$\varTheta _r^k$,再加上所有方程,可以得到

(55)$-EI{\varphi _2^j }^{\prime \prime \prime }(l)Y_r^k + \left[ {EI{\varphi _2^j }^{\prime \prime }(l)-d EI{\varphi _2^j }^{\prime \prime \prime } (l)} \right]\varTheta _r^k = \qquad \omega _j^2 \left[ {m_r Y_r^j Y_r^k + J_r \varTheta _r^j \varTheta _r^k } \right]$

对方程(54)部分积分,再加上方程(55),在系统的边界条件和匹配条件下,可以得到

(56)$$∫_{-l}^l EI \varphi _1^{j^{\prime \prime \prime \prime}} (x) \varphi _1^k (x) d x + ∫_{-l}^l EI \varphi _2^{j^{\prime \prime \prime \prime}} (x) \varphi _2^k (x) d x + \omega _j^2 [{m_r Y_r^j Y_r^k + J_r \Theta _r^j \Theta _r^k }] = ∫_{-l}^l EI \varphi _1^{j^{\prime \prime}}(x) \varphi _1^{k^{\prime \prime }} (x) d x + ∫_{-l}^l EI \varphi _2^{j^{\prime \prime }}(x) \varphi _2^{k^{\prime \prime }}(x) d x + k_L [ \Theta _1^j \Theta _1^k + \Theta _2^j \Theta _2^k]$$

方程(56)代入方程(54)的左边,可以得到

(57)$$\omega _j^2 \left[ {∫_{-l}^l {\rho \varphi _1^j (x)\varphi _1^k } (x) d x + ∫_{-l}^l {\rho \varphi _2^j (x)\varphi _2^k (x)} d x} \right] + \qquad \omega _j^2 \left[ {m_r Y_r^j Y_r^k + J_r \varTheta _r^j \varTheta _r^k } \right] = ∫_{-l}^l EI{\varphi _1^j }^{\prime \prime }(x){\varphi _1^k }^{\prime \prime } (x)d x + \qquad ∫_{-l}^l EI{\varphi _2^j }^{\prime \prime }(x){\varphi _2^k}^{\prime \prime }(x) d x + k_L \left[ {\varTheta _1^j \varTheta _1^k + \varTheta _2^j \varTheta _2^k } \right]$$

交换方程(57)的下标,可以得到

(58)$$\omega _k^2 \left[ {∫_{-l}^l \rho \varphi _1^j (x)\varphi _1^k (x)d x + ∫_{-l}^l \rho \varphi _2^j (x)\varphi _2^k (x) d x } \right]+ \qquad \omega _k^2 \left[ {m_r Y_r^j Y_r^k + J_r \varTheta _r^j \varTheta _r^k } \right] = ∫_{-l}^l {EI{\varphi _1^j }^{\prime \prime }(x){\varphi _1^k}^{\prime \prime }} (x) d x + \qquad ∫_{-l}^l EI{\varphi _2^j}^{\prime \prime }(x){\varphi _2^k}^{\prime \prime }(x) d x + k_L \left[ {\varTheta _1^j \varTheta _1^k + \varTheta _2^j \varTheta_2^k } \right]$$

方程(58)与方程(57)相减有

(59)$ \left( {\omega _j^2-\omega _k^2 } \right)\left[ {∫_{-l}^l {\rho \varphi _1^j (x)\varphi _1^k } (x) d x + ∫_{-l}^l {\rho \varphi _2^j (x)\varphi _2^k (x)} d x } \right] + \qquad \left( {\omega _j^2-\omega _k^2 } \right)\left[ {m_r Y_r^j Y_r^k + J_r \varTheta _r^j \varTheta _r^k } \right] = 0$

由方程(59),可记下列积分为

(60)$ ∫_{-l}^l {\rho \left[ {\varphi _1^k (x)} \right]^2} d x + ∫_{-l}^l {\rho \left[ {\varphi _2^k (x)} \right]^2} d x + \qquad m_r \left[ {Y_r^k } \right]^2 + J_r \left[ {\varTheta _r^k } \right]^2 = M_k$

式中,$M_k$为系统第k阶模态质量. 由方程(60)和方程(57),可记下列积分为

(61)$ ∫_{-l}^l EI\left[ {{\varphi _1^k} ^{\prime \prime }(x)} \right]^2 d x + ∫_{-l}^l EI\left[ {{\varphi _2^k }^{\prime \prime }(x)} \right]^2 d x + \qquad k_L \left[ {\left( {\varTheta _1^k } \right)^2 + \left( {\varTheta _2^k } \right)^2} \right] = K_k$

式中,$K_k$为系统第k阶模态刚度.

2.4 系统的状态空间模型

系统的位移可由全局模态乘以模态坐标的形式表示为

(62)$$ w_i (x,t) = \sum_{j = 1}^n {\varphi _i^j (x)q_j (t)} , \ \ \theta _i = \sum_{j = 1}^n {\varTheta _i^j q_j (t)} y_r = \sum_{j = 1}^n {Y_r^j q_j (t)} , \ \ \theta _r = \sum_{j = 1}^n {\varTheta _r^j q_j (t)}$$

其中,$q_j (t)$为系统的模态坐标.

将式(62)分别代入梁的运动微分方程(25),铰链传递扭矩的非线性表达式(29),端部刚体的运动方程(34)和(35),可以得到

(63)$ \sum_{j = 1}^n {\rho \varphi _i^j \ddot {q}_j } + \sum_{j = 1}^n {\xi _a \varphi _i^j \dot {q}_j } + \sum_{j = 1}^n {EI{{\varphi _i^j }^{\prime \prime \prime }}^\prime q_j } + \sum_{j = 1}^n {\eta I{{\varphi _i^j }^{\prime \prime \prime }}^\prime \dot {q}_j } = \qquad \rho \ddot {w}_s (t) + CU_i (t)\left[ {{\delta }'(x-x_{i1} )-{\delta }'(x-x_{i2} )} \right]$

(64)$ M_i^T = \sum_{j = 1}^n {c\varTheta _i^j \dot {q}_j } + \sum_{j = 1}^n {k_L \varTheta _i^j q_j } + \sum_{j = 1}^n {\mu _i {\rm sign}(\varTheta _i^j \dot {q}_j )} + \qquad \sum_{j = 1}^n {\sum_{k = 1}^n {\sum_{r = 1}^n {k_N \varTheta _i^j \varTheta _i^k \varTheta _i^r q_j } } q_k q_r }$

(65)$\sum_{j = 1}^n {m_r Y_r^j \ddot {q}_j } + \sum_{j = 1}^n {\xi _b Y_r^j \dot {q}_j }-\sum_{j = 1}^n {EI{\phi _2^j }^{\prime \prime \prime }(x)q_j } = m_r \ddot {w}_s$

(66)$ \sum_{j = 1}^n {J_r \varTheta _r^j \ddot {q}_j } + \sum_{j = 1}^n {\xi _c \varTheta _r^j \dot {q}_j } + \sum_{j = 1}^n {EI{\varphi _2^j }^{\prime \prime }(x)q_j }-d\sum_{j = 1}^n {EI{\varphi _2^j} ^{\prime \prime \prime }(x)q_j } = 0$

方程(63)的两边乘以$\phi _{i}^{ k}$,再沿梁的全长积分,方程(65)和(66)两边分别乘以$Y_r^k$和$\varTheta _r^k$,再加上所有方程,在系统的匹配条件和边界条件下,利用系统全局模态的正交性,可以得到

(67)$$\ddot {q}_k + 2\alpha _k \omega _k \dot {q}_k + \omega _k^{2} q_k + \gamma _k \dot {q}_j + e_k sign (\Theta _i^j \dot {q}_{j} ) + p_k q_j q_r q_s = f_k (t) + \hat {B}_k (t), k = 1,2 \cdots ,n$$

式中,$\alpha _k $为梁的模态阻尼,$\gamma _k$为铰链的阻尼,$e_k$为铰链的摩擦扭矩,方程(67)中各相关系数为

$$ \begin{array}{l} \alpha _k = {\left( {a / {\omega _k } + b \omega _k } \right)} / 2 ,\ \ \gamma _k = \frac{c}{M_k }\sum_{i = 1}^2 {\sum_{j = 1}^n {\Theta _i^j \Theta _i^k } } e_k = \frac{\mu }{M_k }\sum_{i = 1}^2 {\sum_{j = 1}^n {\Theta _i^k } } p_k = \frac{k_N }{M_k }\sum_{i = 1}^2 {\sum_{j = 1}^n {\sum_{r = 1}^n {\sum_{s = 1}^n {\varTheta _i^j \varTheta _i^r \varTheta _i^s \varTheta _i^k } } } } f_k (t) = \frac{\ddot {w}_s (t)}{M_k }\sum_{i = 1}^2 {\left[ {∫_{-l}^l {\rho \varphi _i^k } d x + m_r Y_r^k } \right]} \hat {B}_k (t) = \frac{C}{M_k }\sum_{i = 1}^2 {U_i } (t)\left[ {{\varphi }'_i (x_{i2} )-{\varphi }'_i (x_{i1} )} \right] \end{array} $$

引入状态向量

(68)$${\pmb x}(t) = \left[ {\pmb q}^{\rm T}(t) \ \ {\dot {\pmb q}^{\rm T}(t)} \right]^{\rm T} = \qquad [ {q_1(t) } \ \ {q_2 (t)} \ \cdots \ {q_n(t) } \ \ {\dot {q}_1(t) } \ \ {\dot {q}_2 (t)} \ \cdots \ {\dot {q}_n (t)} ]^{\rm T}$$

则系统的状态方程可表示为

(69)$$ \dot {\pmb x}(t) = {\pmb A}x(t) + {\pmb B}(t)$$

其中

(70)$$ \begin{array}{l} {\pmb A} = \left[ \begin{array}{cc} {\bf 0}_{n\times n} &{\pmb I}_{n\times n}-{\pmb \Omega}^2 &-2{\pmb \varDelta}-{\pmb C} \end{array} \right] {\pmb B} = \left[ {{\bf 0}_{1\times n} } \ \ {B_1 } \ \ {B_2 } \ \ \cdots \ \ {B_n } \right] {\pmb \Omega} = {\rm diag}\left[ {\omega _1 } \ \ {\omega _2 } \ \ \cdots \ \ {\omega _n } \right] {\pmb \varDelta} = {\rm diag}\left[ \begin{array}{cccc} {\alpha _1 \omega _1 } & {\alpha _2 \omega _2 } & \cdots & {\alpha _n \omega _n } \end{array} \right] \\ {\pmb C} = \left[ \begin{array}{cccc} {\gamma _{11} } & {\gamma _{12} } & \cdots & {\gamma _{1j} } {\gamma _{21} } & {\gamma _{22} } & \cdots & {\gamma _{2j} } \vdots & \vdots & \ddots & \vdots {\gamma _{k1} } & {\gamma _{k2} } & \cdots & {\gamma _{kj} } \end{array} \right] \gamma _{kj} = \sum_{i = 1}^2 {\varTheta _i^j \varTheta _i^k } B_k = f_k (t) + \hat {B}_k (t)-e_k {\rm sign}(\varTheta _i^j \dot {q}_{_j } )-p_k q_j q_r q_s \end{array}$$

式(67)为系统的非线性动力学方程组,其方程组的线性部分相互独立,非线性部分相互耦合,这样就使得方程组适用于解析方法来研究系统产生的非线性振动. 同时,基于该方法构建的低维状态空间模型(69),可以方便地进行振动控制器设计.

2.5 模态提取方法的有效性验证

为了验证本文提出的全局模态提取方法,这里以有限元方法得出的结果作为参考值,将本文方法给出的固有频率与采用有限元方法计算出的结果进行对比,以验证本文方法的有效性和正确性. 铰链连接的双梁结构的几何尺寸和材料参数见表1.

Table 1
表1
表1铰链连接的多梁结构的几何尺寸和材料参数
Table 1Geometric and material parameters of the multi-beam structure connected with joints

l/m	E/GPa	b/mm	h"mm
0.15	210	50	5
p/(kg.m^-1)	kL/(Nm.rad^-1)	mr /kg	J"(kg.m²)
1.962 5	1000	0.353 3	1.33 x 10^-4

新窗口打开|下载CSV

图10和图11分别给出了采用商业软件Ansys和本文解析方法得到的结构前八阶固有频率和振型. 定义采用两种方法得到的固有频率的相对误差为

(71)$$ e = \frac{\left| {\omega _i-\omega _i^{\rm ANS } } \right|}{\omega _i^{\rm ANS } }\times 100 % , \ \ i = 1,2, \cdots$$

式中,$\omega _i$和$\omega _i^{\rm ANS}$分别为由解析方法和Ansys得到的结构第i阶固有频率.

图10

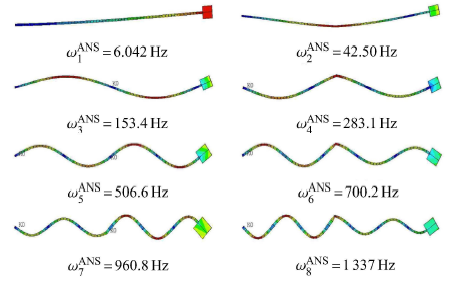新窗口打开|下载原图ZIP|生成PPT
图10ANSYS得到的结构前八阶固有频率和振型

Fig.10The first eight natural frequencies and mode shapes of the structure from Ansys

图11

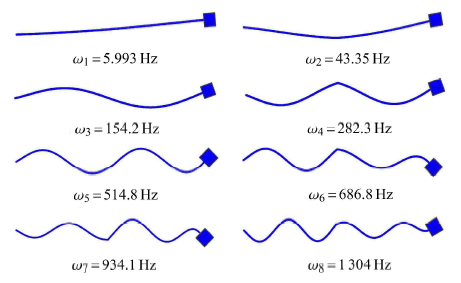新窗口打开|下载原图ZIP|生成PPT
图11解析方法得到的结构前八阶固有频率和振型

Fig.11The first eight natural frequencies and mode shapes of the structure from the analytic method

通过对图10和图11进行对比,可以看出由本文方法求解的固有频率与采用Ansys计算得到的固有频率吻合较好.在前八阶固有频率中,两种方法所得到结果的最大相对误差为$e = 2.7%$,表明本文提出的方法是有效的和正确的.

3 结论

针对复合柔性结构,提出了获取系统全局模态的一般性解析方法. 采用笛卡尔坐标描述系统的运动,给出了建立系统模态方程的策略和步骤. 通过描述柔性部件弹性变形的偏微分方程、描述刚体的运动微分方程以及描述连接界面力、力矩、位移和转角的匹配条件以及系统的边界条件,利用分离变量法给出了统一形式的频率方程,据此获取系统的固有频率和反映结构动力学特征的全局模态. 该方法具有以下优点：

(1) 所求解的系统全局模态是反映系统中各个部件弹性振动的真实模态.在此基础上,方便于分析参数对系统全局模态的影响,从而更深入地揭示系统中各个部件之间的振动耦合关系.

(2)由于所求解的全局模态为系统的真实模态,相比采用单个柔性部件的假设模态得到的动力学模型,基于全局模态方法得到的动力学模型,其精度更高、自由度更少.

(3)在考虑非线性因素的情况下,基于全局模态方法得到的系统非线性振动方程,其方程的线性部分相互独立,非线性部分相互耦合.这可以为采用解析方法求解复合柔性结构的非线性动态响应提供有效的低阶动力学模型.

(4) 基于解析形式的全局模态函数可以方便地构建系统的低维状态空间模型,有利于主动振动控制器的设计.

The authors have declared that no competing interests exist.

作者已声明无竞争性利益关系。

参考文献原文顺序
文献年度倒序
文中引用次数倒序
被引期刊影响因子

[1]

张军徽, 崔洋洋, 佟安 . 条带式太阳帆的结构动力学分析
力学学报, 2019,51(1):237-244

[本文引用: 1]

( Zhang

Junhui

, Cui

Yangyang

, Tong

. Structural dynamic and stability analysis of a stripped solar sail
Chinese Journal of Theoretical and Applied Mechanics, 2019,51(1):237-244 (in Chinese))

[本文引用: 1]

[2]

胡璐, 闫寒, 张文明等. 黏性流体环境下V 型悬臂梁结构流固耦合振动特性研究
力学学报, 2018,50(3):643-653