吴建营^,*,?,2), 陈万昕^?, 黄羽立^***华南理工大学亚热带建筑科学国家重点实验室, 广州 510641
^?华南理工大学土木工程系, 广州 510641
^**清华大学土木工程系, 北京 100084

COMPUTATIONAL MODELING OF SHRINKAGE INDUCED CRACKING IN EARLY-AGE CONCRETE BASED ON THE UNIFIED PHASE-FIELD THEORY¹⁾

Wu Jianying^,*,?,2), Chen Wanxin^?, Huang Yuli^***State Key Laboratory of Subtropical Building Science, South China University of Technology, Guangzhou 510641, China
^?Department of Civil Engineering, South China University of Technology, Guangzhou 510641, China
^**Department of Civil Engineering, Tsinghua University, Beijing 100084, China

通讯作者: 2)吴建营, 教授, 主要研究方向: 固体和结构损伤破坏力学. E-mail:jywu@scut.edu.cn

收稿日期:2021-01-14接受日期:2021-02-22网络出版日期:2021-05-19

基金资助:

1)国家自然科学基金.51878294 国家自然科学基金.51678246 土木工程防灾国家重点实验室开放课题.SLDRCE20-01

Received:2021-01-14Accepted:2021-02-22Online:2021-05-19
作者简介 About authors


摘要
受水化反应和热量传输等过程影响, 混凝土在养护阶段会发生受约束收缩变形, 并由此在结构内引发较大的拉应力, 而此时混凝土力学性能往往还处于较低水平, 容易导致建造期混凝土结构即出现裂缝等病害. 这种早龄期混凝土裂缝对核安全壳、桥梁隧道、地下结构、水工或海工结构等重大土木工程和基础设施的全生命周期完整性、耐久性和安全性造成严重影响. 为了准确预测早龄期混凝土抗裂性能并量化裂缝演化对混凝土结构行为的不利影响, 亟需开展化-热-力多场耦合环境下的混凝土裂缝建模与抗裂性能分析研究. 针对这一需求, 本工作在前期提出的固体结构损伤破坏统一相场理论基础上, 考虑开裂过程与水化反应、热量传输等之间的相互影响, 建立裂缝相场演化特征(包括基于强度的裂缝起裂准则、基于能量的裂缝扩展准则和基于变分原理的扩展方向判据等)与混凝土水化度和温度之间的定量联系, 提出混凝土化-热-力多场耦合相场内聚裂缝模型, 发展相应的多场有限元数值实现算法并应用于若干验证算例. 数值模拟结果表明, 上述多场耦合相场内聚裂缝模型合理地考虑了水化反应、热量传输、力学行为以及裂缝演化之间的耦合效应, 揭示了早龄期混凝土热膨胀变形和自收缩变形的相互竞争机理, 且分析结果不受裂缝尺度和网格大小等数值参数的影响, 实现了早龄期裂缝演化全过程准确模拟和抗裂性能定量预测, 有望在混凝土结构早龄期裂缝预测和控制方面发挥重要作用.
关键词： 混凝土;早龄期裂缝;多物理场;相场理论;相场内聚裂缝模型

Abstract
During curing of concrete, hydration and thermal transfer inevitably result in expansion and shrinkage and hence, large tensile stresses in early-age concrete structures. As the mechanical properties of young concrete are still very low, structures are vulnerable in the construction stage to defects induced by crack nucleation, propagation and evolution, severely threatening the integrity, durability and safety of concrete structures and infrastructures like nuclear containment vessels, bridges and tunnel linings, hydraulic and off-shore structures. In order to predict the fracture property of early-age concrete and quantify its adverse effects on structural performances, it is pressing to investigate the computational modeling of early-age cracking in concrete structures under the chemo-thermo-mechanically coupled environment. To the above end, in this work we propose a multi-physically coupled phase-field cohesive zone model within our previously established framework of the unified phase-field theory. The interactions between the crack phase-field with the hydration reaction and thermal transfer are accounted for, and the dependence of the characteristics of crack phase-field evolution, e.g., the strength-based nucleation criterion, the energy-based propagation criterion and the variational principle based crack path chooser, etc., on the hydration degree and/or temperature, are quantified. Moreover, the numerical implementation of the proposed model in the context of the multi-field finite element method is also addressed. Representative numerical examples indicate that, with the couplings among hydration, thermal transfer, mechanical deformations and cracking as well as the competition between thermal expansion and autogenous shrinkage both properly accounted for, the proposed multiphysical phase-field cohesive zone model is able to reproduce the overall cracking process and fracture property quantitatively. Remarkably, the numerical predictions are affected by neither the phase-field length scale nor the mesh discretization, ensuing its promising prospective in fracture control of early-age concrete structures.
Keywords：concrete;early-age cracking;multi-physics;phase-field theory;phase-field cohesive zone model


PDF (4207KB)元数据多维度评价相关文章导出EndNote|Ris|Bibtex收藏本文
本文引用格式
吴建营, 陈万昕, 黄羽立. 基于统一相场理论的早龄期混凝土化-热-力多场耦合裂缝模拟与抗裂性能预测¹⁾. 力学学报, 2021, 53(5): 1367-1382 DOI:10.6052/0459-1879-21-020
Wu Jianying, Chen Wanxin, Huang Yuli. COMPUTATIONAL MODELING OF SHRINKAGE INDUCED CRACKING IN EARLY-AGE CONCRETE BASED ON THE UNIFIED PHASE-FIELD THEORY¹⁾. Chinese Journal of Theoretical and Applied Mechanics, 2021, 53(5): 1367-1382 DOI:10.6052/0459-1879-21-020

引言

混凝土是全世界范围内应用最为广泛的土木工程材料, 在建筑结构、桥梁隧道、能源设施(水电大坝、核电安全壳、液化天然气或石油储罐、风电基础等)等土木工程和基础设施建设中发挥着重要作用. 然而, 一旦混凝土结构在建造期出现裂缝, 在服役期载荷和环境作用下这些早龄期裂缝将逐渐扩展和演化, 对结构全生命周期的完整性、耐久性和安全性造成严重影响. 因此, 准确预测早龄期混凝土的抗裂性能并由此控制混凝土结构建造质量, 是预防结构先天病患、保证结构优生健康的重要手段.

混凝土浇筑养护过程中, 水泥水化过程会释放热量使得混凝土升温, 热传导和热对流等机制将导致混凝土温度达到峰值后逐渐降低. 这一过程中, 与温度有关的热变形以及与水化度有关的自收缩变形会引发较大的拉应力, 而此时混凝土力学性能如强度等仍然处于较低水平, 导致早龄期混凝土开裂并逐渐扩展和演化^[1]. 上述过程涉及化学、热学、力学等复杂的多场耦合效应, 已有理论模型和数值方法尚不能定量预测早龄期混凝土的抗裂性能并准确描述早龄期裂缝的演化过程, 这也成为混凝土结构全寿命性能调控研究的主要难点问题之一.

早龄期混凝土裂缝理论模型和数值模拟方法的相关研究可以追溯至20世纪90年代. 根据所采用的混凝土裂缝模型, 大致可以分为以下4类:

(1) 经验或塑性力学模型. 早期研究大多采用塑性模型描述开裂后的早龄期混凝土性能, 如文献[2,3,4,5]等.

(2) 弥散裂缝模型. 这方面的代表性研究工作包括De Borst等^[6-8]提出的早龄期混凝土弥散裂缝模型.

(3) 损伤力学模型. 随着混凝土损伤力学的兴起, 若干****^[9-14]将部分经典的损伤模型推广至早龄期混凝土.

(4) 微细观力学模型. 为了考虑混凝土细观结构特别是粗骨料对早龄期混凝土抗裂性能的影响, 部分****发展了相应的微细观力学模型^[15-17].

上述工作为研究早龄期混凝土力学性能奠定了良好的基础. 然而, 这些研究大多采用较为简单的裂缝模型, 也未能考虑裂缝演化与水化反应、热量传输等过程之间的相互耦合, 自然也很难描述早龄期混凝土裂缝的演化过程并量化开裂后材料的力学性能.

近年来, 法国****Nguyen等^[18-20]采用脆性断裂相场模型描述开裂混凝土的力学行为, 并考虑裂缝与水化反应、热量传输之间的耦合效应, 能够较好地描述早龄期的裂缝演化过程. 脆性断裂相场模型根植于Francfort和Marigo^[21]提出的线弹性断裂能量变分原理, 解决了Griffith能量方法需要预设裂缝路径的难题; 由于将尖锐裂缝正则化为具有一定尺度的裂缝带, 并引入在[0, 1]之间连续分布的裂缝相场及其梯度描述裂缝状态, 脆性断裂相场模型非常便于通过有限元、无网格、物质点等多种数值方法加以实现. 可以严格证明^[22]: 当裂缝尺度趋近于零时, 脆性断裂相场模型$\varGamma$-收敛于Griffith线弹性断裂力学.

然而, 脆性断裂相场模型仅适用于脆性材料, 难以合理反映具有明显软化段的混凝土损伤破坏行为. 更糟糕的是, 模型的定量分析结果存在严重的裂缝尺度敏感性问题: 裂缝尺度越小, 结构起裂载荷和峰值载荷越大. 因此, 若将裂缝尺度视为数值参数, 则此类模型与Griffith线弹性断裂力学类似, 同样无法描述完好或仅有弱奇异性固体的裂缝起裂. 迫不得已, 研究人员只好将裂缝尺度视为材料属性^[23-25], 这一处理方式仍然无法完全解决裂缝起裂问题^[26-27].

近年来, 笔者将断裂力学和经典损伤力学有机结合, 建立了同时适用于脆性断裂和准脆性破坏的统一相场理论^[28]. 从该理论出发, 不仅经典的脆性断裂相场模型^[23,29]可以作为特例给出, 还在国际上首次提出了一类相场内聚裂缝模型PF-CZM^[30-32]. 理论分析证明: 当裂缝尺度趋近于零时, PF-CZM收敛为一类混合型破坏的内聚裂缝模型^[33-34], 能够准确预测线性软化以及指数软化、双曲软化、混凝土科内列森软化^[35]等非线性软化曲线. 该模型同时考虑了基于强度的裂缝起裂准则、基于能量的裂缝扩展准则以及基于变分原理的裂缝扩展方向判据, 仅需少量标准材料参数即可定量预测工程结构的损伤破坏行为; 特别是, 当裂缝尺度小于某一上限时, 其取值对脆性断裂和准脆性破坏的分析结果几乎不产生影响. 归功于上述优点, 统一相场理论和相场内聚裂缝模型一经提出, 迅速得到了国内外****的广泛认可和跟踪研究^[36-41].

基于上述考虑, 在统一相场理论框架内, 本工作拟进一步考虑裂缝相场与水化反应、热量传输等过程之间的双向耦合影响, 建立裂缝相场演化特征与混凝土水化度和温度之间的定量联系, 提出混凝土化$\!-\!$热$\!-\!$力多场耦合相场内聚裂缝模型, 发展相应的多场有限元数值实现算法, 并应用于早龄期混凝土的裂缝模拟和抗裂性能预测.

1 化$\!-\!$热$\!-\!$力多场耦合相场内聚裂缝模型

不失一般性, 考虑如图1所示内嵌裂缝/界面的固体$\varOmega \subset \mathbb{R}^{n_{\dim}}$ ($n_{\dim} = 1, 2, 3$), 其外边界记为$\partial \varOmega \subset \mathbb{R}^{n_{\dim} - 1}$, 外法向矢量记为$\boldsymbol{n}$. 虽然统一相场理论同样适用于有限变形^[42], 这里仅考虑小应变情况, 故可以通过位移场$\boldsymbol{u} ({x})$和线性应变场$\epsilon ({x}) := \nabla^{s} {u} ({x})$描述固体的变形状态, 这里${x}$为空间坐标, $\nabla^{s} (\cdot)$表示对称梯度算子. 为保证边值问题的适定性, 将外边界$\partial \varOmega$分成互不重叠的两部分$\partial \varOmega_{u}$和$\partial \varOmega_{t}$, 并分别施加给定的位移边界${u}^{\ast} ({x})$和力边界$\boldsymbol{t}^{\ast} ({x})$.

图1

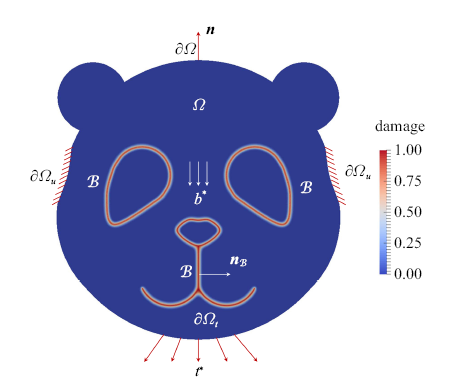新窗口打开|下载原图ZIP|生成PPT
图1固体内嵌裂缝/界面及其几何正则化^[34]

Fig.1A cracking solid and its geometric regularization^[34]



相场模型中, 尖锐裂缝/界面$\mathcal{S}$被弥散为如图1所示的裂缝带$\mathcal{B} \subseteq \varOmega$, 其尺度记为$b > 0$, 并引入相场变量$d ({x}) : \mathcal{B} \to [0, 1]$描述裂缝状态; 裂缝带$\mathcal{B}$的外边界记为$\partial \mathcal{B}$, 其外法向矢量表示为$\boldsymbol{n}_{\mathcal{B}}$. 需要指出的是, 在固体损伤破坏过程中, 裂缝带$\mathcal{B}$并非预先设定或保持固定, 而是遵循自身特定本构行为发生起裂、扩展和演化等过程. 类似于位移场, 裂缝相场也可以施加某种强迫边界条件: 例如, 对于弹性区域有$d ({x}) = 0$; 对于初始预设裂缝有$d ({x}) = 1$等.

1.1 混凝土开裂过程

首先介绍描述混凝土开裂行为的相场内聚裂缝模型, 包括裂缝相场$\!-\!$位移场耦合方程、应力$\!-\!$应变关系以及本构特征函数等. 需要指出的是, 早龄期混凝土的变形和力学性能与龄期或水化程度、温度等因素有关, 裂缝建模时必须合理地加以考虑.

1.1.1 裂缝相场$\!-\!$位移场耦合方程

统一相场理论^[28,32]中, 准静态条件下开裂固体的行为由如下耦合方程描述

(1a)$\left.\begin{array}{ll}\nabla \cdot \boldsymbol{\sigma}+\boldsymbol{b}^{*}=\mathbf{0}, & \text { in } \Omega \\\boldsymbol{\sigma} \cdot \boldsymbol{n}=\boldsymbol{t}^{*}, & \text { on } \partial \Omega\end{array}\right\}$
(1b)$\left.\begin{array}{ll}\nabla \cdot q+Q=0, & \text { in } \mathcal{B} \\q \cdot n_{B}=0, & \text { on } \partial \mathcal{B}\end{array}\right\}$
其中, $\boldsymbol{b}^{\ast}$为体积均布力, 裂缝相场通量$\boldsymbol{q}$和裂缝相场源$Q$分别表示为

(1c)$\begin{eqnarray}\label{eq:phase-field-sources} \boldsymbol{q} = \frac{2 b}{c_{\alpha}} G_{\rm f} \nabla d, \quad Q =-\omega' (d) \mathcal{H} - \alpha' (d) \dfrac{G_{\rm f}}{c_{\alpha} b}\end{eqnarray}$
式中, 应力$\sigma$和裂缝有效驱动力$\mathcal{H}$由下节的本构关系给出; 尺度$b > 0$为表征裂缝带宽度的数值参数, 其值越小, 裂缝带宽越小, 越接近尖锐裂缝; 裂缝几何函数$\alpha (d) \in [0, 1]$和能量退化函数$\omega (d) \in [0, 1]$分别为裂缝相场$d$的递增和递减函数, 并满足条件^[28,43]

(2a)$\alpha^{\prime}(d) \geqslant 0 ; \quad \alpha(0)=0, \alpha(1)=1$
(2b)$\omega^{\prime}(d) \leqslant 0 ; \quad \omega(0)=1, \omega(1)=0, \omega^{\prime}(1)=0$
这里, 引入了归一化参数$c_{\alpha} := 4 \int_{0}^{1} \sqrt{\alpha (\beta)} \; \beta$, 以保证固体完全破坏时单位裂缝面积的耗能为材料断裂能属性.

需要指出, 上述相场模型隶属于断裂力学或梯度损伤力学领域的Bourdin等^[29]变分相场断裂模型, 与物理学领域常用的Ginzburg-Landau相变方程^[44]、 Cahn-Hillard^[45]或Allan-Cahn^[46]相分离、晶体生长或多相流方程有明显区别, 虽然后者也曾经被美国****Karma等^[47]用于固体裂缝扩展分析.

变分相场断裂模型的理论基础来源于线弹性断裂力学中的Griffith能量原理^[48] 以及后续Francfort-Marigo提出的变分原理^[21], 并借鉴了图像分割理论中Mumford-Shah泛函^[49]的Ambrosio-Tortorelli椭圆正则化^[50]. 因此, 变分相场断裂模型通常满足$\varGamma$-收敛性质, 即: 当正则化尺度参数$b$趋近于零时, 其近似解在能量泛函意义上收敛于原尖锐裂缝问题的精确解, 具体可参见Braide^[51]和Bourdin等^[22]中的证明和论述.

除应用范围不同外, 以上变分相场断裂模型与Ginzburg-Landau方程主要有以下区别.

(1) Ginzburg-Landau方程中的裂缝几何函数通常采用双势阱函数$\alpha (d) = d^{2} (1 - d)^{2}$, 该函数满足性质$\alpha (0) = \alpha (1) = 0$, 即裂缝相场(或序参数)处于中间过渡状态$d \in (0, 1)$时的裂缝耗散比纯相$d = 0$或$d = 1$时更大, 这一特点与材料相变、多相流或结构拓扑优化等问题较为契合. 然而, 虽然双势阱函数也满足$\varGamma$-收敛特性, 但该函数在$[0, 1]$之间不具有单调递增性, 也无法区分$d = 0$和$d = 1$这两种截然不同的裂缝状态. 因此, 若将其作为裂缝几何函数, 将会导致裂缝面积和能量耗散随裂缝扩展呈现先增大后减小的趋势, 这与固体断裂时Griffith能量原理(即应变能持续减小、裂缝表面能单调增大、最终总能量达到最小值)相矛盾.

在断裂力学领域被广泛认可的变分相场断裂模型中, 其裂缝几何函数必须为裂缝相场(或序参数) $d$的单调递增函数, 且满足条件(2a), 这与裂缝扩展的不可逆特征一致, 其具体形式将在后文给出.

(2) Ginzburg-Landau方程通常会引入与裂缝相场(或序参数)一阶时间导数有关的松弛项或黏性项, 当松弛模量趋近于零(或黏性系数趋近无穷大)时, 方程退化为准静态式(1b)的形式. 因此, 此类模型先天具有一定的动力阻尼效应.

类似地, 对于动态裂缝扩展问题, 变分相场断裂模型中的控制方程(1b)$_{1}$(下标1指式(1b)中的第1式, 下同), 其等号右边也可以引入与裂缝相场一阶时间导数(裂缝扩展速率)有关的粘性阻尼项或(和)与裂缝相场二阶时间导数(裂缝扩展加速度)有关的惯性项, 以描述裂缝动态扩展时的应变率效应, 具体参见作者的相关工作^[52-53].这里仅考虑早龄期混凝土裂缝的准静态扩展过程, 因此并未引入阻尼项和惯性项.

需要指出的是, 这里所采用的相场内聚裂缝模型基于作者2017年提出的统一相场理论^[28], 虽也属于变分相场断裂范畴, 但与脆性断裂变分相场模型有本质不同: 归功于后文给出的本构特征函数, 相场内聚裂缝模型$\varGamma$-收敛于Barenblatt内聚裂缝模型^[54], 而非传统的Griffith线弹性断裂理论^[48]. 因此, 相场内聚裂缝模型不仅同时适用于脆性断裂, 也适用于粘聚断裂, 且模型预测给出的整体响应(包括裂缝路径和破坏模式等定性结果以及峰值载荷和软化段等定量结果)与裂缝正则化尺度无关.

1.1.2 应力$\!-\!$应变本构关系

为了考虑水化过程对混凝土变形的影响, 通常可以将总应变张量$\epsilon$分解为力学应变$\epsilon_{m}$、热膨胀应变$\epsilon_{t}$和自收缩应变$\epsilon_{a}$三部分之和的形式, 即

(3)$\begin{eqnarray} \epsilon = \epsilon_{m} + \epsilon_{t} + \epsilon_{a} \end{eqnarray}$
为简单起见, 热膨胀应变$\epsilon_{t}$和自收缩应变$\epsilon_{a}$均假定为二阶各向同性张量, 分别表示为

(4)$\begin{eqnarray} \epsilon_{t} = \eta_{t} \big( \theta - \theta_{0} \big) \boldsymbol{\mathit{1}}, \ \ \epsilon_{a} =-\eta_{a} \xi (\chi) \boldsymbol{\mathit{1}} \end{eqnarray}$
式中, $\boldsymbol{\mathit{1}}$为二阶单位张量; $\eta_{t}$为热膨胀系数, 表征与当前温度$\theta$和初始温度$\theta_{0}$之差有关的热膨胀应变; MacAuley括号定义为$\langle x \rangle := \max (x, 0)$; 当水化度$\chi$超过阈值$\chi_{0}$(本文统一取为$\chi_{0} = 0.05$)后, 混凝土出现自收缩变形, 其大小与系数$\eta_{a}$有关; $\xi (\chi)$为截断线性函数, 表示为^[55]

(5)$\begin{eqnarray}\label{eq5} \xi (\chi) = \Big\langle \dfrac{\chi - \chi_{0}}{\chi_{_\infty} - \chi_{0}} \Big\rangle, \quad \chi_{{\infty}} = 1 - \exp \Big(-3.3 \dfrac{w}{c} \Big) \end{eqnarray}$
其中, 混凝土最终水化度$\chi_{{\infty}}$与水灰比$w / c$之间服从上述指数关系^[56].

早龄期混凝土裂缝通常处于受拉张开状态, 因此可采用如下各向同性损伤本构关系

(6)$\begin{eqnarray}\label{eq6} \sigma = \omega (d) \bar{\sigma}, \ \ \mathcal{H} = \max_{n \in [0, T]} \big( \bar{Y}_{0}, \bar{Y}_{n} \big) \end{eqnarray}$
其中, 有效应力张量$\bar{\sigma}$、有效损伤能释放率$\bar{Y}$及其初始值$\bar{Y}_{0}$分别表示为

(7)$\begin{eqnarray}\label{eq7} \bar{\sigma} = {G}_{0} : \epsilon_{m}, \ \ \bar{Y} = \frac{1}{2 E_{0}} \langle \bar{\sigma}_{1} \rangle^{2},\ \ \bar{Y}_{0} = \frac{f_{\rm t}^{2}}{2 E_{0}} \end{eqnarray}$
式中${ G}_{0}$为弹性刚度张量, $E_{0}$为杨氏模量; $\bar{\sigma}_{1}$为有效应力张量$\bar{\sigma}$的最大主应力, $f_{\rm t}$为材料破坏强度. 由于龄期的影响, 这里杨氏模量和材料破坏强度均为水化度的递增函数, 详见1.2.2节.

1.1.3 本构特征函数

为了反映混凝土开裂引起的应变软化行为, 相场内聚裂缝模型采用如下裂缝几何函数和能量退化函数^[28,30-31]

(8)$\left.\begin{array}{l}\alpha(d)=2 d-2 d^{2} \Longrightarrow c_{\alpha}=\pi \\\omega(d)=\frac{(1-d)^{m}}{(1-d)^{m}+a_{1} d\left(1+a_{2} d+a_{3} d^{2}\right)}\end{array}\right\}$
模型参数$m \geqslant 2$以及$a_{1} > 0, a_{2}$和$a_{3}$分别确定为

(9a)$a_{1}=\frac{4}{\pi} \cdot \frac{l_{\mathrm{ch}}}{b}$
(9b)$a_{2}=2 \beta_{k}^{2 / 3}-\left(m+\frac{1}{2}\right) \geqslant-\frac{1}{2}$
(9c)$a_{3}=\left\{\begin{array}{ll}0, & m>2 \\\frac{1}{2} \beta_{w}^{2}-\left(1+a_{2}\right), & m=2\end{array}\right.$
式中, Irwin特征长度$l_{{\rm ch}} := E_{0} G_{\rm f} / f_{\rm t}^{2}$表征固体的脆性程度: 其值越小, 材料越脆; $\beta_{k}$和$\beta_{w}$分别表示为

(10)$\begin{eqnarray} \beta_{k} : = \dfrac{k_{0}}{-\dfrac{1}{2} f_{\rm t}^{2} / G_{\rm f}} \geqslant 1, \ \ \beta_{w} : = \dfrac{w_{c}}{2 G_{\rm f} / f_{\rm t}} \end{eqnarray}$
对于线性软化和混凝土常用的科内列森软化曲线^[35], 模型给出的软化曲线如图2所示; 其他如指数软化、双曲线软化等也可以类似给出, 见文献[57].

图2

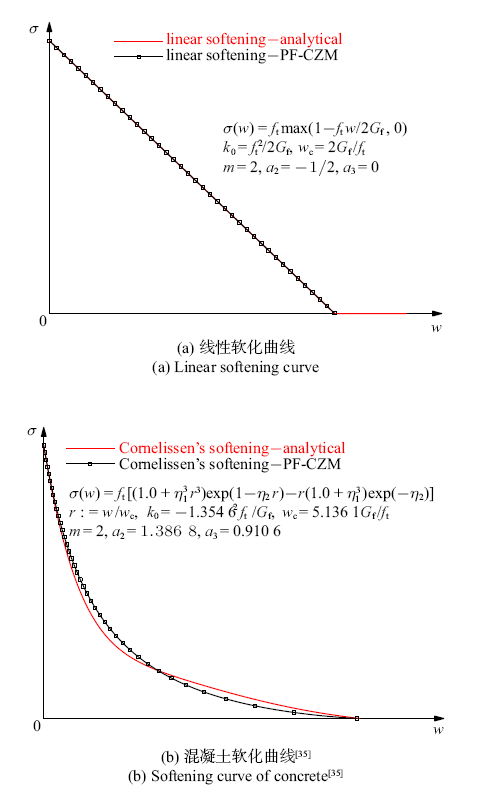新窗口打开|下载原图ZIP|生成PPT
图2相场内聚裂缝模型PF-CZM给出的软化曲线^[28,32]

Fig.2Softening curves given by the PF-CZM^[28,32]



前期研究表明^[58]: 裂缝尺度$b$的取值越小, 裂缝面积和相应能量耗散的计算精度就越高; 极限情况下, 裂缝尺度$b \to 0$时, 裂缝带宽度也趋近于零, 模型退化为一类混合型的内聚裂缝模型^[34]. 另一方面, 模型给出的应力$\!-\!$名义位移跳跃曲线$\sigma (w)$与裂缝尺度$b$无关, 而仅取决于弹性模量$E_{0}$、破坏强度$f_{\rm t}$、断裂能$G_{\rm f}$以及软化段参数(即初始斜率$k_{0}$、裂缝极限位移$w_{c}$)等材料属性. 因此, 对于裂缝几何函数和能量退化函数(8), 统一相场理论退化为一类相场内聚裂缝模型. 于是, 相场内聚裂缝模型不仅适用于各类准脆性破坏, 还可通过线性软化描述脆性断裂^[31], 实现了固体材料结构两种典型破坏特征的统一反映; 详见文献[28, 31-33]中的讨论.

1.2 混凝土水化过程

早龄期混凝土养护过程中, 水化反应会释放热量而导致材料升温膨胀; 随后, 传导和对流等热传输过程导致温度达到最高值后逐渐降低并引发收缩变形.

一方面, 裂缝会对混凝土水化反应和热传输过程产生影响; 另一方面, 混凝土力学性能也会随着龄期或水化度而发生变化, 共同构成了一类典型的化$\!-\!$热$\!-\!$力多场耦合问题, 如图3所示.

图3

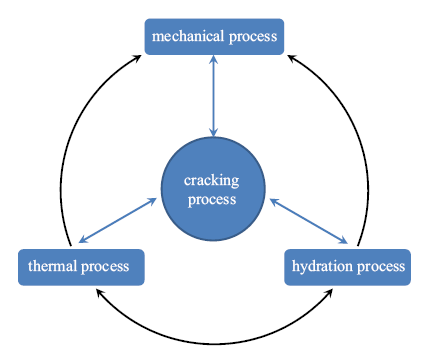新窗口打开|下载原图ZIP|生成PPT
图3早龄期混凝土开裂过程中的化$\!-\!$热$\!-\!$力多场耦合

Fig.3Multi-physical coupling in the cracking process of ear-age concrete



1.2.1 水化度场$\!-\!$温度场耦合控制方程

早龄期混凝土水化过程中, 化$\!-\!$热耦合问题由如下傅里叶定律和阿伦尼乌斯定律描述

(11a)$\left.\begin{array}{ll}\rho c \dot{\theta}+\nabla \cdot \boldsymbol{h}=\Theta, & \text { in } \Omega \\\Theta=p_{\infty} \dot{\chi}, & \text { in } \Omega \\\boldsymbol{h} \cdot \boldsymbol{n}=h^{*}+g^{*}\left(\theta-\theta_{a}\right), & \text { on } \partial \Omega_{h} \cup \partial \Omega_{c}\end{array}\right\}$
式中, 热流(通量)$\boldsymbol{h}$和水化速率$\dot{\chi}$分别表示为

(11b)$\left.\begin{array}{l}\boldsymbol{h}=-\boldsymbol{\kappa} \cdot \nabla \theta=-\omega(d) \boldsymbol{\kappa}_{0} \cdot \nabla \theta \\\dot{\chi}=\omega(d) \phi(\chi) \exp \left(-\frac{E_{a}}{R \cdot \theta}\right)\end{array}\right\}$
其中, $\rho c$为单位体积热容; $\varTheta$为水化反应所释放的热量, $p_{{\infty}}$为水化反应的潜热; 热传导系数假设为二阶各向同性张量且与水化度$\chi$无关, 即$\kappa_{0} = \kappa_{0} \boldsymbol{\mathit{1}}$, 其中$\kappa_{0}$为初始热传导系数; 边界$\partial \varOmega_{h}$上给定的热流记为$h^{\ast}$, 边界$\partial \varOmega_{c}$上与空气温度$\theta_{a}$有关的对流/辐射系数记为$g^{\ast}$, 剩余边界$\partial \varOmega_{\theta} := \partial \varOmega \backslash \varOmega_{h} \backslash \varOmega_{c}$的温度为给定值$\theta^{\ast}$; 活化能$E_{\rm a}$表征热生成速率, $R = 8.314$ J/(K $\cdot$ mol)为理想气体常数; 对于混凝土, 水化反应演化函数$\phi (\chi)$一般可由如下多项式函数拟合给出^[13] 系数$b_{i} \; (i = 0, 1, 2, \cdots, 6)$根据等温量热法试验数据拟合确定, 如图4所示.

(12)$\begin{eqnarray}&&\phi (\chi) = b_{0} + b_{1} \chi + b_{2} \chi^{2} + b_{3} \chi^{3} + \\&&\qquad b_{4} \chi^{4} + b_{5} \chi^{5} + b_{6} \chi^{6}\end{eqnarray}$

图4

新窗口打开|下载原图ZIP|生成PPT
图4典型混凝土水化反应演化函数^[13]

Fig.4Evolution function of the hydration reaction^[13]



由式(11b)可见, 早龄期裂缝演化会影响混凝土水化反应和热量传输过程.

1.2.2 混凝土龄期效应

随着龄期增加, 混凝土表现出明显的龄期效应, 即弹性模量$E_{0}$、破坏强度$f_{\rm t}$和断裂能$G_{\rm f}$均逐渐增大, 而泊松比$\nu_{0}$基本保持不变.

为简化起见, 这里假设早龄期混凝土开始形成弹性模量、破坏强度和断裂能的水化度阈值与自收缩应变的水化度阈值$\chi_{0}$取为相同值, 即考虑如下混凝土龄期效应^[4,59-60]

(13)$\left.\begin{array}{l}E_{0}(\chi)=E_{\infty} \xi(\chi) \\G_{\mathrm{f}}(\chi)=G_{\mathrm{f}_{\infty}} \xi(\chi) \\f_{\mathrm{t}}(\chi)=f_{\mathrm{t}_{\infty}} \xi(\chi)\end{array}\right\}$
式中, 函数$\xi (\chi)$由式(5)给出; $E_{{\infty}}$, $G_{{\rm f}_{\infty}}$和$f_{{\rm t}_{\infty}}$分别为水化度达到饱和$\chi_{{\infty}}$时的弹性模量、断裂能和抗拉强度.

上述混凝土力学性能的龄期效应也可以采用不同的水化度阈值或其他函数形式, 如文献[19]. 此时, 混凝土的特征长度$l_{{\rm ch}}$将与水化度$\chi$有关; 相应地, 相场内聚裂缝模型中, 参数$a_{1}$也将随之发生变化. 另一方面, 参数$a_{2}$和$a_{3}$仅与软化曲线类型有关, 不受材料力学参数变化的影响.

2 数值实现和求解算法

为便于有限元数值实现, 通常将化$\!-\!$热$\!-\!$力多场耦合控制方程(1)和式(11)表示为如下弱形式

(14)$\left.\begin{array}{l}\int_{\partial \Omega_{h} \cup \partial \Omega_{c}} \delta \theta\left(g \theta_{a}-h^{*}\right) \mathrm{d} A+\int_{\Omega} \delta \theta \cdot \Theta \mathrm{d} V- \\\int_{\Omega} \nabla \delta \theta \cdot \boldsymbol{k} \cdot \nabla \theta \mathrm{d} V-\int_{\Omega} \delta \theta \cdot \rho c \dot{\theta} \mathrm{d} V- \\\int_{\partial \Omega_{h}} \delta \theta \cdot g^{*} \theta \mathrm{d} A=0 \\\int_{\Omega} \delta \chi \Theta \mathrm{d} V-\int_{\Omega} \delta \chi\left(p_{\infty} \dot{\chi}\right) \mathrm{d} V=0 \\\int_{\mathcal{B}} \delta d \cdot Q \mathrm{~d} V-\int_{\mathcal{B}} \nabla \delta d \cdot \boldsymbol{q} \mathrm{d} V=0 \\\int_{\Omega} \delta \boldsymbol{u} \cdot \boldsymbol{b}^{*} \mathrm{~d} V+\int_{\partial \Omega_{t}} \delta \boldsymbol{u} \cdot \boldsymbol{t}^{*} \mathrm{~d} A- \\\int_{\Omega} \nabla^{\mathrm{s}} \delta \boldsymbol{u}: \boldsymbol{\sigma} \mathrm{d} V=0\end{array}\right\}$
式中, $ \delta \theta, \delta \chi, \delta \boldsymbol{u}, \delta d$分别为任意容许的节点温度、水化度、位移和裂缝相场. 与温度和位移条件类似, 裂缝相场$d ({x})$也可以施加诸如$d ({x} \in \varOmega \backslash \mathcal{B}) = 0$或$d ({x} \in \mathcal{S}_{0}) = 1$等强制边界条件, 这里$\mathcal{S}_{0}$为预制裂缝.

上述弱形式的化$\!-\!$热$\!-\!$力多场耦合问题可以采用多场有限元方法进行空间离散, 并选用合理的数值算法进行求解.

2.1 多场有限元数值实现

在进行有限元空间离散时, 可以将整个计算区域内所有单元节点均赋予温度、水化度、位移和裂缝相场自由度. 为提高计算效率, 也可以(不一定必要)将整个计算域$\varOmega$分为两部分^[58], 即: 可能出现裂缝的相场子区域$\mathcal{B}$和剩余部分$\varOmega \backslash \mathcal{B}$. 对于前者, 单元节点同时具有温度、水化度、位移和裂缝相场等自由度, 而后者的单元节点仅考虑温度、水化度和位移等自由度. 已有研究表明^{[28,30-31,57,61]}, 对于三角形常应变单元, 为保证计算精度, 相场子区域$\mathcal{B}$内的单元大小一般取为$h_{e} \leqslant b/5$; 四节点双线性单元, 其大小可适当放宽至$h_{e} \leqslant b/3$.

有限元空间离散后, 温度、水化度、裂缝相场和位移等场变量及其梯度分别表示为(数值实现时, 张量采用Voigt标记)

(15a)$\theta({x})=\tilde{\boldsymbol{N}} \tilde{\boldsymbol{a}}, \quad \nabla \theta({x})=\tilde{\boldsymbol{B}} \tilde{\boldsymbol{a}}$
(15b)$\chi({x})=\hat{\boldsymbol{N}} \hat{\boldsymbol{a}}, \quad \nabla \chi({x})=\hat{\boldsymbol{B}} \hat{\boldsymbol{a}}$
(15c)$d(x)=\bar{N} \bar{a}, \quad \nabla d(x)=\bar{B} \bar{a}$
(15d)$\boldsymbol{u}({x})=\boldsymbol{N a}, \quad \boldsymbol{\epsilon}({x})=\boldsymbol{B} a$
其中, $\tilde{{ a}}$, $\hat{{ a}}$, $\bar{{ a}}$和${ a}$分别为节点温度、水化度、裂缝相场和位移列向量, 插值函数分别记为$\tilde{{ N}}$, $\hat{{ N}}$, $\bar{{ N}}$和${ N}$, 协调矩阵(梯度算子)分别记为$\tilde{{ B}}$, $\hat{{ B}}$, $\bar{{ B}}$和${ B}$.

相应的, 由弱形式(14)可以给出如下平衡方程

(16)$\left.\begin{array}{rl}\tilde{r}=\tilde{f}^{\mathrm{ext}}+\int_{\Omega} \tilde{N}^{\mathrm{T}} \Theta \mathrm{d} V-\int_{\Omega} \tilde{\boldsymbol{N}}^{\mathrm{T}} \rho c \dot{\theta} \mathrm{d} V- \\\int_{\Omega} \tilde{\boldsymbol{B}}^{\mathrm{T}} \boldsymbol{\kappa} \nabla \theta \mathrm{d} V-\int_{\partial \Omega_{c}} \tilde{\boldsymbol{N}}^{\mathrm{T}} g^{*} \theta \mathrm{d} A=\mathbf{0} \\\hat{\boldsymbol{r}}=\int_{\Omega} \hat{\boldsymbol{N}}^{\mathrm{T}} \Theta \mathrm{d} V-\int_{\Omega} \hat{\boldsymbol{N}}^{\mathrm{T}} p_{\infty} \dot{\chi} \mathrm{d} V=\mathbf{0} \\\overline{\boldsymbol{r}}=\int_{\mathcal{B}} \overline{\boldsymbol{N}}^{\mathrm{T}} Q \mathrm{~d} V-\int_{\mathcal{B}} \overline{\boldsymbol{B}}^{\mathrm{T}} \boldsymbol{q} \mathrm{d} V=\mathbf{0} \\\boldsymbol{r}=f^{\mathrm{ext}}-\int_{\Omega} \boldsymbol{B}^{\mathrm{T}} \boldsymbol{\sigma} \mathrm{d} V=\mathbf{0}\end{array}\right\}$
式中, 列向量${ f}^{\text{ext}}$和$\tilde{{ f}}^{\text{ext}}$表示为

(17a)$\tilde{f}^{\operatorname{ext}}=\int_{\partial \Omega_{c}} \tilde{\boldsymbol{N}}^{\mathrm{T}} g^{*} \theta_{a} \mathrm{~d} A-\int_{\partial \Omega_{h}} \tilde{\boldsymbol{N}}^{\mathrm{T}} h^{*} \mathrm{~d} A$
(17b)$f^{\mathrm{ext}}=\int_{\Omega} N^{\mathrm{T}} \boldsymbol{b}^{*} \mathrm{~d} V+\int_{\partial \Omega_{t}} N^{\mathrm{T}} t^{*} \mathrm{~d} A$
方程组(16)通常采用增量迭代法进行求解, 即将时间步划分为$N$个子步. 对于某典型子步$[T_{n}, T_{n+1}]$, 其时间增量为$\Delta T := T_{n+1} - T_{n}$且$T_{n}$时刻所有的状态变量已知, 在此基础上采用迭代方法求解$T_{n+1}$时刻的状态变量.

2.2 控制方程的数值求解

考虑早龄期混凝土开裂过程的时间尺度较水化作用小得多, 上述多场耦合问题可以视为化$\!-\!$热耦合以及裂缝相场$\!-\!$位移耦合两个串行过程, 分别进行求解后判断是否收敛; 如不收敛, 则重新进行迭代. 上述数值求解过程如流程图5所示.

图5

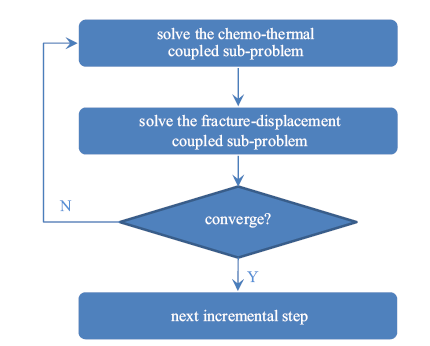新窗口打开|下载原图ZIP|生成PPT
图5化$\!-\!$热$\!-\!$力多场耦合相场内聚裂缝模型数值求解流程图

Fig.5Flow chart in solving chemo-thermo-mechanical coupled problems



2.2.1 水化过程

对于水化过程, 采用后退欧拉法进行时间离散

(18)$\begin{eqnarray}\label{eq18} \dot{\tilde{{ a}}} = \dfrac{\tilde{{ a}} - \tilde{{ a}}_{n}}{\Delta T}, \ \ \dot{\hat{{ a}}} = \dfrac{\hat{{ a}} - \hat{{ a}}_{n}}{\Delta T} \end{eqnarray}$
下标$n$标识上一增量步结束后的状态变量.

水化过程涉及的裂缝相场均采用上一增量步结束后的$d_{n}$. 由此, 化$\!-\!$热耦合方程(16)$_{1}$和(16)$_{2}$可采用牛顿迭代方法进行求解, 其线性化形式表示为

(19)$\begin{eqnarray}\label{eq19} \begin{bmatrix} { K}_{{\theta \theta}} & { K}_{{\theta \chi}} \\ { K}_{{\chi \theta}} & { K}_{{\chi \chi}} \\ \end{bmatrix} \begin{Bmatrix} \delta \tilde{{ a}} \\ \delta \hat{{ a}} \\ \end{Bmatrix} = \begin{bmatrix} \tilde{{ r}} \\ \hat{{ r}} \\ \end{bmatrix} \end{eqnarray}$
式中, 子刚度矩阵分别表示为

(20a)$\begin{aligned}\boldsymbol{K}_{\theta \theta}=& \int_{\Omega} \tilde{\boldsymbol{N}}^{\mathrm{T}} \frac{\rho c}{\Delta T} \tilde{\boldsymbol{N}} \mathrm{d} V+\int_{\Omega} \tilde{\boldsymbol{B}}^{\mathrm{T}} \boldsymbol{\kappa} \tilde{\boldsymbol{B}} \mathrm{d} V+\int_{\partial \Omega_{c}} \tilde{\boldsymbol{N}}^{\mathrm{T}} g \tilde{\boldsymbol{N}} \mathrm{d} A\end{aligned}$
(20b)$\boldsymbol{K}_{\theta \chi}=-\int_{\Omega} \tilde{\boldsymbol{N}}^{\mathrm{T}} \frac{\partial \Theta}{\partial \chi} \hat{\boldsymbol{N}} \mathrm{d} V$
(20c)$\boldsymbol{K}_{\chi \theta}=-\int_{\Omega} \hat{\boldsymbol{N}}^{\mathrm{T}} \frac{\partial \Theta}{\partial \theta} \tilde{\boldsymbol{N}} \mathrm{d} V$
(20d)$\boldsymbol{K}_{\chi \chi}=\int_{\Omega} \hat{\boldsymbol{N}}^{\mathrm{T}}\left(\frac{p_{\infty}}{\Delta T}-\frac{\partial \Theta}{\partial \chi}\right) \hat{\boldsymbol{N}} \mathrm{d} V$
上述刚度矩阵不对称, 这是耦合问题的特点之一.

2.2.2 开裂过程

对于早龄期混凝土开裂和演化过程, 有效应力$\bar{\sigma}$需考虑热膨胀应变$\epsilon_{t}$和自收缩应变$\epsilon_{a}$, 这些状态变量可通过水化过程求解得到的温度和水化度计算给出. 相应的, 裂缝相场$\!-\!$位移场耦合控制方程的求解与纯力学问题基本相同.

在求解耦合方程(16)$_{3}$和(16)$_{4}$时, 整体牛顿迭代或子问题交错迭代等算法存在收敛性差、计算速度慢等问题. 近年来, 笔者^[62-63]首次将整体BFGS拟牛顿迭代算法^[64]应用于这一耦合方程的求解, 在保证算法收敛性的同时, 收敛速度和计算效率大幅度提升. 这里对该算法做简要介绍.

在整体BFGS拟牛顿算法中, 对于非线性方程组${ f} ({ z}) = {\ 0}$, 其状态变量${ z}$按如下格式进行更新

(21)$\begin{eqnarray} { z} = { z}^{(k-1)} + s \delta { z}, \ \delta { z}= \tilde{{ K}}^{-1} \; \delta { f} \end{eqnarray}$
上标$k - 1$表示前一迭代步结束后的状态变量; $\delta { f} := { f} - { f}^{(k-1)}$ 和$\delta { z} := { z} - { z}^{(k-1)}$分别为当前迭代步结束后的残量和修正量; 柔度矩阵$\tilde{{ K}}^{-1}$按下式进行计算

(22a)$\tilde{\boldsymbol{K}}^{-1}=\left(\boldsymbol{I}-\boldsymbol{A}_{1}\right)\left(\tilde{\boldsymbol{K}}^{(k-1)}\right)^{-1}\left(\boldsymbol{I}-\boldsymbol{A}_{1}\right)^{\mathrm{T}}+\boldsymbol{A}_{2}$
(22b)$\boldsymbol{A}_{1}=\frac{\delta \boldsymbol{z} \delta \boldsymbol{f}^{\mathrm{T}}}{\delta \boldsymbol{z}^{\mathrm{T}} \delta \boldsymbol{f}}, \boldsymbol{A}_{2}=\frac{\delta \boldsymbol{z} \delta \boldsymbol{z}^{\mathrm{T}}}{\delta \boldsymbol{z}^{\mathrm{T}} \delta \boldsymbol{f}}$
式中, 缩放系数$s$由线性搜索方法确定^[64].

可以证明^[65], 若初始刚度矩阵$\tilde{{ K}}^{(0)}$对称且正定, 则更新后的矩阵$\tilde{{ K}}$同样如此. 此时, 虽然初始刚度矩阵$\tilde{{ K}}^{(0)}$不影响收敛性, 但对于收敛速度有一定的影响. 笔者建议采用如下对称、正定的初始刚度$\tilde{{ K}}^{(0)}$

(23)$\begin{eqnarray}\label{eq23} \tilde{{ K}}^{(0)} = \begin{bmatrix} { K}_{{d d}} & { K}_{{d u}} \\ { K}_{{d u}}^{\text{T}} & { K}_{{u u}} \\ \end{bmatrix} \end{eqnarray}$
其中, 子刚度矩阵分别表示为

(24a)$\boldsymbol{K}_{d d}=\int_{\mathcal{B}}\left[\overline{\boldsymbol{N}}^{\mathrm{T}}\left(-\frac{\partial Q}{\partial d}\right) \overline{\boldsymbol{N}}+\frac{2 b}{c_{\alpha}} G_{\mathrm{f}} \overline{\boldsymbol{B}}^{\mathrm{T}} \overline{\boldsymbol{B}}\right] \mathrm{d} V$
(24b)$\boldsymbol{K}_{u u}=\int_{\Omega} \boldsymbol{B}^{\mathrm{T}}\left(\frac{\partial \boldsymbol{\sigma}}{\partial \boldsymbol{\epsilon}}\right) \boldsymbol{B} \mathrm{d} V$
(24c)$\boldsymbol{K}_{d u}=\int_{\mathcal{B}} \overline{\boldsymbol{N}}^{\mathrm{T}}\left(\vartheta \omega^{\prime} \frac{\partial \mathcal{H}}{\partial \boldsymbol{\epsilon}}\right) \boldsymbol{B} \mathrm{d} V$
对于式(7)给出的裂缝相场驱动力$\bar{Y}$, 为保证初始刚度(23)的正定性, 系数$\vartheta$确定为

(25)$\begin{eqnarray} 0 \leqslant\vartheta \leqslant\vartheta_{\max} =-\dfrac{1}{\langle \bar{\sigma}_{1} \rangle \omega'} \sqrt{ -\dfrac{\partial Q}{\partial d} \dfrac{1 + \nu_{0}}{1 + \bar{\nu}_{0}} \omega E_{0}} \end{eqnarray}$
式中, $\bar{\nu}_{0}$与应力状态和材料泊松比$\nu_{0}$有关, 即: 单轴应力条件下$\bar{\nu}_{0} = \nu_{0}$, 平面应力条件下$\bar{\nu}_{0} = \nu_{0} / (1 - \nu_{0})$, 其它情况下$\bar{\nu}_{0} = \nu_{0} / (1 - 2 \nu_{0})$. 实际应用时, 通常采用$\vartheta = 0.95 \vartheta_{\max}$.

整体BFGS拟牛顿算法可以根据需要设置一定迭代次数($5 \sim 10$次)间隔后才对刚度矩阵$\tilde{{ K}}$进行更新计算. 因此, 虽然所需迭代次数可能比牛顿迭代算法更多(后者不一定能收敛), 但BFGS算法计算过程较为简单且无需每次迭代都更新刚度矩阵, 计算效率通常更高. 较之子问题交错迭代算法, 整体BFGS拟牛顿算法的求解效率提高5$\sim$8倍^{[62-63,66-67]}.

3 应用算例

针对上述化$\!-\!$热$\!-\!$力多场耦合相场内聚裂缝模型, 本节给出其在早龄期混凝土裂缝演化模拟和抗裂性能预测的验证算例. 对于混凝土, 采用科内列森软化曲线. 这里, 二维问题均假定为平面应力状态, 网格划分采用四节点双线性插值单元.

由于水化反应过程的控制方程(11)涉及时间导数, 在求解过程中按照式(18)进行时间离散并需要给定节点温度和节点水化度的初始值. 算例1中, 节点温度的初始值取为室温20$^{\circ}$C, 算例2中, 节点温度的初始值取为室温21$^{\circ}$C; 算例1和算例2中, 节点水化度的初始值均取为0.

3.1 带方形孔早龄期混凝土圆板收缩开裂^[18]

首先考虑如图6所示的早龄期混凝土圆形板, 其外直径为0.5 m, 方形孔的尺寸为0.4 m. 材料参数由文献[13, 18]给出(详见表1); 所采用混凝土水化反应演化函数如图4所示. 由于模型具有对称性, 建模时仅考虑右上角1/4模型, 并分别在模型左侧和底部施加$X$向和$Y$向位移约束.

图6

新窗口打开|下载原图ZIP|生成PPT
图6带方形孔圆板收缩开裂: 试件几何尺寸、边界和环境条件^[18]

Fig.6A circular plate with a square hole: Specimen geometry, boundary and environment conditions^[18]



Table 1
表1
表1带方形孔早龄期混凝土圆形板模型参数取值
Table 1Model parameters for the cracking analysis of an early-age circular concrete?plate with a square hole

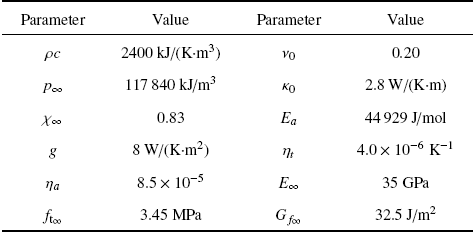新窗口打开|下载CSV

首先考虑裂缝尺度对数值模拟结果的影响. 模拟中, 采用$b = 5$ mm和$b = 10$ mm两种裂缝尺度, 相应的单元大小取为$h_{\rm e} = b/5$. 模拟给出典型时刻的裂缝相场云图如图7所示. 可以看出, 裂缝尺度$b$的取值大小仅改变损伤带的宽度, 而对裂缝路径和破坏模式几乎没有任何影响.

图7

新窗口打开|下载原图ZIP|生成PPT
图7带方形孔圆板收缩开裂: 不同时刻的裂缝相场云图(上排: $b = 10$ mm; 下排: $b = 5$ mm)

Fig.7A circular plate with a square hole: Predicted damage profiles at various instants (top: $b = 10$ mm; bottom: $b = 5$ mm)



图8给出了两种尺度参数情况下圆形板内、外边缘和中间位置处混凝土的温度、水化度、弹性模量、破坏强度以及断裂能随养护龄期的演化曲线. 同样, 相场尺度参数$b$不影响上述定量计算结果.

图8

新窗口打开|下载原图ZIP|生成PPT
图8带方形孔圆板收缩开裂: 不同位置处温度、水化度和典型力学参数随时间的演化曲线

Fig.8A circular plate with a square hole: Predicted evolution curves of the temperatures, hydration degrees and mechanical properties



对于$b = 5$mm和$h_{\rm e} = 1$ mm情况, 图9中给出了不同时刻的裂缝相场、温度场和水化度场云图. 可以看出, 早龄期混凝土的裂缝演化过程主要分为以下3个阶段:

图9

新窗口打开|下载原图ZIP|生成PPT
图9带方形孔圆板收缩开裂: 不同时刻的裂缝相场、温度场和水化度场云图

Fig.9A circular plate with a square hole: Predicted evolution curves of the temperatures and hydration degrees at various instants



(1) 时间段[0 h, 16 h]: 水化加速和裂缝起裂阶段. 该阶段混凝土水化度快速增大, 水化反应释放的热量超过周边环境对流和热传导引起的热量损失, 导致混凝土温度升高, 方孔边缘处混凝土的最高温度可达35.3$^{\circ}$C. 热膨胀变形引起方形孔顶点处应力集中, 裂缝起裂首先出现此处.

(2) 时间段[22 h, 80 h]: 水化减速和裂缝缓慢发展阶段. 该阶段水化速率降低, 释放的热量小于环境对流和热传导引起的热量损失, 导致混凝土温度降低, 材料逐渐从热膨胀变形转变为收缩变形. 由于水化作用, 混凝土获得了70%的破坏强度, 足以抵抗热膨胀和自收缩引起的裂缝扩展, 该阶段裂缝发展缓慢.

(3) 时间段[80 h, $+\infty$]: 水化减速和裂缝迅速扩展并贯穿阶段. 该阶段, 自收缩应变进一步增大, 方形孔顶点位置处的拉应力逐渐增加并超过破坏强度, 致使裂缝迅速扩展并最终贯穿整个试件, 应力得到释放. 最后, 混凝土获得了80%左右的破坏强度, 其他部位不再有裂缝进一步发展.

可以看出, 在不同阶段, 混凝土热膨胀变形和自收缩变形两种机制相互竞争, 并对早龄期混凝土的应力发展和抗裂性能产生显著影响.

与纯力学载荷作用下的分析结果类似, 化$\!-\!$热$\!-\!$力多场耦合相场内聚裂缝模型的数值模拟结果不受裂缝尺度和网格大小影响, 能够合理描述早龄期混凝土水化反应和热量传输耦合作用下复杂的开裂过程, 特别是热膨胀变形和自收缩变形这两类相互竞争的物理机制得到了准确反映.

3.2 受约束圆环试验收缩开裂试验

考虑如图10所示的受约束圆环收缩开裂试验^[68].

图10

新窗口打开|下载原图ZIP|生成PPT
图10约束混凝土圆环试验: 试件几何尺寸和环境条件^[68]

Fig.10Restrained concrete ring test: Specimen geometry and environment conditions^[68]



混凝土圆环试件的厚度为75 mm, 内直径为150 mm, 外直径为300 mm. 试验中考虑了3.1 mm, 9.5 mm和19.0 mm 3种厚度的约束钢环(其中, 3.1 mm厚度圆环试件由于刚度较小、约束效应较低, 混凝土试件并未开裂, 故这里仅考虑后两种厚度情况), 并通过贴在钢环中部位置的4个应变片监测钢环应变. 所采用的材料(水灰比$w/c = 0.3$的砂浆)和模型参数见表2; 混凝土水化反应演化函数如图11所示.

Table 2
表2
表2受约束圆环试验收缩开裂试验参数取值
Table 2Model parameters for the cracking analysis of a constrained concrete ring

新窗口打开|下载CSV

图11

新窗口打开|下载原图ZIP|生成PPT
图11约束混凝土圆环试验: 混凝土水化反应演化函数

Fig.11Restrained concrete ring test: Evolution function of the hydration reaction



类似地, 为考察裂缝尺度和网格大小的影响, 数值模拟采用两种裂缝尺度$b = 2.5$mm和$b = 5.0$mm, 相应的单元网格大小取为$h_{{e}} = b/5$.

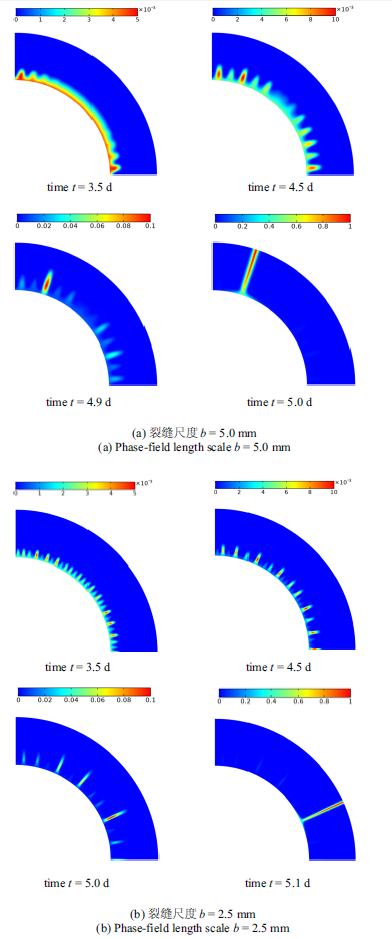
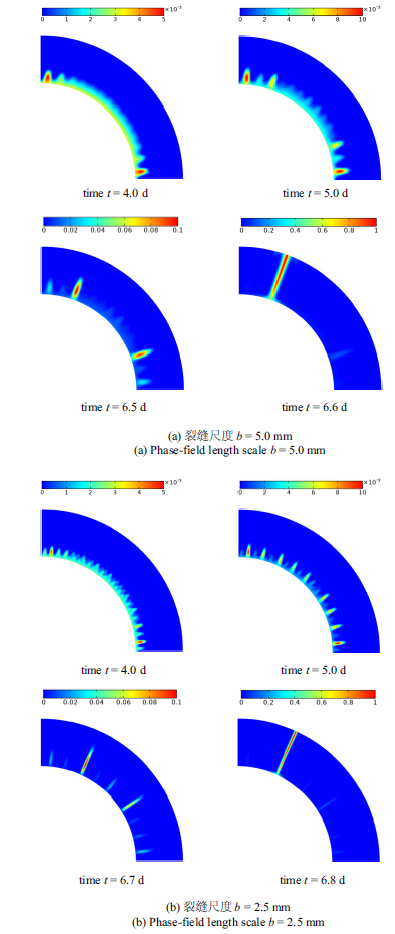
图12和图13分别给出了9.5 mm和19.0 mm两种厚度试件不同时刻的裂缝相场云图. 可以看出, 在开始阶段, 由于试件的环向应力均匀, 微裂缝沿内边缘均匀分布; 随着龄期增长, 收缩应变增大, 由于随机性的影响(这里体现为网格划分引起的数值误差), 部分微裂缝较其他裂缝扩展更为明显, 但混凝土强度也逐渐提高, 导致该阶段总体上裂缝扩展缓慢; 随着龄期进一步增长, 收缩应变显著增大, 导致环向某一位置出现控制性宏观裂缝并迅速扩展至圆环试件外边缘.

图12

新窗口打开|下载原图ZIP|生成PPT
图12约束混凝土圆环试验: 约束混凝土圆环试验: 两种相场尺度参数条件下不同时刻的裂缝相场云图模拟结果(圆环厚度19 mm)

Fig.12Restrained concrete ring test: Predicted contours of the crack phase-field at various time instants for different length scale parameters (thickness of the steel ring: 19 mm)

图13

新窗口打开|下载原图ZIP|生成PPT
图13约束混凝土圆环试验: 两种相场尺度参数条件下不同时刻的裂缝相场云图模拟结果(圆环厚度为9.5 mm)

Fig.13Restrained concrete ring test: Predicted contours of the crack phase-field at various time instants for different length scale parameters (thickness of the steel ring: 9.5 mm)



数值模拟给出的9.5 mm和19.0 mm两种厚度圆钢环应变演化曲线与试验结果的对比如图14所示. 可以看出, 考虑化$\!-\!$热$\!-\!$力多场耦合效应的相场内聚裂缝模型能够反映两种不同厚度钢环试件的应变演化规律, 特别是能够准确地预测混凝土开裂后、环向拉应力得到释放导致钢环所受压应力迅速降低、压应变出现陡降等典型特征.

图14

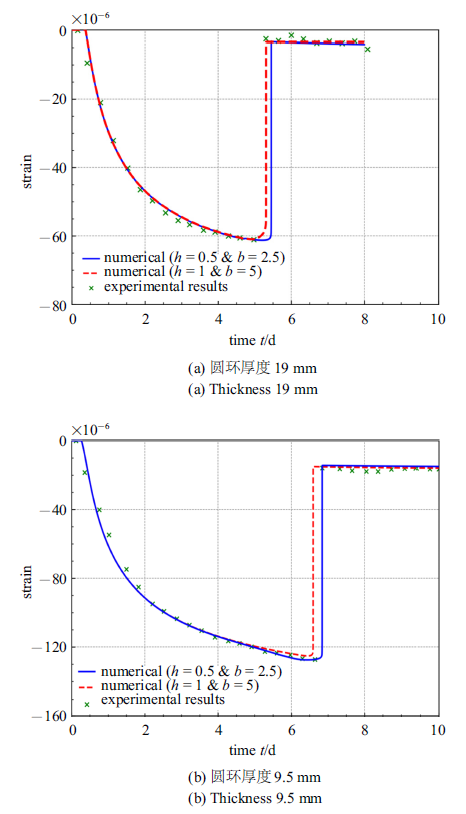新窗口打开|下载原图ZIP|生成PPT
图14约束混凝土圆环试验: 圆环应变演化曲线

Fig.14Restrained concrete ring test: predicted evolution curves of the compressive strains of the steel ring at various time instants



同样, 与纯力学载荷作用下的结果类似, 无论是定性的裂缝演化过程、亦或是定量的圆环压应变演化曲线, 裂缝尺度和网格大小对预测结果几乎不产生影响, 这一结果对模型应用于实际混凝土结构早龄期裂缝演化过程的准确模拟、抗裂性能的定量预测至关重要.

4 结论与展望

针对早龄期混凝土的抗裂性能预测和裂缝扩展全过程模拟, 本工作在统一相场理论的理论框架内, 采用相场内聚裂缝模型描述裂缝相场$\!-\!$位移场耦合效应, 进一步考虑了温度引起的混凝土热膨胀变形、水化反应导致的混凝土收缩和随龄期变化的力学性能(弹性模量、抗拉强度和断裂能等), 以及裂缝对水化过程和传热过程的影响, 建立了化$\!-\!$热$\!-\!$力多场耦合相场内聚裂缝模型.

与纯力学载荷作用下的相场内聚裂缝模型一样, 该模型同时融入了基于强度的裂缝起裂准则、基于能量的裂缝扩展准则以及基于变分原理的裂缝扩展方向判据, 因此能够描述早龄期混凝土水化作用和热量传输引起的复杂裂缝起裂和演化过程. 不同的是, 刻画裂缝起裂的混凝土抗拉强度并非固定值, 而是随龄期增长而逐渐增大并趋于最终强度; 类似地, 随龄期变化的混凝土弹性模量和断裂能, 也影响着早龄期混凝土的力学性能和裂缝演化行为. 因此, 不同阶段的水化作用和热量传输导致混凝土变形在热膨胀和自收缩之间相互竞争, 并对混凝土的早龄期抗裂性能产生显著影响.

上述化$\!-\!$热$\!-\!$力多场耦合相场内聚裂缝模型可以采用有限元等数值方法加以实现, 相应单元节点的自由度除位移和裂缝相场外, 还包括温度和水化度. 考虑到水化过程和传热过程的时间尺度远比开裂过程大, 将化$\!-\!$热$\!-\!$力多场耦合作用作用下早龄期混凝土的裂缝演化过程视为化学$\!-\!$热学耦合以及位移$\!-\!$裂缝相场耦合两个串行过程并分别进行求解. 前者采用标准的牛顿迭代方法进行求解, 而后者则利用笔者近期发展的整体BFGS拟牛顿算法, 实现收敛性和计算效率的双提升.

数值算例表明, 所提出的化$\!-\!$热$\!-\!$力多场耦合相场内聚裂缝模型不仅能够描述早龄期混凝土水化反应和热量传输条件下复杂的裂缝演化过程, 特别是热膨胀变形引起的裂缝起裂以及自收缩变形导致的裂缝扩展这两类相互竞争的裂缝演化机制, 而且数值模拟结果不受裂缝尺度和网格大小的影响, 实现了早龄期混凝土抗裂性能的准确预测.

需要指出的是, 上述工作忽略了徐变变形(包括基本徐变和瞬态徐变)^[20,69]以及水分挥发引起的干缩裂缝^[1,70]对混凝土早龄期力学性能的影响, 这将在后续研究中进一步加以考虑.

参考文献 View Option 原文顺序
文献年度倒序
文中引用次数倒序
被引期刊影响因子

[1] Ba?ant ZP, Jirásek M. Creep and Hygrothermal Effects in Concrete Structures
Dordrecht: Springer, 2018
[本文引用: 2]

[2] Ulm FJ, Coussy O. Modeling of thermochemomechanical couplings of concrete at early ages
Journal of Engineering Mechanics, 1995,121(7):785-794
DOIURL [本文引用: 1]

[3] Ulm FJ, Coussy O. Strength growth as chemo-plastic hardening in early age concrete
Journal of Engineering Mechanics, 1996,122(12):1123-1132
DOIURL [本文引用: 1]

[4] De Schutter G. Finite element simulation of thermal cracking in massive hardening concrete elements using degree of hydration based material laws
Computers & Structures, 2002,80(27-30):2035-2042
DOIURL [本文引用: 2]

[5] Lackner R, Mang HA. Chemoplastic material model for the simulation of early-age cracking: From the constitutive law to numerical analyses of massive concrete structures
Cement and Concrete Composites, 2004,26(5):551-562
DOIURL [本文引用: 1]

[6] De Borst R, Van Den Boogaard A. Finite-element modeling of deformation and cracking in early-age concrete
Journal of Engineering Mechanics, 1994,120(12):2519-2534
DOIURL [本文引用: 1]

[7] Song HW, Cho HJ, Park SS, et al. Early-age cracking resistance evaluation of concrete structures
Concrete Science and Engineering, 2001,3(10):62-72


[8] Ba?ant ZP, Kim JK, Jeon SE. Cohesive fracturing and stresses caused by hydration heat in massive concrete wall
Journal of Engineering Mechanics, 2003,129(1):21-30
DOIURL [本文引用: 1]

[9] Cervera M, Oliver J, Prato T. Thermo-chemo-mechanical model for concrete. I: Hydration and aging
Journal of Engineering Mechanics, 1999,125(9):1018-1027
DOIURL [本文引用: 1]

[10] Gawin D, Pesavento F, Schrefler BA. Hygro-thermo-chemo-mechanical modelling of concrete at early ages and beyond. Part I: Hydration and hygro-thermal phenomena; Part II: Shrinkage and creep of concrete
International Journal for Numerical Methods in Engineering, 2006,67(3):332-363
DOIURL

[11] Faria R, Azenha M, Figueiras JA. Modelling of concrete at early ages: Application to an externally restrained slab
Cement and Concrete Composites, 2006,28(6):572-585
DOIURL

[12] 金贤玉, 田野, 金南国. 混凝土早龄期性能与裂缝控制
建筑结构学报, 2010,31(6):204-212


(Jin Xianyu, Tian Ye, Jin Nanguo. Early age properties and cracking control of concrete
Journal of Building Structures, 2010,31(6):204-212 (in Chinese))


[13] Briffaut M, Benboudjema F, Torrenti JM, et al. Numerical analysis of the thermal active restrained shrinkage ring test to study the early age behavior of massive concrete structures
Engineering Structures, 2011,33(4):1390-1401
DOIURL [本文引用: 4]

[14] Wang Q, Ren X, Ballarini R. A multifield model for early-age massive concrete structures: Hydration, damage, and creep
Journal of Engineering Mechanics, 2020,146(10):04020115
DOIURL [本文引用: 1]

[15] Yuan Y, Wan ZL. Prediction of cracking within early-age concrete due to thermal, drying and creep behavior
Cement and Concrete Research, 2002,32(7):1053-1059
DOIURL [本文引用: 1]

[16] Grassl P, Wong HS, Buenfeld NR. Influence of aggregate size and volume fraction on shrinkage induced micro-cracking of concrete and mortar
Cement and Concrete Research, 2010,40(1):85-93
DOIURL

[17] Idiart AE, López CM, Carol I. Modeling of drying shrinkage of concrete specimens at the meso-level
Materials and Structures, 2011,44(2):415-435
DOIURL [本文引用: 1]

[18] Nguyen TT, Waldmann D, Bui TQ. Computational chemo-thermo-mechanical coupling phase-field model for complex fracture induced by early-age shrinkage and hydration heat in cement-based materials
Computer Methods in Applied Mechanics and Engineering, 2019,348:1-28
DOIURL [本文引用: 5]

[19] Nguyen TT, Weiler M, Waldmann D. Experimental and numerical analysis of early age behavior in non-reinforced concrete
Construction and Building Materials, 2019,210:499-513
DOIURL [本文引用: 1]

[20] Nguyen TT, Waldmann D, Bui TQ. Phase field simulation of early-age fracture in cement-based materials
International Journal of Solids and Structures, 2020,191:157-172
[本文引用: 2]

[21] Francfort GA, Marigo JJ. Revisiting brittle fracture as an energy minimization problem
Journal of the Mechanics and Physics of Solids, 1998,46(8):1319-1342
DOIURL [本文引用: 2]

[22] Bourdin B, Francfort GA, Marigo JJ. The Variational Approach to Fracture
Berlin: Springer, 2008
[本文引用: 2]

[23] Pham K, Amor H, Marigo JJ, et al. Gradient damage models and their use to approximate brittle fracture
International Journal of Damage Mechanics, 2011,20(4):618-652
DOIURL [本文引用: 2]

[24] Mesgarnejad A, Bourdin B, Khonsari MM. Validation simulations for the variational approach to fracture
Computer Methods in Applied Mechanics and Engineering, 2015,290:420-437
DOIURL

[25] TannéE, Li T, Bourdin B, et al. Crack nucleation in variational phase-field models of brittle fracture
Journal of the Mechanics and Physics of Solids, 2018,110:80-99
DOIURL [本文引用: 1]

[26] Mandal TK, Nguyen VP, Wu JY. Length scale and mesh bias sensitivity of phase-field models for brittle and cohesive fracture
Engineering Fracture Mechanics, 2019,217:106532
DOIURL [本文引用: 1]

[27] Kumar A, Bourdin B, Francfort GA, et al. Revisiting nucleation in the phase-field approach to brittle fracture
Journal of the Mechanics and Physics of Solids, 2020: 104027
[本文引用: 1]

[28] Wu JY. A unified phase-field theory for the mechanics of damage and quasi-brittle failure
Journal of the Mechanics and Physics of Solids, 2017,103:72-99
DOIURL [本文引用: 9]

[29] Bourdin B, Francfort GA, Marigo JJ. Numerical experiments in revisited brittle fracture
Journal of the Mechanics and Physics of Solids, 2000,48(4):797-826
DOIURL [本文引用: 2]

[30] Wu JY. A geometrically regularized gradient-damage model with energetic equivalence
Computer Methods in Applied Mechanics and Engineering, 2018,328:612-637
DOIURL [本文引用: 3]

[31] Wu JY, Nguyen VP. A length scale insensitive phase-field damage model for brittle fracture
Journal of the Mechanics and Physics of Solids, 2018,119:20-42
DOIURL [本文引用: 4]

[32] 吴建营. 固体结构损伤破坏统一相场理论, 算法和应用
力学学报, 2021,53(2):301-329
[本文引用: 4]

(Wu Jianying. On the theoretical and numerical aspects of the unified phase-field theory for damage and failure in solids and structures
Chinese Journal of Theoretical and Applied Mechanics, 2021,53(2):301-329 (in Chinese))
[本文引用: 4]

[33] Wu JY, Nguyen VP, Nguyen CT, et al. Phase field modeling of fracture
Advances in Applied Mechancis, 2020,53:1-183
[本文引用: 2]

[34] Wu JY, Nguyen VP, Zhou H, et al. A variationally consistent phase-field anisotropic damage model for fracture
Computer Methods in Applied Mechanics and Engineering, 2020,358:112629
DOIURL [本文引用: 4]

[35] Cornelissen H, Hordijk D, Reinhardt H. Experimental determination of crack softening characteristics of normalweight and lightweight
Heron, 1986,31(2):45-46
[本文引用: 2]

[36] Zhang P, Hu X, Wang X, et al. An iteration scheme for phase field model for cohesive fracture and its implementation in Abaqus
Engineering Fracture Mechanics, 2018,204:268-287
DOIURL [本文引用: 1]

[37] Zhang P, Hu X, Yang S, et al. Modelling progressive failure in multi-phase materials using a phase field method
Engineering Fracture Mechanics, 2019,209:105-124
DOI
In this paper, a new phase field method is proposed for modelling of progressive failure in multi-phase materials. Material properties of the interface between inclusion and matrix are regularized by an auxiliary interface phase field. In addition, crack initiation and propagation are simulated by using another crack phase field. Different failure mechanisms such as interface debonding, matrix cracking and the interaction between these two failure mechanisms are modelled in a unified framework. For general application of the framework, an image processing method is employed to identify the individual phases for a given multi-phase material. The proposed method is implemented into the commercial software ABAQUS through a user subroutine UEL (user defined element). The derived method is validated through an example of a single fiber reinforced composite system. Moreover, a procedure for choosing parameters of the proposed phase field model is discussed. Further, the validated method is applied to fracture analysis of a multi-phase concrete structure and complex failure mechanisms within and across the phases are captured.

[38] Wang Q, Feng YT, Zhou W, et al. A phase-field model for mixed-mode fracture based on a unified tensile fracture criterion
Computer Methods in Applied Mechanics and Engineering, 2020,370:113270
DOIURL

[39] Marboeuf A, Bennani L, Budinger M, et al. Electromechanical resonant ice protection systems: Numerical investigation through a phase-field mixed adhesive/brittle fracture model
Engineering Fracture Mechanics, 2020,230:106926
DOIURL

[40] Wang Q, Zhou W, Feng YT. The phase-field model with an auto-calibrated degradation function based on general softening laws for cohesive fracture
Applied Mathematical Modelling, 2020,86:185-206
DOIURL

[41] Loew PJ, Poh LH, Peters B, et al. Accelerating fatigue simulations of a phase-field damage model for rubber
Computer Methods in Applied Mechanics and Engineering, 2020,370:113247
DOIURL [本文引用: 1]

[42] Mandal TK, Nguyen VP, Wu JY. A length scale insensitive anisotropic phase field fracture model for hyperelastic composites
International Journal of Mechanical Sciences, 2020,188:105941
DOIURL [本文引用: 1]

[43] Miehe C, Welschinger F, Hofacker M. Thermodynamically consistent phase-field models of fracture: Variational principles and multi-field FE implementations
International Journal for Numerical Methods in Engineering, 2010,83(10):1273-1311
DOIURL [本文引用: 1]

[44] Ginzburg V, Landau L. Toward the superconductivity theory
Zhurnal Eksp. Yheoret. Physics, 1950,29:1064
[本文引用: 1]

[45] Cahn JW, Hilliard JE. Free energy of a nonuniform system. I. Interfacial free energy
The Journal of Chemical Physics, 1958,28(2):258-267
DOIURL [本文引用: 1]

[46] Allen SM, Cahn JW. A microscopic theory for antiphase boundary motion and its application to antiphase domain coarsening
Acta Metallurgica, 1979,27(6):1085-1095
DOIURL [本文引用: 1]

[47] Karma A, Kessler DA, Levine H. Phase-field model of mode III dynamic fracture
Physical Review Letters, 2001,87(4):045501
DOIURL [本文引用: 1]

[48] Griffith AA. The phenomena of rupture and flow in solids
Philosophical Transactions of the Royal Society of London, 1920,221:163-198.
[本文引用: 2]

[49] Mumford DB, Shah J. Optimal approximations by piecewise smooth functions and associated variational problems
Communications on Pure and Applied Mathematics, 1989,42(5):577-685
DOIURL [本文引用: 1]

[50] Ambrosio L, Tortorelli VM. Approximation of functional depending on jumps by elliptic functional via t-convergence
Communications on Pure and Applied Mathematics, 1990,43(8):999-1036
DOIURL [本文引用: 1]

[51] Braides A. Approximation of Free-Discontinuity Problems
Berlin: Springer Science & Business Media, 1998
[本文引用: 1]

[52] Nguyen VP, Wu JY. Modeling dynamic fracture of solids with a phase-field regularized cohesive zone model
Computer Methods in Applied Mechanics and Engineering, 2018,340:1000-1022
DOIURL [本文引用: 1]

[53] Mandal TK, Nguyen VP, Wu JY. Evaluation of variational phase-field models for dynamic brittle fracture
Engineering Fracture Mechanics, 2020,235:107169
DOIURL [本文引用: 1]

[54] Barenblatt GI. The formation of equilibrium cracks during brittle fracture. General ideas and hypotheses. Axially-symmetric cracks
Journal of Applied Mathematics and Mechanics, 1959,23(3):622-636
DOIURL [本文引用: 1]

[55] Ulm FJ, Coussy O. Couplings in early-age concrete: From material modeling to structural design
International Journal of Solids and Structures, 1998,35:4295-4311
DOIURL [本文引用: 1]

[56] Waller V. Relationship between mix design of concrete, generation of heat during hydration and compressive strength. [PhD Thesis]
France: école Nationale de Ponts et Chaussées, 1999
[本文引用: 1]

[57] Feng DC, Wu JY. Phase-field regularized cohesive zone model (CZM) and size effect of concrete
Engineering Fracture Mechanics, 2018,197:66-79
DOIURL [本文引用: 2]

[58] Wu JY, Qiu JF, Nguyen VP, et al. Computational modeling of localized failure in solids: XFEM vs PF-CZM
Computer Methods in Applied Mechanics and Engineering, 2019,345:618-643
DOIURL [本文引用: 2]

[59] De Schutter G, Taerwe L. Degree of hydration-based description of mechanical properties of early age concrete
Materials and Structures, 1996,29(6):335-344
DOIURL [本文引用: 1]

[60] De Schutter G, Taerwe L. Fracture energy of concrete at early ages
Materials and Structures, 1997,30(2):67-71
DOIURL [本文引用: 1]

[61] Wu JY. Robust numerical implementation of non-standard phase-field damage models for failure in solids
Computer Methods in Applied Mechanics and Engineering, 2018,340:767-797
DOIURL [本文引用: 1]

[62] Wu JY, Huang Y, Nguyen VP. On the BFGS monolithic algorithm for the unified phase field damage theory
Computer Methods in Applied Mechanics and Engineering, 2020,360:112704
DOIURL [本文引用: 2]

[63] Wu JY, Huang Y. Comprehensive implementations of phase-field damage models in abaqus
Theoretical and Applied Fracture Mechanics, 2020,106:102440
DOIURL [本文引用: 2]

[64] Nocedal J, Wright S. Numerical Optimization
New York: Springer, 2006.
[本文引用: 2]

[65] Matthies H, Strang G. The solution of nonlinear finite element equations
International Journal for Numerical Methods in Engineering, 1979,14(11):1613-1626
DOIURL [本文引用: 1]

[66] Kristensen PK, Martínez-Pa?eda E. Phase field fracture modelling using quasi-Newton methods and a new adaptive step scheme
Theoretical and Applied Fracture Mechanics, 2020,107:102446
DOIURL [本文引用: 1]

[67] Wu J Y, Huang Y, Zhou H, et al. Three-dimensional phase-field modeling of mode I$+$ II/III failure in solids
Computer Methods in Applied Mechanics and Engineering, 2021,373:113537
DOIURL [本文引用: 1]

[68] Hossain AB, Weiss J. Assessing residual stress development and stress relaxation in restrained concrete ring specimens
Cement and Concrete Composites, 2004,26(5):531-540
DOIURL [本文引用: 3]

[69] 刘宁, 刘光廷. 大体积混凝土结构随机温度徐变应力计算方法研究
力学学报, 1997,29(2):189-202
[本文引用: 1]

(Liu Ning, Liu Guangting. Methods for random thermal creep stress analysis of massive concrete structures
Acta Mechanica Sinica, 1997,29(2):189-202 (in Chinese))
[本文引用: 1]

[70] 崔溦, 陈王, 王宁. 考虑性态变化的早期混凝土热湿力耦合分析及其应用
土木工程学报, 2015,48(2):44-53
[本文引用: 1]

(Cui Wei, Chen Wang, Wang Ning. Thermo-hydro-mechanical coupling analysis of early-age concrete with behavioral changes considered and its application
China Civil Engineering Journal, 2015,48(2):44-53 (in Chinese))
[本文引用: 1]