HTML
--> --> -->The LASG/IAP Climate Ocean Model (LICOM) was developed at the State Key Laboratory of Numerical Modeling for Atmospheric Sciences and Geophysical Fluid Dynamics (LASG), Institute of Atmospheric Physics (IAP), Chinese Academy of Sciences (CAS) around 30 years in the late 1980s (Zhang and Liang, 1989). The latest version of LICOM is LICOM3, preparing for CMIP6. Currently, LICOM3 is the ocean component of two climate system models of CMIP6: the CAS Flexible Global Ocean–Atmosphere–Land System model, finite-volume version 3 (CAS FGOALS-f3) and the CAS Flexible Global Ocean–Atmosphere–Land System model, grid-point version 3 (CAS FGOALS-g3), which in CMIP5 were FGOALS-s2 (Bao et al., 2013, Lin et al., 2013a) and FGOALS-g2 (Li et al., 2013; Lin et al., 2013b), respectively. In addition, the previous version of LICOM, LICOM2.0 (Liu et al., 2012), is also employed as the ocean component in CAS-ESM (the CAS Earth System Model), version 1.0 (Dr. Minghua ZHANG, personal communication, 2016).
The OMIP simulations of LICOM3 were finished in June 2019 and the data have submitted to the Earth System Grid (ESG) data server (
2.1. Introduction to the model
Since LICOM2.0 (Liu et al., 2012), LICOM has been substantially upgraded in the interface with the flux coupler, the dynamic core, and the physical packages (Table 1). First, we upgraded the LICOM interface from the NCAR flux coupler 6 to coupler 7 (Lin et al., 2016), because the new version has been optimized for high-resolution modeling (Craig et al., 2012). Here, LICOM3 coupled with the Community Ice Code, version 4 (CICE4), i.e., the ocean–ice coupled model, is used to conduct the OMIP experiments. The prescribed atmospheric data have been input from the atmospheric data model and then passed to the coupler to drive the ocean–sea-ice coupled model.| Configuration | LICOM2.0 | LICOM3 | |
| Grid | Grid | Longitude/Latitude | Tripolar |
| Resolution | ~1°, 30 levels | ~1°, 30 or 80 levels | |
| Dynamic core | Tracer advection | Central differential scheme | Preserved shape scheme (Yu, 1994) |
| Momentum time integration | Explicit | Implicit | |
| Physics | Diapycnal mixing | Mixing in the mixed layer (Canuto et al., 2001, 2002) | Mixing in the mixed layer (Canuto et al., 2001) and internal tide mixing (St. Laurent et al., 2002) |
| Isopycnal mixing | Isopycnal mixing (Redi, 1982) and advection (Gent and McWilliams, 1990) | Isopycnal mixing (Redi, 1982) and advection (Gent and McWilliams, 1990) with N2 thickness diffusivity (Ferreira et al., 2005) | |
| Computing technics | Coupler interface | NCAR Flux Coupler 6 | NCAR Flux Coupler 7 |
| Parallel | 1D MPI and OMP | 2D MPI and OMP | |
| Data | Initial condition | WOA01 (Conkright et al., 2002) | PHC3.0 (Steele et al., 2001) |
| Bathymetry | DBDB5 (https://www.bodc.ac.uk/resources/inventories/edmed/report/356/) | ETOPO2 (https://ngdc.noaa.gov/mgg/global/etopo2.html) | |
Table1. Comparison of model configurations between two versions of LICOM (LICOM2.0 and LICOM3).
Second, the orthogonal curvilinear coordinate (Madec and Imbard, 1996; Murray, 1996) has been introduced in LICOM. The tripolar grid can be used with the North Pole split into two poles on the land in the Northern Hemisphere (NH) at (65°N, 65°E) and (65°N, 115°W). This improvement has solved the problem of the singularity of the North Pole in the normal longitude–latitude grid. Meanwhile, the spatial filter in the high latitudes of LICOM has also been eliminated, and the scalability and efficiency of the parallel algorithm has been extensively improved. Besides, preserved shape advection in the tracer formulation (Xiao, 2006) and implicit vertical viscosity (Yu et al., 2018) are also employed in the present version.
Third, the tidal mixing of St. Laurent et al. (2002) and the buoyancy frequency (N2)–related thickness diffusivity of Ferreira et al. (2005) have been introduced into LICOM after CMIP5. The effects of tidal mixing on the Atlantic meridional overturning circulation (AMOC) was preliminary evaluated by Yu et al. (2017). The effects of thickness diffusivity with temporal and spatial variation were evaluated by Li et al. (2019). Besides, the chlorophyll-a-dependent solar penetration of Ohlmann (2003), the vertical diffusivity of Canuto et al. (2001, 2002), and the isopycnal mixing of Redi (1982) and Gent and McWilliams (1990, GM90 hereafter) are also used in LICOM3. The isopycnal mixing coefficient is constant, with a value of 300 m2 s?1. The thickness mixing coefficient (i.e., diffusivity) for GM90 employs the scheme of Ferreira et al. (2005), in which the coefficient is dependent on the spatial distribution of N2 and varies with location and time. The coefficient is set to 300 m2 s?1 within the mixed layer or in the coastal region where the water depth is shallower than 60 m, while it varies between 300 and 2000 m2 s?1 in other places.
The virtual salinity flux is computed as the freshwater flux multiplied by a constant salinity of 34.7 psu. A restoring term with a piston velocity of 20 m yr?1 has been applied to the virtual salinity flux. If there is sea ice, a piston velocity of 50 m (20 d)?1 is applied under sea ice.
Here, the low-resolution LICOM3, which is used both for CMIP6 and OMIP, has 360 and 218 grid numbers for the zonal and the meridional directions, respectively. LICOM3 has two resolutions in the vertical (30 and 80 levels), but only the 30-level resolution is used for OMIP and CMIP6 to save computing resources. The depths of the W-grid and T-grid are shown in Table 2. At the same time, an eddy-resolving version of LICOM3 has also been developed, with a horizontal resolution of about 10 km at the equator and 2.7 km around the Antarctic, and 55 levels in the vertical. This version has also been implemented in an ocean forecast system for short-term ocean prediction①.
| Level | Depth for T | Depths for W |
| 1 | ?5 | 0 |
| 2 | ?15 | ?10 |
| 3 | ?25 | ?20 |
| 4 | ?35 | ?30 |
| 5 | ?45 | ?40 |
| 6 | ?55 | ?50 |
| 7 | ?65 | ?60 |
| 8 | ?75 | ?70 |
| 9 | ?85 | ?80 |
| 10 | ?95 | ?90 |
| 11 | ?105 | ?100 |
| 12 | ?115 | ?110 |
| 13 | ?125 | ?120 |
| 14 | ?135 | ?130 |
| 15 | ?145 | ?140 |
| 16 | ?156.9303 | ?150 |
| 17 | ?178.4277 | ?163.8606 |
| 18 | ?222.5018 | ?192.9948 |
| 19 | ?303.1057 | ?252.0088 |
| 20 | ?432.5961 | ?354.2027 |
| 21 | ?621.1931 | ?510.9896 |
| 22 | ?876.5334 | ?731.3966 |
| 23 | ?1203.337 | ?1021.67 |
| 24 | ?1603.2 | ?1385.003 |
| 25 | ?2074.526 | ?1821.396 |
| 26 | ?2612.596 | ?2327.656 |
| 27 | ?3209.772 | ?2897.536 |
| 28 | ?3855.835 | ?3522.009 |
| 29 | ?4538.428 | ?4189.662 |
| 30 | ?5243.597 | ?4887.194 |
| 31 | ? | ?5600 |
Table2. Depths of model levels for the T- and W-grid. Positive is upward.
2
2.2. Experiment designs
The OMIP experiment follows the protocol of CORE-II, which is an ocean–sea-ice coupled hindcast simulation forced by about 60 years of modified reanalysis atmospheric variables with diurnal to decadal signals. Usually, the experiment is conducted for five or six cycles to reach an equilibrium state. Details of the experiments are shown in Table 3. Here, LICOM3 is coupled with CICE4 using the NCAR flux coupler 7. Two standard OMIP experiments have been conducted: one forced with CORE-II data derived from NCEP–NCAR reanalysis (Large and Yeager, 2004), named OMIP1; and the other forced with the surface dataset for driving ocean–sea-ice models based on Japanese 55-year atmospheric reanalysis (JRA55-do, Tsujino et al., 2018), named OMIP2. The atmospheric variables include atmospheric surface wind vectors at 10 m, temperature at 10 m, specific humidity at 10 m, air density at 10 m, precipitation (both rain and snow), surface downward shortwave radiation, downward longwave radiation, and sea level pressure. The period of the forcing data is 62 years (1948–2009) for CORE-II, while the period is 61 years (1958–2018) for JRA55-do. Although the frequencies of the two datasets are different, we use 6-h intervals for both datasets to force the model. Here, experiments with six cycles (one cycle corresponds to 1948-2009 for CORE-II and 1958?2018 for JRA55-do) have been completed and uploaded onto ESG nodes for both OMIP1 and OMIP2. Details of the primary output and diagnostic variables are given in Table 4.| Experiment_id | Model | Initial condition (TS/currents) | Forcing data | Period | Frequency |
| OMIP1 | LICOM3/CICE4.0 | PHC3.0/Zero | CORE-II | 1948–2009 (6 cycles) | 6 h |
| OMIP2 | LICOM3/CICE4.0 | PHC3.0/Zero | JRA55-do | 1958–2018 (6 cycles) | 6 h |
Table3. Descriptions of the experiments of LICOM3.
| Name | Description | Frequency |
| hfbasin | Northward ocean heat transport | Monthly |
| hfbasinpmadv | Northward ocean heat transport due to parameterized mesoscale advection | Monthly |
| hfbasinpmdiff | Northward ocean heat transport due to parameterized mesoscale diffusion | Monthly |
| hfds | Downward heat flux at sea water surface | Monthly |
| masscello | Ocean grid-cell mass per area | Monthly |
| mlotst | Ocean mixed-layer thickness defined by sigma T | Monthly |
| msftbarot | Ocean barotropic mass streamfunction | Monthly |
| msftmz | Ocean meridional overturning mass streamfunction | Monthly |
| msftmzmpa | Ocean meridional overturning mass streamfunction due to parameterized mesoscale advection | Monthly |
| obvfsq | Square of Brunt–Vaisala frequency in sea water | Monthly |
| pbo | Sea water pressure at sea floor | Monthly |
| pso | Sea water pressure at sea water surface | Monthly |
| so | Sea water salinity | Monthly |
| sob | Sea water salinity at sea floor | Monthly |
| soga | Global mean sea water salinity | Monthly |
| sos | Sea surface salinity | Monthly |
| sosga | Global average sea surface salinity | Monthly |
| thetao | Sea water potential temperature | Monthly |
| thetaoga | Global average sea water potential temperature | Monthly |
| tob | Sea water potential temperature at sea floor | Monthly |
| tos | Sea surface temperature | Monthly |
| tosga | Global average sea surface temperature | Monthly |
| uo | Sea water X velocity | Monthly |
| umo | Ocean mass X transport | Monthly |
| vo | Sea water Y velocity | Monthly |
| vmo | Ocean mass Y transport | Monthly |
| wfo | Water flux into sea water | Monthly |
| wo | Sea water vertical velocity | Monthly |
| wmo | Upward ocean mass transport | Monthly |
| zos | Sea surface height above geoid | Monthly |
| omldamax | Mean daily maximum ocean mixed-layer thickness defined by mixing scheme | Daily |
| areacello | Grid-cell area for ocean variables | Fixed |
| deptho | Sea floor depth below geoid | Fixed |
| thkcello | Ocean model cell thickness | Fixed |
| ugrido | UGRID grid specification | Fixed |
| volcello | Ocean grid-cell volume | Fixed |
Table4. Descriptions of dataset variables of Priority 1.
3.1. Global mean variables
The basic results of OMIP1 and OMIP2 from LICOM3 are evaluated before submitting the whole datasets. Here, some primary aspects are selected to demonstrate the performance of the model. After six cycles of integration, the surface fluxes are balanced by the adjustment of intrinsic processes and the model reaches a quasi-steady state. Figure 1 shows six cycles of the global mean sea surface temperature (SST), the volume mean temperature, the global mean sea surface salinity (SSS), and the volume mean ocean temperature for both OMIP1 (blue curve) and OMIP2 (red curve). The global mean SST value quickly reaches an equilibrium state (Fig. 1a). The global volume mean temperature increases at a rate of 0.09°C (100 yr)?1 due to the input of net heat flux at the sea surface (Fig. 1b). The gradual decrease in net heat flux (from 0.4 W m?2 for the first cycle to 0.17 W m?2 for the sixth cycle), due to the SST increase, leads to the decrease in the temperature trend from cycle to cycle. The simulated global mean temperature reaches a quasi-stable state after the fifth to the sixth cycle. The global mean SSS value has a small trend, with a value of 0.02–0.03 psu (100 yr)?1, and reaches a near steady state after the second cycle. Meanwhile, the global mean volume ocean salinity has a trend of 0.01 psu (100 yr)?1 due to the positive virtual salt flux at the sea surface.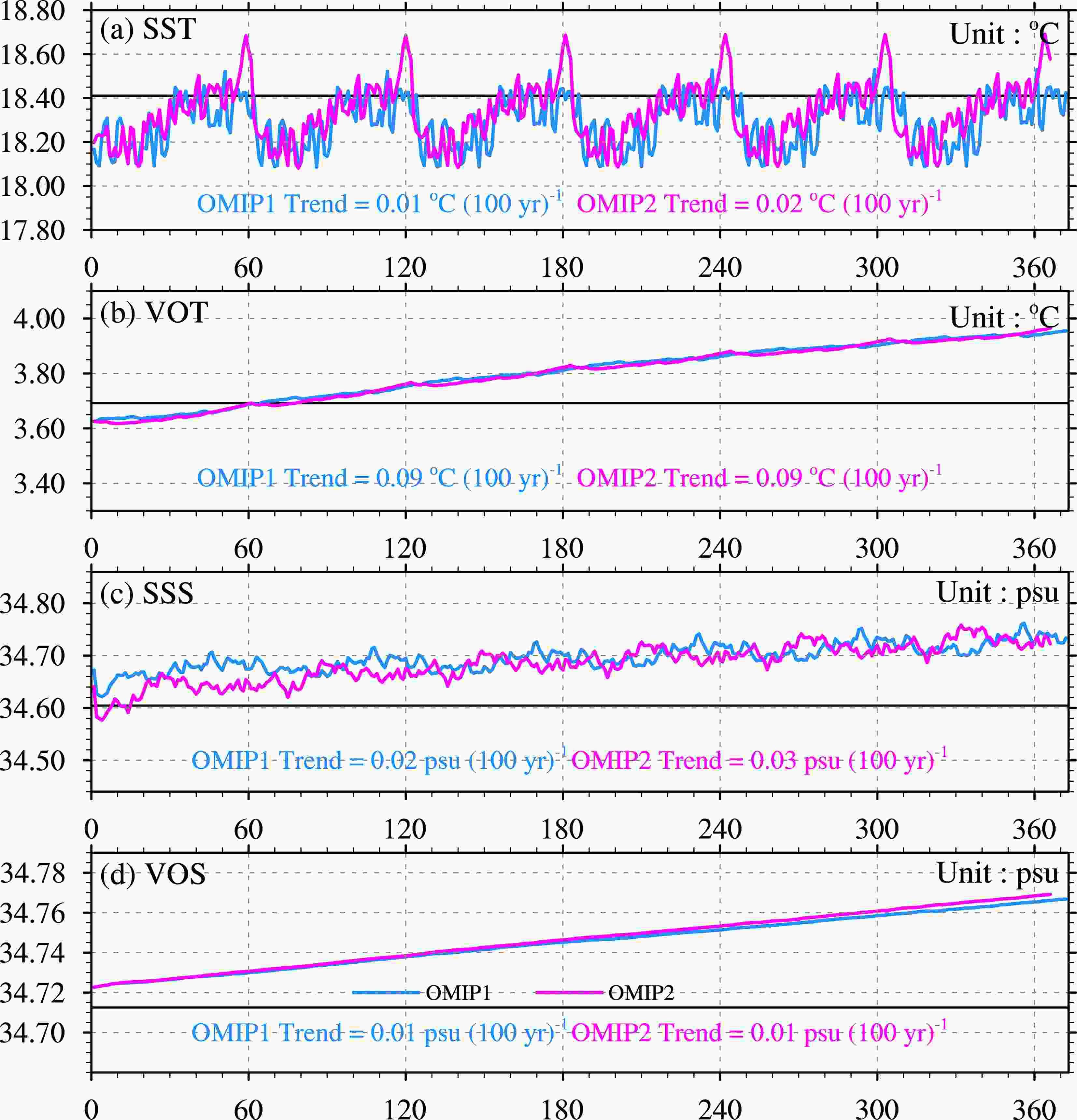
Figure1. Annual global mean (a) SST (units: °C), (b) volume ocean temperature (VOT; units: °C), (c) SSS (units: psu), and (d) volume ocean salinity (VOS; units: psu) for OMIP1 (cyan) and OMIP2 (purple) during all the six cycles. The black lines in the figure indicate the reference value calculated from WOA13 observation.The global annual mean SSTs from the last cycle of the two experiments are compared with Extended Reconstructed SST, version 5 (ERSST.v5) data (Huang et al., 2017), which serve as the observed reference values (Fig. 2a). The SSTs for OMIP1 and OMIP2 follow the observation well. The strong, warm ENSO events can be captured very well; for instance, 1982–83, 1997–98 and 2015–16. The correlation coefficient between OMIP2 and the observation is 0.95, which is much higher than that (0.81) between OMIP1 and the observation during the period of 1958–2009. After removing the linear trend, the standard deviations (STDs) of SST, indicative of the amplitude of interannual–decadal variability, are 0.08°C and 0.07°C for OMIP1 and OMIP2, respectively. However, the simulated global warming trends [calculated by linear trends; 0.06°C (10 yr)?1] are smaller than the observed ones [0.09°C (10 yr)?1] during 1958–2009, indicating a relatively fast vertical heat exchange in LICOM3.
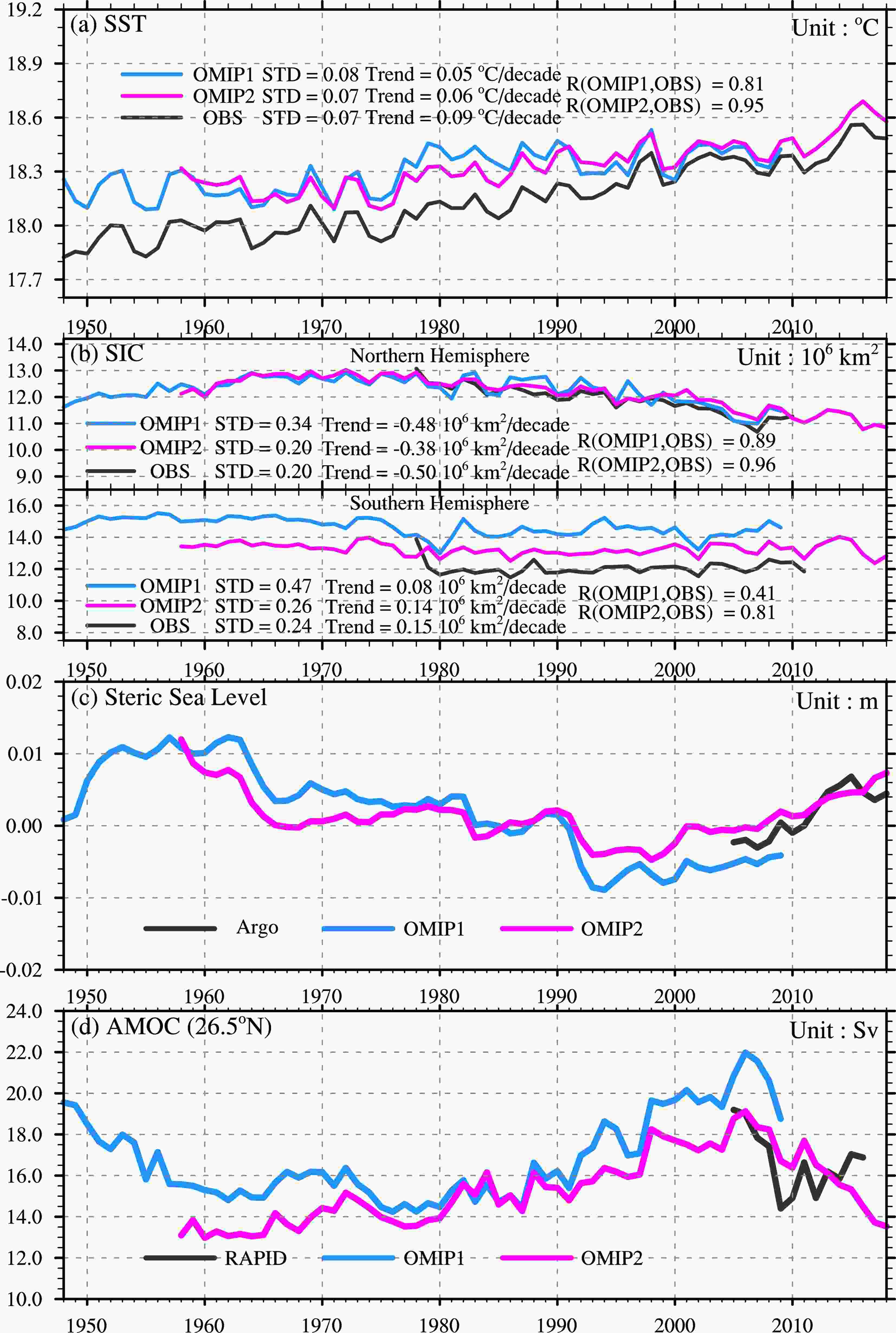Figure2. (a) Annual global mean SST (units: °C), (b) annual mean SIC in the NH and SH, (c) steric sea level, and (d) AMOC, for OMIP1 (cyan), OMIP2 (purple) and ERSST.v5/NSIDC/Argo/RAPID (black) during the sixth cycles. The STDs (removing linear trend), linear trends and correlation coefficients (SST during 1958–2009, SIC for 1980–2009, 2005–2018 for Argo, 2004–16 for RAPID) are provided in the figures.Associated with global warming, the sea-ice areas in the polar regions also shrink significantly. The change in sea ice will further affect the albedo and greatly perturb the heat entering the ocean. The simulated annual mean NH and Southern Hemisphere (SH) sea-ice cover (SIC) are shown along with their observed values from the National Snow and Ice Data Center (NSIDC; Fetterer et al., 2017; Fig. 2b). The annual mean values in the NH for models and observations match very well. The correlation coefficients between OMIP1 and OMIP2 and observations during 1980–2009 are 0.89 and 0.96, respectively. The simulated amplitude of interannual–decadal variability for OMIP2 is also better than that for OMIP1. The STDs are both 0.2 × 106 km2 for NSIDC and OMIP2, but much larger for OMIP1 (0.34 × 106 km2).
In the SH, the results from OMIP2 are also much closer to observation than that of OMIP1. The annual mean SIC values are overestimated by about 1.0–2.0 × 106 km2 in OMIP1 and OMIP2, although the latter is closer to the observation. The correlation coefficient between the simulation and the observation is 0.81 for OMIP2, but only 0.41 for OMIP1, during 1980–2009. The STDs are 0.24, 0.47 and 0.26 × 106 km2 for NSIDC, OMIP1 and OMIP2, respectively.
The simulated linear trends of SIC are negative for both the NH and SH. However, both OMIP1 and OMIP2 underestimate the magnitude, which is similar to the situation for SST. The observed linear trends are ?0.50 and 0.15 × 106 km2 (10 yr)?1 for the NH and SH, respectively. For comparison, the simulated trends are ?0.48 and 0.08 × 106 km2 (10 yr)?1 for OMIP1 and ?0.38 and 0.14 × 106 km2 (10 yr)?1 for OMIP2.
The global mean thermosteric sea level and the volume transport at 26.5°N in the Atlantic, which is used to measure the magnitude of AMOC, are also shown in Fig. 2 against the observational data from the Array for Real-time Geostrophic Oceanography (Argo;
2
3.2. SST and SSS biases
To understand the origin of systematic biases of ocean model, the biases of SST and SSS are shown in Figs. 3a–b and Figs. 3d–e, respectively. In general, the SST biases for the two experiments are similar, including large cold SST biases located in the regions of strong western boundary currents and the Arctic connected with the North Atlantic, and warm SST biases in the eastern boundary regions. The root-mean-square errors (RMSEs) of SST are 0.66°C for OMIP1 and 0.63°C for OMIP2. The improvements in SST simulation for OMIP2 occur in the eastern boundary, the warm pool, southern Indian Ocean, the Norwegian Sea, and Barents Sea (Fig. 3c).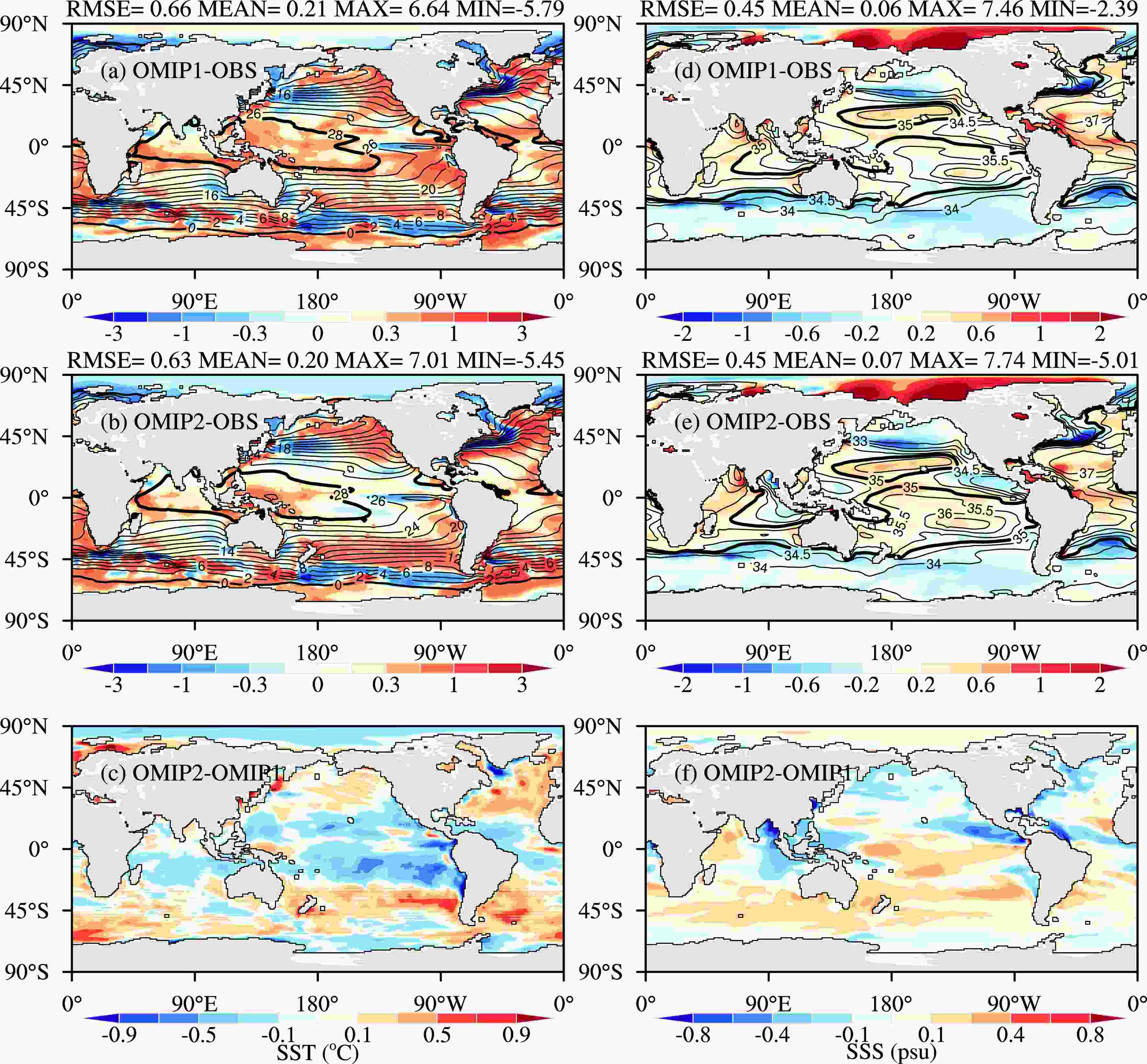
Figure3. Simulations (contours) and biases (shaded) of SST (units: °C) for (a) OMIP1 and (b) OMIP2, and (c) the difference betweeen OMIP2 and OMIP1 (OMIP2 minus OMIP1). (d–f) As in (a–c), respectively, but for SSS (units: psu). The annual SST and SSS distributions employ the data from 1980–2009 of the sixth cycle. The RMSEs, mean values, and minimim and maximal values are provided in the figures.The SSS for OMIP1 and OMIP2 have the same bias patterns and the same RMSEs (0.45 psu). The common biases include larger than 1.5 psu salty biases in the East Siberian Sea, Chukchi Sea and Beaufort Sea of the Arctic Ocean; 0.2–0.8 psu salty biases in the tropical Atlantic Ocean; ~0.2 psu salty biases in the tropical Pacific and western Indian Ocean; fresher than ?1.5 psu biases in the Gulf Stream region; and fresher biases of about ?0.8 to ?0.2 psu in the Greenland Sea, Barents Sea, Southern Ocean south of 40°S, and high-latitude North Pacific north of 30°N.
The improvements in SSS simulation for OMIP2 occur in the South China Sea, eastern Indian Ocean, western Atlantic Ocean, and in the Southern Ocean (south of 40°S; Fig. 3f). However, the SSS biases for OMIP2 increase in the western Indian Ocean. In the eastern Indian Ocean and western Pacific warm pool near the western coast, the salty SSS bias becomes the fresh SSS bias from OMIP1 to OMIP2. Meanwhile, the salty biases for OMIP2 also increase slightly in the subtropics of the South Pacific.
2
3.3. Sea surface height
The simulated sea surface height (SSH) is compared with satellite data from AVISO (Archiving, Validation and Interpretation of Satellite Oceanographic Data;
Figure4. The (a) observed and (b, c) OMIP1- and OMIP2-simulated SSH (units: m) during 1993–2009 of the sixth cycle. The spatial correlations between the observation and simulation are noted in the top right.2
3.4. AMOC
The AMOC results are displayed in Fig. 5. Both OMIP1 and OMIP2 can capture the features of that derived from the World Ocean Circulation Experiment hydrographic sections (Lumpkin and Speer, 2007). In the upper ocean (0–400 m), there are wind-driven cells connecting the tropics and subtropics. The cell is northward (southward) in the NH (SH). In the NH, the cell includes an equatorial current system and western boundary currents (Gulf Stream). In the middle and high latitudes of the NH, the upper-ocean cell is southward, corresponding to the sub-polar gyre. The maximal volume transport of North Atlantic Deep Water (NADW), which is located at the latitude of 38°N and a depth of about 1000 m, can reach 22 Sv. The upper branch of NADW can enter the Arctic Ocean (> 60°N). The low branch of NADW returns southward. The minimal volume transport of Antarctic Bottom Water (AABW) is ?6 Sv and limited below 3500 m.
Figure5. The simulated AMOC (units: Sv; 1Sv = 1 × 106 m3 s?1) for (a) OMIP1 and (b) OMIP2 during the last 30 years (1980–2009) of the sixth cycle. (c) AMOC values at the latitude of 26.5°N for OMIP1, OMIP2 and RAPID (2005–09). The maximal values and STD (+/-) between 2005–09 are noted. The y-axises represent ocean depth (units: km).Compared with the observed one at 26.5°N during the period 2005–09, the depth of maximal AMOCs is close to the observed. The depth from NADW shifting to AABW is located at about 3000 m for both simulations, which is shallower than the 4500 m for the observed and robust diagnostic values from Lee et al. (2019). The upward shift of the transition depth is a common problem for surface forced ocean-ice simulations (Danabasoglu et al., 2014, 2016). The simulated magnitudes of maximal AMOC transport are likely overestimated by 0.5–2.2 Sv than observation at 26.5°N. At 26.5°N, the AMOC for OMIP2 is about 18.24 Sv, which is slightly larger than the observed value from RAPID (17.57 Sv), but smaller than the value for OMIP1 (20.75 Sv).
2
3.5. Interannual–decadal SST amplitude
The interannual–decadal SST amplitude is denoted as the STD. The STDs of SST are presented in Fig. 6. The simulated amplitudes from OMIP1 and OMIP2 can capture well the observed one, with spatial correlations of 0.96. The large amplitudes are located in the tropical Pacific, the North Atlantic (> 40°N) and North Pacific (> 30°N) for the observation and simulations. However, the amplitudes are overestimated in the tropical Pacific and North Atlantic both in OMIP1 and OMIP2. Further comparisons show the simulated amplitude is closer to the observed in OMIP2 than that in OMIP1, including the tropical Pacific and North Atlantic. In the Ni?o3 region, the amplitudes are overestimated by about 32% and 16% for OMIP1 and OMIP2, respectively, compared with the observed (0.91). This indicates the OMIP2 forcing is better for simulating interannual–decadal variabilities than that of OMIP1.
Figure6. The STDs (units °C) of observed SST anomalies from (a) ERSST.v5 and (b, c) OMIP1- and OMIP2-simulated SST anomalies during 1960–2009. Before calculating the STD, both the linear trend and annual cycle were removed from the SST. The spatial correlation coefficients (R) with the observation are denoted in the top middle. The STDs in the Ni?o3 region (5°S–5°N, 150°–90°W) are denoted in the top right.The variables of Priority 1 for OMIP include monthly ocean temperature, salinity, ocean velocities, SSH, etc. (Table 4). There are 31 Priority 1 variables. Additionally, the daily maximal ocean mixed-layer thickness, defined as the depth that potential density is 0.03 kg m?3 larger than that on the sea surface, is also provided. Data grid information, such as area, mask, cell thickness and volume for every ocean grid, is also provided.
Acknowledgements. This study was supported by the National Key R&D Program for Developing Basic Sciences (Grant Nos. 2016YFC1401401 and 2016YFC1401601), the Strategic Priority Research Program of the Chinese Academy of Sciences (Grant No. XDC01000000), and the National Natural Science Foundation of China (Grants Nos. 41576026, 41576025, 41776030, 41931183 and 41976026).
Open Access This article is distributed under the terms of the Creative Commons Attribution License which permits any use, distribution, and reproduction in any medium, provided the original author(s) and the source are credited.
