Hui-Hua Zhao^,1,2,∗, Li-Chun Zhang^1,2, Fang Liu^1,21Institute of Theoretical Physics, Shanxi Datong University, Datong 037009, China
²Department of Physics, Shanxi Datong University, Datong 037009, China

First author contact: ^∗Author to whom any correspondence should be addressed.
Received:2021-02-13Revised:2021-04-1Accepted:2021-05-21Online:2021-07-16


Abstract
We discuss black hole solutions of Einstein-Λ gravity in the presence of nonlinear electrodynamics in dS spacetime. Considering the correlation of the thermodynamic quantities respectively corresponding to the black hole horizon and cosmological horizon of dS spacetime and taking the region between the two horizons as a thermodynamic system, we derive effective thermodynamic quantities of the system according to the first law of thermodynamics, and investigate the thermodynamic properties of the system under the influence of nonlinearity parameter α. It is shown that nonlinearity parameter α influences the position of the black hole horizon and the critical state of the system, and along with electric charge has an effect on the phase structure of the system, which is obvious, especially as the effective temperature is below the critical temperature. The critical phase transition is proved to be second-order equilibrium phase transition by using the Gibbs free energy criterion and Ehrenfest equations.
Keywords： dS black holes;nonlinear source;effective thermodynamic quantities;phase transition


PDF (701KB)MetadataMetricsRelated articlesExportEndNote|Ris|BibtexFavorite
Cite this article
Hui-Hua Zhao, Li-Chun Zhang, Fang Liu. Thermodynamics and phase transition of topological dS black holes with a nonlinear source. Communications in Theoretical Physics, 2021, 73(9): 095401- doi:10.1088/1572-9494/ac03ac

1. Introduction

Since the four laws of black hole mechanics were discovered, black holes have been widely believed to be thermodynamic and they possess standard thermodynamic quantities, such as temperature and entropy [1, 2]. The particular thermodynamic quantities of a black hole and its holographic properties are quantum essentially, so a black hole is a macroscopical quantum system. The studies of black hole thermodynamic properties provide an important window to investigate quantum gravity [1–3]. Phase transitions and critical properties are important and interesting subjects when studying a thermodynamic system, and recently, the critical behavior and phase transitions of black holes in anti-de Sitter (AdS) spacetime have been extensively investigated. By considering the cosmological constant as thermodynamic pressure, charged black holes show similar phase transitions to that of van der Waals–Maxwell’s liquid-gas. [4–25]. Researchers have also been trying to discover a series of common thermodynamic systems to simulate the phase transition and critical properties of black holes [26–29].

In the early period of inflation, the Universe is in quasi de Sitter (dS) space. The cosmological constant corresponding to vacuum energy is usually considered as a candidate for dark energy, with which our Universe will evolve into another dS phase. In order to construct the entire evolution history of our universe, we should have a clear perspective of the classical and quantum properties of dS space. Promoted by the recent success of AdS/CFT correspondence in theoretical physics, the duality relation of dS space has also aroused interest [30, 31]. Therefore, from an observational or theoretical point of view, it is rewarding to have a better understanding of the classical and quantum properties of dS space.

As the thermodynamic properties of AdS black holes are investigated in depth, the study of the physical properties of dS black holes has gradually aroused great interest [32–37], and more attention has also been paid to the phase transitions and critical behavior of dS black holes [38–47]. It is known that with the appropriate parameters dS space possesses not only a black hole event horizon (BEH) but a cosmological event horizon (CEH). Moreover, the two horizons generally have different radiation temperatures. Considering the two horizons as two thermodynamic systems that respectively correspond to two sets of thermodynamic quantities, both satisfy the first law of thermodynamics [48, 49]. Taking into account the thermodynamic relevance of the two horizons of dS black holes and considering the region between the two horizons as a thermodynamic system in non-thermal equilibrium [42–45, 50, 51], the thermodynamic behavior of some dS black holes is discussed by utilizing effective thermodynamic quantities.

As advances in related fields steadily grow, we start to gain a better understanding of the nature of black holes. In this work, we want to generalize the phase transition study to dS black hole solutions in Einstein gravity coupled to a nonlinear electromagnetic field. Nonlinear electrodynamics (NLED) theories are considerably richer than Maxwell field and in a special case they reduce to the linear Maxwell theory. About eighty years ago, Born and Infeld introduced an interesting kind of NLED in order to remove the divergence of the self-energy of a point-like charge [52]. Recently, it was shown that NLED objects can remove both the big bang and black hole singularities [53–58]. The first attempt to couple the NLED with gravity was made by Hoffmann [59]. Since then, the effect of Born–Infeld (BI)-type NLED coupled to the gravitational field has been extensively studied. In addition, BI NLED has another impetus, since it naturally arises in the low-energy limit of open string theory [60–62].

In previous works, researchers discussed the thermodynamic properties and stability of AdS black holes with a series of nonlinear sources [63, 64, 20, 65], and it is shown that the structures of black holes in the presence of nonlinear electrodynamics are quite different compared to the linear Maxwell theory, and its phenomenology also describes a more general case. In the work of Nam [66], the thermodynamics of dS black holes with a nonlinear electromagnetic Lagrangian was investigated in extended spacetime and with the system entropy S = S_c − S₊. The stability of Reissner–Nordström dS black holes has been investigated with a linear electromagnetic Lagrangian [42, 39]. In this paper, we investigate the effects of the nonlinearity of a series of weak nonlinear sources on the phase transitions and critical properties of charged dS black holes. Taking the region between the black hole horizon and cosmological horizon in dS space as a thermodynamic system, M as the mass (energy), and the entropy, volume and electric charge Q as thermodynamic variables of the system, the expression of the first law is obtained on the basis of the entropy S = S_c + S₊ [67] and the volume V = V_c − V₊ [68] of the system.

The outline of the paper is as follows. In the next section, we introduce the topological black hole solutions with a weak nonlinear source and two sets of thermodynamic quantities corresponding to the BEH and CEH of dS spacetime. In the third section, we analyze the relations of the thermodynamic quantities corresponding to the two horizons, and derive the effective thermodynamic quantities of the (n + 1) dimensional dS black hole with the nonlinear source. The critical state quantities and possible phase transitions of dS spacetime with the effect of the nonlinearity parameters are analyzed for n = 3 in the fourth section. In the fifth section, using the Ehrenfest scheme we prove that the critical behavior belongs to second-order equilibrium phase transition. In the final section, we discuss the findings and make some conclusions. (We use the units G_d = ℏ = k_B = c = 1).

2. Topological black hole solutions with a nonlinear source in dS spacetime

The (n + 1)-dimensional action of Einstein gravity in the presence of NLED is [64]:(2.1)$\begin{eqnarray}\begin{array}{c}{I}_{G}=-\frac{1}{16\pi }{\int }_{{ \mathcal M }}{{\rm{d}}}^{n+1}x\sqrt{-g}[R-2{\rm{\Lambda }}+L(F)]\\ \,-\frac{1}{8\pi }{\int }_{\partial { \mathcal M }}{{\rm{d}}}^{n}x\sqrt{-\gamma }{\rm{\Theta }}(\gamma ),\end{array}\end{eqnarray}$where R is the scalar curvature and Λ is the cosmological constant. In this action,(2.2)$\begin{eqnarray}L({ \mathcal F })=-{ \mathcal F }+\alpha {{ \mathcal F }}^{2}+{ \mathcal O }({\alpha }^{2}),\end{eqnarray}$is the Lagrangian of NLED. ${ \mathcal F }={F}_{\mu \nu }{F}^{\mu \nu }$ is the Maxwell invariant, in which F_μν = ∂_μA_ν − ∂_νA_μ is the electromagnetic field tensor and A_μ is the gauge potential. In addition, α denotes the nonlinearity parameter, which is small, so the effects of nonlinearity should be considered as a perturbation (α is proportional to the inverse value of the nonlinearity parameter in BI-type theories), and the series expansion for the Lagrangian is only preserved up to the first-order term of α . In this paper, we take into account equation (2.2) as the NLED source, because both BI types of NLED, in logarithmic form [69] and in exponential form [70], tend to the form of equation (2.2) for weak nonlinearity.

The (n + 1)-dimensional topological black hole solutions can be written as [64],(2.3)$\begin{eqnarray}{{\rm{d}}{s}}^{2}=-f(r){{\rm{d}}{t}}^{2}+\displaystyle \frac{{{\rm{d}}{r}}^{2}}{f(r)}+{r}^{2}{\rm{d}}{{\rm{\Omega }}}_{n-1}^{2},\end{eqnarray}$where(2.4)$\begin{eqnarray}\begin{array}{l}f(r)=k-\displaystyle \frac{m}{{r}^{n-2}}-\displaystyle \frac{2{\rm{\Lambda }}{r}^{2}}{n(n-1)}\\ \quad +\displaystyle \frac{2{q}^{2}}{(n-1)(n-2){r}^{2n-4}}\\ \quad -\displaystyle \frac{4{q}^{4}\alpha }{[2(n-2)(n+2)+(n-3)(n-4)]{r}^{4n-6}},\end{array}\end{eqnarray}$in which m is an integration constant related to the mass of the black hole, and the last term in equation (2.4) indicates the effect of nonlinearity, where only the first-order term of α is preserved. The asymptotical behavior of the solution is AdS or dS, provided that Λ < 0 or Λ > 0 and the case of an asymptotically flat solution is permitted for Λ = 0 and k = 1.

We consider asymptotically dS (Λ > 0) and spherically topological black holes, which have BEH and CEH, whose positions are located at r₊ and r_c, and f(r_+,c) = 0.

In figure 1, on each curve, the two points on the right, at which the curve intersects the horizontal axis, correspond to r₊ and r_c, and r₊ < r_c. One can see that the nonlinearity parameter α influences the position of BEH (r₊) obviously, but the effect on r_c is not obvious.

Figure 1.

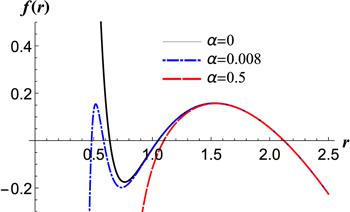New window|Download| PPT slide
Figure 1.Black hole solution in dS spacetime with k = 1, n = 4, q = 1, m = 1.2, Λ = 1 and different nonlinearity parameter α.


The radiation temperatures on the two horizons, respectively, are [64],(2.5)$\begin{eqnarray}\begin{array}{rcl}{T}_{+} & = & \displaystyle \frac{f^{\prime} ({r}_{+})}{4\pi }=\displaystyle \frac{1}{2\pi (n-1)}\left(\displaystyle \frac{(n-1)(n-2)k}{2{r}_{+}}\right.\\ & & \left.-{\rm{\Lambda }}{r}_{+}-\displaystyle \frac{{q}^{2}}{{r}_{+}^{2n-3}}+\displaystyle \frac{2{q}^{4}\alpha }{{r}_{+}^{4n-5}}\right),\\ {T}_{c} & = & -\displaystyle \frac{f^{\prime} ({r}_{c})}{4\pi }=-\displaystyle \frac{1}{2\pi (n-1)}\left(\displaystyle \frac{(n-1)(n-2)k}{2{r}_{c}}\right.\\ & & \left.-{\rm{\Lambda }}{r}_{c}-\displaystyle \frac{{q}^{2}}{{r}_{c}^{2n-3}}+\displaystyle \frac{2{q}^{4}\alpha }{{r}_{c}^{4n-5}}\right).\end{array}\end{eqnarray}$The ADM (Arnowitt–Deser–Misner) mass M and electric charge Q of the black holes are,(2.6)$\begin{eqnarray}M=\displaystyle \frac{{V}_{n-1}\left(n-1\right)m}{16\pi },\end{eqnarray}$(2.7)$\begin{eqnarray}Q=\displaystyle \frac{q}{4\pi }{V}_{n-1},\end{eqnarray}$in which ${V}_{n-1}=\tfrac{2{\pi }^{n/2}}{{\rm{\Gamma }}\left(n/2\right)}$ is the volume of the unit-radius (n-1)-sphere.

Considering f(r_+,c) = 0, the mass of the black holes can be expressed by r₊ or r_c as,(2.8)$\begin{eqnarray}\begin{array}{rcl}M & = & \displaystyle \frac{{V}_{n-1}(n-1)}{16\pi }\left({{kr}}_{+,c}^{n-2}-\displaystyle \frac{2{\rm{\Lambda }}{r}_{+,c}^{n}}{n(n-1)}\right.\\ & & +\displaystyle \frac{2{q}^{2}}{(n-1)(n-2){r}_{+,c}^{n-2}}\\ & & \left.-\displaystyle \frac{4{q}^{4}\alpha }{[2(n-2)(n+2)+(n-3)(n-4)]{r}_{+,c}^{3n-4}}\right).\end{array}\end{eqnarray}$The entropy and thermodynamic volume of the black holes corresponding to BEH and CEH are [67],(2.9)$\begin{eqnarray}\begin{array}{rcl}{S}_{+} & = & \displaystyle \frac{{V}_{n-1}{r}_{+}^{n-1}}{4},\quad {S}_{c}=\displaystyle \frac{{V}_{n-1}{r}_{c}^{n-1}}{4},\\ {V}_{+} & = & \displaystyle \frac{{V}_{n-1}{r}_{+}^{n}}{n},\quad {V}_{c}=\displaystyle \frac{{V}_{n-1}{r}_{c}^{n}}{n}.\end{array}\end{eqnarray}$

From the perspective of extended phase space, the cosmological constant is considered as the thermodynamic pressure of black holes [71, 72],(2.10)$\begin{eqnarray}{P}_{0}=-\displaystyle \frac{{\rm{\Lambda }}}{8\pi }.\end{eqnarray}$

From equations (2.5), (2.8), (2.9) and (2.10), two sets of thermodynamic quantities can be obtained corresponding to BEH and CEH, respectively, whose thermodynamic properties have been dealt with separately in some researches [49]. They satisfy, respectively, the first law of thermodynamics,(2.11)$\begin{eqnarray}\begin{array}{ccc}\delta M & = & {T}_{+}\delta {S}_{+}+{{\rm{\Phi }}}_{+}\delta Q+{V}_{+}\delta {P}_{0},\\ \delta M & = & -{T}_{{\rm{c}}}\delta {S}_{c}+{{\rm{\Phi }}}_{c}\delta Q+{V}_{c}{P}_{0},\end{array}\end{eqnarray}$in which Φ₊ and Φ_c are the charge potentials of BEH and CEH, respectively.

Two baffling problems exist with regard to the two horizons being two thermodynamic systems unless they are very far apart. First, the energy M, electric charge Q and cosmological constant Λ are the common state parameters of the two systems. Thus, the thermodynamic quantities of the two systems are not independent. Second, the black hole horizon radiation temperature T₊ and cosmological radiation temperature T_c present in equations (2.5) and (2.11) are usually different. Consequently, we take the dS space region between BEH and CEH as a thermodynamic system, but it is generally in thermal non-equilibrium. At present, a mature and general purpose method to analyze a non-equilibrium thermodynamic system has not been found. However, the fact that the two horizons possess some common state parameters reminds us that we need to build a set of effective thermodynamic quantities to investigate the thermodynamic properties of the thermal non-equilibrium system in dS spacetime.

3. Effective thermodynamic quantities of a (n + 1) dimensional dS black hole with a nonlinear source

Taking the dS space region between BEH and CEH as a thermodynamic system, the thermodynamic volume and entropy of the spherically symmetric dS spacetime satisfy [67, 68],(3.1)$\begin{eqnarray}\begin{array}{rcl}V & = & {V}_{c}-{V}_{+}=\displaystyle \frac{{V}_{n-1}{r}_{c}^{n}}{n}\left(1-{x}^{n}\right),\\ S & = & {S}_{c}+{S}_{+}=\displaystyle \frac{{V}_{n-1}{r}_{c}^{n-1}}{4}\left(1+{x}^{n-1}\right),\end{array}\end{eqnarray}$in which $x=\tfrac{{r}_{+}}{{r}_{c}}\leqslant 1$. From equation (2.8), considering the conserved quantities M, Q and Λ of the dS spacetime, the energy (mass) M can be expressed as,(3.2)$\begin{eqnarray}\begin{array}{l}M=\displaystyle \frac{{V}_{n-1}(n-1)}{16\pi (1-{x}^{n})}{r}_{c}^{n-2}\left[k({x}^{n-2}-{x}^{n})\right.\\ +\displaystyle \frac{2{q}^{2}}{(n-1)(n-2){r}_{c}^{2(n-2)}}\displaystyle \frac{(1-{x}^{2n-2})}{{x}^{n-2}}\\ -\displaystyle \frac{4{q}^{4}\alpha }{[2(n-2)(n+2)+(n-3)(n-4)]{r}_{c}^{2(2n-3)}}\\ \left.\displaystyle \frac{(1-{x}^{4n-4})}{{x}^{3n-4}}\right].\end{array}\end{eqnarray}$

Taking α as a constant and combining equations (3.1) and (3.2), one can see that the energy (mass) of the dS black hole system is a function of the entropy, thermodynamic volume and electric charge, that is:(3.3)$\begin{eqnarray}M=M(S,V,Q).\end{eqnarray}$They should satisfy the first law of thermodynamics:(3.4)$\begin{eqnarray}{\rm{d}}{M}={T}{\rm{d}}{S}+{\rm{\Phi }}{\rm{d}}{Q}-{P}{\rm{d}}{V},\end{eqnarray}$in which T is the effective temperature, Φ is the effective electric potential and P is the effective pressure. They are conjugates of S, Q and V of dS spacetime, respectively.(3.5)$\begin{eqnarray}\begin{array}{l}T={\left(\displaystyle \frac{\partial M}{\partial S}\right)}_{Q,V}=\displaystyle \frac{1}{4\pi {x}^{n-2}(1-{x}^{n})(1+x){r}_{c}}\\ \left[k\left({x}^{n-3}(n-2-{{nx}}^{2})(1-{x}^{n})+{{nx}}^{n-1}({x}^{n-2}-{x}^{n})\right.\right.\\ \left.+(n-2){x}^{n-1}({x}^{n-2}-{x}^{n}\right)\\ +\displaystyle \frac{2{q}^{2}}{(n-1)(n-2){r}_{c}^{2(n-2)}}\left(\displaystyle \frac{(2n-2){x}^{n}-{{nx}}^{2n-2}-(n-2)}{{x}^{n-1}}\right.\\ \left.-\displaystyle \frac{(n-2)(1-{x}^{2n-2})}{{x}^{-1}}\right)\\ +\displaystyle \frac{4{q}^{4}\alpha }{[2(n-2)(n+2)+(n-3)(n-4)]{r}_{c}^{4n-6}}\\ \left(3n-4)\displaystyle \frac{(1-{x}^{4n-4})}{{x}^{2n-3}}\right.\\ \left.\left.-\displaystyle \frac{(4n-4){x}^{n}-{{nx}}^{4n-4}-(3n-4)}{{x}^{3n-3}}\right)\right],\end{array}\end{eqnarray}$(3.6)$\begin{eqnarray}\begin{array}{l}{\rm{\Phi }}={\left(\displaystyle \frac{\partial M}{\partial Q}\right)}_{S,V}=\displaystyle \frac{(n-1)(1-{x}^{2n-2})q}{(1-{x}^{n}){r}_{c}^{n-2}{x}^{n-2}}\\ \left[\displaystyle \frac{1}{(n-1)(n-2)}\right.\\ -\displaystyle \frac{4{q}^{2}\alpha }{[2(n-2)(n+2)+(n-3)(n-4)]{r}_{c}^{2n-2}}\\ \left.\displaystyle \frac{(1+{x}^{2n-2})}{{x}^{2n-2}}\right],\end{array}\end{eqnarray}$(3.7)$\begin{eqnarray}\begin{array}{rcl}P & = & -{\left(\displaystyle \frac{\partial M}{\partial V}\right)}_{Q,S}\\ & = & \displaystyle \frac{1}{16\pi (1+x){\left(1-{x}^{n}\right)}^{2}}\\ & & \left[\displaystyle \frac{-\left(n-1\right)k}{{r}_{c}^{2}{x}^{2}}\left(\left(n-2\right)\left({x}^{2n+2}-x\right)\right.\right.\\ & & \left.-{{nx}}^{2n}+2{x}^{n+2}-2{x}^{n+1}+{{nx}}^{3}\right)\\ & & +\displaystyle \frac{2{q}^{2}\left({x}^{n}+x\right)}{{r}_{c}^{2n-2}{x}^{2n}}\left(\displaystyle \frac{(2n-2)\left({x}^{n+2}-{x}^{2n}\right)}{(n-2)}\right.\\ & & \left.+\left({x}^{n}-x\right)\left({x}^{2n}+x\right)\right)\\ & & -\displaystyle \frac{4{q}^{4}\alpha (x+{x}^{n})}{{r}_{c}^{4n-4}{x}^{4n}}\left(\displaystyle \frac{(4n-4)\left({x}^{n+4}-{x}^{4n}\right)}{(3n-4)}\right.\\ & & \left.\left.\left({x}^{n}-x\right)\left({x}^{2n}+x\right)\left({x}^{2n}+{x}^{2}\right)\right)\right].\end{array}\end{eqnarray}$These effective thermodynamic quantities reflect the thermodynamic properties of the whole dS spacetime rather than that of a horizon. Therefore, they give a more comprehensive view of dS spacetime.

4. Critical state and phase transition in the dS black holes with the effect of NLED

We investigate the critical properties, phase transitions and phase structure of the spherical topology (k = 1) of dS black holes with a weak nonlinear source by using effective thermodynamic quantities. According to van der Waals’ idea regarding phase transitions, we analyze the relation of the effective pressure and thermodynamic volume when the effective temperature is kept constant. And using the Gibbs free energy criterion, we discuss the phase transition of the system.

When both the electric charge q and the nonlinearity parameter α are kept as constants, the critical points of the phase transition of the system can be obtained by,(4.1)$\begin{eqnarray}{\left(\displaystyle \frac{\partial P}{\partial V}\right)}_{T}=0,\quad {\left(\displaystyle \frac{{\partial }^{2}P}{\partial {V}^{2}}\right)}_{T}=0,\end{eqnarray}$in which all the quantities V, T and P are functions of (r_c, x) according to equations (3.1), (3.5) and (3.7). Thus, the critical quantities can be derived when Q, α and dimensionality n are given certain values. Table 1 below shows some critical values at the dimensionality n = 3.


Table 1.
Table 1.Critical values of effective thermodynamic quantities for different Q and α when n = 3.

n=3	Q = 1					Q = 2	Q = 3
	α = 0.001	α = 0.01	α = 0.1	α = 0.3	α = 0.6	α = 0.1	α = 0.1	α = 0.3	α = 0.6
xc	0.732 22	0.732 30	0.733 16	0.735 59	0.742 74	0.134 43	0.086 97	0.157 71	0.237 08
${r}_{c}^{c}$	3.5058	3.5025	3.4675	3.3760	3.1627	7.5636	13.915	10.554	8.8944
Tc	0.008 015	0.008 018	0.008 053	0.008 139	0.008 312	4.103E-5	6.541E-6	4.562E-5	1.601E-4
Pc	6.055E-4	6.061E-4	6.128E-4	6.298E-4	6.666E-4	1.775E-6	1.550E-7	1.409E-6	5.746E-6
Vc	109.64	109.30	105.82	97.019	78.215	1807.9	11 278.4	4904.4	2908.1
Mc	1.2086	1.2080	1.2007	1.1821	1.1404	2.1764	3.6970	3.1454	2.9283
Sc	59.315	59.207	58.077	55.179	48.758	182.96	612.89	358.61	262.50
Gc	0.7996	0.7995	0.7979	0.7942	0.7873	2.172	3.695	3.136	2.903

New window|CSV

Under the conditions 0 < x < 1 and T > 0, the relation of P and V is derived numerically as T takes some certain values near the critical temperature T^c, and the corresponding effective isotherms of the system are depicted in figure 2.

Figure 2.

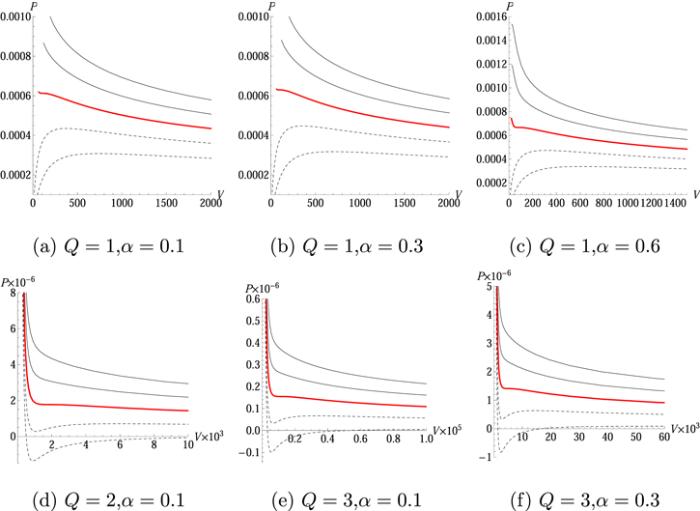New window|Download| PPT slide
Figure 2.Isotherms in P − V diagrams of a (n + 1) dimensional dS black hole with the weak nonlinear source of n = 3. Dashed lines match T < T^c, red thick solid lines match T = T^c and thin solid lines match T > T^c.


It can be seen in table 1 that with increasing α the critical values x^c, critical temperature T^c and critical pressure P^c are all increasing as Q is given, and when Q is greater, they increase faster. The other critical quantities in the table are just the opposite. With the increasing of α, they decrease. Similarly, as α = 0.1, x^c, T^c and P^c are all increasing with increasing Q. The other critical quantities in the table decrease oppositely when Q increases. From table 1, it is shown that the nonlinearity parameters α along with the electric charge Q influence the position of the critical state. When α = 0, the values of the critical quantities are consistent with those in the work [42], which is not shown in the table 1.

Figure 2 shows that when either of the values α or Q is greater or both are greater, a more acceptable thermodynamic state exists within the smaller volume ranges and the isotherms are more like the ones of the van der Waals liquid-gas system. Thus, compared with linear electrodynamics, NLED has an effect on the phase structure of the dS black hole system. In figures 2(a), (b) and (c), when T < T^c, the slopes of the isotherms are positive in the region of smaller volume, which means the system is thermodynamically unstable, while the slopes of the isotherms are negative in the region of larger volume, which means the system can be thermodynamically stable. All the isotherms with T > T^c in figure 2 always have negative slopes, which means the system can always be thermodynamically stable. In figures 2(d), (e) and (f), where Q is larger than the ones in figures 2(a), (b) and (c), each isotherm for T < T^c implies two thermodynamically stable regions with negative slopes. The two regions are discontinuous on the isotherms, but they may be connected by a phase transition at a certain pressure according to Maxwell’s equal area law, which can also be tested by the Gibbs free energy criterion, that is, the lower the Gibbs free energy, the more stable the thermodynamic system is at certain pressure and temperature. It is worth noting that, when either of the values of α or Q is smaller or both are smaller, each isotherm has a left endpoint corresponding to the smallest volume, which is too small, which means that we do not have a proper real horizon solution for the dS black hole. This is also the case when α = 0 [42]. However, when either of the values of α or Q becomes greater, the endpoints on the isotherms gradually move to the left until they disappear, which qualitatively shows the effect of NLED on the phase structure of dS black holes as its effect on a AdS black hole [65].

Gibbs free energy is defined [73] as:(4.2)$\begin{eqnarray}G\,=\,M-{TS}+{PV}.\end{eqnarray}$Under the conditions 0 < x < 1 and T > 0, we depict the G − P diagrams with some certain effective temperatures near the critical temperatures T^c for the dS black hole systems with the nonlinear source in figure 3.

Figure 3.

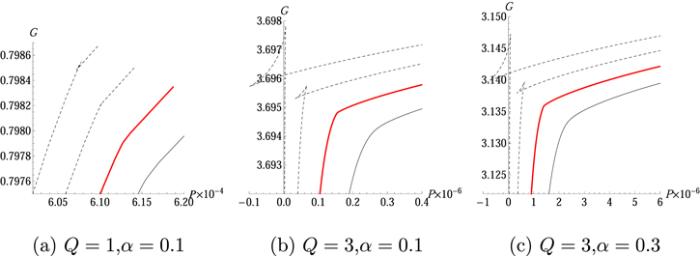New window|Download| PPT slide
Figure 3.Isotherms in the G − P diagrams of a (n + 1) dimensional dS black hole with a weak nonlinear source of n = 3. Dashed lines match T < T^c, red thick solid lines match T = T^c and thin solid lines match T > T^c.


In figure 3, the curves for Gibbs free energy G versus effective pressure P present a swallow-tail form when T < T^c is near the critical effective temperature. According to the Gibbs free energy criterion, below the critical temperature a first-order phase transition can exist with volume discontinuous change, but above the critical temperature, it does not. The larger Q and α, the more obvious the phase transition. Figure 3 shows that, near the effective critical points, the phase structure of the system is similar to that of the van der Waals–Maxwell’s liquid-gas system.

5. Analytical check of critical behavior by using classical Ehrenfest scheme

According to Ehrenfest scheme, at the phase transition point, the fact that the chemical potential (molar Gibbs free energy) and its first partial derivatives are continuous but its second partial derivatives are discontinuous and divergent indicates that the phase transition belongs to second-order equilibrium phase transition. The specific heat C_P, expansion coefficient β and isothermal compressibility κ of a thermodynamic system are defined by:(5.1)$\begin{eqnarray}{C}_{P}=T{\left(\displaystyle \frac{\partial S}{\partial T}\right)}_{P}=-T\displaystyle \frac{{\partial }^{2}G}{\partial {T}^{2}},\end{eqnarray}$(5.2)$\begin{eqnarray}\beta =\displaystyle \frac{1}{V}{\left(\displaystyle \frac{\partial V}{\partial T}\right)}_{P}=\displaystyle \frac{1}{V}\displaystyle \frac{{\partial }^{2}G}{\partial T\partial P},\end{eqnarray}$(5.3)$\begin{eqnarray}\kappa =-\displaystyle \frac{1}{V}{\left(\displaystyle \frac{\partial V}{\partial P}\right)}_{T}=-\displaystyle \frac{1}{V}\displaystyle \frac{{\partial }^{2}G}{\partial {P}^{2}}.\end{eqnarray}$C_P − P, β − P and κ − P curves of the dS black holes with NLED for given values of Q and α are shown in figure 4 for n = 3. The red thick solid lines corresponding to T = T^c are all divergent at P = P^c, so the phase transitions at the critical points belong to second-order equilibrium phase transition. Phase transitions below the critical temperature are more complex, while no phase transition is displayed by the curves above the critical temperature in figure 4.

Figure 4.

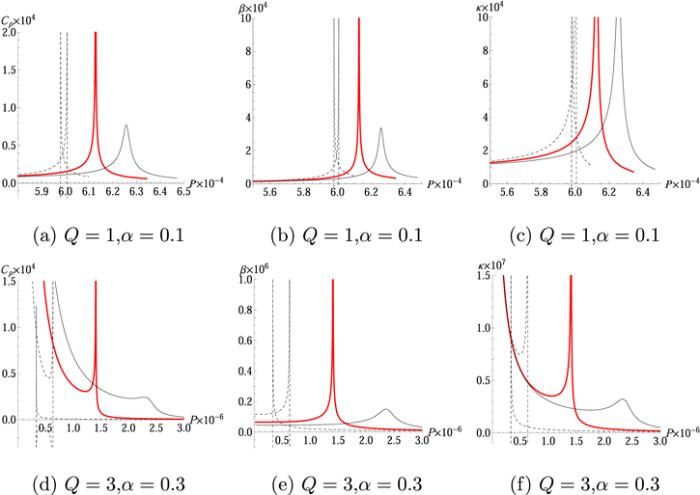New window|Download| PPT slide
Figure 4.Isotherms in the C_P − P, β − P, κ − P diagrams of (n + 1) dimensional dS black holes with NLED of n = 3. Black dashed lines, red thick solid lines and black thin solid lines represent T < T_c, T = T_c and T > T_c, respectively.


The Ehrenfest equations are:(5.4)$\begin{eqnarray}{\left(\frac{\partial P}{\partial T}\right)}_{S}=\frac{{C}_{P}^{2}-{C}_{P}^{1}}{{T}^{{\rm{c}}}{V}^{{\rm{c}}}({\beta }_{q}^{2}-{\beta }_{q}^{1})}=\frac{{\rm{\Delta }}{C}_{P}}{{T}^{{\rm{c}}}{V}^{{\rm{c}}}{\rm{\Delta }}{\beta }_{q}},\end{eqnarray}$(5.5)$\begin{eqnarray}{\left(\displaystyle \frac{\partial P}{\partial T}\right)}_{V}=\displaystyle \frac{{\beta }_{q}^{2}-{\beta }_{q}^{1}}{{\kappa }_{q}^{2}-{\kappa }_{q}^{1}}=\displaystyle \frac{{\rm{\Delta }}{\beta }_{q}}{{\rm{\Delta }}{\kappa }_{q}},\end{eqnarray}$where superscripts 1 and 2 represent phases 1 and 2, respectively, and subscript q represents q remaining unchanged.

According to Maxwell relations:(5.6)$\begin{eqnarray}\begin{array}{l}{\left(\displaystyle \frac{\partial V}{\partial S}\right)}_{P}={\left(\displaystyle \frac{\partial T}{\partial P}\right)}_{S},\\ \left[{\left(\displaystyle \frac{\partial S}{\partial V}\right)}_{T}\right]=\left[{\left(\displaystyle \frac{\partial P}{\partial T}\right)}_{V}\right],\end{array}\end{eqnarray}$(5.7)$\begin{eqnarray}\begin{array}{c}{\left[{\left(\frac{\partial P}{\partial T}\right)}_{S}\right]}^{{\rm{c}}}={\left[{\left(\frac{\partial S}{\partial V}\right)}_{P}\right]}^{{\rm{c}}},\\ {\left[{\left(\frac{\partial S}{\partial V}\right)}_{T}\right]}^{{\rm{c}}}={\left[{\left(\frac{\partial P}{\partial T}\right)}_{V}\right]}^{{\rm{c}}}.\end{array}\end{eqnarray}$Note that the superscript ‘c’ denotes the physical quantities at the critical point. By substituting equation (5.7) into (5.4) and (5.5), we get:(5.8)$\begin{eqnarray}\begin{array}{c}\frac{{\rm{\Delta }}{C}_{P}}{{T}^{{\rm{c}}}{V}^{{\rm{c}}}{\rm{\Delta }}{\beta }_{q}}={\left[{\left(\frac{\partial S}{\partial V}\right)}_{P}\right]}^{{\rm{c}}},\\ \frac{{\rm{\Delta }}{\beta }_{q}}{{\rm{\Delta }}{\kappa }_{q}}={\left[{\left(\frac{\partial S}{\partial V}\right)}_{T}\right]}^{{\rm{c}}}.\end{array}\end{eqnarray}$

From equation (4.1), when the independent variables are (r_c, x), the critical points meet.(5.9)$\begin{eqnarray}\displaystyle \frac{\partial (P,T)}{\partial ({r}_{c},x)}=0.\end{eqnarray}$Moreover,(5.10)$\begin{eqnarray}{\left(\displaystyle \frac{\partial S}{\partial V}\right)}_{P}=\displaystyle \frac{\tfrac{\partial (S,P)}{\partial ({r}_{c},x)}}{\tfrac{\partial (V,P)}{\partial ({r}_{c},x)}},\quad {\left(\displaystyle \frac{\partial S}{\partial V}\right)}_{T}=\displaystyle \frac{\tfrac{\partial (S,T)}{\partial ({r}_{c},x)}}{\tfrac{\partial (V,T)}{\partial ({r}_{c},x)}}.\end{eqnarray}$By substituting equation (5.9) into (5.10), one can get:(5.11)$\begin{eqnarray}{\left(\frac{\partial S}{\partial V}\right)}_{P}^{{\rm{c}}}={\left(\frac{\partial S}{\partial V}\right)}_{T}^{{\rm{c}}}.\end{eqnarray}$To date, we have proved the validity of both Ehrenfest equations at the critical points. Utilizing equations (5.11) and (5.8), the Prigogine-Defay (PD) ratio can be calculated:(5.12)$\begin{eqnarray}{\rm{\Pi }}=\frac{{\rm{\Delta }}{C}_{P}{\rm{\Delta }}{\kappa }_{q}}{{T}^{{\rm{c}}}{V}^{{\rm{c}}}{\rm{\Delta }}{\beta }_{q}^{2}}=1.\end{eqnarray}$

Hence, the phase transition occurring at T = T^c is a second-order equilibrium phase transition.

6. Discussion and conclusion

In this paper, considering the correlation of the thermodynamic quantities respectively corresponding to BEH and CEH of black holes in dS spacetime in the presence of weak NLED and taking the region between BEH and CEH as a thermodynamic system, we discuss the thermodynamic properties of the system and the effect of NLED. When M is regarded as energy (mass) of the thermodynamic system and the volume V = V_c + V₊, entropy S = S_c + S₊ and electric charge Q are taken as the variable of dS spacetime the first law of spacetime has the same form as that of ordinary thermodynamic systems, and the effective thermodynamic quantities P, T and Φ are obtained with the first-order term of nonlinear parameter α preserved. Using these thermodynamic quantities of dS spacetime, we investigate the possible phase transitions and critical behavior of the dS black hole system, and find that nonlinearity parameter α along with electric charge Q influence the possible phase structure of a thermodynamic system. When either or both nonlinear parameter α and electric charge Q are smaller, the system has a minimum volume with physical meaning at a certain temperature T as T < T_c, which is similar to the results of a previous work [42] where α = 0. It is worth noting that as either or both α and Q increase, the minimum volume becomes smaller and smaller. When α and Q reach certain values, the isotherms in the P − V diagrams are very similar to those of the van der Waals gas-liquid system. A more precise conclusion requires further quantitative discussion. The important effect of NLED on the phase structure of AdS black holes has recently been discussed [65]. We also study the phase structure of the system near the critical points by using the Gibbs free energy criterion and Ehrenfest scheme. Although far from the critical points, the system possibly has a different phase structure to that of the van der Waals gas-liquid system. Near the critical points, there are always similar phase transitions of the van der Waals gas-liquid system in the dS black hole system with NLED, which can be seen from the isotherms of the G − P diagrams and C_p − P, β − P and κ − P diagrams. The critical thermodynamic quantities change regularly under the influence of α, which can be seen in table 1, but the critical phase transitions always belong to second-order equilibrium phase transition, which is verified by the Ehrenfest scheme. The possible phase transitions of the dS black hole system with NLED far away from the critical points needs further study.

Acknowledgments

This work is supported by NSFC under Grant No. 11705107, by the doctoral Sustentation Fund of Shanxi Datong University (2015-B-10).

Reference View Option By original order
By published year
By cited within times
By Impact factor

[1]Bekenstein J D 1973 Phys. Rev. D 7 949
DOI:10.1103/PhysRevD.7.949 [Cited within: 2]

[2]Bardeen J M Carter B Hawking S W 1973 Commun. Math. Phys. 31 161
DOI:10.1007/BF01645742 [Cited within: 1]

[3]Hawking S W 1974 Nature 248 30
DOI:10.1038/248030a0 [Cited within: 1]

[4]Kastor D Ray S Traschen J 2009 Classical Quantum Gravity 26 195011
DOI:10.1088/0264-9381/26/19/195011 [Cited within: 1]

[5]Banerjee R Modak S K Samanta S 2011 Phys. Rev. D 84 064024
DOI:10.1103/PhysRevD.84.064024

[6]Banerjee R Roychowdhury D 2011 J. High Energy Phys. 2011 4
DOI:10.1007/JHEP11(2011)004

[7]Banerjee R Ghosh S Roychowdhury D 2011 Phys. Lett. B 696 156
DOI:10.1016/j.physletb.2010.12.010

[8]Cai R-G Cao L-M Li L Yang R-Q 2013 J. High Energy Phys. 2013 5
DOI:10.1007/JHEP09(2013)005

[9]Ma M-S Liu F Zhao R 2014 Classical Quantum Gravity 31 095001
DOI:10.1088/0264-9381/31/9/095001

[10]Zhao R Ma M-S Li H-F Zhang L-C 2013 Adv. High Energy Phys. 2013 371084
DOI:10.1155/2013/371084

[11]Zhao R Zhao H-H Ma M-S Zhang L-C 2013 Eur. Phys. J. C 73 2645
DOI:10.1140/epjc/s10052-013-2645-x

[12]Ma M-S Zhao R 2014 Phys. Rev. D 89 044005
DOI:10.1103/PhysRevD.89.044005

[13]Ma M-S 2014 Phys. Lett. B 735 45
DOI:10.1016/j.physletb.2014.06.006

[14]Zou D-C Zhang S-J Wang B 2014 Phys. Rev. D 89 044002
DOI:10.1103/PhysRevD.89.044002

[15]Zou D-C Liu Y Wang B 2014 Phys. Rev. D 90 044063
DOI:10.1103/PhysRevD.90.044063

[16]Wei S-W Liu Y-X 2013 Phys. Rev. D 87 044014
DOI:10.1103/PhysRevD.87.044014

[17]Wei S-W Liu Y-X 2015 Phys. Rev. Lett. 115 111302
DOI:10.1103/PhysRevLett.115.111302

[18]Liu Y Zou D-C Wang B 2014 J. High Energy Phys. 2014 179
DOI:10.1007/JHEP09(2014)179

[19]Zhao H-H Zhang L-C Ma M-S Zhao R 2015 Classical Quantum Gravity 32 145007
DOI:10.1088/0264-9381/32/14/145007

[20]Li H-F Zhao H-H Zhang L-C Zhao R 2017 Eur. Phys. J. C 77 295
DOI:10.1140/epjc/s10052-017-4831-8 [Cited within: 1]

[21]Hendi S H Dehghani A 2019 Eur. Phys. J. C 79 227
DOI:10.1140/epjc/s10052-019-6743-2

[22]Dehyadegari A Sheykhi A 2020 Phys. Rev. D 102 064021
DOI:10.1103/PhysRevD.102.064021

[23]Mago D Breton N 2020 Phys. Rev. D 102 084011
DOI:10.1103/PhysRevD.102.084011

[24]Ma Y Zhao R Cao S 2016 Eur. Phys. J. C 76 669
DOI:10.1140/epjc/s10052-016-4532-8

[25]Ma Y-B Zhang L-C Liu J Zhao R Cao S 2017 Adv. High Energy Phys. 2017 3812063
DOI:10.1155/2017/3812063 [Cited within: 1]

[26]Hawking S Page D N 1983 Commun. Math. Phys. 87 577
DOI:10.1007/BF01208266 [Cited within: 1]

[27]Hendi S H Mann R B Panahiyan S Eslam Panah B 2017 Phys. Rev. D 95 021501
DOI:10.1103/PhysRevD.95.021501

[28]Dehyadegaria A Sheykhia A Montakhaba A 2017 Phys. Lett. B 768 235
DOI:10.1016/j.physletb.2017.02.064

[29]Zou D-C Yue R Zhang M 2017 Eur. Phys. J. C 77 256
DOI:10.1140/epjc/s10052-017-4822-9 [Cited within: 1]

[30]David A Neiman Y 2020 J. High Energy Phys. 2020 127
DOI:10.1007/JHEP10(2020)127 [Cited within: 1]

[31]Addazi A Marcian A 2020 Int. J. Geom. Methods Mod. Phys. 17 2050007
DOI:10.1142/S0219887820500073 [Cited within: 1]

[32]Akhmedov E T 2014 Int. J. Mod. Phys. D 23 1430001
DOI:10.1142/S0218271814300018 [Cited within: 1]

[33]Chen X 2012 Int. J. Mod. Phys. A 27 1250166
DOI:10.1142/S0217751X12501667

[34]Arraut I 2013 Mod. Phys. Lett. A 28 1350019
DOI:10.1142/S0217732313500193

[35]Ma Y-B Zhang L-C Cao S Liu T Geng S Xu T Liu Y Pan Y 2018 Eur. Phys. J. C 78 763
DOI:10.1140/epjc/s10052-018-6254-6

[36]Ma Y-B Zhang S-X Wu Y Ma L Cao S 2018 Commun. Theor. Phys. 69 544
DOI:10.1088/0253-6102/69/5/544

[37]Ma Y-B Zhang Y Zhao R Cao S Liu T Geng S Liu Y Huang Y 2020 Int. J. Mod. Phys. D 2020 2050108
DOI:10.1142/S0218271820501084 [Cited within: 1]

[38]Zhao R Ma M-S Zhao H-H Zhang L-C 2014 Adv. High Energy Phys. 2014 124854
DOI:10.1155/2014/124854 [Cited within: 1]

[39]Zhang L-C et al. 2014 Eur. Phys. J. C 74 3052
DOI:10.1140/epjc/s10052-014-3052-7 [Cited within: 1]

[40]Zhang L-C Zhao R 2019 Phys. Lett. B 797 134798
DOI:10.1016/j.physletb.2019.134798

[41]Guo X-Y Li H-F Zhang L-C Zhao R 2020 Commun. Theor. Phys. 72 085403
DOI:10.1088/1572-9494/ab8a25

[42]Ma M-S Zhao H-H Zhang L-C Zhao R 2014 Int. J. Mod. Phys. A 29 1450050
DOI:10.1142/S0217751X1450050X [Cited within: 5]

[43]Zhao H-H Zhang L-C Ma M-S Zhao R 2014 Phys. Rev. D 90 064018
DOI:10.1103/PhysRevD.90.064018

[44]Ma M-S Zhang L-C Zhao H-H Zhao R 2015 Adv. High Energy Phys. 2015 134815
DOI:10.1155/2015/134815

[45]Guo X-Y Li H-F Zhang L-C Zhao R 2015 Phys. Rev. D 91 084009
DOI:10.1103/PhysRevD.91.084009 [Cited within: 1]

[46]Kubiznak D Simovic F 2016 Classical Quantum Gravity 33 245001
DOI:10.1088/0264-9381/33/24/245001

[47]Haroon S Hennigar R A Mann R B Simovic F 2020 Phys. Rev. D 101 084051
DOI:10.1103/PhysRevD.101.084051 [Cited within: 1]

[48]Cai R G 2002 Nucl. Phys. B 628 375
DOI:10.1016/S0550-3213(02)00064-0 [Cited within: 1]

[49]Sekiwa Y 2006 Phys. Rev. D 73 084009
DOI:10.1103/PhysRevD.73.084009 [Cited within: 2]

[50]Ma Y-B Zhang Y Zhang L-C Wu L Huang Y Pan Y 2020 Euro. Phys. J. C 80 213
DOI:10.1140/epjc/s10052-020-7763-7 [Cited within: 1]

[51]Chabab M El Moumni H Khalloufi J 2021 Nucl. Phys. B 963 115305
DOI:10.1016/j.nuclphysb.2021.115305 [Cited within: 1]

[52]Born M Infeld L 1934 Proc. R. Soc. London A 143 410
DOI:10.1098/rspa.1934.0010 [Cited within: 1]

Born M Infeld L 1934 Proc. R. Soc. London A 144 425
DOI:10.1098/rspa.1934.0010 [Cited within: 1]

[53]Ayon-Beato E Garcia A 1999 Gen. Relativ. Gravitation 31 629
DOI:10.1023/A:1026640911319 [Cited within: 1]

[54]Ayon-Beato E Garcia A 1999 Phys. Lett. B 464 25
DOI:10.1016/S0370-2693(99)01038-2

[55]De Lorenci V A Klippert R Novello M Salim J M 2002 Phys. Rev. D 65 063501
DOI:10.1103/PhysRevD.65.063501

[56]Dymnikova I 2004 Classical Quantum Gravity 21 4417
DOI:10.1088/0264-9381/21/18/009

[57]Corda C Cuesta H J Mosquera 2010 Mod. Phys. Lett. A 25 2423
DOI:10.1142/S0217732310033633

[58]Corda C Mosquera Cuesta H J 2011 Astropart. Phys. 34 587
DOI:10.1016/j.astropartphys.2010.12.002 [Cited within: 1]

[59]Hoffmann B 1935 Phys. Rev. 47 877
DOI:10.1103/PhysRev.47.877 [Cited within: 1]

[60]Fradkin E Tseytlin A 1985 Phys. Lett. B 163 123
DOI:10.1016/0370-2693(85)90205-9 [Cited within: 1]

[61]Andreev O Tseytlin A 1988 Nucl. Phys. B 311 221
DOI:10.1016/0550-3213(88)90148-4

[62]Leigh R 1989 Mod. Phys. Lett. A 04 2767
DOI:10.1142/S0217732389003099 [Cited within: 1]

[63]Hendi S H Vahidinia M H 2013 Phys. Rev. D 88 084045
DOI:10.1103/PhysRevD.88.084045 [Cited within: 1]

Hendi S H Eslam Panah B Momennia M Panahiyan S 2015 Eur. Phys. J. C 75 457
DOI:10.1103/PhysRevD.88.084045 [Cited within: 1]

Hendi S H Naderi R 2015 Phys. Rev. D 91 024007
DOI:10.1103/PhysRevD.88.084045 [Cited within: 1]

Hendi S H Panahiyan S Momennia M 2016 Int. J. Mod. Phys. D 25 1650063
DOI:10.1103/PhysRevD.88.084045 [Cited within: 1]

Hendi S H Panahiyan S Momennia M Eslam Panah B 2017 Int. J. Mod. Phys. D 26 1750026
DOI:10.1103/PhysRevD.88.084045 [Cited within: 1]

Hendi S H Moradi R Armanfard Z Talezadeh M S 2016 Eur. Phys. J. C 76 263
DOI:10.1103/PhysRevD.88.084045 [Cited within: 1]

Hendi S H Panahiyan S Eslam Panah B Armanfard Z 2016 Eur. Phys. J. C 76 396
DOI:10.1103/PhysRevD.88.084045 [Cited within: 1]

Hendi S H Eslam Panah B Panahiyan S Talezadeh M S 2017 Eur. Phys. J. C 77 133
DOI:10.1103/PhysRevD.88.084045 [Cited within: 1]

Hendi S H Taghadomi Z S Corda C 2018 Phys. Rev. D 97 084039
DOI:10.1103/PhysRevD.88.084045 [Cited within: 1]

Sajadi S N Riazi N Hendi S H 2019 Eur. Phys. J. C 79 775
DOI:10.1103/PhysRevD.88.084045 [Cited within: 1]

[64]Hendi S H Momennia M 2015 Eur. Phys. J. C 75 54
DOI:10.1140/epjc/s10052-015-3283-2 [Cited within: 4]

[65]Momennia M Hendi S HCritical Phenomena and Reentrant Phase Transition of Asymptotically Reissner- Nordström Black Holeshttps://arxiv.org/abs/2101.12039
[Cited within: 3]

[66]Nam C H 2018 Eur. Phys. J. C 78 418
DOI:10.1140/epjc/s10052-018-5922-x [Cited within: 1]

[67]Dolan B P et al. 2013 Phys. Rev. D 87 104017
DOI:10.1103/PhysRevD.87.104017 [Cited within: 3]

[68]Urano M Tomimatsu A 2009 Classical Quantum Gravity 26 105010
DOI:10.1088/0264-9381/26/10/105010 [Cited within: 2]

[69]Soleng Harald H 1995 Phys. Rev. D 52 6178
DOI:10.1103/PhysRevD.52.6178 [Cited within: 1]

[70]Hendi S H 2012 J. High Energy Phys. 2012 065
DOI:10.1007/JHEP03(2012)065 [Cited within: 1]

[71]Gunasekaran S Kubiznak D Mann R B 2012 J. High Energy Phys. 2012 110
DOI:10.1007/JHEP11(2012)110 [Cited within: 1]

[72]Cvetic M Gibbons G W Kubiznak D Pope C N 2011 Phys. Rev. D 84 024037
DOI:10.1103/PhysRevD.84.024037 [Cited within: 1]

[73]Mo J-X Li G-Q Liu W-B 2014 Phys. Lett. B 730 111
DOI:10.1016/j.physletb.2014.01.036 [Cited within: 1]