U. S. Okorie^,1,*, A. N. Ikot², G. J. Rampho³, R. Sever⁴1 Department of Physics, AkwaIbom State University, IkotAkpaden, Uyo. Nigeria
2 Department of Physics, University of Port Harcourt, Choba, Nigeria
3 Department of Physics, University of South Africa, Florida Park 1710, South Africa
4 Department of Physics, Middle East Technical University, 06800, Ankara, Turkey

Corresponding authors: * E-mail:uduakobongokorie@aksu.edu.ng

Received:2019-03-21Online:2019-10-9


Abstract
We discuss the thermodynamic properties of a modified Rosen-Morse potential using the $q$-deformed superstatistics approaches. We obtain the partition function with the help of the generalized Boltzmann factor from the modified Dirac delta distribution and uniform distribution. Other thermodynamic function is obtained for the superstatistics of the two distributions considered. We also discuss our results graphically and obtain the ordinary statistical quantities when the deformation parameter tends to zero.
Keywords： superstatistics;partition function;thermodynamic function


PDF (1041KB)MetadataMetricsRelated articlesExportEndNote|Ris|BibtexFavorite
Cite this article
U. S. Okorie, A. N. Ikot, G. J. Rampho, R. Sever. Superstatistics of Modified Rosen-Morse Potential with Dirac Delta and Uniform Distributions. [J], 2019, 71(10): 1246-1252 doi:10.1088/0253-6102/71/10/1246

1 Introduction

Studies of various interaction potential models became the focus of many researchers after Morse^[1] proposed a three parameter empirical potential for diatomic molecules. This development significantly added value to quantum mechanics, with application in physics and chemistry especially in areas of molecular spectroscopy and dynamics. Since then, many researchers have remodeled this potential by developing different potentials with more parameters. The new models include Rosen-Morse potential,^[2] Manning-Rosen potential,^[3] and may others.^[4-12] Modified and improved versions of the above-mentioned potentials have been studied.^[13-17] This has made practical applications possible in areas like calculation of thermodynamic properties,^[18-21] determination of rotation-vibrational levels for diatomic molecules,^[22-26] and many others.^[27-30]

As an extension to thermodynamic studies, Beck and Cohen^[31] initiated the concept of superstatistics where non-equilibrium systems with complex dynamics in stationary states were considered. Due to the large fluctuations observed and its statistical properties, they obtained different effective statistical mechanical descriptions. Superstatistics is defined as a superposition of two different statistics that describe non-equilibrium systems with a stationary state and parameter fluctuations.^[32] In non-equilibrium statistical mechanics, $\beta$ is not an inverse temperature, thus being seen as a random variable, but regarded as a constant parameter in the case of equilibrium statistical mechanics.^[33]

Many investigates have been conducted on the concept of superstatistics both in the equilibrium and non-equilibrium statistical mechanics regime.^[34-39] Most recently, Sobhani $et al.$^[40] studied the effects of cosmic-string framework on the thermodynamic properties of anharmonic oscillator, within the confinement of the ordinary statistics and the $q$-deformed superstatistics approaches. In another development, $q$-deformed superstatistics of the Schr?dinger equation in the commutative and non-commutative spaces with magnetic field was studied by Sargolzaeipor et al.^[41] In the relativistic regime of quantum mechanics, the superstatistics of the Klein-Gordon equation in deformed formalism for modified Dirac delta distribution was studied.^[42] A deeper understanding of superstatistic of systems involving long range interactions is required, hence further investigations into thermodynamics of such systems are still necessary. The aim of this work is to extend the studies carried out by Dong and Cruz-Irison^[43] on thermodynamic properties of a modified Rosen-Morse potential to the superstatistics regime. These results may be useful to studies of thermal fluctuations in atomic and molecular systems involving long range interactions.

2 Modified Rosen-Morse Potential

Different versions of Rosen-Morse potential have been proposed over the years to describe inter-atomic interactions in diatomic molecules. It begun in 1932 with Rosen and Morse^[2] who proposed a potential model of the form

(1)$$ V_{\rm RM}(r) = V_5\,{\rm tanh}\Big(\frac{r}{a_3}\Big)-V_4\,{\rm sech}^2\Big(\frac{r}{a_3}\Big),$$
where $V_i$ are potential strength parameters and $a_3$ the potential range parameter. This potential is able to describe diatomic and poly-atomic molecules. In an effort to provide a more realistic potential energy model, Zhang et al.^[14] modified the Rosen-Morse potential by considering effects of inner-shell radii of the two constituent atoms in diatomic molecules. The resulting modified Rosen-Morse potential is of the form

(2)$$ {\displaystyle V_{\rm MRM} = D_e\Big(1 - \frac{{\rm e}^{\alpha(r_e-r_{ij})}+1}{{\rm e}^{\alpha(r-r_{ij})}+1} \Big)^2},$$
where $D_e$ is the dissociation energy, $r_e$ the bond length and $r_{ij}=r_e-\sqrt{({KD_e})/({k_e})}$. Here $r_{ij}$ is related to the inner-shell radii of the two atoms, $K$ a dimensionless constant and $k_e$ the equilibrium harmonic vibrational force constant. In Ref. ^[14] the authors established that the modified Rosen-Morse potential (2) is superior to the Morse and conventional Rosen-Morse potentials in fitting experimental data for diatomic molecules.

In another development, Sun et al. ^[44] studied the deformed modified Rosen-Morse potential function proposed by Egrifes et al. ^[45]

(3)$$ V_{\rm DMRM }(r)\!=\!\frac{V_3}{2}[ (1\!+\!{\rm tanh}_q\,{a}_2 r)]\!\! +\!\! \frac{V_2}{4}[\left(1\!+\!{\rm tanh^2}_q\,a_2 r\right)], $$
where tanh$_q\,x$ represents a deformed hyperbolic tangent function and $q$ is the deformation parameter. From hereon $V_i$ and $a_j$ represent potential strength and range parameters, respectively, unless otherwise indicated. By applying the explicit parameters of equilibrium bond length and dissociation energy, Sun et al.^[44] obtained an improved modified Rosen-Morse potential model of the form

(4)$$ {\displaystyle V_{\rm DMRM} = D_e \Big(1-\frac{{\rm e}^{2\alpha r_e}+q}{{\rm e}^{2\alpha r}+q}\Big)^2}\,. $$
It was discovered that the improved expression for the deformed modified Rosen-Morse potential model is defined in terms of four independent parameters. The energy spectra of the modified Rosen-Morse potential have been obtained by different authors using various methods.^[46-50]

Dong and Cruz-Irrison^[43] studied a modified Rosen-Morse potential of the form^[51]

(5)$$ V(r)=-\frac{V_0-V_1\,{\rm sinh}({r}/{a})}{{\rm cosh}^2\left({r}/{a}\right)}. $$
The authors applied the proper quantization rule to obtain the energy spectrum of the modified Rosen-Morse potential of Eq. (5) to be

(6)$$ E_n\!=\!\frac{\hbar^2}{8Ma^2}[G_0\!-\!2n\!-\!1]^2, n\!=\!0, 1, 2, \ldots, \Big[\frac{G_0-1}{2}\Big]\!,$$
where $[f]$ means the largest integer less than $f$, and

(7)$$ G^2_0\!=\!\frac{1}{2}\!+\!\frac{4Ma^2V_0}{\hbar^2}\!+\!\sqrt{\Big(\frac{1}{2}\!+\!\frac{4Ma^2V_0}{\hbar^2}\Big)^2\!\! +\!\!\Big(\frac{4Ma^2V_1}{\hbar^2}\Big)^2}.$$
Thermodynamic properties of this potential (5) were also determined in ordinary statistics. We determine the thermodynamic quantities of this potential in superstatistics, in the next sections.

A number of superstatistics models, based on different generalizations of the Boltzmann factor, exit.^[31] Beck and Cohen^[31] also proved that all these models display a universal behaviour at low energies or low fluctuations. Hence, in this paper we focus only on the modified Dirac delta and the uniform distribution superstatistics, which are particular models of superstatistics.

3 Thermodynamic Functions for Modified Dirac Delta Distribution

In statistical mechanics, the Boltzmann factor $B_o(E)={\rm exp}(-\beta\,E)$, where $\beta=1/(k_BT)$ with $k_B$ as the Boltzmann constant and $T$ temperature, is an essential tool used to determine thermodynamic quantities such as the partition function $Z(\beta)$, free energy $F(\beta)$, mean energy $U(\beta)$, entropy $S(\beta)$, and specific heat capacity $C(\beta)$, for a given system. The thermodynamic quantities are obtained from the partition function as^[52-53]

(8)$$ F=\frac{\ln Z}{\beta}\,,\quad U=-\frac{\partial \ln Z}{\partial\beta}\,, S=k\,\ln Z-k\,\beta\,\frac{\partial \ln Z}{\partial\beta}\,,\quad C=k\beta^2\,\frac{\partial^2 \ln Z}{\partial\beta^2}\,.$$
In superstatistics, the Boltzmann factor is averaged in the form

(9)$$ B(E) = \int^\infty_0{\rm e}^{-\beta^\prime E}\,f(\beta^\prime,\beta)\,{\rm d}\beta^\prime,$$
where $f(\beta^\prime,\beta)$ is a probability density distribution of the random parameter $\beta^\prime$ around the mean $\beta$. In ordinary statistics, the probability density is defined by the Dirac delta function, $f(\beta^\prime,\beta) =\delta(\beta^\prime-\beta)$.^[31]

In $q$-deformed superstatistics, the probability density is modified to a more general form^[41]

(10)$$ f(\beta^\prime,\beta) =c_0\,\delta(\beta^\prime-\beta) + c_1\,\beta^\prime\,\frac{\partial}{\partial \beta^\prime}\,\delta(\beta^\prime-\beta) +c_2\,\beta^{\prime 2}\,\frac{\partial^2}{\partial \beta^{\prime 2}}\,\delta(\beta^\prime-\beta), $$
where $c_i$ are constants determined by requiring $f(\beta^\prime,\beta)$ to conform to certain physical conditions.^[58] Such conditions lead to the values

(11)$$ c_0=1+q,\quad c_1=2q,\quad c_2=\frac{1}{2}q,$$
for the constants, where $q$ is a deformation parameter. The generalized Boltzmann factor for this distribution has the form

(12)$$ B_q(E)=\Big[\,1+\frac{q}{2}\,(\,\beta\,E\,)^2\,\Big]\,{\rm e}^{-\beta \,E}\,,$$
which reduces to the Boltzmann factor for $q = 0$. The $q$-deformed superstatistics reduce to ordinary statistical mechanics when $q\to 0$.^[54-56]

The partition function $Z_q$ in $q$-deformed superstatistics for the modified Rosen-Morse potential (5) is given by

(13)$$ Z_q=\int^\infty_0B_q(E_n)\,{\rm d}n\,.$$
Using Eq. (11) with $E_n$ is given by Eq. (6), the integral evaluates to

(14)$$ Z(\beta) \!=\!\frac{1}{8}\frac{1}{\sqrt{2\beta}}\Big[3q\,\sqrt{\pi}\!+\! 8\sqrt{\pi} \! +\! q{\rm e}^{{({1}/{2})}\beta\lambda^2}\sqrt{2\beta}(\beta\,\lambda^3\! -\! 3\lambda) -3q\sqrt{\pi}{\rm erf}\Big(\frac{\beta\lambda}{\sqrt{2\beta}}\Big)\!-\!8\sqrt{\pi}{\rm erf}\Big(\frac{\beta\lambda}{\sqrt{2\beta}}\Big)\Big],$$
where $\lambda=(G_0-1)/2$ and we have adopted the natural units $\hbar=M=a=1$.

4 Thermodynamic Functions for Uniform Distribution

A uniform distribution $f(\beta)$ of a parameter $\beta>0$ on an interval $[\,a,a+b\,]$ is defined by^[57]

(15)$$ f(\beta)=\frac{1}{b}\,,\quad \beta\in[\,a,a+b\,], $$
and $f(\beta)=0$ elsewhere. This distribution has a mean $\beta_0=a+b/2$ and variance $\langle\,\beta^2\,\rangle-\beta^2_0=b^2/12$. The superstatistics of the uniform distribution is derived from the generalized Boltzmann factor^[58]

(16)$$ B_b(E)\int^\infty_0{\rm e}^{-\beta E}\,f(\beta)\,{\rm d}\beta \approx \Big[\,1+\frac{1}{24}\,(\,b\,E\,)^2\,\Big]\,{\rm e}^{-\beta \,E} ,$$
where the effective Boltzmann factor (16) follows from low energy reduction of the generalized Boltzmann factor. The superstatistics of the uniform distribution reduces to ordinary statistics when $b\to 0$.

The partition function $Z_b$ in the uniform distribution superstatistics for the modified Rosen-Morse potential is given by

(17)$$ Z_b=\int^\infty_0B_b(E_n)\,{\rm d}n, $$
with $E_n$ given by Eq. (6). Evaluating the integral leads to

(18)$$ Z(\beta) =\frac{1}{96\,\beta^2}\frac{1}{\sqrt{2\,\beta}}\Big[\,-3\,b^2\,\sqrt{\pi}- 96\,\beta^2\,\sqrt{\pi} + b^2\,{\rm e}^{\,({1}/{2})\beta\lambda^2}\!\sqrt{2\beta}(\beta\lambda^3\!-\!3\lambda)\!-\!3b^2\sqrt{\pi}{\rm erf}\Big(\frac{\beta\lambda}{\sqrt{2\beta}}\Big)- 96\,\beta^2\,\sqrt{\pi}\,{\rm erf}\Big(\frac{\beta\,\lambda}{\sqrt{2\,\beta}}\Big)\Big].$$
Thermodynamic functions of the modified Rosen-Morse potential using uniform distribution superstatistics can also be obtained using relations given in Eq. (12).

5 Results and Discussion

The variation of the thermodynamic functions with $\beta$ for the modified Dirac delta distribution are shown in Figs. 1$-$3. In these figures, we have considered $\lambda=15$. The partition function $Z$ is plotted in Fig. 1 in terms of $\beta$ for various deformation parameters. It is seen that $Z$ increases monotonically with increasing $\beta$ and $q$. Figure 2 shows the free energy and mean energy variation with $\beta$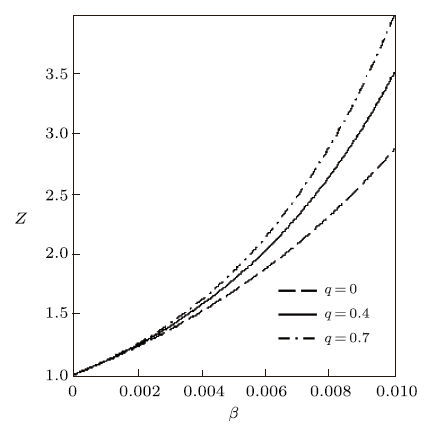

New window|Download| PPT slide
Fig. 1Partition function vs. $\beta$ for different deformation paramters, with the modified Dirac delta distribution.

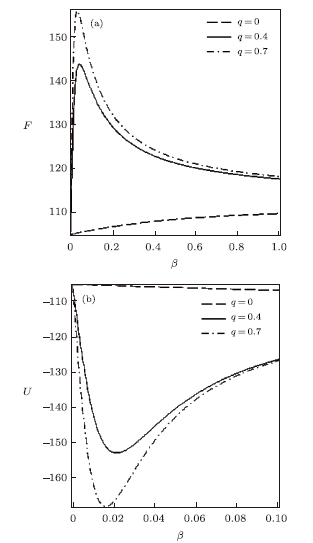New window|Download| PPT slide
Fig. 2Free energy vs. $\beta$ (a) and Mean energy vs. $\beta$ (b), for different deformation paramters, with the modified Dirac delta distribution.

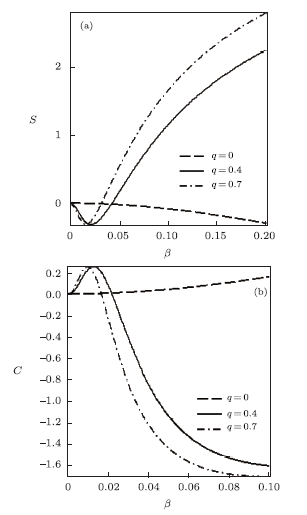New window|Download| PPT slide
Fig. 3Entropy vs. $\beta$ (a) and Specific heat capacity vs. $\beta$ (b), for different deformation paramters, with the modified Dirac delta distribution.



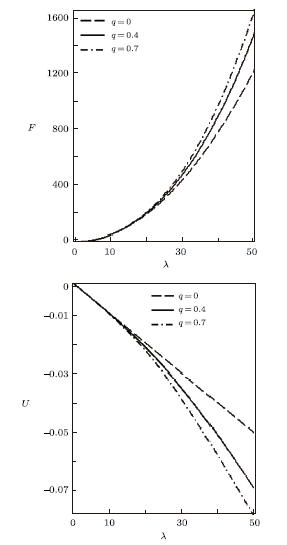
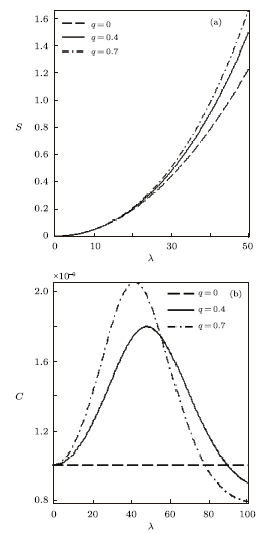
for different deformation parameters. In the absence of $q$, the free energy increases slowly with increase in temperature parameter. Also, there is a sharp increase in the free energy as $q>0$, for increasing $\beta$. As the temperature parameter continues to increase, the free energy drops and later increases slowly. The inverse behaviour of the free energy is observed for the mean energy. Figure 3 shows the behavior of the entropy and specific heat capacity as $\beta$ varies at different deformation parameters. Here, there is a slow decrease in entropy as $\beta$ increases when $q=0$. When $q>0$, the entropy $S$ deceases first and then increases sharply for increasing $\beta$. A reverse behaviour is observed for specific heat capacity, where $C$ increases and later decreases deeply for increasing $\beta$ in the presence of the deformation parameters. In the absence of the deformation parameter $q$, $C$ increases slowly with increasing $\beta$.

We have also plotted the variation of different thermodynamic functions with $\lambda$ at $\beta=0.01$ using the modified Dirac delta distribution, in the absence and presence of deformation parameters as shown in Figs. 4$-$6. We see from these figure that all the thermodynamic functions follow an orderly trend as $\lambda$ increases in the absence and presence of the deformation parameter, except for the specific heat capacity. In Fig. 6, the specific heat capacity decreases very slightly for increasing $\lambda$, in the absence of the deformation parameter. But in the presence of the deformation parameter, $C$ increases tangibly and later decreases for increasing $\lambda$.

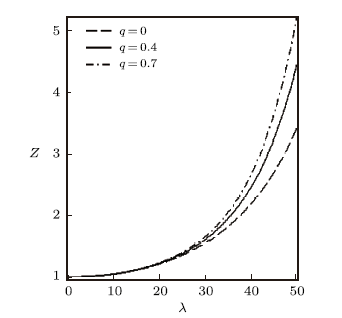New window|Download| PPT slide
Fig. 4Partition function vs. $\lambda$ for different deformation paramters, with the modified Dirac delta distribution.

New window|Download| PPT slide
Fig. 5Free energy vs. $\lambda$ (a) and Mean energy vs. $\lambda$ (b), for different deformation paramters, with the modified Dirac delta distribution.

New window|Download| PPT slide
Fig. 6Entropy vs. $\lambda$ (a) and Specific heat capacity vs. $\lambda$ (b), for different deformation paramters, with the modified Dirac delta distribution.



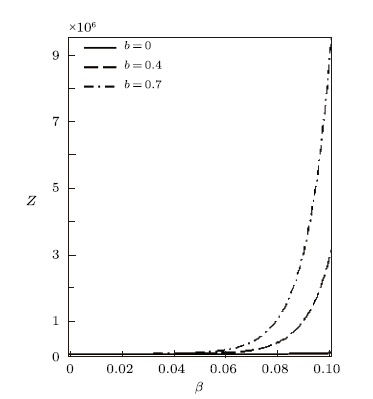
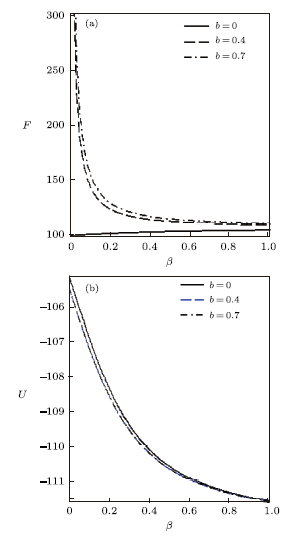
Using the effective Boltzmann factor of the uniform distribution, the partition function variation with $\beta$ for different deformation parameter $b$, and other thermodynamic functions, are shown in Figs. 7$-$9. The thermodynamic functions variation with $\lambda$ for different deformation parameter with uniform distribution are shown in Figs. 10$-$12. The variations of the thermodynamic functions display a similar trend as in the case of the modified Dirac delta distribution. This is expected because of the universal behaviour of superstatistics models at low energies.

New window|Download| PPT slide
Fig. 7Partition function vs. $\beta$ for different deformation paramters, with the uniform distribution.

New window|Download| PPT slide
Fig. 8Free energy vs. $\beta$ (a) and Mean energy vs. $\beta$ (b), for different deformation paramters, with the uniform distribution.

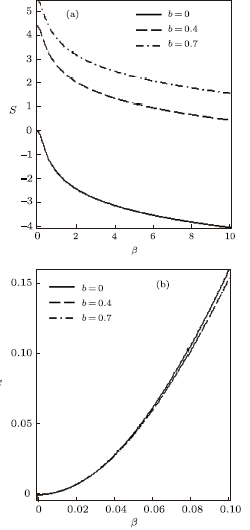New window|Download| PPT slide
Fig. 9Entropy vs. $\beta$ (a) and Specific heat capacity vs. $\beta$ (b), for different deformation paramters, with the uniform distribution.

New window|Download| PPT slide
Fig. 10Partition function vs. $\lambda$ for different deformation paramters, with the uniform distribution.

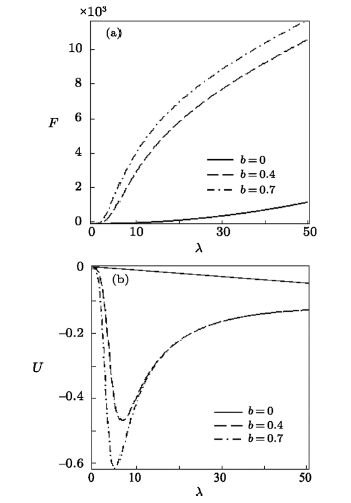New window|Download| PPT slide
Fig. 11Free energy vs. $\lambda$ (a) and Mean energy vs. $\lambda$ (b), for different deformation paramters, with the uniform distribution.

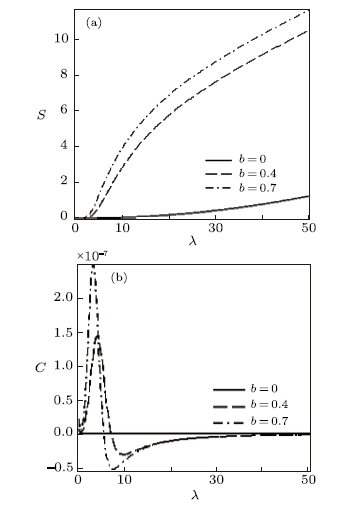New window|Download| PPT slide
Fig. 12Entropy vs. $\lambda$ (a) and Specific heat capacity vs. $\lambda$ (b), for different deformation paramters, with the uniform distribution.

6 Concluding Remarks

In this study, the energy spectra obtained for modified Rosen-Morse potential have been adopted to calculate the partition function, with the help of the generalized Boltzmann factor for both modified Dirac delta distribution and uniform distribution. Other thermodynamic functions such as free energy $F$, mean energy $U$, entropy $S$, and specific heat capacity $C$ have been obtained using these superstatistics approaches. The variations of these thermodynamic functions with $\beta$ and $\lambda$ in the absence and presence of the deformation parameters have been displayed graphically and discussed extensively. Specifically, interesting manifestations are obtained in the presence of the deformation parameter for uniform distribution. Also, our results agree with the ordinary statistics in the absence of the deformation parameter ($q=b=0$) using both superstatistical approaches.

Reference View Option By original order
By published year
By cited within times
By Impact factor

[1] M. Morse , Phys. Rev. 34(1929) 57.
[Cited within: 1]

[2] N. Rosen and P.M. Morse, Phys. Rev. 42(1932) 210.
[Cited within: 2]

[3] M.F Manning .and N. Rosen, Phys.Rev. 44(1933) 951.
[Cited within: 1]

[4] G. Poschl and E. Teller,, Z. Phys. 83(1933) 143.
[Cited within: 1]

[5] Z.H Deng . and Y. P. Fan , Shandong Univ. J. 7(1957) 162.


[6] A.A Frost .and B. Musulin, J.Chem. Phys. 22(1954) 1017.


[7] T. Tietz, J. Chem. Phys . 38(1963) 3036.


[8] M. Badawi, N. Bessis, G. Bessis , J. Phys. B At Mol. Phys. 5(1972) L157.


[9] D. Shioberg , Mol. Phys. 59(1986) 1123.


[10] H. Wei , Phys. Rev. A 42(1990) 2524.


[11] A. A. Zavitsas, J. Am. Chem . Soc. 113(1991) 4755.


[12] J. X. Sun , Acta. Phys. Sin. 48(1999) 1992.
[Cited within: 1]

[13] C. S. Jia, Y. F. Diao, X. J. Liu , et al., J. Chem.Phys. 137(2012) 014101.
[Cited within: 1]

[14] G. D. Zhang, J. Y. Liu, L. H. Zhang , et al., Phys. Rev. A 86( 2012) 062510.
[Cited within: 2]

[15] C. S. Jia, T. Che, L. Z. Yi, S. R. Lin, J. Math. Chem . 51(2013) 09.


[16] O. Mustafa , Phys. Scri. 90(2015) 065002.


[17] P. Q. Wang, L. H. Zhang, C. S. Jia, J. Y. Liu, J. Mol. Spectrosc . 278(2012) 23.
[Cited within: 1]

[18] C. S. Jia, L. H. Zhang, C. W. Wang , Chem. Phys. Lett. 667(2017) 211.
[Cited within: 1]

[19] U. S. Okorie, E. E. Ibekwe, A. N. Ikot , et al., J. Kor.Phys. Soc. 79(2018) 1211.


[20] U. S. Okorie, A. N. Ikot, M. C. Onyeaju, E. O. Chukwuocha , Rev. Mex. De Fis. 64(2018) 608.


[21] U. S. Okorie, A. N. Ikot, M. C. Onyeaju, E. O. Chukwuocha, J. Mol. Mod . 24(2018) 289.
[Cited within: 1]

[22] C. S. Jia, J. W. Dai, L. H. Zhang , et al., Phys. Lett. A 379 ( 2015) 137.
[Cited within: 1]

[23] Y. Sun, G. D. Zhang, C. S.Jia , Chem. Phys. Lett. 636(2015) 197.


[24] A. K. Roy , Int. J. Quant. Chem. 114(2014) 383.


[25] A. N. Ikot, H. Hassanabadi, H. P. Obong , et al., Chin. Phys. B 23( 2014) 120303.


[26] J. J. Pena, J. Garcia-Martinez, J. Garcia-Ravelo, J. Morales , Int. J. Quantum Chem. 115(2015) 158.
[Cited within: 1]

[27] O. Mustafa, J. Phys . B: At. Mol.Opt. Phys. 48(2015) 065101.
[Cited within: 1]

[28] A. N. Ikot, E. J. Ibanga, H. Hassanabadi , Int. J. Quant. Chem. 116(2016) 81.


[29] G. Ovando, J. J. Pena, J. Morales , Theor. Chem. Acc. 135(2016) 62.


[30] H. Yanar, O. Aydogdu, M. Salti , Mol. Phys. 114(2016) 3134.
[Cited within: 1]

[31] C. Beck and E. G. D. Cohen , Physica A 322(2003) 267.
[Cited within: 4]

[32] C. Beck , Phys. Rev. Lett. 87(2001) 180601.
[Cited within: 1]

[33] C. Beck , Physica A 331(2004) 173.
[Cited within: 1]

[34] M. Ausloos and K. Ivanova , Phys. Rev. E 68(2003) 046122.
[Cited within: 1]

[35] C. Beck , Continuum Mech. Thermodyn. 16(2004) 293.


[36] C. Beck and E. G. D. Cohen , Physica A 344(2004) 393.


[37] C. Tsallis and A. M. C. Souza , Phys. Rev. E 67(2003) 026106.


[38] C. Beck , Europhys. Lett. 64(2003) 151.


[39] A. Reynolds , Phys. Rev. Lett. 91(2003) 084503.
[Cited within: 1]

[40] H. Sobhani, H. Hassnanbadi, W. S. Chung , Euro. Phys. J. C 78(2018) 106.
[Cited within: 1]

[41] S. Sargolzaeipor, H. Hassanabadi, W. S. Chung , Euro. Phys. J. Plus 133(2018) 5.
[Cited within: 2]

[42] S. Sargolzaeipor, H. Hassanabadi, W. S. Chung , Mod. Phys. Lett. A 33(2018) 1850060.
[Cited within: 1]

[43] S.H Dong . and M. Cruz-Irison , J.Math. Chem. 50(2012) 881.
[Cited within: 2]

[44] Y. Sun, S. He, C. S. Jia , Physica Scripta. 87(2013) 025301.
[Cited within: 2]

[45] H. Egrifes, D. Demirhan, F. Buyukkilic , Physica Scripta. 59(1999) 90.
[Cited within: 1]

[46] X. Y. Gu, S. H. Dong, Z. Q. Ma, J. Phys . A: Math. Theor. 42(2009) 035303.
[Cited within: 1]

[47] H. M. Tang, G. C. Liang, L. H. Zhang, et al., Can. J. Chem. 92 , (2014) 341.


[48] X. T. Hu, L. H. Zhang, C. S. Jia , Can. J. Chem. 92(2014) 386.


[49] J. Y. Liu, X. T. Hu, C. S. Jia , Can. J. Chem. 92(2014) 40.


[50] M. S. Tan, S. He, C. S. Jia , Eur. Phys. J. Plus 129(2014) 264.
[Cited within: 1]

[51] V. G. Bagrov and D. M. Gitman , Exact Solution of Relativistic Wave Equations,Kluwer,Dordrecht ( 1990).
[Cited within: 1]

[52] A. N. Ikot, B. C. Lutfuoglu, M. I. Ngwueke , et al., Eur. Phys. J. Plus 131(2016) 419.
[Cited within: 1]

[53] A. N. Ikot, E. O. Chukwuocha, M. C. Onyeaju , et al., Pramana J. Phys. 90(2018) 22.
[Cited within: 1]

[54] C. Tsallis , Braz. J. Phys. 29(1999) 1.
[Cited within: 1]

[55] J. Luczka and B. Zaborek , Acta Phys. Pol. B 35(2004) 2151.


[56] N. Mordant, A. M. Crawford, E. Bodenschatz , Physica D 193 (2004) 245.
[Cited within: 1]

[57] S. Sargolzaeipor, H. Hassanabadi, W. S. Chung , Mod. Phys. Lett. A 34 (2019) 1950023.
[Cited within: 1]

[58] S. Sargolzaeipor, H. Hassanabadi, W. S. Chung , Euro. Phys. J. Plus 133 (2018) 125.
[Cited within: 2]