陈娅昵, 孟文静, 钱有华^,2)浙江师范大学数学与计算机科学学院,浙江金华 321004

FIXED POINT CHAOS AND FOLD/FOLD BURSTING OF A CLASS OF DUFFING SYSTEMS AND THE MECHANISM ANALYSIS ¹⁾

Chen Yani, Meng Wenjing, Qian Youhua^,2)College of Mathematics and Computer Science,Zhejiang Normal University,Jinhua 321004,Zhejiang,China

通讯作者: 2)钱有华,副教授,主要研究方向：非线性动力学. E-mail:qyh2004@zjnu.edu.cn

收稿日期:2020-04-1接受日期:2020-04-1网络出版日期:2020-09-18

基金资助:

1)国家自然科学基金.11572288 浙江省自然科学基金.LY20A020003

Received:2020-04-1Accepted:2020-04-1Online:2020-09-18
作者简介 About authors


摘要
本文主要探究了一类含有两个慢变量的双稳态 Duffing 型系统,通过时间历程图、相图、分岔图等对系统进行数值模拟,然后从理论上分析不同参数下系统的动力学机理. 首先,研究发现当振幅参数取值大于 1 时,系统会表现出不动点混沌现象,并进一步解释了产生不动点混沌的机理. 其次, 介绍了参数空间中的簇发振荡现象,即系统穿过鞍结曲面的一侧到达另一侧所发生的行为,这里也称为鞍结簇发振荡. 事实上,当系统穿过鞍结曲面的时候,它的平衡点个数发生了变化. 然后,使用纵向抛物线路径说明了 Fold/Fold 簇发振荡产生的机理,发现无论常系数项和振幅的取值为多少,只要满足一定的关系,总会产生 Fold/Fold 簇发振荡,之后使用线性路径阐明了新增常系数项会使得系统发生簇发振荡的原因. 并且发现路径与鞍结曲面交点的位置会影响簇发振荡的对称性;路径的跨度会影响簇发振荡的大小. 最后,使用多拐折曲线路径讨论当两个激励项存在 $n$ 倍关系时系统产生的现象. 结果表明当 $n=3$ 时,常系数项的变化会使得系统表现出不同重数的 Fold/Fold 簇发振荡,最高可达到三重簇发振荡. 并且发现在理想状况下如果可以找到一条路径可以分割为 $n$ 段,并且每一段都会与鞍结曲面有交点,那么会产生 $n$ 重 Fold/Fold 簇发振荡.
关键词： Fold/Fold 簇发振荡;Duffing 系统;参数空间;不动点混沌

Abstract
In this paper, a class of bistable Duffing type system with two slow variables under new materials is explored. The system is simulated by time history diagram, phase diagram and bifurcation diagram, then the dynamic mechanism of the system under different parameters is analyzed theoretically. Firstly, this manuscript describes that when the amplitude parameter value is greater than 1, the system may exhibit fixed point chaos and explains the reason of fixed point chaos. Secondly, this manuscript introduces the phenomenon of Fold/Fold bursting in parameter space which is caused by the movement of the system from one side of the saddle-node surface to the other side. We also call it saddle-node bursting. In fact, when the system passes through the saddle-node surface, the number of equilibrium points changes. Then this manuscript uses the path of longitudinal parabolic to explain the mechanism of Fold/Fold bursting. And it is found that regardless of the value of constant coefficient term and amplitude, as long as a certain relationship is satisfied, there will always be Fold/Fold bursting. Next this manuscript uses the linear path to discuss the influence of newly added constant coefficient term. It is found that the position where the path intersects the saddle-node surface will affect the symmetry of the bursting, and the span of the path will affect the magnitude of the bursting oscillation. Finally, this manuscript uses the multiple inflection curve path to discuss the phenomenon when two incentive terms have specific relation. When $n=3$, the change of the constant coefficient term will make the system show Fold/Fold bursting with different times, and the maximum can reach triple bursting. Moreover, it is found that if you can find a path that can be divided into $n$ segments, and each segment will have an intersection with the saddle surface, then $n$ times Fold/Fold bursting will occur.
Keywords：Fold/Fold bursting;Duffing system;parameter space;fixed point chaos


PDF (20033KB)元数据多维度评价相关文章导出EndNote|Ris|Bibtex收藏本文
本文引用格式
陈娅昵, 孟文静, 钱有华. 一类 Duffing 型系统的不动点混沌和Fold/Fold 簇发现象及机理分析 ¹⁾. 力学学报[J], 2020, 52(5): 1475-1484 DOI:10.6052/0459-1879-20-098
Chen Yani, Meng Wenjing, Qian Youhua. FIXED POINT CHAOS AND FOLD/FOLD BURSTING OF A CLASS OF DUFFING SYSTEMS AND THE MECHANISM ANALYSIS ¹⁾. Chinese Journal of Theoretical and Applied Mechanics[J], 2020, 52(5): 1475-1484 DOI:10.6052/0459-1879-20-098

引言

在非线性动力学中有一类问题中有一些参数随着时间的推移而缓慢变化,造成了在同一个系统中存在两个或两个以上的时间尺度,这也被称为多时间尺度问题^[1-3]. 多时间尺度问题具有广泛的应用背景,包括生物网络系统中的神经放电模式^[4]、金属氧化反应等. 但由于其复杂性,很难用准静态解、奇异摄动法等来研究其解析解,一般只能得到近似的数值解,因此数值分析的方法应运而生. 通过数值模拟发现多时间尺度相比于单一的时间尺度具有更复杂的系统表现,如混合模式振荡^[5-10].

混合模振荡又称簇发振荡,是一种复杂的振荡模式,它的特征是小振幅振荡和大振幅振荡的结合,可以用快速和缓慢的子系统来描述. 当系统处于小幅度振荡时,系统表现出静息状态;当系统经过相关分岔点时会突然失去平衡,进入大幅度振荡,此时系统表现出活跃态. 随着慢变量的引导,系统又会从不平衡走向平衡,由活跃态走向静息态,振幅也从大幅度的振荡变为小幅度的振荡,而一旦达到分岔点时,会类似地出现之前的现象. 此时的系统就是在静息态和活跃态之间不停地跳跃,称为混合模式振荡.

混合模式振荡也会出现在快慢耦合系统中. Rinzel^[11]提出了利用冻结子系统的方法来解释混合模式振荡的想法,这就是我们所知道的快慢分析,它的应用取得了很大的效果. 在 Izikevich^[12]的工作中,创建了混合模式振荡的分类. 在快速慢分析的基础上,在研究多时间尺度的系统中,众多****研究了快慢系统中混合模式振荡出现的机理. 例如：Qian 等^[13]研究了含两个慢变量的耦合系统的混合振荡模式,讨论了不同时间尺度下的系统动力学行为以及时滞对系统行为的影响;Qian 等^[14]研究了单参数激励和双参数激励下的两自由度非线性耦合的 Duffing 方程,利用快慢分析方法对耦合系统进行离散,通过数值模拟发现系统会产生簇发振荡现象,并讨论了相关的振动动力学行为;Han 等 ^[8]使用缓慢参数和外部激励来模拟两个缓慢变化的周期参数,并研究了不同时间尺度下的动态响应问题;Zhang 等^[15]使用双稳态不对称复合材料层合板对两个慢参数激励的系统以及动态跳跃现象进行了实验和理论分析,提出了一种利用时变原理曲率描述双稳态非对称层合板动态切换现象的新方法;Chen 等^[16]展现了具有周期激励的耦合振荡器的弛豫振荡,以典型分叉模式为例,讨论了外激励变化对动态响应的影响,在静止状态和重复尖峰振荡之间交替可以得到簇发振荡;Li 等^[17]在具有缓慢周期性参数激励的 Duffing 振荡器中,通过数值模拟发现了一种称为周期混沌运动的新型响应,称为不动点混沌;Lin 等^[18]研究了一种简单的三元记忆电路周期簇发振荡的分岔机理;Wang 等^[19]也研究了简单的自主记忆电路,分析了其对称性、耗散性和平衡稳定性. 苟向锋等^[20]基于参数平面的耦合研究了单自由度齿轮传动系统安全盆侵蚀与分岔;毕勤胜等^[21-22]讨论了不同系统的簇发振荡及其分岔行为;张正娣等^[23-24]对于几类非光滑的系统进行了簇发振荡分析;Han 等^[25-26]对 Duffing 系统进行研究,发现了时滞周转引起的新的簇发振荡类型,叉式翻转迟滞簇发振荡以及复合叉式迟滞簇发振荡.

本文通过构造参数空间来解释簇发现象产生的机理. 下面简述这一理论的发展过程.

Rinzel 等^[11,27]是第一个利用 "解剖" 方法来研究 快慢系统的簇发振荡,他认为簇发振荡取决于所 遇到的分岔,即通过研究慢变量经历不同分岔时产生的跃迁可以对簇发振荡行为进行分类. 1995 年,Bertram 等^[28]研究了 Chay-Cook 模型的两参数分岔图,他们发现这可以看成是三参数焦点型退化 Takens-Bogdanov 奇点展开的一个切片. 2000 年,Izhikevich^[12]发现簇发振荡开始和偏移时的不同位置会导致动态响应发生质的变化. 并且基于起始/偏移分岔对来编译可能的簇发振荡分类法. 2008 年,Stern 等^[29]发现其中一种亚临界 Hopf 簇发振荡,它不在余维三奇点展开中出现. 2016 年, Osinga 等^[30],研究了生物学和数学的交叉流：伪高原簇发的余维. 在一个立方 Liénard 系统的分岔中,发现 Fold/homoclinic 簇发振荡与 Fold/subHopf 簇发振荡具有非常相似的潜在分岔图,但它不是余维三的,因此可以预测具有余维四. 最后通过展示了一个双退化的 Bodganov-Takens 点的部分展开中识别出一个三维切片,并证明这个余维四奇异性导致了几乎所有已知的簇发振荡类型. 2017 年,Saggio 等^[31]提出几乎可以产生所有种类的簇发振荡模型,模型包含两个子系统,对于快子 系统,可使用高余维奇点平面展开. 在分岔图中,可以确定簇发振荡所需穿过正确分岔序列的路径. 而慢子系统沿路径来回引导快子系统.

本文在文献^[15] 的基础上,针对一类含有两个慢变量的系统使用数值模拟的方法研究了在参数较大的情况下产生的分岔. 本文使用数值模拟和基于参数空间的理论分析研究了在参数较小的情况下系统存在双稳态和 Fold/Fold 簇发振荡现象,并为控制该系统得到簇发振荡现象提供了参考数据. 其中对参数大小的界定标准如下：以 1 为界,若参数大于 1,认为它是较大的;小于 1,则认为它是较小的.

1 不动点混沌

在这一部分我们主要分析了一类含有两个慢变量的 Duffing 系统在参数较大情况下的动力学行为. 通过数值模拟,得到了系统的时间历程图和相位图. 研究表明系统存在不动点混沌,并且随着参数的变化,不动点混沌会表现为单支存在或者双支合并形式. 之后本文进一步解释了系统不动点混沌产生的机理.

考虑如下系统

(1) $ \left.\begin{array}{l} \dot x = y \\ \dot y = - x^3 + (\mu _2 + a)x + \mu _1 - yv \end{array}\right\} $
其中,$\mu _1 = \beta _1 \cos (\omega _1 t)$, $\mu _2 = \beta _2 \cos (\omega _2 t)$. $\beta_1$, $\beta _2 $ 表示振幅,$v$ 表示阻尼系数,$\omega _1$, $\omega _2 $ 表示频率,$v, \beta _1 , \beta _2$ 被认为是扰动参量. 文献^[15] 对该系统在实验上发现了双稳态现象,并从理论上解释了双稳态现象及动态跳跃现象.

首先假设 $a = 0$, 则系统 (1) 变为

(2) $ \left.\begin{array}{l} \dot x = y \\ \dot y = - x^3 + \mu _2 x + \mu _1-yv \end{array}\right\} $
对于系统 (2),为不失一般性, 选择 $\beta _1 ,\beta _2 > 0$ 并假设 $\omega _1 = \omega _2 = \omega $. 由于刚度和外激励项是周期性时变的,因此它在一个半周期是正的,在另一个半周 期是负的. 由于刚度和外激励项的周期性时变性,导致系统产生高度复杂和不寻常的动态响应,包括周期混沌运动的新现象.

固定 $\beta _1 = 0.1$,$\beta _2 = 6.257 5$,$v = 0.3$ 时,当 $\omega = 0.7$ 和 $\omega = 0.135 5$ 时,混沌吸引子存在,如图 1 和图 2 所示.

图 1

新窗口打开|下载原图ZIP|生成PPT
图 1$\beta _1 = 0.1$, $\beta _2 = 6.257 5$, $v = 0.3$,当 $\omega$ 分别取 0.7 和 0.135 5 时系统 (2) 表现出混沌吸引子

Fig. 1$\beta _1 = 0.1$, $\beta _2 = 6.257 5$, $v = 0.3$,system (2) shows chaotic attractors when $\omega = 0.7$ and $\omega = 0.135 5$, respectively

图 2

新窗口打开|下载原图ZIP|生成PPT
图 2$\omega = 0.1355$, $\beta _1 = 0.1$, $v = 0.3$,系统 (2) 在 $\beta _2 = 2.0$ 时表现出单独左支行为,在 $\beta _2 = 2.7$ 时表现出单独右支行为

Fig. 2$\omega = 0.1355$, $\beta _1 = 0.1$, $v = 0.3$,system (2) shows the behaviors of left branch alone with $\beta _2 = 2.0$ and right branch alone with $\beta _2 = 2.7$



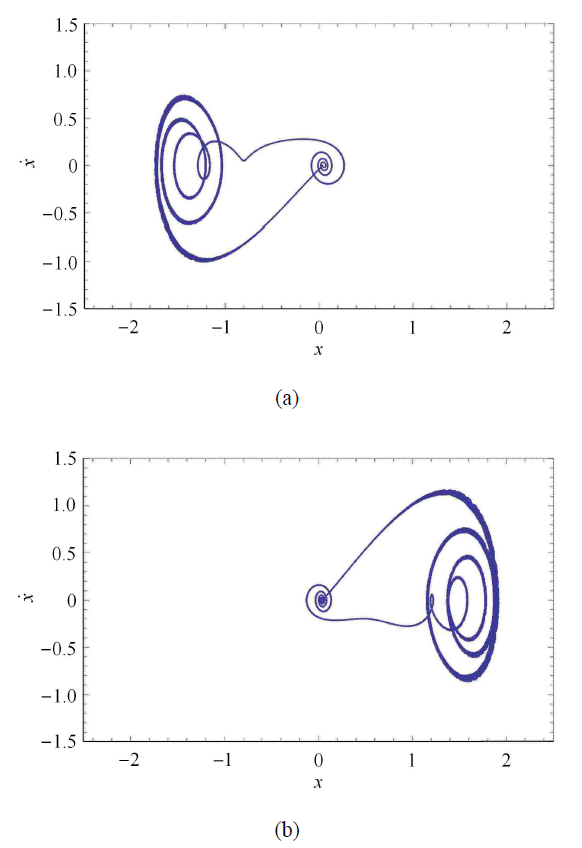
文献^[17] 介绍了不动点混沌,这是一种新的混沌现象. 它的典型特点是存在一个不动点. 对于 $\omega \ll 1$,系统具有两个不同的时间尺度,一个是快时间尺度,一个是慢时间尺度. 当 $\omega = 0.135 5$, $\beta _1 = 0.1$,$\beta _2 = 3.3$,$v = 0.3$ 时,两个混沌吸引子共存. 这些吸引子的最大 Lyapunov 指数是正的,表明它们确实是混沌的. 而当 $\beta _2 $ 改变时,两个共存的吸引子会分开,出现单独左支或者单独右支的情况. 下面保持其他参数不变,而只改变 $\beta _2 $ 分别为 2.0 和 2.7.

为进一步研究不动点混沌的机理,讨论了快慢系统的分岔行为. 对于 $\omega \ll 1$,周期激励 $\mu _1 =$ $ \beta _1 \cos (\omega t)$, $\mu _2 = \beta _2 \cos (\omega t)$ 分别在 $[ - \beta _1 ,\beta _1 ]$ 和 $[ - \beta _2 ,\beta _2 ]$ 之间变化缓慢. 将 $\mu _1$, $\mu _2 $ 近似地视为一个常数,并将 $\cos (\omega t)$ 用作自治系统的分岔参数.

系统 (2) 的静态平衡解满足

(3) $ - x^3 + [\beta_2\cos (\omega t)]x = 0 $
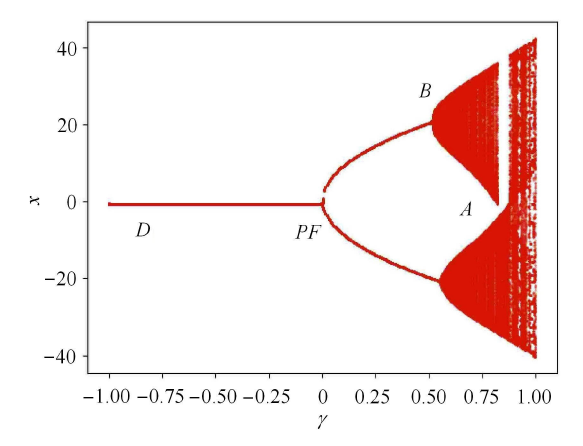
当 $\cos (\omega t) < 0$ 时,系统只有一个稳定平衡点;当 $\cos (\omega t) > 0$ 时,平衡解由于发生分岔而失稳,产生了一对对称稳定的分岔解,$x = \pm \sqrt {\beta _2 \cos (\omega t)} $;因此 pitchfork 分岔发生在 $\cos (\omega t) = 0$,记为 $PF$. 图 3 给出了系统的离散分岔图.

图 3

新窗口打开|下载原图ZIP|生成PPT
图 3$\beta _1 = 0.1$, $\beta _2 = 6.257 5$, $\omega = 0.135 5$, $v = 0.3$ 时系统 (2) 的离散分岔图

Fig. 3Discrete bifurcation diagram of system (2) when $\beta _1 = 0.1$, $\beta _2 = 6.257 5$, $\omega = 0.135 5$, $v = 0.3$



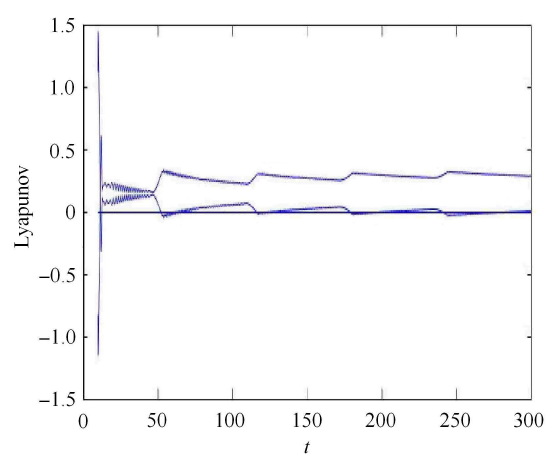
取 $\beta _1 = 0.1$, $\beta _2 = 6.257 5$, $\omega = 0.135 5$, $v = 0.3$ 来说明不动点混沌机理. 图 3 显示了规则运动和混沌变化的过程. 与上图对应,我们可以看到,$A$ 所在的地方是未被红色区域覆盖,此时系统的状态是静默的. 在 $A$ 的右侧,已被红色覆盖,$A$ 可以跳跃到上分支或者是下分支,变成了尖峰状态. 因此点 $A$ 是静止状态到尖峰状态的转换点. 随着激励的增大,系统在稳定分支的周围拥有尖峰状态. 当激励达到最大值,它们开始改变方向,并在同一稳定分支上移动,随着激励的减小而停留在静默状态. 当轨迹到达分岔点 $PF$ 时,它可能被左稳定点吸引,并开始接近稳定平衡点. 激励达到最小值,它处于 $D$ 点. 此时,它重新向 $A$ 点移动,通过平衡点 (0,0). 然后平衡点 (0,0) 变得不稳定,逐渐到达 $B$ 点. 在轨迹集合中显示的随机性很可能是因为当系统被吸引到稳定平衡 (0,0) 时,响应变量 $x$ 和 $y$ 是非零的并且很小. 在数值计算或实验中,这样的小数字实际上是随机的. 因此,离开平衡 (0,0) 的轨迹在每个周期都有不同的初始条件. 对该系统中的初始条件的敏感性显然是混沌的一种性质,所以构成了一个混沌运动. 图 4 中显示的最大 Lyapunov 指数从 0.3 变化到小于 0.01. 它显示了系统丰富的动力学性质,暗示了系统的复杂性. 因此,我们对该系统进行了一系列的数值研究. 图 4 也说明了这一点,当最 大Lyapunov 指数大于 0 时,表示系统存在混沌现象.

图 4

新窗口打开|下载原图ZIP|生成PPT
图 4$\beta _1 = 0.1$, $\beta _2 = 6.257 5$, $\omega = 0.135 5$, $v = 0.3$ 时系统 (2) 的最大 Lyapunov 指数

Fig. 4Maximum lyapunov exponent of system (2) when $\beta _1 = 0.1$, $\beta _2 = 6.257 5$, $\omega = 0.135 5$, $v = 0.3$

2 Fold/Fold 簇发振荡

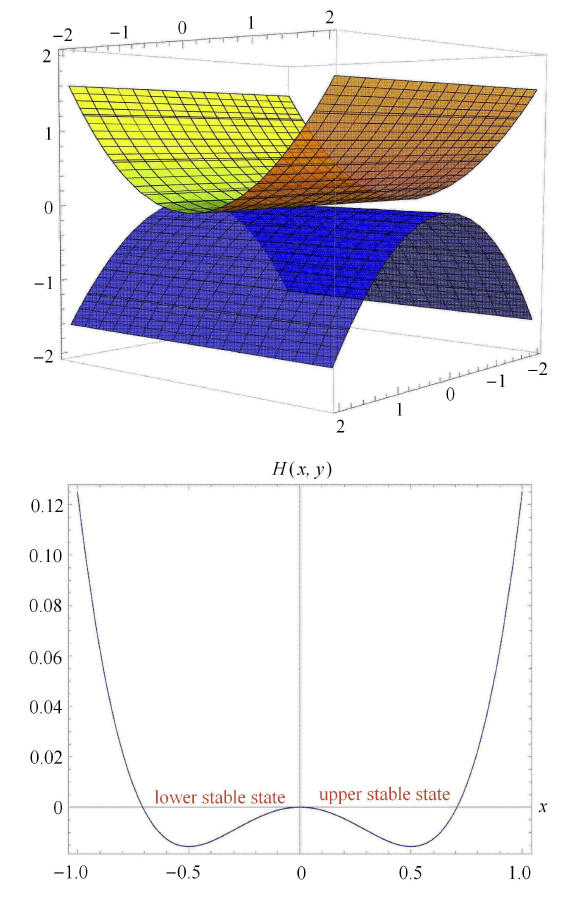
在这一部分,主要考虑系统 (2) 中当两个慢变量的振幅 $\beta _1 < 1$, $\beta _2 < 1$ 时系统簇发振荡现象. 系统 (2) 来源于文献^[15]. 文献^[15] 从理论和实验两个方面研究了双稳态不对称层合板在外激励的作用下的动态跳跃现象和非线性振动行为,并且发现双稳态不对称层合板在位于中间的不稳定平衡位置时附近会有两个稳定态振动,分别位于上稳定态分支或下稳定态分支. 如图 5 所示. 当系统从一稳定分支跳跃到另一稳定分支时,它就会产生出文献^[15] 所说的动态跳跃现象,从理论上分析也就是 Fold/Fold 簇发振荡. 本文对文献^[15] 中的系统考虑了不同参数的情况.

图 5

新窗口打开|下载原图ZIP|生成PPT
图 5双稳态不对称层合板与动态跳跃现象

Fig. 5Bistable unsymmetrical composite square panel and dynamic fracture phenomenon



在快慢系统中,会产生一系列的簇发振荡、尖峰和静止的周期性变化. 其中,一个或多个慢变量通过一系列分岔传递快变量,这些 分岔介入导致振荡和稳态之间的转换. 在以往的研究中发现折叠分岔是余维一的,而两个折叠分岔曲面相交会形成二余维的尖点,如图 6 所示. 本文通过折叠分岔来解释 Fold/Fold 簇发振荡现象.

图 6

新窗口打开|下载原图ZIP|生成PPT
图 6以单位球面为边界的分岔

Fig. 6Bifurcation with unit sphere as boundary



此时系统写作快慢系统形式

(4) $ \left.\begin{array}{l} \dot x = y \\ \dot y = - x^3 + \mu _2 x + \beta _1 z- yv \\ z = \omega f(z) \end{array}\right\} $
其中

(5) $ z = \cos (\omega t) , \ \ \ f(z) = - \sin (\omega t) $
现在将系统视为一个质点,它受到作用在两 个垂直方向 $\mu _1$,$\mu _2 $ 轴上的简谐运动,此时这两个分振动可以表示为

(6) $ \left.\begin{array}{l} \mu _1 = \beta _1 \cos (\omega _1 t + \varphi _1 ) \\ \mu _2 = \beta _2 \cos (\omega _2 t + \varphi _2 ) \end{array}\right\} $
其中,$\omega _1 :\omega _2 = 1:2$,$\varphi _1 - \varphi _2 = \pi $. 可以给出轨迹方程如下

(7) $ \mu _2 = \beta _2 \Big (1 - \dfrac{2}{\beta _1 ^2}\mu _1 ^2 \Big ) $
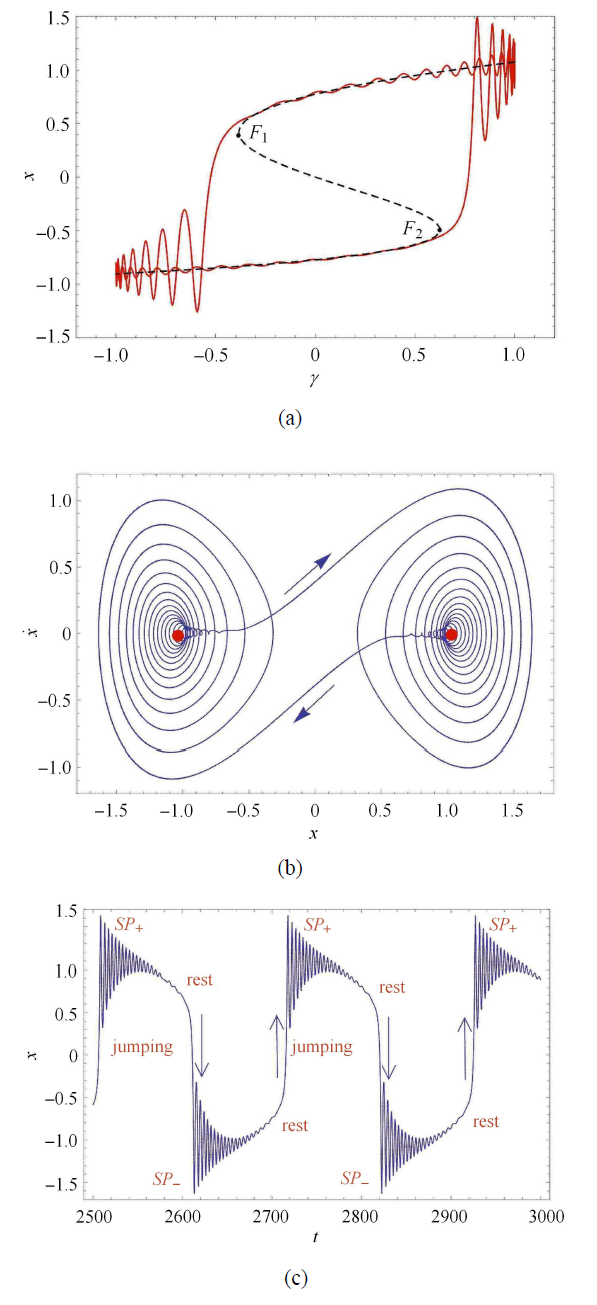
此时任意的 $\beta _1$,$\beta _2$,$a$ 都会产生 Fold/Fold 簇发振荡,见图 7. 下面将详细介绍系统是如何运动的.

图 7

新窗口打开|下载原图ZIP|生成PPT
图 7Fold/Fold簇发振荡的叠加图、相图和时间历程图

Fig. 7Composition diagram, phase diagram and time history diagram of Fold/Fold bursting



在这里可以将相图与时间历程图对应起来解释更微观的情况. 图 7 显示了簇发振荡,当 $\beta _1 = 0.38$, $\omega = 0.03$, $v = 0.1$ 时 系统发生 Fold/Fold 簇发振荡,在这里可以发现,吸引子在两个平衡点 $F_1 $,$F_2 $ 附近振荡. $F_1 $ 位于平衡上分支,$F_2 $ 位于平衡下分支. 其中的两段尖峰振荡分别对应于平衡点附近,被平衡点 $F_1 $,$F_2 $ 之间的跳跃运动所连接. 在时间历程图上,发现轨迹可以在两个重复的尖峰振荡 $SP_ + $,

$SP_ - $ 之间跳跃,这一运动是对称的. 从更深层次的来看,重复尖峰振荡的频率在改变,那是因为相关平衡点在平衡分支上的位置变化,造成了特征值的变化,使得 $SP_\pm $ 随着慢变参量 $\omega $ 的变化而变化.

文献^[16] 表示重复尖峰状态可能围绕 $F_1 $ 振荡,平衡点可能从一种状态转变为另一种状态,尖峰行为可以主要由具有较小实部的相关特征值来 决定.

假设系统从下分支上的某一点开始运动,它沿着 S 型曲线缓慢向右侧移动,直到到达折叠点,它突然从下分支跳跃到上分支,并且围绕上平衡分支 $F_1 $ 开始大幅振荡,表现为 $SP_ +$. 随着慢变参数的增大,振荡逐渐减弱. 直到慢变参数到达 1,系统开始反向运动,然后进入弛豫振荡状态,最后进入静息态,随着慢变参数的不断减小,系统到达另一个折叠点,并且在此时从上分支突然跳跃到下分支,开始围绕 $F_2 $ 进行大幅振荡,表现为 $SP_ - $. 振荡逐渐减弱,直到慢变参数到达 $-1$,系统开始反向运动,然后进入弛豫振荡状态,最后进入静息态. 如此循环. 这就构成了一个周期完整的 Fold/Fold 簇发振荡.

该簇发振荡的类型可以称为对称周期性 Fold / Fold 簇发振荡,因为此时的系统穿过路径曲线的位置是对称的.

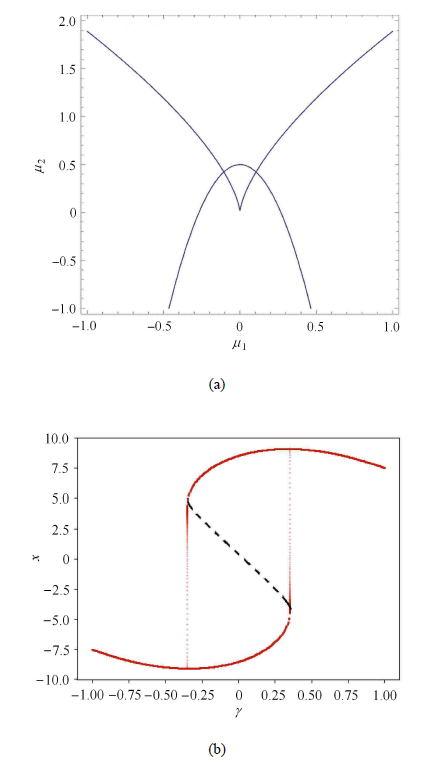
然后解释 $\beta _1 ,\beta _2 ,a$ 的任意性不改变系统的动力学行为. 使用一条曲线路径,轨迹的形状如图 8(a) 所示. 为了方便观察双稳态现象,对系统进行欧拉离散,得到图 8(b). 可以看出图 8(b) 存在两条分支,如果没有 Fold/Fold 簇发振荡,离散后的分岔图只会有一条曲线. 当 $a= 0$ 时,它有一个点 $(0,\beta _2 )$ 始终在 $\mu _2 $ 轴的上方,而规定了 $\beta _2 $ 必须大于 0,这也就解释了在 $0 < \beta _1 < 1$,$0 < \beta _2 < 1$ 的情况下,无论 $\beta _1$,$\beta _2$ 的取值为多少,都不影响 Fold/Fold 簇发振荡产生. 并且,此时的 Fold/Fold 簇发振荡是对称的.

图 8

新窗口打开|下载原图ZIP|生成PPT
图 8对应的路径与离散分岔图

Fig. 8The corresponding path and dispersed bifurcation diagram



其次,考虑 $a$ 的变化会带来什么影响,此时,鞍结曲面的位置由 $(\mu _1 ,\mu _2 ,v) \to (\mu _1 ,\overline {\mu _2 } ,v)$. 路径方程变成为

(8) $ \mu _2 = \beta _2 \Big(1 - \dfrac{2}{\beta _1 ^2}\mu _1 ^2 \Big) + a $
相当于原路径向上平移了 $a$ 个单位,路径与鞍结曲面必然会相交,只需要保证是在有效范围内相交即可. 又由于任意 $a \in (0,1)$ 都满足 $0 < \mu _2 < 1$. 这也解释了无论 $a$ 为何值,在上述的路径下,这并不影响系统从一侧曲面穿到另一侧曲面. 更多关于不同频率比与相位差的情况,如表 1 所示.

Table 1
表1
表1$\beta _1 = 0.38$, $\beta _2 = 0.34$ 三种情况下的分岔图
Table 1Three bifurcation diagrams in case of $\beta _1 = 0.38$, $\beta _2 = 0.34$

新窗口打开|下载CSV

3 当 $a \ne 0$ 时,Fold/Fold 簇发振荡的出现与消失

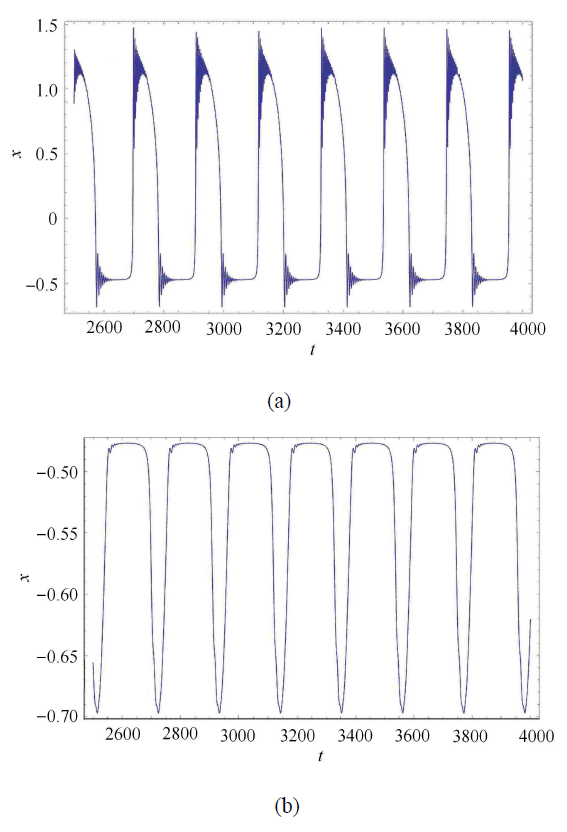
在上一部分中主要介绍了系统 (2) 的相关簇发振荡,结合时间历程图、相位图、叠加图仔细地说明了系统是如何运动的. 通过参数空间的展开理论和路径说明了系统 (2) 发生簇发振荡的原因,以及在不同参数下系统的不同行为. 在这一部分,介绍新增的常系数项会对系统 (1) 的动力学行为带来什么影响. 先通过数值模拟,发现了一种现象,之后进一步揭示这一现象产生的机理.

考虑当 $a \ne 0$ 时对簇发振荡产生什么影响. 固定 $\beta _1 = 0.38$,$\omega = 0.03$,$\beta _2 = 0.80$,当 $a$ 从 0.22 变化到 0.23 时系统的动力学行为发生巨大的变化.

由图 9 可以看出在 $a = 0.22$ 时,时间历程图表示了簇发振荡的存在,而当 $a = 0.23$ 时,时间历程图由原来的两段振荡变成了只有上部分的细微振荡,此时簇发振荡已经消失. 使用线性路径解释常系数项 $a$ 的出现却会带来 Fold/Fold 簇发振荡.

图 9

新窗口打开|下载原图ZIP|生成PPT
图 9$\beta _1 = 0.38$, $\omega = 0.03$, $\beta _2 = 0.80$, $v = 0.1$,系统 (1) 的时间历程图当 $a = 0.22$ 时表现出簇发振荡,当 $a = 0.23$ 时簇发振荡消失

Fig. 9$\beta _1 = 0.38$, $\omega = 0.03$, $\beta _2 = 0.80$, $v = 0.1$, time history diagram of system (1) showing the bursting oscillation with $a = 0.22$ and bursting oscillations disappear with $a = 0.23$



事实上,若没有常数项,此时路径方程为

(9) $ \mu _2 = \dfrac{\beta _2 }{\beta _1 }\mu _1 $
此时路径表现为经过原点的线性函数,无论如何,都不会实现从一侧曲面穿越到另一侧曲面. 若增加了常系数项,则为从一侧曲面穿越到另一侧曲面提供了可能的路径,仅在二维空间角度考虑,我们有线性路径

(10) $ \mu _2 = \dfrac{\beta _2 }{\beta _1 }\mu _1 + a $
当 $\beta _1 = 0.38$, $\beta _2 = 0.80$, $a = 0.22$ 时,路径为 $\mu _2 = 2.11\mu _1 + 0.22$,此时正处于临界状态. 路径经过鞍结曲线的一侧,但与另一侧相切,也就是恰好没有经过,此时若控制 $a < 0.22$,路径会穿过左右两侧的曲线,可以实现不对称的 Fold/Fold 簇发振荡.

4 多重 Fold/Fold 簇发振荡

本节将讨论当 $\mu _1 = \beta _1 \cos (n\theta )$ 而 $\mu _2 = \beta _2 \cos (\theta )$ 时会产生什么现象,并且给出了不同现象背后的原因. 此时系统变成如下形式

(11) $ \left.\begin{array}{l} \dot x = y \\ \dot y = - x^3 + (\beta _2 \cos \theta + a)x + \beta _1 \cos (n\theta ) - yv \end{array}\right\} $
此处,令 $\cos (n\theta ) = f_n (\cos \theta )$,使用结合二项式展开和 de Moivre 公式,可以得到

(12) $ \begin{array}{l} f_n (x) = C_n^0 x^n - C_n^2 x^{n - 2}(1 - x^2) + \cdots +\\ \qquad i^mC_n^m x^{n - m}(1 - x^2)^{\tfrac{m}{2}} \end{array} $
当 $n = 3$ 时,代入 $\cos (3\theta ) = 4\cos ^3 \theta - 3\cos \theta $,可以得到路径方程为

(13) $ \mu _1 = \dfrac{4\beta _1 }{\beta _2 ^3}\left( {\mu _2 - a} \right)^3 - \dfrac{3\beta _1 }{\beta _2 }\left( {\mu _2 - a} \right) $
此时的路径关于 $\mu _2 = a$ 成中心对称. 选用不同的参数可以实现该路径与鞍结曲面交点个数的不同,该系统的动力学行为最高可以 表现出三重 Fold/Fold 簇发振荡. 固定 $\beta _1 = 0.436$, $\beta _2 = 0.327$, $\omega = 0.03$,$v = 0.1$ 通过改变参数 $a$ 来实现不同重数的 Fold/Fold 簇发振荡. $a = 0.5$ 的时间历程图、叠加图和路径图如图 10 所示.

图 10

新窗口打开|下载原图ZIP|生成PPT
图 10对应的时间历程图、叠加图、路径图

Fig. 10Time history diagram, composition diagram and path diagram



在图 10 中的时间历程图中,阴影部分表示一个周期内的动力学行为,黑色方框表示这个周期内发生 Fold/Fold 簇发振荡. 可以很明显看出,当 $a=0.5$ 时系统发生三次 Fold/Fold 簇发振荡. 随着$a$ 的减小,系统的 "1, 2, 3" 振荡 会出现不同程度的 变化. 当 $a= 0.2$ 时,系统将只发生两次 Fold / Fold 簇发振荡,与 $a = 0.5$ 相比,振荡 "1,2" 的差别不大,但是振荡 "3" 会明显减弱. 当 $a = - 0.1$ 时,系统的动力学行为会再次改变,与前两者相比,振荡 "1" 的差别不大,振荡 "2" 明显减弱,而振荡 "3" 几乎 不显示振荡行为. 最后当 $a = - 0.5$ 时,振荡 "1, 2, 3" 几乎都会消失了,此时没有发生 Fold/Fold 簇发振荡. 其中振荡 "3" 消失的最为彻底,其次是振荡 "2",振荡 "1" 还残存着微弱的振荡.

这是由于路径最多与鞍结曲面三次相交,即穿过鞍结曲面的两侧. 一次相交就对应于一次 Fold/Fold 簇发振荡. 在 $a = 0.5$ 时,产生了三次相交,在 $a = 0.2$ 时,产生了两次相交;$a = - 0.1$ 时,产生了一次相交;$a = - 0.5$ 时没有相交.

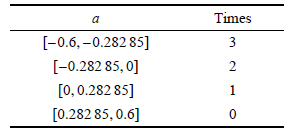
从路径图中可以发现路径与曲面相交的情况复杂多样. 表 2 表示了不同情况下的 Fold/Fold 簇发振荡.

Tabel 2
表2
表2Fold/Fold 簇发振荡重数与 $a$ 的关系
Tabel 2The relationship between $a$ and the number of Fold/Fold bursting

新窗口打开|下载CSV

关注最大重数在理想的状态下与 $n$ 之间存在的联系. 以 $n$ 为奇数时为例,结合二项式展开和 de Moivre 公式,整理系数可以得到

(14) $ \begin{array}{l} \dfrac{\mu _1 }{\beta _1 } = \alpha _1 \left( {\dfrac{\mu _2 - a}{\beta _2 }} \right)^n - \alpha _2 \left( {\dfrac{\mu _2 - a}{\beta _2 }} \right)^{n - 2} +\\ \qquad \alpha _3 \left( {\dfrac{\mu _2 - a}{\beta _2 }} \right)^{n - 4} - \cdots + ( - 1)^{\tfrac{n - 1}{2}}\alpha _{\tfrac{n + 1}{2}} \left( {\dfrac{\mu _2 - a}{\beta _2 }} \right)^1 \end{array}$
从该式的固有特点可以看出路径都可以分割成 $n$ 段,因此理想状态下,如果能使得 $n$ 段都与鞍结曲面有交点,就可以产生 $n$ 重 Fold/Fold 簇发振荡.

5 结论

系统 (1) 是一类含有两个慢变量的Duffing型方程,实验研究表明其存在双稳态现象. 为了深入理解系统 (1) 的动力学 行为,本文从数值模拟和理论分析两个方面来讨论该系统的混沌特性及簇发振荡行为,得到的结论如下.

(a) 对于系统 (2) 当振幅参数取值大于 1 时,系统表现出了不动点混沌. 并且随着参数的变化,不动点混沌可能表现为 单支存在或者双支合并. 通过叉式分岔解释了当慢变参数小于 0 时,系统只有一个稳定解,而当慢变参数大于 0 时,系统产生一对稳定的解,随 着慢变参数的继续增大,系统出现了 混沌.

(b) 对于系统 (1) 来说,它只会产生 Fold/Fold 簇发振荡,这是由于它的参数空间中只有鞍结曲面,如果存在其它曲面,对于路径的变化应该十分敏感. 并且产生的 Fold/Fold 簇发振荡与 $v$ 的取值无关. 对于 $\mu _2 - \mu _1 $ 型的路径不论是曲线还是线性只要保证它能在有效范围内穿过鞍结曲面的两侧,就能发生 Fold/Fold 簇发振荡现象. 这能解释第二部分中 $a,\beta _2 $ 选择时的任意性的原因;第三部分中常系数项$a$的变化使得系统发生巨大改变的原因. 值得一提的是穿越鞍结曲面的位置还与 Fold/Fold 簇发振荡的对称有关. 更多关于不同频率比与相位差的情况,表 1 标注了它们是否会得到 Fold/Fold 簇发振荡.

(c) 当 $\mu _1 = \beta _1 \cos (n\theta )$,$\mu _2 = \beta _2 \cos (\theta )$ 时使用 $\mu _1 - \mu _2 $ 路径讨论产生的现象,此时系统表现出多簇发振荡现象. 探究了不同重数的 Fold/Fold 簇发振荡与 $n$ 值之间的关系. 由于路径都可以分割成 $n$ 段,因此理想状态下,$n$ 段都会与鞍结曲面有交点,从而产生 $n$ 重 Fold/Fold 簇发振荡.

参考文献 View Option 原文顺序
文献年度倒序
文中引用次数倒序
被引期刊影响因子

[1] Popovi? N, Marr C, Swain PS. A geometric analysis of fast-slow models for stochastic gene expression
Journal of Mathematical Biology, 2016,72(1):87-122
[本文引用: 1]

[2] Chtouki A, Lakrad F, Belhaq M. Quasi-periodic bursters and chaotic dynamics in a shallow arch subject to a fast--slow parametric excitation
Nonlinear Dynamics, 2020,99(570):283-298


[3] Podogova SD, Mishagin KG, Medvedev SY, et al. An algorithm for a group time scale using a moving average over multiple time scales
Measurement Techniques, 2015,58(3):532-538
DOIURL [本文引用: 1]

[4] Karami H, Karimi S, Bonakdari H, et al. Predicting discharge coefficient of triangular labyrinth weir using extreme learning machine, artificial neural network and genetic programming
Neural Computing and Applications, 2018,29(7):983-989
DOIURL [本文引用: 1]

[5] Sun J, Wu ZH. Isolating spatiotemporally local mixed Rossby-gravity waves using multi-dimensional ensemble empirical mode decomposition
Climate Dynamics, 2020,54(3):1383-1405
[本文引用: 1]

[6] Zhang Y, Li T, Gao JY, et al. Origins of quasi-biweekly and intraseasonal oscillations over the South China Sea and Bay of Bengal and scale selection of unstable equatorial and off-equatorial modes
Journal of Meteorological Research, 2020,34(2):137-149
DOIURL

[7] Ngueuteu GSM, Yamapi R, Woafo P. Quasi-static transient and mixed mode oscillations induced by fractional derivatives effect on the slow flow near folded singularity
Nonlinear Dynamics, 2014,78(4):2717-2729


[8] Han XJ, Bi QS, Zhang C, et al. Study of mixed-mode oscillations in a parametrically excited van der Pol system
Nonlinear Dynamics, 2014,77(4):1285-1296
[本文引用: 1]

[9] Han XJ, Xia FB, Zhang C, et al. Origin of mixed-mode oscillations through speed escape of attractors in a Rayleigh equation with multiple-frequency excitations
Nonlinear Dynamics, 2017,88(4):2693-2703


[10] Zhang XF, Zheng JK, Wu GQ, et al. Mixed mode oscillations as well as the bifurcation mechanism in a Duffing's oscillator with two external periodic excitations
Science China Technological Sciences, 2019,62(10):1816-1824
[本文引用: 1]

[11] Rinzel J. Bursting oscillations in an excitable membrane model
Ordinary and Partial Differential Equations, 1985,1151:304-316
[本文引用: 2]

[12] Izhikevich EM. Neural excitablity, spiking and bursting
International Journal of Bifurcation and Chaos, 2000,10(6):1171-1266
[本文引用: 2]

[13] Meng WJ, Qian YH. Mixed-mode oscillation in a class of delayed feedback system and multistability dynamic response
Complexity, 2020,2020:1-18
[本文引用: 1]

[14] Qian YH, Yan DM. Fast slow dynamics analysis of a coupled duffing system with periodic excitation
International Journal of Bifurcation and Chaos, 2016,28(8):1850148
[本文引用: 1]

[15] Zhang W, Liu YZ, Wu MQ. Theory and experiment of nonlinear vibrations and dynamic snap-through phenomena for bi-stable asymmetric laminated composite square panels under foundation excitation
Composite Structures, 2019,225:111140
[本文引用: 7]

[16] Chen XK, Li SL, Zhang ZD, et al. Relaxation oscillations induced by an order gap between exciting frequency and natural frequency
Science China Technological Sciences, 2017,60(2):289-298
[本文引用: 2]

[17] Li XH, Shen YJ, Sun JQ, et al. New periodic-chaotic attractors in slow-fast Duffing system with periodic parametric excitation
Science Reports, 2019,9(1):422-434
[本文引用: 2]

[18] Lin Y, Liu WB, Bao H, et al. Bifurcation mechanism of periodic bursting in a simple three-element-based memristive circuit with fast-slow effect
Chaos, Solitons and Fractals, 2020,131(3):109524
DOIURL [本文引用: 1]

[19] Wang N, Zhang GS, Bao H. Bursting oscillations and coexisting attractors in a simple memristor-capacitor-based chaotic circuit
Nonlinear Dynamics, 2019,97(2):1477-1494
[本文引用: 1]

[20] 苟向锋, 韩林勃, 朱凌云 等. 单自由度齿轮传动系统安全盆侵蚀与分岔
振动与冲击, 2020,39(2):123-131
[本文引用: 1]

( Gou Xiangfeng, Han Linbo, Zhu Lingyun, et al. Safety basin erosion and bifurcation of single-degree-of-freedom gear transmission system
Chinese Journal of Vibration and Shock, 2020,39(2):123-131 (in Chinese))
[本文引用: 1]

[21] 魏梦可, 韩修静, 张晓芳 等. 正负双向脉冲式爆炸及其诱导的簇发振荡
力学学报, 2019,51(3):904-911
[本文引用: 1]

( Wei Mengke, Han Xiujing, Zhang Xiaofang, et al. Positive and negative two-way pulse explosion and its induced cluster oscillation
Chinese Journal of Theoretical and Applied Mechanics, 2019,51(3):904-911 (in Chinese))
[本文引用: 1]

[22] 郑健康, 张晓芳, 毕勤胜. 一类混沌系统中的簇发振荡及其延迟叉形分岔行为
力学学报, 2019,51(2):540-549
[本文引用: 1]

( Zheng Jiankang, Zhang Xiaofang, Bi Qinsheng. Clusters oscillation and delayed fork bifurcation in a class of chaotic systems
Chinese Journal of Theoretical and Applied Mechanics, 2019,51(2):540-549 (in Chinese))
[本文引用: 1]

[23] 张正娣, 李静, 刘亚楠 等. 非光滑系统不同簇发模式之间的演化及其机理
中国科学: 技术科学, 2019,49(9):1031-1039
[本文引用: 1]

( Zhang Zhengdi, Li Jing, Liu Yanan, et al. Evolution and mechanism of different cluster patterns in non-smooth systems
Chinese Journal of Science in China, 2019,49(9):1031-1039 (in Chinese))
[本文引用: 1]

[24] 曲子芳, 张正娣, 彭淼 等. 双频激励下 Filippov 系统的非光滑簇发振荡机理
力学学报, 2018,50(5):1145-1155
[本文引用: 1]

( Qu Zifang, Zhang Zhengdi, Peng Miao, et al. Oscillation mechanism of non-smooth clusters in Filippov system under dual-frequency excitation
Chinese Journal of Theoretical and Applied Mechanics, 2018,50(5):1145-1155 (in Chinese))
[本文引用: 1]

[25] Han XJ, Yu Y, Zhang C, et al. Turnover of hysteresis determines novel bursting in Duffing system with multiple-frequency external forcings
International Journal of Non-Linear Mechanics, 2017,89:69-74
[本文引用: 1]

[26] Han XJ, Zhang Y, Bi QS, et al. Two novel bursting patterns in the Duffing system with multiple-frequency slow parametric excitations
Chaos, 2018,28:043111
DOIURLPMID [本文引用: 1]
This paper aims to report two novel bursting patterns, the turnover-of-pitchfork-hysteresis-induced bursting and the compound pitchfork-hysteresis bursting, demonstrated for the Duffing system with multiple-frequency parametric excitations. Typically, a hysteresis behavior between the origin and non-zero equilibria of the fast subsystem can be observed due to delayed pitchfork bifurcation. Based on numerical analysis, we show that the stable equilibrium branches, related to the non-zero equilibria resulted from the pitchfork bifurcation, may become the ones with twists and turns. Then, the novel bursting pattern turnover-of-pitchfork-hysteresis-induced bursting is revealed accordingly. In particular, we show that additional pitchfork bifurcation points may appear in the fast subsystem under certain parameter conditions. This creates multiple delay-induced hysteresis behavior and helps us to reveal the other novel bursting pattern, the compound pitchfork-hysteresis bursting. Besides, effects of parameters on the bursting patterns are studied to explore the relation of these two novel bursting patterns.

[27] Rinzel J, Lee YS. Dissection of a model for neuronal parabolic bursting
Journal of Mathematical Biology, 1987,25(4):653-675
DOIURL [本文引用: 1]

[28] Bertram R, Butte MJ, Kiemel T, et al. Topological and phenomenological classifification of bursting oscillations
Bulletin of Mathematical Biology, 1995,57(3):413-439
URLPMID [本文引用: 1]

[29] Stern JV, Osinga HM, LeBeau A, et al. Resetting behavior in a model of bursting in secretory pituitary cells: distinguishing plateaus from pseudo-plateaus
Bulletin of Mathematical Biology, 2008,70(1):68-88
DOIURLPMID [本文引用: 1]
We study a recently discovered class of models for plateau bursting, inspired by models for endocrine pituitary cells. In contrast to classical models for fold-homoclinic (square-wave) bursting, the spikes of the active phase are not supported by limit cycles of the frozen fast subsystem, but are transient oscillations generated by unstable limit cycles emanating from a subcritical Hopf bifurcation around a stable steady state. Experimental time courses are suggestive of such fold-subHopf models because the spikes tend to be small and variable in amplitude; we call this pseudo-plateau bursting. We show here that distinct properties of the response to attempted resets from the silent phase to the active phase provide a clearer, qualitative criterion for choosing between the two classes of models. The fold-homoclinic class is characterized by induced active phases that increase towards the duration of the unperturbed active phase as resets are delivered later in the silent phase. For the fold-subHopf class of pseudo-plateau bursting, resetting is difficult and succeeds only in limited windows of the silent phase but, paradoxically, can dramatically exceed the native active phase duration.

[30] Krauskopf B, Osinga HM. A codimension-four singularity with potential for action
Mathematical Sciences with Multidisciplinary Applications, 2016,157:253-268
[本文引用: 1]

[31] Saggio ML, Spiegler A, Bernard C, et al. Fast--slow bursters in the unfolding of a high codimension singularity and the ultra-slow transitions of classes
The Journal of Mathematical Neuroscience, 2017,7:7
URLPMID [本文引用: 1]