非线性海洋内波的理论、模型与计算1)

删除或更新信息，请邮件至freekaoyan#163.com(#换成@)

本站小编 Free考研考试/2022-01-01

王展^,^*^,^?^,²⁾, 朱玉可^**

^*中国科学院力学研究所, 北京 100190

^†中国科学院大学工程科学学院, 北京 100049

^**浙江大学数学科学学院, 杭州 310027

THEORY, MODELLING AND COMPUTATION OF NONLINEAR OCEAN INTERNAL WAVES¹⁾

Wang Zhan^,^*^,^?^,²⁾, Zhu Yuke^**

^*Institute of Mechanics, Chinese Academy of Sciences, Beijing 100190, China

^†School of Engineering Sciences, University of Chinese Academy of Sciences, Beijing 100049, China

^**School of Mathematical Sciences, Zhejiang University, Hangzhou 310027, China

通讯作者: 2) 王展, 研究员, 主要研究方向: 水动力学. E-mail:zwang@imech.ac.cn

收稿日期:2019-10-25接受日期:2019-11-10网络出版日期:2019-11-18

基金资助:

1) 国家自然科学基金资助项目.11772341
国家自然科学基金资助项目.11911530171

Received:2019-10-25Accepted:2019-11-10Online:2019-11-18
作者简介 About authors

摘要
海水因盐度与温度的垂向差异造成密度层结现象, 进而由于海洋系统的内部扰动(如海潮流过局部隆起的海底地形)与外部扰动(如死水现象)造成等密面的波动, 这一现象称为“内波”. 内波在全球范围内大量存在, 尤其是在海峡入海口等密度层结现象较为明显和稳定的区域会有内波频繁活动. 海洋通常呈现“三明治”状的结构: 密度相对稳定的混合层与深水层, 以及位于中间密度连续过渡的密跃层. 密跃层的整体脉动对于海洋工程和海洋生态环境有重大的影响; 而密跃层内部的波动对于潜艇的非声探测(反过来说, 对于潜艇的隐身作战)具有潜在的应用价值. 而造成这些重大影响的根源在于内波在水平和垂直方向都具备传播能力, 这是有别于海洋表面波浪的关键之处.本文针对两类海洋密度模型-连续分层模型与间断分层模型, 从理论研究、数值模拟、实验室机理实验等方面论述了研究海洋内波的各类非线性模型(包括弱非线性的Korteweg-de Vries方程、Benjamin-Ono方程, Kadomtsev-Petviashvili方程等著名模型以及强非线性Miyata-Choi-Camassa方程、非线性势流理论、带密度变化的不可压缩Navier-Stokes方程等), 讨论各自的适用范围, 并借此探讨内波在海洋质量动量能量输运中所起的至关重要的作用.
关键词： 海洋内波;间断分层模型;连续分层模型;非线性波;边界积分法;Boussinesq方程;高阶算法

Abstract
Salinity and temperature variations in the vertical direction lead to density stratification in oceans, and the fluctuation of isopycnal surfaces resulting from internal perturbations (such as stratified shear flow over a bottom topography) or external disturbances (such as the dead water phenomenon) is called the internal wave. Internal waves are ubiquitous in the ocean and usually arise in the situation when the density stratification is obvious and stable such as at the mouth of strait. Oceans are usually characterized by a sandwich-like structure: a mixing layer and a deep-water layer featuring an almost uniform density, and a transition layer in the middle with continuous density variation. Fluctuations of the transition layer have great impact on ocean engineering and ocean ecology, while waves inside the transition layer has potential applications in the non-acoustic detection of submarines (conversely, in the stealth operation of submarines). The main reason for these important influences lies in the ability of internal waves to propagate in both horizontal and vertical directions, which is the essential difference from that of ocean surface waves. In the current paper, two types of ocean density models, continuously stratified models and discontinuous layered models, are thoroughly discussed. Various nonlinear models used to study ocean internal waves (including celebrated weakly nonlinear models, such as the Korteweg-de Vries equation, the Benjamin-Ono equation, and the Kadomtsev-Petviashvili equation, and strongly nonlinear models, such as the Miyata-Choi-Camassa equation, the fully nonlinear potential theory, and the incompressible Navier-Stokes equation with density variations), as well as their respective scope of application, are reviewed from the aspects of theoretical analyses, numerical simulations, and laboratory experiments. Particular attention is paid to the important role of internal waves in transferring mass, momentum and energy in oceans.
Keywords：internal ocean wave;layered model;continuously stratified model;nonlinear wave;boundary integral method;Boussinesq equations;high-order numerical scheme

PDF (23102KB)元数据多维度评价相关文章导出EndNote|Ris|Bibtex 收藏本文
本文引用格式
王展, 朱玉可. 非线性海洋内波的理论、模型与计算¹⁾. 力学学报[J], 2019, 51(6): 1589-1604 DOI:10.6052/0459-1879-19-326
Wang Zhan, Zhu Yuke. THEORY, MODELLING AND COMPUTATION OF NONLINEAR OCEAN INTERNAL WAVES¹⁾. Chinese Journal of Theoretical and Applied Mechanics[J], 2019, 51(6): 1589-1604 DOI:10.6052/0459-1879-19-326

引言

Nansen船长与死水现象

1893年, 32岁的挪威极地探险家Fridtjof Nansen驾驶着自行设计的Fram号前往北极探险. 他在穿越北西伯利亚诺登舍尔德群岛的时候遇到了一种被当地水手称为“死水”的奇怪现象, Fram号遭遇了来自海水的某种神秘拖拽力, 在周围海洋环境没有太大变化的情况下, 其航速从原先的6至7节直接降至1.5节以下. Nansen船长在日记中写道:

“When caught in dead water Fram appeared to be held back, as if by some mysterious force, and she did not always answer the helm. In calm weather, with a light cargo, Fram was capable of 6 to 7 knots. When in dead water she was unable to make 1.5 knots. We made loops in our course, turned sometimes right around, tried all sorts of antics to clear of it, but to very little purpose.”

探险归来, Nansen船长前去请教同事Vilhelm Bjerknes教授, 后者大胆地提出了一种猜想, 即在密度明显分层的流体上航行时, 船舶的动能会有很大一部分转移到流体内部密度跳跃的分界面上导致密跃层波动, 而这种内部波动又反过来干扰船舶的正常前进. Bjerknes教授安排了一名博士生, 也就是后来成为一代海洋学大家的瑞典人Vagn Walfrid Ekman, 对此假说进行检验. Ekman在他1904年的博士论文中设计了一个简单的模型, 并开展了一系列精巧的实验证实了这一解释. 2011年为纪念Nansen 船长诞辰150周年, 法国里昂高等师范学院物理实验室的Matthieu Mercier等实验流体力学家利用现代实验手段复现了Ekman的死水实验^[1]. 从所拍摄的视频中清晰可见内波产生的全过程, 以及如何反作用于船上阻碍其前进(见图1).

图1

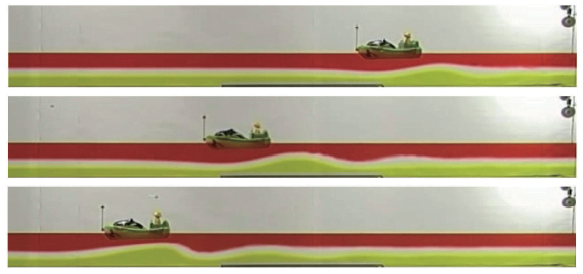新窗口打开|下载原图ZIP|生成PPT
图1法国里昂高等师范学院物理实验室利用现代技术复现Ekman的“死水”实验. 以三层流体系统为实验模型(由上而下以红白黄三色区分), 每层的厚度分别为5.0 cm, 3.0 cm与5.5 cm, 而密度分别为0.9967 g/cm$^3$, 1.0079 g/cm$^3$和1.0201 g/cm$^3$. 模型船由左向右航行, 3张图由上至下表示不同时刻模型船的位置以及内波的波形

Fig.1Ekman’s dead water experiments reproduced with modern technology by French experimentalists in the laboratory of physics at of Lyon. The three-layer fluid system was used as the model(distinguished by red, white and yellow from top to bottom). The of each layer was 5.0 cm, 3.0 cm, and 5.5 cm respectively, and the density was 0.9967 g/cm$^3$, 1.0079 g/cm$^3$, and 1.0201 g/cm$^3$. The model ship sails from left to right, and snapshots from top to bottom show the position of the model as well as the internal wave profiles at different times

死水现象使人类认识到海洋远比我们想象的要复杂得多, 即使在风平浪静的时候, 海面下依然可能存在轩然大波. 1980年8月12日, 纽约时报科学版刊出题为《隐藏在海面下的巨大波浪产生的表面波纹》的文章, 对内波的存在做了长篇报道. 对于大振幅内波可能对人类活动和环境造成的影响, 该文主要提出以下三点: ①对潜艇等海洋内部航行器的影响; ②对海洋作业平台及水下生产设施的影响; ③对海洋物质输运及生态环境的影响. 之后人类对于海洋的研究与实践活动使得这些预测被一一证实.

大自然中的海洋

海水因盐度与温度的差异造成密度跃层, 进而引发内部密跃层的波动, 这一现象称为“内波”. 由于实际海水密度的层间变化很小(跃层上下的相对密度差仅约为0.1${\%}$), 所以海水只要受到很小的扰动就会偏离其平衡位置而产生“轩然大波”. 因此了解海洋的密度结构是内波研究的前提.

海洋是处于旋转地球上受重力作用的广袤含盐水体. 在海面受太阳辐射的不均匀加热, 以及不同气候带的大气动力学和热力学作用下, 各海区海水的温度、盐度和密度都有显著的差异, 但其垂直分布却呈现出某种有规律的宏观层次结构. 观测表明, 海洋中普遍存在的层结现象可以由海水的温度和盐度差异引起, 主跃层出现在深度约200$\sim$1000 m处, 据此我们可以将海洋沿垂直方向大致分为三层(见图2): 主跃层往上($<$200 m)海洋表层由于风剪切和表面波破碎造成上层混合层, 在该区域由于湍流混合, 温度、盐度与密度几乎都是均匀的; 主跃层(200$\sim$1000 m)内温度、盐度与密度均出现大幅变化, 三者呈现某种相关性; 深水区($>$1000 m)海水的物理性质相对稳定, 各个量变化很小. 这种分层结构称为Holmboe密度模式.

图2

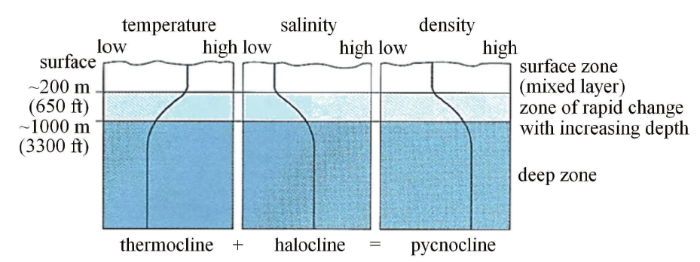新窗口打开|下载原图ZIP|生成PPT
图2海洋中温度、盐度、密度随水深的变化函数

Fig.2Temperature, salinity, and density in the ocean as function of depth

因此海洋内部波动的数学模型一般分为两大类: 密度强分层模型(即将Holmboe模型式中的密跃层看作一个整体, 用于研究密跃层的整体脉动)与密度连续分层模型; 前者称为界面波, 而后者称为体波.

内波的观测

自1893年Nansen船长在北冰洋附近遭遇“死水效应”以及Ekman的实验发表之后不断有关于海洋内波的观测报告, 如1910年“Michael Sars”号的考察, 1927年“Meteor”号的考察, 1929年“Snellius”号的考察等. 这一时期, 人们对内波的特性与运动规律知之甚少. 自20世纪40年代起, 温深仪的发明及各种快速密集取样调查仪器与方法的相继出现, 使得内波的观测工作迅速发展起来. 早期观测中最著名的是Perry和Schimke^[2]在安达曼海的测量. 他们在1500 m深的海域中约500 m处的主温跃层上发现了高达80 m, 长约2000 m的海洋内波, 并伴随着海面上大片的短波区. Osborne和Burch^[3]的研究表明, 此内波是由安达曼和尼科巴岛链中的潮汐流产生的, 并向数百公里外的苏门答腊海岸线传播; 特别地, 他们注意到当内波通过这片海域之后海面变得极度平静, 这一引人注目的现象被称为“贮水池效应”(millpond effect). 中国科学院南海海洋研究所“实验3号”考察船在东沙群岛西侧、西北和南部地区进行定点观测; 美国-加拿大于1983年和1984年在佐治亚海峡及纽约长岛附近海岸开展联合观测; 2001年亚洲海域国际声学实验采用多条温度链对南海北部的内波进行观测, 得到了非线性内波的传播演化情况. 图3显示的是Duda等^[4]在中国南海地区观测到的内孤立波, 其水深约为340 m, 内波振幅竟高达150 m.

图3

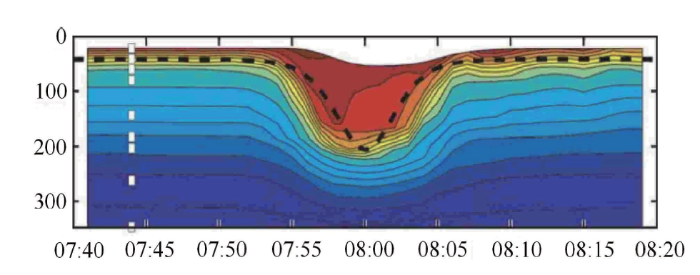新窗口打开|下载原图ZIP|生成PPT
图3中国南海观测到的大振幅内孤立波. 水平坐标表示时间, 每隔1 min采样一次; 垂直坐标表示海洋深度(总深度约为340 m)

Fig.3A large-amplitude internal solitary wave observed in the South China Sea. The horizontal coordinate represents the time, sampling every 1 min, and the vertical coordinate indicates the depth of the ocean (the total depth is about 340 m)

随着合成孔径雷达(synthetic aperture radar, SAR)这一卫星遥感技术的出现, 大范围的海洋摄影成为可能. 相比于现场观测, 合成孔径雷达具有全天时、全天候、远距离、大范围、高分辨率等优势^[5]; 当有大振幅内孤立波通过被监测海域时, 海洋表面会出现完全局部化的剧烈浪潮区, 这使得SAR图像上反映出来的海面反射特性发生明显变化, 研究人员正是通过对激潮区的观测来判定内波的存在(见图4). 卫星遥感探测大振幅内波的基本原理为: 内孤立波通过其所诱导的剪切流场调制海表波, 引起海洋表面波浪的辐聚和辐散, 从而改变海洋表面的粗糙度, 使得SAR遥感图像表现出明暗相间的条纹特征^[6]. 由内波激发的局部海面波群称为“激潮”(tide rip), 意为潮水冲突引起的波浪, 亦被称为内波的“表面特征”(surface signature). 内波激潮现象的存在为强内波的参数反演提供了途径, 即利用表面波群的水动力学参数及其他水文资料提取内波的波速、波长、振幅等关键参数^[7]. 值得一提的是, 除SAR以外另有其他卫星可用于内波表面特征的观测, 其中比较著名的是Moderate Resolution Imaging Spectroradiometer (有兴趣的读者可参看文献^[8]).

图4

新窗口打开|下载原图ZIP|生成PPT
图4东沙群岛北部(北纬21度, 东经116度51分)内孤立波的卫星遥感图像. ? CNES 2000 - Spot Image distribution

Fig.4SAR image of an internal solitary wave in the northern Dongsha islands (21 degrees north latitude, 116 degrees and 51 minutes east longitude)

内波的生成机制与现实影响

由于海洋内部跃层两侧的密度差远小于海水与空气的密度差, 致使海洋内波的振幅巨大. 这导致内波在整个海洋生态系统的平衡以及动量能量的垂向和水平输运中显得至关重要, 尤其是大振幅的内孤立波可携带质量和动量传播上百公里, 可将海底营养物质带到混合层从而使海洋生物受益. 在军事领域, 海洋内波导致的等密面脉动能造成鱼雷脱靶、潜艇失控等重大事故, 同时还能极大地影响声波的传播, 给水下目标的探测与定位以及水声通信带来严重影响. 在民用上, 海上作业平台和水下生产设施因遭受内波影响而引发事故时有发生, 典型事件如: 安达曼海石油钻机被内孤立波扭转90$^\circ$并推移至30 m开外; “南海VI号”石油钻机遭遇强内波袭击致使作业受到严重影响; 流花油田半潜式平台在锚泊系统解脱过程中因内孤立波来袭造成在15 min内向北漂移120 m等^[9].

我国南海是海洋油气聚集中心之一, 也是内波这种自然现象频发的海域(见图5), 吕宋海峡更被认为是南海内孤立波的重要源头^[10-11]. 为保障海上作业安全, 深刻理解内波在我国南海地区的生成与发展、演化、传播机制, 建立早期的预警系统进行实时监控, 以便在强内波来袭时获得应对的时间, 对于我国的海洋工程尤其是深海油气资源的开发具有重要的意义^[12].

图5

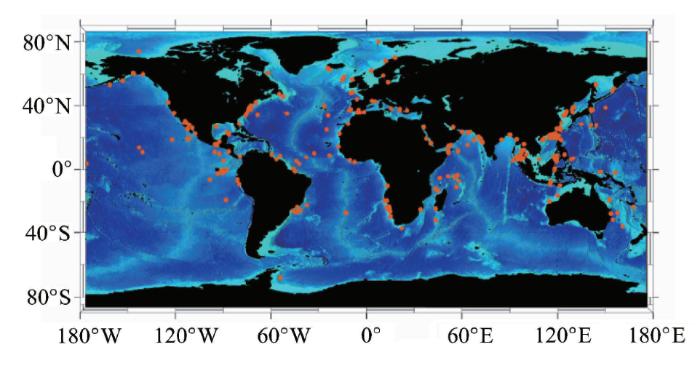新窗口打开|下载原图ZIP|生成PPT
图5目前已观测到的内孤立波全球分布图; 大部分位置通过卫星遥感探知

Fig.5Global distribution of internal solitary waves. Most of the locations were obtained by satellite remote sensing

1 密度强分层模型

20世纪70年代海洋卫星高空遥感技术的发展与应用成为人类认识海洋动力学的新起点. 对于海洋表面波与内波的研究成为了海洋工程、卫星遥感、水动力学、应用数学的汇聚之处. 随着人类对海洋探索的不断深入以及海洋工程的迫切需求, 近年来对于内波的理论与计算研究均取得了很大的进展, 许多非线性数学模型被构造出来, 而机理性的研究也朝着精细化和数学化的方向发展.

1.1 二维两层模型

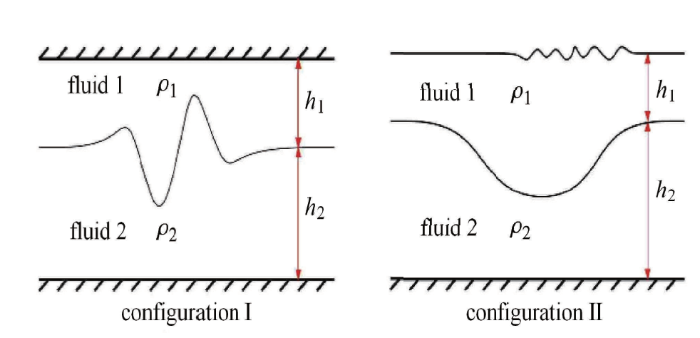
图6是两种不同密度的不混溶液体内界面波的典型示意图: 其中$\rho_1(\rho_2)$ 表示上(下)层流体的密度; $h_1(h_2)$表示上(下)层流体静止时的平均深度; $\eta(x,t)$ 表示其偏离平衡位置的高度. 相比于内波的巨大振幅, 海洋表面波浪的尺度要小得多, 因此在开展内波理论与数值研究时, 大部分****往往将海洋自由面假设为一个没有形变的固定壁, 这一简化称为“刚盖假设” (如图6左边所示). 但是在研究内波的表面特征(即内波激潮)时往往需要将海洋表面当作自由面来处理(如图6右边所示).

图6

新窗口打开|下载原图ZIP|生成PPT
图6“刚盖假设”下的内界面波(左); 带有自由表面的内界面波(右)

Fig.6Left panel: interfacial waves under the rigid-lid approximation. Right panel: interfacial waves with a free surface

1.1.1 弱非线性模型

在实际工程应用当中, 由于Korteweg-de Vries (KdV)方程能兼顾非线性与频散效应, 且形式简单便于分析, 同时又易于在其中引入反映地形变化、海底摩擦、科里奥利力等影响因素的项, 是目前处理内波问题时使用最为广泛的方程. 量化非线性和频散效应重要性的一种普遍接受的方法是引入两个独立的无量纲参数: 振幅参数$\alpha = a/h_1$和波长参数$\beta = h_1/\lambda$, 其中$a$和$\lambda$表示内波的振幅和波长. 对于两层流体间界面的波动规律, 还有一个十分重要的参数, 那就是上下流体层的平均深度之比$\gamma = h_1/h_2$. 即使在弱非线性假设之下($\alpha \ll 1$和$\beta \ll 1$), $\gamma$ 的不同也可以导致不同的模型. 下层流体厚度相比于波长可以是很小的, 也可以是相同量级的, 甚至下层流体厚度可以看作是无限深的($h_2 \to \infty$).

对于上下层流体厚度相差不大的情形$\gamma=O(1)$, 在满足$\alpha=O(\beta^2)$ 和$\alpha \ll 1$的假设之下, 可以导出经典的KdV方程作为描述单向传播和弱非线性波动的演化控制方程

(1) $\begin{eqnarray} \eta_t+c\eta_x+\alpha_1\eta \eta_x+\beta_1\eta_{xxx}=0 \end{eqnarray}$

其中

$\begin{eqnarray*} &&c = \left[\dfrac{g(\rho_2-\rho_1)h_2h_1}{\rho_2h_1+\rho_1h_2}\right]^{1/2}\\[1.5mm]&& \alpha_1 =\dfrac{3}{2}\dfrac{c}{h_1h_2}\dfrac{\rho_2h_1^2-\rho_1h_2^2}{\rho_2h_1+\rho_1h_2} \\[1.5mm]&& \beta_1 = \dfrac{ch_1h_2}{6}\dfrac{\rho_1h_1+\rho_2h_2}{\rho_2h_1+\rho_1h_2} \end{eqnarray*}$

对于一个小振幅谐波$\eta={\rm e}^{{\rm i}(kx-\omega t)}$, 线性化方程(1)可以得到其频散关系

(2) $\begin{eqnarray} \omega=ck -\beta_1 k^3 \end{eqnarray}$

当下层的深度远大于上层时($\gamma \gg 1$), 在$\alpha=O(\beta)$和$h_2=O(\lambda)$的假设之下可以导出中长波方程^[14-15]

(3) $\begin{eqnarray} \eta_t+c\eta_x+\alpha_1\eta \eta_x+\dfrac{\partial }{\partial x} \int^{+\infty}_{-\infty} G(x-x’)\eta(x’,t){\rm d}x’=0 \end{eqnarray}$

其中

$G(\xi)=\dfrac {c}{2\pi} \int^{+\infty}_{-\infty} \lt[1-\dfrac{kh_1}{2} ({\rm coth} (kh_2)-1/kh_2) ] {\rm e}^{{\rm i}k\xi} {\rm d}k$

以及

$c=\lt( \dfrac{\rho_2-\rho_1}{\rho_1} gh_1 )^{1/2}, \ \ \alpha_1=-\dfrac {3c}{2h_1}$

其相应的频散关系为

(4) $\begin{eqnarray} \omega = ck\left[1-\frac{kh_1}{2}(\coth kh_2 - 1/kh_2)\right] \end{eqnarray}$

当下层流体无限深时, 即$\gamma \to \infty$, 方程(3)即为著名的Benjamin-Ono方程^[16-18]

(5) $\begin{eqnarray} \eta_t + c\eta_x + \alpha_1\eta \eta_x + \dfrac{\beta_1}{\pi}\dfrac{\partial^2}{\partial x^2} \int_{-\infty}^{+\infty} \dfrac{\eta(x’,t)}{x-x’} {\rm d}x’ = 0 \end{eqnarray}$

其中

$\alpha_1 = -\frac{3c}{2h_1},\ \ \beta_1 = \frac{ch_1\rho_2}{2\rho_1}$

其相应的频散关系为

(6) $\begin{eqnarray} \omega = ck - \beta_1k|k| \end{eqnarray}$

最近, Matsuno和Yoshimasa^[19]和Choi和Camassa^[20]在弱非线性假设下得出了对任意$\gamma$有效的更一般的方程形式.

在研究单一孤立波性质中, 实验表明弱非线性的KdV理论在许多研究中显示出相当大的有效范围^[21-23]. Koop和Butler^[22]发现对于深水中的弱非线性波, KdV模型要优于Benjamin-Ono模型. 在上下流体厚度比$\gamma=0.24$的一系列实验中, Grue等^[21]发现当波的振幅与上层流体厚度比约为0.4时, KdV理论具有很好的预测效果. 但是当振幅继续增大时, 受限于弱非线性假设, KdV理论表现的不是那么理想. 虽然高阶KdV理论在$\gamma \ll 1$时改善了这种情况, 但最终也因同样的原因而失败. Michallet等^[23]发现eKdV理论提供了一些改进, 特别是当$0.4<h_1/(h_1+h_2)<0.6$时理论与实验具有很好的一致性. eKdV方程就是在KdV方程的基础上引入三阶非线性项, 其一般形式为^[24]

(7) $\begin{eqnarray} \eta_t + \left(c+\alpha_1\eta+\alpha_2\eta^2\right)\eta_x + \beta_1\eta_{xxx} = 0 \end{eqnarray}$

它可用来描述海洋中实际观测到的内孤立波脉冲变平变宽的性质, 且在振幅的适用范围上比KdV更广^[25]. 所有这些模型的共同特点是对原始控制方程及初始条件, 通过传统的渐进分析技巧利用小参数(例如$\alpha$或$\beta$)消除对垂直坐标的依赖性, 从而达到了对原问题的实质性简化.

1.1.2 强非线性模型

在理论研究中, 这些弱非线性弱色散模型在解释现象和阐述机理方面起到了本质性的作用. 尽管如此, 为了精确描述海洋观测结果, 用于刻画大振幅内波的强非线性模型($\alpha=O(1)$和$\beta \ll 1$)最终仍然是需要的. Miyata^[26]和Choi和Camassa^[27]各自独立导出了强非线性的内波模型

(8a) $\begin{eqnarray} && {\eta_1}_t + (\eta_1\bar{u}_1)_x = 0, \ \ \eta_1 = h_1-\eta\\ \end{eqnarray}$

(8b) $\begin{eqnarray} && {\eta_2}_t + (\eta_2\bar{u}_2)_x = 0, \ \ \eta_2 = h_2-\eta\\ \end{eqnarray}$

(8c) $\begin{eqnarray} && {\bar{u}_{1t}} + \bar{u}_1\bar{u}_{1x} + g\eta_x = \dfrac{P_x}{\rho_1} + \dfrac{1}{\eta_1}\left(\dfrac{1}{3}\eta_1^3G_1\right)_x + O\left(\beta^4\right)\qquad\\ \end{eqnarray} $

(8d) $\begin{eqnarray} && {\bar{u}_{2t}} + \bar{u}_2\bar{u}_{2x} + g\eta_x = \dfrac{P_x}{\rho_2} + \dfrac{1}{\eta_2}\left(\dfrac{1}{3}\eta_2^3G_2\right)_x + O\left(\beta^4\right) \end{eqnarray} $

其中

(9a) $\begin{eqnarray} && \bar{u}_1(x,t) = \dfrac{1}{\eta_1}\int_{\eta}^{h_1}u_1(x,z,t) {\rm d}z\\ \end{eqnarray} $

(9b) $\begin{eqnarray}&& G_1(x,t) = \bar{u}_{1xt} + \bar{u}_1\bar{u}_{1xx} - (\bar{u}_{1x})^2 \end{eqnarray} $

$\bar{u}_2(x,t)$以及$G_2(x,t)$ 的定义类似, $ P(x,t)$表示界面处的压强. 方程(8)是Choi和 Camassa^[27]在浅水假设下推导出来的演化方程, 在弱非线性假设下($\alpha = O(\beta^2)$), 可进一步导出KdV方程.

与真实实验的比较显示, Miyata-Choi-Camassa方程在求解大振幅内孤立波时要明显好于KdV方程. 但是Miyata-Choi-Camassa方程存在着一个潜在的问题: Jo和 Choi^[28]在做时间依赖的数值计算时发现, 当振幅足够大时内孤立波在演化过程中会出现Kelvin-Helmholtz不稳定性, 从而导致计算终止, 并且无从判断这是否是由于模型本身的问题所造成的. Ostrovsky和 Grue^[29]利用黎曼不变量在Miyata-Choi-Camassa 方程的基础之上导出一个单向传播的强色散强非线性唯象模型

(10) $\begin{eqnarray} \eta_t + c(\eta)\eta_x + (\beta(\eta)\eta_{xx})_x = 0 \end{eqnarray}$

式中$c(\eta)$表示非线性速度, 而

$\beta(\eta) = \frac{1}{6}c(\eta)(h_1+\eta)(h_2-\eta)$

则是KdV方程色散项系数的推广.

1.1.3 完全非线性的位势方程

在忽略两层液体间表面张力的情况下, 完全非线性的位势流方程可表示为如下的形式:

(1) 流体的速度位势在各自区域内满足Laplace方程: $\Delta\phi_{1}=\Delta\phi_{2}=0$;

(2) 流体在上下固壁上满足无穿透边界条件: $ \phi_{1z}=\phi_{2z}=0$;

(3) 在两层流体交界面$z=\eta(x,t)$上满足运动学边界条件(kinematic boundary condition)与动力学边界条件(dynamics boundary condition), 即

(11) $\begin{eqnarray} &&\eta_t=\phi_{1z}-\eta_x\phi_{1x}=\phi_{2z}-\eta_x\phi_{2x}\\ \end{eqnarray}$

(12) $\begin{eqnarray}&&\rho_1\left(\phi_{1t}+\dfrac12|\nabla\phi_1|^2+g\eta\right)-\\&&\qquad\rho_2\left(\phi_{2t}+\dfrac12|\nabla\phi_2|^2+g\eta\right)=0 \end{eqnarray}$

Turner和 Vanden-Broeck^[30]利用速度图变换和边界积分法对大振幅的内孤立波进行了数值计算, 他们发现随着振幅的增大内孤立波的脉冲部分变得越来越平、越来越宽, 最终演变成共轭流(conjugate flow). Laget和Dias^[31]利用相同的数值方法进行了计算, 并将结果与Koop和 Butler^[22]的实验数据进行了比较. Kataoka^[32]采用渐近分析技巧与数值谱分析法, 对内孤立波进行了纵向(二维扰动)和横向(三维扰动)的稳定性分析, 得到的基本结论是: 随着振幅的增大内孤立波出现不稳定, 但横向不稳定出现在纵向不稳定之前.

非线性位势方程框架下的内界面波计算一般采用边界积分方法(boundary integral method). 为了提高计算的精度和效率, 通常有两种方法对其进行改进: 基于单复变函数理论(主要是保形映射与Cauchy积分公式)的高效算法, 以及基于Newton位势理论的双层位势法.

对于行波解(包括孤立波与周期波), 保形变换可在保持Laplace算子不变的前提下将自由边界问题转化为固定边界问题, 从而简化了数值计算格式的设计; 通常的保形变换是将自变量 $(x,y)$与因变量$(\phi,\psi)$的位置互换(其中$\phi$表示流体的速度位势, 而$\psi$ 代表流函数), 所以有时这种变换也称为速度图变换(hodograph transformation). 这一方法广泛应用于单层流体的自由边界问题; 对于两层流体的自由界面问题而言, 由于在界面上切向速度并不连续(但法向速度连续), 因此需要引入一个未知的辅助函数将界面两侧的速度位势连接起来, 这一方法很早出现在Vanden-Broeck^[33]的研究中, 用于计算两层流体界面上的重力毛细波, 后被应用于计算重力内波的极限构型^[34]及其他相关问题. 虽然保形变换方法便于程序化, 但此法若想推广至非定常流的计算有一定困难. 另外, 在物理空间中直接使用Cauchy公式设计算法也为大家广泛接受, 特别是使用弧长作为参数来描述界面形状, 如文献^{[35,36,37,38]}. 受限于数学理论基础, 基于单复变理论的算法均无法推广至三维.

利用Laplace方程的基本解推导双层位势法, 并结合拟谱方法可设计出高精度的计算格式. 此法最早由Baker^[39]提出, 一般称其为广义涡方法(generalized vortex method). Hou等^[40]以弧长和切向角为因变量重构控制方程, 进一步发展的这一方法. 广义涡方法的优势在于, 此法可用于计算行波解^[41-43]、驻波解^[44-45]与非定常流^[39-40]. 并且由于Laplace方程基本解在任意维空间中存在, 因此可以相对简单地推广到三维内波的计算之中.

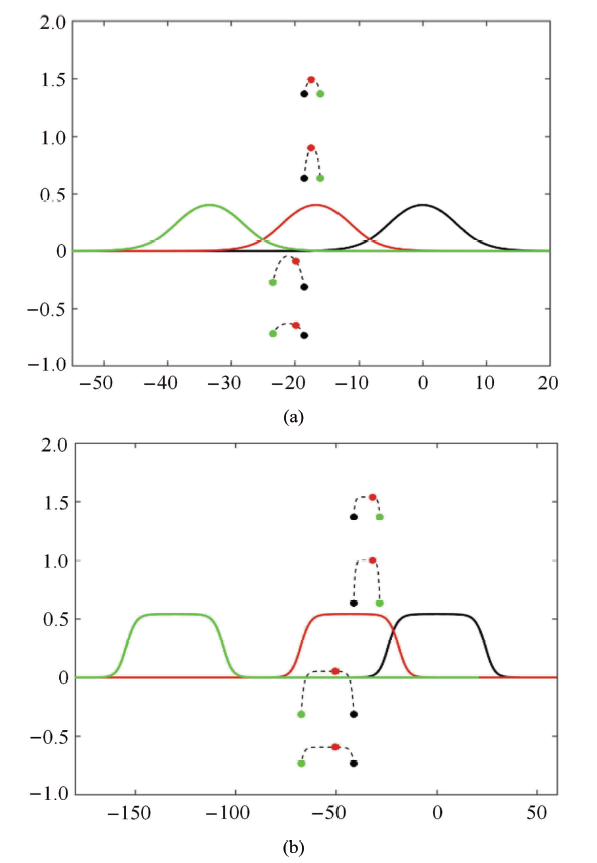
强内波表现出强烈的“流”的性质, 这一点可从引言中的海洋平台事故中认识到, 平台在强内波作用下能够漂移出很远的距离. 对于完全非线性的位势流问题, Wang 和Guan^[46]计算了内波作用下水质点的运动轨迹; 图7给出了内孤立波作用下水质点运动的例子. 随着时间推进,内波与水质点依黑红绿的顺序演化. 考虑到内波波长以公里计, 水质点事实上在水平方向经历了非常长距离的迁移.

图7

新窗口打开|下载原图ZIP|生成PPT
图7水质点在内孤立波诱导的剪切流场作用下的运动规律. 内孤立波由左向右传播(依黑红绿的顺序), 对应水质点的位子也以相应的颜色标记. 数值计算对于完全非线性的势流问题进行模拟, 其参数选取为: 密度比为0.9, 水深比为2(上层比下层)

Fig.7Particle trajectories under internal solitary waves. Internal solitary waves move from left to right labeled with black, red, and green in order, and particle positions are marked as the corresponding colors. Numerical experiments were carried out in the fully nonlinear irrotational equations with the density ratio of 0.9 and depth ratio of 2

1.1.4 带自由面的二流体系统

对于带自由面二流体系统, 其数学描述只需要将上边界的无穿透条件$\phi_{1z}=0$ 换成运动学与动力学边界条件

(13) $\begin{eqnarray} &&\widetilde{\eta}_t=\phi_{1z}-\widetilde{\eta}_{x}\phi_{1x} \\ \end{eqnarray}$

(14) $\begin{eqnarray}&&\phi_{1t}+\dfrac12|\nabla\phi_1|^2+g\widetilde{\eta}=0 \end{eqnarray}$

其中$z=\widetilde{\eta}(x,t)$ 代表上边界的自由面. Kodaira等^[47]与Forgia和 Sciortino^[48]均从理论和实验两方面考察了自由表面边界条件(13)和(14)对于内孤立波的影响, 他们的研究均表明, 与“刚盖假设”相比, 自由边界条件对于内孤立波的波形和波速都有一定的影响, 而自由边界条件下导出的Miyata-Choi-Camassa与实验结果吻合得很好.

带自由面的二流体系统的简化模型一般以长波近似为主(内波的波长远大于上层流体的厚度). 除文献^[47]中的Miyata-Choi-Camassa方程外, Craig等^[49]利用系统的Hamilton结构以及Dirichlet-Neumann算子的解析性质, 得到了一系列的长波模型; Haut和 Ablowitz^[50]利用Fokas发明的用于处理线性偏微分方程和可积系统边值问题的一般方法—全局关系法(global relation), 构造了一个带自由面的完全非线性二流体系统的等价描述, 从而能够以一种相对统一的方式推导出各种弱非线性模型. 其他的长波模型还包括文献^[51,52,53]等, 用于研究广义孤立波、内嵌孤立波、波前、共轭流等非线性波动现象.

关于数值模拟, 带自由面的二流体系统的完全非线性位势流问题的高精度高效算法也受到越来越多的重视. Moni和 King^[54]利用速度图变换和边界积分法得到正压(barotropic)孤立波(即上自由面与内部的界面具有相同的相位)的数值解. 由于长短波的相互作用, 广义孤立波在带自由面的二流体系统中广泛存在, 此类波在尾部是小振幅的周期波动, 而在中间则是一个大振幅的孤立脉冲. 内广义孤立波在位势流方程中的数值解由Michallet等^[23,55]、 P$\breve{a}$r$\breve{a}$u和Dias^[56], 以及Wang等^[57]得到. 必须指出的是他们的数值方法无法用于计算大振幅的波形; 在处理类似问题时, 利用柯西积分公式代替傅里叶变换的方案可以解决这一数值缺陷(感兴趣的读者可阅读参考文献^[58,59]等).

1.2 二维三层模型

实际海洋中的密度层结大致是一个三层结构(见图2), 因此近年来研究人员逐渐地从两层流体模型过渡到三层模型. 关于三层结构最早的数值计算由Vanden-Broeck和 Turner^[60]与Sha和 Vanden-Broeck^[61]完成, 得到正压(barotropic) 和斜压(baroclinic)的广义孤立波解; 在计算中他们将上层和下层的密度设为常数, 而将中间层的密度设为一个线性函数用于连接上下层. 此后, Rusas和 Grue^[58]采用Cauchy积分公式与边界积分法对三层密度均为常数的情形进行了数值研究, 而Fructus和 Grue^[59]则考虑了三层密度均为线性函数的情形.

在实验研究方面, 里昂物理实验室开展“死水现象”的部分实验采用了三层密度模式^[1]. 纽卡斯尔大学的Magda Carr教授及其合作者近几年对三层模型开展了一系列的实验研究; 实验采用传统的“重力塌陷法”造波, 可得到二模态的孤立波(即斜压孤立波)群(见图8). Carr等^[62]考察了二模态内孤立波的稳定性; Deepwell等^[63]研究二模态内孤立波越过局部地形时的发展演化与模态转化规律; Carr等^[64]从实验和数值两方面研究二模态内孤立波的爬坡问题.

图8

新窗口打开|下载原图ZIP|生成PPT
图8上图: 重力塌陷法生成内波的实验设计示意图.下图: 使用DigiFlow的时间序列功能得到二模态内孤立波群(具体参数详见文献^[62])

Fig.8Experimental setup for three-layer internal waves (top); group of mode-2 internal solitary waves (bottom). See Ref.^[62] for more details

2 连续密度分层

密度间断模型从表面上来看简化了我们所要处理的问题, 对于研究具有清晰界面的多相系统(如两种不相溶液体间的界面问题)提供了一个非常好的近似. 但我们必须指出, 从数学上来讲, 由于Kelvin-Helmholtz不稳定性的存在, 整个偏微分系统的适定性(指解的存在性、唯一性和稳定性)是值得深究的. 事实上, Ambrose^[65]利用泛函分析的方法从理论上严格证明了两层密度间断系统在不考虑界面上表面张力的情况下, 非定常流的模型是不适定的. 这一事实给我们的数值计算(特别是非定常流动的计算)造成了很大的困扰. 因此,采用密度连续分层的模型既符合海洋的物理实际也消除了密度间断模型所固有的不良性质.

2.1 密跃层整体的脉动

密跃层的整体脉动在海洋系统的质量、动量与能量的转移和传递过程中扮演着极其重要的角色. 采用连续密度分层模型以后, 我们不再假设密跃层是一个突变界面. 此时一般采用Boussinesq假设(即在浮力驱动的流动中假设密度变化只出现在重力项中)对问题稍加简化. 弱非线性的情形依然可以用KdV方程近似描述整个系统, 但完全非线性的控制方程与间断密度分层模型相比则要更加复杂.

2.1.1 弱非线性理论

本节中我们简要介绍连续分层流体在浅水中的弱非线性理论. 对于Boussinesq 近似下的二维Euler方程组, 假设背景密度函数$\rho_0+\tilde \rho(z)$ 依赖于垂直坐标, 其中$\rho_0$ 表示恒定的参考密度. 选择合适的尺度对方程进行无量纲化得到关于流函数$\psi$ 以及密度扰动$\rho’$的控制方程(具体推导过程可见文献^[66,67])

(15a) $\begin{eqnarray}&& \dfrac{\partial }{\partial t} \psi_{zz} - b_x = \varepsilon J(\psi, \psi_{zz})-\mu\dfrac{\partial }{\partial t} \psi_{xx} + \varepsilon\mu J(\psi, \psi_{xx})\qquad\\ \end{eqnarray}$

(15b) $\begin{eqnarray}&& b_t + N^2(z)\psi_x = \varepsilon J(\psi, b) \end{eqnarray}$

其中速度场满足$(u, w) = (\psi_z, -\psi_x)$, $b = g\rho’$, $J(A, B) = A_xB_z-A_zB_x$以及

$\begin{eqnarray*} N(z)=\sqrt{-\dfrac{g}{\rho_0} \dfrac{\partial \tilde \rho}{\partial z}} \end{eqnarray*}$

为浮频率. KdV方程的推导基于流函数$\psi$和扰动密度$\rho’$关于两个小参数$\varepsilon$ 和$\mu$ 的渐进展开, 其一般形式为

$\begin{eqnarray*} &&\psi = A(x,t)\phi(z) + \varepsilon A^2(x,t)c^2\phi^{(1,0)}(z) +\\&&\qquad\mu A_{xx}(x,t)c\phi^{(0,1)}(z) +\varepsilon^2A^3(x,t)\phi^{(2,0)}(z)+ \cdots\\ &&b = A(x,t)\frac{N^2(z)}{c}\phi(z) + \varepsilon A^2(x,t)D^{(1,0)}(z) + \\&&\qquad\mu A_{xx}(x,t)D^{(0,1)}(z) + \varepsilon^2A^3(x,t)D^{(2,0)}(z) + \cdots \end{eqnarray*}$

其中每项都可写成分离变量解的形式. 振幅函数$B(x,t)$ 满足

(16) $\begin{eqnarray} B(x,t) = \dfrac{A(x,t)}{c} \end{eqnarray}$

由$O(1)$项可以导出$\phi(z)$满足如下特征值问题

(17) $\begin{eqnarray} \mathcal{L}(\phi) = \phi''+\dfrac{N^2}{c^2}\phi = 0 \end{eqnarray}$

相应边界条件为

(18) $\begin{eqnarray} \phi(0) =\phi(1) = 0 \end{eqnarray}$

其中$c$表示波的相速度. 波的振幅函数$B(x,t)$满足

(19) $\begin{eqnarray} B_t + cB_x = 0 \end{eqnarray}$

由$O(\varepsilon) $和$O(\mu)$项可以得到经典的KdV方程

(20) $\begin{eqnarray} B_t + cB_x + \varepsilon\alpha BB_x + \mu\beta B_{xxx} = 0 \end{eqnarray}$

其系数可以通过相应的$\phi^{(i,j)}$来求解. 考虑$O(\varepsilon ^2)$项时可以导出eKdV方程

(21) $\begin{eqnarray} B_t + cB_x + \varepsilon\alpha BB_x + \mu\beta B_{xxx}+ \varepsilon^2\alpha_1 B^2B_x = 0 \end{eqnarray}$

2.1.2 完全非线性理论

连续分层流体中的大振幅内孤立波可以通过求解Dubreil-Jacotin-Long (DJL)方程来获得, 该方程用于描述定常不可压缩Euler流^[68-69]. 设 $\eta(x,t)$表示等密度位移, 在Boussinesq假设下DJL方程可以表示为

(22) $\begin{eqnarray} \nabla ^2 \eta + \frac{N^2(z-\eta)}{c^2}\eta =0 \end{eqnarray}$

此处$c$表示波的相速度, 需要和$\eta$一起求解. 其边界条件为

(23) $\begin{eqnarray} \eta(x,0) = \eta(x,H) = 0 , \ \ \mathop{\lim}\limits_{x\to \pm \infty} \eta(x,z) = 0 \end{eqnarray}$

在没有Boussinesq假设时, 相应形式的DJL方程可表示为

(24) $\begin{eqnarray} &&\nabla ^2 \eta + \dfrac{N_{\rm NB}^2(z-\eta)}{c^2}\eta + \\&&\qquad\dfrac{N_{\rm NB}^2(z-\eta)}{2g} \left[\eta_x^2+\eta_z(\eta_z-2)\right] = 0 \end{eqnarray}$

此时, 浮频率$N_{\rm NB}^2 = -\dfrac{g}{\widetilde{\rho}(z)} \dfrac{\partial \widetilde{\rho}}{\partial z}$. 对于背景流场 $U(z)$对内孤立波的影响, 可以参考Stastna和 Lamb^[70]的研究.

2.2 密跃层内部的波动

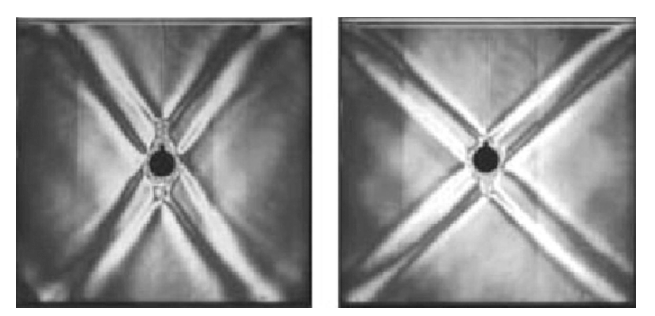
2.2.1 圣$\cdot $安德鲁十字现象

连续分层流体中的另一个基本现象是所谓的圣$\cdot $安德鲁十字(St. Andrew’s Cross), 它通常是由在局部区域上周期性地扰动密度均匀分层流体所导致的. Mowbray 和 Barity^[71]的开创性工作表明, 当他们将一个圆柱体横置于密度一致分层的流体中(即流体在未经扰动时密度与深度呈线性关系)并使其做周期性的上下振动时, 通过纹影法观察到从振动源长出四条“胳膊”, 而局部扰动正是沿着这些胳膊的方向进行传播的(见图9). 每个胳膊相对于水平方向具有相同的倾角, 该角度由振荡频率$\omega $和浮频率$N$ 决定.

图9

新窗口打开|下载原图ZIP|生成PPT
图9(纹影图)密度分层流体中由垂直方向周期振动的小圆柱产生的四个内波波束形成圣$\cdot $安德鲁十字现象, 不同频率的振动可产生不同的夹角: 左图振动周期为5 s, 右图为6 s (http://www.gfd-dennou.org/library/gfd_exp/exp_e/doc/iw/guide01.htm)

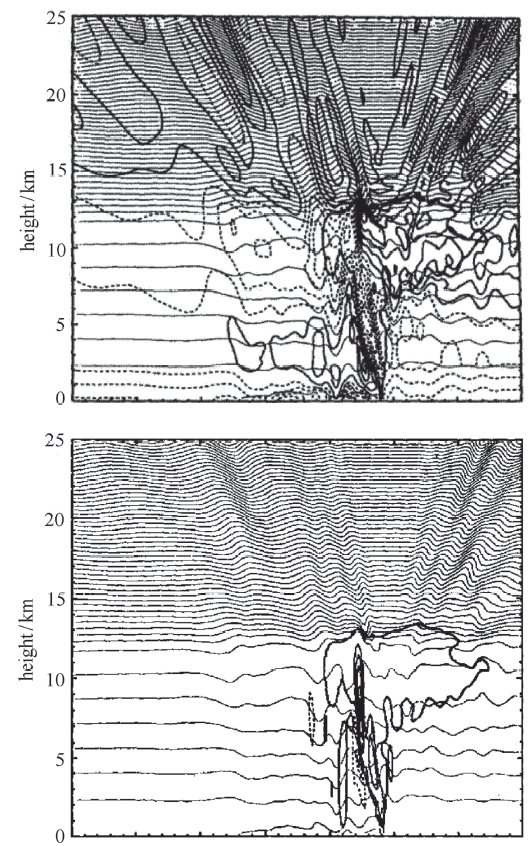
Fig.9Visualization of St. Andrew’s cross excited by a vertically oscillating circular cylinder in a density stratified fluid with constant buoyance frequency. The angle of arms is determined by the period (or frequency equivalently) of oscillation which is 5 s on the left and 6 s on the right (http://www.gfd-dennou.org/library/gfd_exp/exp_e/doc/iw/guide01.htm)

圣$\cdot $安德鲁十字是大气地球物理流体力学中一个十分基本且极端重要的现象. 这一现象告诉我们一个基本的事实, 即在一个密度分层的流体当中, 其内部波动的传播有别于我们所熟知的海洋表面波动: 区别在于海洋表面波动只能沿着水平方向传播, 而内部波动的传播可沿任何方向进行, 而在密度一致分层流体中的传播方向仅由扰动源的振动频率与浮频率之比所决定(见文献^[72]). 圣$\cdot $安德鲁十字这种可以在垂直方向上传播的特性在大气与海洋动力学中发挥着至关重要的作用. 如数值计算与实际观测的结果表明, 大气中对流层顶部的雷电造成的局部扰动可向上传播至平流层并呈现出扇状的结构(见图10), 这一平流层与对流层的动态耦合对对流层的循环造成重大影响^[73]. Fovell等^[74]及Alexander等^[75]探讨了由二维飑线(在气象上, 飑线是指局部范围内气压和风发生突变的狭窄强对流天气带; 从天气雷达图上看, 飑线就像糖葫芦一样穿起一串雷电)产生的重力内波, 并将之类比于机械振子, 这就是大气中的圣$\cdot $安德鲁十字现象; 但由于飑线内的振动频率不唯一, 故而观察到的是扇形结构的波动而非交叉十字.

图10

新窗口打开|下载原图ZIP|生成PPT
图10纬向速度的等高线(上)、垂直速度的等高线(下)、温度等高线(虚线), 以及云的轮廓(粗线). 对流层顶端在离地12 km左右的高空^[74]

Fig.10Contours of zonal velocity (upper panel) and vertical velocity (lower panel) superposed on temperature contours (dashed lines) and cloud contours (heavy solid lines) near the 12 km level^[74]

对于一个连续分层的Boussinesq流体, 利用未经扰动的密度仅依赖于垂直坐标以及小振幅运动的假设, 可以得到线性化方程的色散关系

(25) $\begin{eqnarray} \omega = N\sqrt{\dfrac{k_x^2+k_y^2}{k_x^2+k_y^2+k_z^2}} = N \cos\theta \end{eqnarray}$

其中, $\theta$表示波数向量$K = (k_x, k_y, k_z)$ 与水平面的夹角. 设静止状态下流体的密度为$\rho_0+\widetilde{\rho}(z)$ 是关于$z$的线性函数, 则浮频率$N$为常数. 色散关系表明连续分层流体中内波的频率仅取决于波数矢量的方向, 而与其大小无关, 这与界面波形成鲜明对比. 此外一旦确定了分层结构, 波的频率就决定了它的传播角度. 由色散关系以及波的相速度与群速度的定义得到

$\begin{eqnarray*} &&{c}_p = \dfrac{\omega}{K}{e}_{K} = \dfrac{\omega}{K^2}(k_x, k_y, k_z)\\ &&{c}_g = \dfrac{{\rm d}\omega}{{\rm d} {K}}=\dfrac{Nk_z}{K^3\sqrt{k_x^2+k_y^2}} \lt[k_xk_z, k_yk_z, -(k_x^2+k_y^2)] \end{eqnarray*}$

因此, ${c}_p\cdot{c}_g=0$, 即相速度矢量和群速度矢量是垂直的. 这从理论上解释了四条胳膊产生且与水平方向夹角相同的原因. 图9是二维情况下不同频率的振动得到的实验观测结果(http://www.gfd-dennou.org/library/gfd_exp/exp_e/doc/iw/guide01.htm); 图11是对Boussinesq方程进行高精度直接数值模拟得到的三维情况下的圣$\cdot $安德鲁十字现象, 代替四条波束的是一个圆锥, 与线性理论预测一致(见文献^[76]).

图11

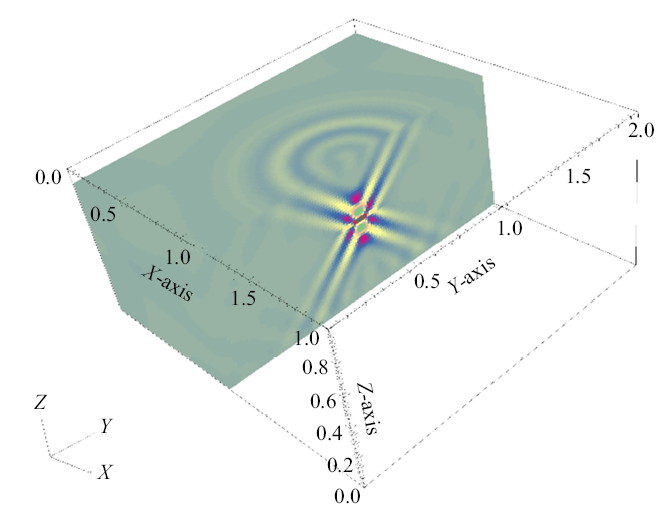新窗口打开|下载原图ZIP|生成PPT
图11直接数值模拟得到三维情况下的圣$\cdot $安德鲁十字现象, 对Boussinesq方程采用时空一致四阶精度的计算格式得到

Fig.11DNS of St. Andrew’s cross in 3D Boussinesq equations based on a fourth-order (in both space and time) numerical scheme

2.2.2 圣$\cdot $安德鲁十字的理论与数值进展

近几十年来, 对圣$\cdot $安德鲁十字的理论分析主要依靠对线性的Boussinesq方程的深入研究. Thomas和 Stevenson^[77]找到了考虑黏性时的相似解, 他们也使用振动圆柱进行了实验, 并发现此相似解与实验结果吻合得很好; 理论与实验表明, 黏性项会使波束的宽度增加而振幅减小. Gordon等^[78]将理论拓展到浮频率为非常数的情形, 此时四条波束并非笔直延伸. Hurley和 Keady^[79]在最近的理论研究中拓展了其对振动椭圆柱的无黏结果, 得到了黏性近似解, 在远场时接近Thomas和 Stevenson的相似解. 该理论预测与Sutherland和 Linden^[80]报导的垂直振荡椭圆柱的详细实验观察具有很好的一致性. 关于非线性内波束的理论研究, Tabaei和 Akylas^[81]的工作表明, 在具有恒定浮频率的Boussinesq流体中, 非线性效应在有限振幅内波的传播中并不显著. 但他们同时也指出, 不同于单波束的传播规律, 当考虑两个沿着不同方向传播的波束相互作用时, 非线性效应变得非常重要^[82].

数值方面的研究大多基于对Boussinesq近似下的二维不可压缩的Navier-Stokes方程的求解, 而小球或圆柱的振动由一个外加的体积力近似

(26a) $\begin{eqnarray} && \frac{\partial u}{\partial x} + \frac{\partial v}{\partial z} =0\\ \end{eqnarray}$

(26b) $\begin{eqnarray} && \frac{\partial u}{\partial t} + u\frac{\partial u}{\partial x} + v\frac{\partial u}{\partial z} = -\frac{p_x}{\rho_0}+\nu \Delta u\\ \end{eqnarray}$

(26c) $\begin{eqnarray}&& && \frac{\partial v}{\partial t} + u\frac{\partial v}{\partial x} + v\frac{\partial v}{\partial z} = -\frac{p_z}{\rho_0} - \frac{\rho g}{\rho_0} + \nu \Delta v + F\\ \end{eqnarray}$

(26d) $\begin{eqnarray}&& \frac{\partial \rho}{\partial t} + u\frac{\partial \rho}{\partial x} + v\frac{\partial \rho}{\partial z} + v\frac{\partial \widetilde{\rho}}{\partial z} = 0 \end{eqnarray}$

其中$u$和$v$分别表示水平和垂直方向的速度分量, $\nu$ 为动力学黏性系数, 密度则由三个分量表示: 恒定的参考密度$\rho_0$、静止密度$\tilde \rho$和扰动密度$\rho$. $F$为外力强迫项, 用于描述圣$\cdot $安德鲁十字中圆柱的简谐振动^[83-86]. 其中Liu^[87]尝试采用了开放边界条件(open boundary condition)进行内波的数值计算, 开放边界条件允许在计算域内部生成的波传播至计算域外, 从而减少边界所导致的波反射, 然而开放边界条件本身可能引入数值反射和不稳定性, 同时不利于高阶格式的设计. Javam等^[84]用数值方法研究了二维情况下内波在斜边界的反射, 以及两个振动源所产生的波束的相互作用. 数值上, 他们采用非交错网格上的有限体积法进行离散, 用SIMPLE策略求解Navier-Stokes方程. 结果显示, 在两束波的交互区域出现了除振源频率$\omega$ 以外的其他频率, 能量积聚并导致密度倒置. Diamessis等^[88]提出了用于求解高雷诺数紊流的带罚多域谱元方法(spectral multidomain penalty method). 该方法被用于研究在刚盖假设下的内波在海面的反射和在密跃层附近的折射和反射.

2.2.3 内波湍流与潜艇隐身

密度分层流体内部波动可以垂向传播的性质在海洋军事尤其是水下非声探测上有潜在应用. 在现代战争中, 潜艇是海军作战的重要力量, 无论是用于击沉敌舰还是在海面下隐藏核弹, 潜艇的隐蔽性令其极具威慑力. 传统上, 声呐是各国海军进行水下监视所使用的主要技术, 用于对水下目标进行探测、分类、定位和跟踪. 但随着降噪技术的不断升级, 潜艇已经能够在这场探测与反探测的较量中与声呐势均力敌. 为了提高海军反潜作战的能力, 发展新的探测手段是当务之急. 潜艇水下航行会在海面产生不同类型的水动力尾迹, 包括伯努利水丘(Bernoulli bump)、开尔文尾迹(Kelvin wake)、涡尾迹(vortex wake)、湍流尾迹(wake turbulence)等(见图12所示). 潜艇近自由面航行时, 海面会产生驼峰一样的凸起以及V字形波形, 分别被称为伯努利水丘与开尔文尾迹. 这两种尾迹原则上可通过机载或星载雷达的遥感手段进行精确探测, 但由于它们在海洋表面上所反映出来的波高随潜艇速度和深度的增大而迅速减小, 潜艇可以通过改变航行的速度和深度轻易地隐藏这两种尾迹. 但是当潜艇在密度分层的流体中运动时, 其湍流尾迹造成的扰动便可向上传播; 当其传播到水面时就会改变海洋表面波浪场的特征, 进而引发遥感雷达后向散射特性的变化, 我们便有可能据此进行潜艇探测.

图12

新窗口打开|下载原图ZIP|生成PPT
图12水下潜艇产生的各种尾迹示意图, 包括开尔文尾迹、内波、湍流尾迹、涡尾迹、煎饼旋涡; 图片来自网络

Fig.12Various wakes generated by motion of submarine: Kelvin wake, internal waves, wake turbulence, vortex wake, and pancake eddies. The figure comes from the internet

海洋中潜体产生的湍流尾迹是海洋内波形成的重要来源之一^[89-91]. 研究这一问题的常用方法是采用连续分层水体中运动球体来生成湍流尾迹, 并由湍流尾迹来辐射生成内波, 这一做法的好处是用来产生湍流的球体本身就符合地球物理学和海军研究对象的基本几何特征. 运动小球产生的湍流尾迹通常用雷诺数(Reynolds number)和弗汝德数(Froude number)来表征. 以往的实验结果表明在雷诺数$Re$达到$3.0\times 10^{4}$和弗汝德数满足 $0.5 \leqslant Fr \leqslant 12.7$的条件下有两种内波形成机理. 第1种称为“Lee wave” 机理. 这种内波生成需要满足$Fr \leqslant 4$ 的条件; 第2种情形主要由湍流尾迹辐射出拟随机的内波, 它需要满足 $Fr \geqslant 4$的条件^[92-95]. Spedding等^[96]采用了拖曳小球的方式来产生湍流进而产生内波, 他将实验参数设为$Re=1.2\times 10^{4}$, $Fr \in \{ 4, 64\}$. 这是首次有人尝试使用观测的内波特性来反演湍流尾迹的控制参数, 并且成功得到了观测内波波长与$Fr^{1/3}$ 成正比关系.

相比于实验室观测, 数值计算可以在更高的雷诺数和弗氏数下进行研究. Abdilghanie 和Diamessis^[91]将雷诺数提高到10$^{5}$、弗汝德数提高到64, 在这样的参数条件下对这一问题进行了数值模拟. 与低雷诺数的结果对比, 他们发现在更高雷诺数下湍流尾迹辐射内波的时间长度有显著提高, 这种现象是由于受到二次Kelvin-Helmholz不稳定性影响所导致的^[97-99]. 进一步的研究表明随着雷诺数的增大, 内波的生成受黏性作用影响降低^[100], 更多的动量从湍流尾迹中被抽取出来, 由此导致内波携带的动量显著增大^[101].

综上所述, 利用潜艇运动产生的湍流尾迹及其在密度分层流体中的垂向传播, 并借助于现代遥感技术反演潜艇的位置和航行速度, 是非声探测的潜在发展方向. 而对于己方潜艇, 我们则需设法降低潜艇的水动信号特征以达到反探测的目的. 因此对这一领域开展理论分析、高精度数值模拟和实验室机理实验研究具有重要的应用价值.

3 展望

尽管人们对海洋内波的研究工作已开展多年, 但随着人类对海洋探索的不断深入以及海洋工程和海洋军事的迫切需求, 我们必须重视海洋内波, 尤其是我国南海海域内波的研究. 在内波的理论、建模与计算方面, 以下的研究方向值得关注.

3.1 内波中的多界面耦合问题

(1) 内波与海洋表面波的相互作用: 大振幅内孤立波诱发海洋表面的激潮现象, 为使用卫星遥感图片反演内孤立波提供了途径, 具有重要的应用价值; 但这一问题的多尺度效应极为明显, 以早期印度洋东部安达曼海实测数据为例, Perry 和Schimke^[2]观测到南北(波脊线方向)长30 km、东西宽200$\sim $800 m的波浪区, 这样的浪区至少有5个, 间隔约为3200 m, 其中的波浪是高为0.3$\sim $0.6 m、波峰很尖的短波, 他们通过测量仪器辨明了浪区下有振幅达80 m、长约2 km的内孤立波通过; 这里的尺度仅在空间上来讲就需要考虑米量级(表面短波)、百米量级(表面波波包)、千米量级(内波)等; 目前一般使用射线理论(Ray theory)来解释“激潮现象”(见文献^{[47-48, 102]}等), 但仍存在一定的争议; 因此构造新的多尺度模型或者发展高精度的计算方法用于理解内孤立波激发海洋表面波群的机理, 以及确定两个自由面间的定量关系是当务之急.

(2) 内波高阶模态的性质: 内波的高阶模态一般无法用“两层模型+刚盖假设”来描述, 但当外在条件(如海底地形)变化时内波模态之间的相互转换具有重要的科学意义(见文献^[63]中的实验研究和文献^[103]中的理论研究); 为深刻理解这其中的关键, 发展三层乃至更多层的内波模型研究多界面耦合问题是一个有意义的方向.

3.2 三维内波问题

如前所述, 二维内波问题经过几十年的发展, 已经在理论研究、多尺度建模、高精度计算等方面取得了明显的进展; 相对而言, 三维内波问题的研究才刚刚起步. 之前少量关于三维内波的研究主要是利用Kadomtsev-Petviashvili (KP)方程展开讨论的(如文献^[104,105]等); 一般常系数的KP方程是一个可积系统(integrable system), 在开展理论研究时具有诸多优点. 但是KP方程在研究实际问题时有两个固有的缺陷: (1) KP方程是一个各向异性的方程 (即波前进方向的尺度与波展向的尺度不同), 这并不适合研究像内波Kelvin尾迹(见图13)和波-波斜相互作用(见图14)这样的问题; (2) KP方程是一个单向传播的模型(即假设波几乎只朝一个方向传播), 这样就无法反映海洋内流遇到海底局部隆起地形时引发的波反射现象. 因此, 发展各向同性、双向传播的多尺度三维内波模型用于研究海底地形对内波的影响以及内波与内波之间的斜相互作用就显得非常重要.

图13

新窗口打开|下载原图ZIP|生成PPT
图13乔治亚海峡的内波Kelvin尾迹的SAR图像^[106]

Fig.13SAR image of a Kelvin wake in the strait of Georgia^[106]

图14

新窗口打开|下载原图ZIP|生成PPT
图14安达曼海域的水深填色图. 卫星遥感图片是ERS-2卫星在1997年2月11日0359UTC于安达曼海域拍摄的合成孔径雷达(SAR)图片, 展示了多个内孤立波(波列)以及波-波相互作用. 此图由中国海洋大学袁春鑫博士提供

Fig.14Bathymetric map of part of the Andaman Sea (left panel). SAR image taken by ERS-2 satellite in the Andaman Sea on February 11, 1997 (right panel) shows wave-wave interactions of internal solitary waves. This figure is provided by Dr. Yuan from Ocean University of China

3.3 密度连续分层模型的高精度数值计算

近来, 对于圣$\cdot $安德鲁十字和潜艇湍流尾迹的海洋表面特征等内波现象的研究已逐渐从理想模型过渡到实际应用(如文献^[76]中研究了南海真实浮频率下的圣$\cdot $安德鲁十字与潜艇湍流尾迹, 见图15). 但目前的研究还是主要集中在远场的性质(即远离运动物体的流场的行为). 为了获得近场的性质(即靠近运动物体的流场性质), 必须对带非规则动边界的Boussinesq方程或者Navier-Stokes方程发展高阶精度的算法. 这类算法除了能分析分层流体中潜艇运动所诱导的流场及潜艇本身的受力情况之外, 还能直接应用于内波对各种海洋结构物和部件(平台、立管、锚链等)的作用力计算, 是进一步分析海洋结构物的响应、强度和疲劳的前提和基础.

图15

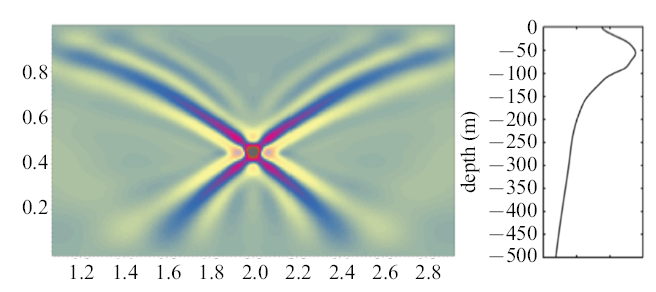新窗口打开|下载原图ZIP|生成PPT
图15中国南海实际浮频率下的圣$\cdot $安德鲁十字现象, 采用时空一致四阶精度的算法对Boussinesq方程进行直接数值模拟, 其中雷诺数为$2.5\times 10^{4}$^[76]

Fig.15St. Andrew’s cross in the South China Sea. The fourth-order numerical scheme was used to carry out the numerical experiment in the Boussinesq equations with the Reynolds number of $2.5\times 10^{4}$^[76]

致谢

感谢中国海洋大学袁春鑫博士、浙江大学张庆海教授及黎志轩为本文提供素材; 感谢中科院力学研究所劳铁涛及期刊编辑在文字排版上给予的帮助.

参考文献原文顺序
文献年度倒序
文中引用次数倒序
被引期刊影响因子

[1]

Mercier

, Vasseur

, Dauxois

. Resurrecting dead-water phenomenon
Nonlinear Processes in Geophysics, 2011,18:193-208

DOI URL [本文引用: 2]

[2]

Perry

, Schimke

. Large-amplitude internal waves observed off the northwest coast of Sumatra
Journal of Geophysical Research, 1965,70(10):2319-2324

DOI URL [本文引用: 2]

[3]

Osborne

, Burch

. Internal solitons in the Andaman Sea
Science, 1980,208(4443):451-460

DOI URL PMID [本文引用: 1]

The solitary wave is a localized hydrodynamic phenomenon that can occur because of a balance between nonlinear cohesive and linear dispersive forces in a fluid. It has been shown theoretically, and observed experimentally, that some solitary waves have properties analogous to those of elementary particles, and the waves have therefore been named solitons. During a measurement program in the Andaman Sea near northern Sumatra, large-amplitude, long internal waves were observed with associated surface waves called tide rips. Using theoretical results from the physics of nonlinear waves, it is shown that the internal waves are solitons and their interactions with surface waves are described.

[4]

Duda

, Lynch

, Irish

, et al. Internal tide and nonlinear internal wave behavior at the continental slope in the northern South China Sea
IEEE Journal of Oceanic Engineering, 2005,29(4):1105-1130

DOI URL [本文引用: 1]

[5]

5种劲松, 周晓中 . 合成孔径雷达图像海洋内波探测研究综述
雷达学报, 2013,2(4):406-421

DOI URL [本文引用: 1]

近年来，利用合成孔径雷达(Synthetic Aperture Radar, SAR)图像进行海洋内波探测在海洋遥感领域受到极大关注，成为SAR 重要的海洋应用之一。该文围绕SAR 图像海洋内波探测专题，综述了具有代表性的国内外研究成果，分别在内波参数反演方法、雷达参数及风场对SAR 内波成像的影响、内波2 维SAR 成像仿真等3 个研究方面进行了详细的介绍以及归纳总结。

( Chong

Jinsong

, Zhou

Xiaozhong

. Survey of study on internal waves detection in synthetic aperture radar image
Journal of Radars, 2013,2(4):406-421 (in Chinese))

DOI URL [本文引用: 1]

Caponi

, Crawford

, Yuen

, et al. Modulation of radar backscatter from the ocean by a variable surface current
Journal of Geophysical Research Oceans, 1988,93(C10):12249-12263

[本文引用: 1]

[7]

Alpers

. Theory of radar imaging of internal waves
Nature, 1985,314(6008):245-247

DOI URL [本文引用: 1]

[8]

Jackson

. Internal wave detection using the moderate resolution imaging spectroradiometer (modis)
Journal of Geophysical Research: Oceans, 2007,112:C11012

[本文引用: 1]

[9]

李家春 . 水面下的波浪--海洋内波
力学与实践, 2005,27(2):3-8

[本文引用: 1]

( Li

Jiachun

. Billow under the sea surface-Internal waves in the ocean
Mechanics in Engineering, 2005,27(2):3-8 (in Chinese))

[本文引用: 1]

[10]

蔡树群, 何建玲, 谢皆烁 . 近10年来南海孤立内波的研究进展
地球科学进展, 2011,26(7):703-710

URL [本文引用: 1]

孤立内波是存在于海洋密度跃层中的非线性大振幅波动。综述了近10年来随着卫星遥感资料、现场观测资料的日益增多和数值模拟技术的发展，关于南海北部孤立内波的特征、形成的动力机制和传播演变规律及孤立内波在海洋工程中的载荷估算等方面所取得的研究成果，并讨论了未来南海孤立内波研究中值得关注的一些问题。

( Cai

Shuqun

, He

Jianling

, Xie

Jieshuo

. Recent decadal progress of the study on internal solitons in the South China Sea
Advances in Earth Science, 2011,26(7):703-710 (in Chinese))

URL [本文引用: 1]

孤立内波是存在于海洋密度跃层中的非线性大振幅波动。综述了近10年来随着卫星遥感资料、现场观测资料的日益增多和数值模拟技术的发展，关于南海北部孤立内波的特征、形成的动力机制和传播演变规律及孤立内波在海洋工程中的载荷估算等方面所取得的研究成果，并讨论了未来南海孤立内波研究中值得关注的一些问题。

[11]

蔡树群, 甘子钧 . 南海北部孤立子内波的研究进展
地球科学进展, 2001,16(2):215-219

URL [本文引用: 1]

孤立子内波是由内潮在特殊地形条件下所激发的存在于密度跃层中的大振幅波动。而在其传播过程中产生的扰动可导致海面海水强烈辐聚和突发性的强流(波致流),对海洋工程、石油钻井平台和海底石油管道会造成严重的威胁。介绍了国际上研究海洋中孤立子内波的动态;描述了南海北部存在的孤立子内波现象,讨论了目前南海孤立子内波研究中存在的一些问题,进而提出了今后开展进一步研究的思路。

( Cai

Shuqun

, Gan

Zijun

. Progress in the study of the internal soliton in the northern South China Sea
Advances in Earth Science, 2001,16(2):215-219 (in Chinese))

URL [本文引用: 1]

李家春, 程友良, 范平 . 海洋内波与海洋工程. 庆祝郑哲敏先生八十华诞应用力学报告会--应用力学进展论文集. 北京: 科学出版社, 2004: 41-45

[本文引用: 1]

[13]

Apel

, Ostrovsky

, Stepanyants

, et al. Internal solitons in the ocean and their effect on underwater sound
The Journal of the Acoustical Society of America, 2007,121:695-722

DOI URL PMID

Nonlinear internal waves in the ocean are discussed (a) from the standpoint of soliton theory and (b) from the viewpoint of experimental measurements. First, theoretical models for internal solitary waves in the ocean are briefly described. Various nonlinear analytical solutions are treated, commencing with the well-known Boussinesq and Korteweg-de Vries equations. Then certain generalizations are considered, including effects of cubic nonlinearity, Earth's rotation, cylindrical divergence, dissipation, shear flows, and others. Recent theoretical models for strongly nonlinear internal waves are outlined. Second, examples of experimental evidence for the existence of solitons in the upper ocean are presented; the data include radar and optical images and in situ measurements of wave forms, propagation speeds, and dispersion characteristics. Third, and finally, action of internal solitons on sound wave propagation is discussed. This review paper is intended for researchers from diverse backgrounds, including acousticians, who may not be familiar in detail with soliton theory. Thus, it includes an outline of the basics of soliton theory. At the same time, recent theoretical and observational results are described which can also make this review useful for mainstream oceanographers and theoreticians.

[14]

Joseph

. Solitary waves in a finite depth fluid
Journal of Physics A General Physics, 1977,10(12):L225-L227

DOI URL PMID [本文引用: 1]

Generalized solitary waves propagating at the surface of a fluid of finite depth are considered. The fluid is assumed to be inviscid and incompressible and the flow to be irrotational. Both the effects of gravity and surface tension are included. It is shown that in addition to the classical symmetric waves, there are new asymmetric solutions. These new branches of solutions bifurcate from the branches of symmetric waves. The detailed bifurcation diagrams as well as typical wave profiles are presented.

[15]

Kubota

. Weakly-nonlinear, long internal gravity waves in stratified fluids of finite depth
Journal of Hydronautics, 1978,12(4):157-165

DOI URL [本文引用: 1]

[16]

Benjamin, Brooke

. Internal waves of permanent form in fluids of great depth
Journal of Fluid Mechanics, 1967,29(3):559-592

DOI URL [本文引用: 1]

[17]

Davis

, Acrivos

. Solitary internal waves in deep water
Journal of Fluid Mechanics, 2006,29(3):593-607

DOI URL PMID

Internal solitary waves (ISWs) can cause strong vertical and horizontal currents and turbulent mixing in the ocean. These processes affect sediment and pollutant transport, acoustic transmissions and man-made structures in the shallow and deep oceans. Previous studies of the role of ISWs in suspending seafloor sediments and forming marine nepheloid layers were mainly conducted in shallow-water environments. In summer 2017, we observed at least four thick (70-140?m) benthic nepheloid layers (BNLs) at water depths between 956 and 1545?m over continental slopes in the northern South China Sea. We found there was a good correlation between the timing of the ISW packet and variations of the deepwater suspended sediment concentration (SSC). At a depth of 956?m, when the ISW arrived, the near-bottom SSC rapidly increased by two orders of magnitude to 0.62?mg/l at 8?m above the bottom. At two much deeper stations, the ISW-induced horizontal velocity reached 59.6-79.3?cm/s, which was one order of magnitude more than the seafloor contour currents velocity. The SSC, 10?m above the sea floor, rapidly increased to 0.10?mg/l (depth of 1545?m) and 1.25?mg/l (depth of 1252?m). In this study, we found that ISWs could suspend much more sediments on deepwater areas than previously thought. Specifically, we estimated that ISWs could induce and suspend 78.7 Mt/yr of sediment from shelf to deep-sea areas of the northern South China Sea. The total amount of sediment resuspended by shoaling ISWs was 2.7 times that of river-derived sediment reaching the northern South China Sea. This accounted for 6.1% of the global river-discharged sediment (16.4% of that from Asian rivers) transported to the sea.

[18]

Ono

. Algebraic solitary waves in stratified fluids
Journal of the Physical Society of Japan, 1975,39(4):1082-1091

DOI URL [本文引用: 1]

[19]

Matsuno

. A unified theory of nonlinear wave propagation in two-layer fluid systems
Journal of the Physical Society of Japan, 2007,62(6):1902-1916

DOI URL [本文引用: 1]

[20]

Choi

, Camassa

. Weakly nonlinear internal waves in a two-fluid system
Journal of Fluid Mechanics, 1996,313:83-103

DOI URL [本文引用: 1]

[21]

Grue

, Jensen

, Rus$\mathring{a}$s PO. Properties of large-amplitude internal waves
Journal of Fluid Mechanics, 2000,380:257-278

DOI URL [本文引用: 2]

[22]

Koop

, Butler

. An investigation of internal solitary waves in a two-fluid system
Journal of Fluid Mechanics, 1981,112:225-251

DOI URL [本文引用: 2]

[23]

Michallet

, Barth$\acute{e}$lemy E. Experimental study of interfacial solitary waves
Journal of Fluid Mechanics, 1998,366:159-177

DOI URL PMID [本文引用: 3]

We propose consistent scaling of solitary waves on inertia-dominated falling liquid films, which accurately accounts for the driving physical mechanisms and leads to a self-similar characterization of solitary waves. Direct numerical simulations of the entire two-phase system are conducted using a state-of-the-art finite volume framework for interfacial flows in an open domain that was previously validated against experimental film-flow data with excellent agreement. We present a detailed analysis of the wave shape and the dispersion of solitary waves on 34 different water films with Reynolds numbers Re=20-120 and surface tension coefficients σ=0.0512-0.072 N m(-1) on substrates with inclination angles β=19°-90°. Following a detailed analysis of these cases we formulate a consistent characterization of the shape and dispersion of solitary waves, based on a newly proposed scaling derived from the Nusselt flat film solution, that unveils a self-similarity as well as the driving mechanism of solitary waves on gravity-driven liquid films. Our results demonstrate that the shape of solitary waves, i.e., height and asymmetry of the wave, is predominantly influenced by the balance of inertia and surface tension. Furthermore, we find that the dispersion of solitary waves on the inertia-dominated falling liquid films considered in this study is governed by nonlinear effects and only driven by inertia, with surface tension and gravity having a negligible influence.

[24]

Lee

, Beardsley

. The generation of long nonlinear internal waves in a weakly stratified shear flow
Journal of Geophysical Research, 1974,79(3):453-462

DOI URL [本文引用: 1]

[25]

Helfrich

, Melville

. Long nonlinear internal waves
Annual Review of Fluid Mechanics, 2006,38:395-425

DOI URL PMID [本文引用: 1]

Nonlinear internal waves in shallow water have significant acoustic impacts and cause three-dimensional ducting effects, for example, energy trapping in a duct between curved wavefronts that propagates over long distances. A normal mode approach applied to a three-dimensional idealized parametric model [Lin, McMahon, Lynch, and Siegmann, J. Acoust. Soc. Am. 133(1), 37-49 (2013)] determines the dependence of such effects on parameters of the features. Specifically, an extension of mode number conservation leads to convenient analytical formulas for along-duct (angular) acoustic wavenumbers. The radial modes are classified into five types depending on geometric characteristics, resulting in five distinct formulas to obtain wavenumber approximations. Examples of their dependence on wavefront curvature and duct width, along with benchmark comparisons, demonstrate approximation accuracy over a broad range of physical values, even including situations where transitions in mode types occur with parameter changes. Horizontal-mode transmission loss contours found from approximate and numerically exact wavenumbers agree well in structure and location of intensity features. Cross-sectional plots show only small differences between pattern phases and amplitudes of the two calculations. The efficiency and accuracy of acoustic wavenumber and field approximations, in combination with the mode-type classifications, suggest their application to determining parameter sensitivity and also to other feature models.

[26]

Miyata

. Long internal waves of large amplitude
IUTAM Symposinm of Nonlinear Water Waves, Tokyo, Janpan, Aug. 25-28, 1987-1988

DOI URL PMID [本文引用: 1]

The Fourth Universal Definition of Myocardial Infarction defines electrocardiographic (ECG) Q waves as duration ≥30ms and amplitude ≥1mm or QS complex in two contiguous leads. However, current taskforce criteria may be overly restrictive. Therefore, we investigated the association of isolated, lenient, or strict Q waves with long-term outcome.

[27]

Choi

, Camassa

. Fully nonlinear internal waves in a two-fluid system
Journal of Fluid Mechanics, 1999,396:1-36

DOI URL [本文引用: 2]

[28]

, Choi

. Dynamics of strongly nonlinear internal solitary waves in shallow water
Studies in Applied Mathematics, 2010,109(3):205-227

DOI URL [本文引用: 1]

[29]

Ostrovsky Lev

, Grue

. Evolution equations for strongly nonlinear internal waves
Physics of Fluids, 2003,15(10):2934-2948

DOI URL PMID [本文引用: 1]

Using an integrable Gardner equation as an example, a perturbation theory is developed for systems in which limiting-amplitude solitons exist in the form of a pair of distanced kinks. Approximate equations describing multisoliton interactions are derived and further used for modeling the evolution of an arbitrary set of solitons. The results are compared with an exact solution and numerical results. The theory is applied to data from observation of a train of strongly nonlinear internal waves in the ocean.

[30]

Turner

REL

, Vanden-Broeck

. Broadening of interfacial solitary waves
Physics of Fluids, 1988,31(9):2486-2490

DOI URL PMID [本文引用: 1]

Steady solitary and generalized solitary waves of a two-fluid problem where the upper layer is under a flexible elastic sheet are considered as a model for internal waves under an ice-covered ocean. The fluid consists of two layers of constant densities, separated by an interface. The elastic sheet resists bending forces and is mathematically described by a fully nonlinear thin shell model. Fully localized solitary waves are computed via a boundary integral method. Progression along the various branches of solutions shows that barotropic (i.e. surface modes) wave-packet solitary wave branches end with the free surface approaching the interface. On the other hand, the limiting configurations of long baroclinic (i.e. internal) solitary waves are characterized by an infinite broadening in the horizontal direction. Baroclinic wave-packet modes also exist for a large range of amplitudes and generalized solitary waves are computed in a case of a long internal mode in resonance with surface modes. In contrast to the pure gravity case (i.e without an elastic cover), these generalized solitary waves exhibit new Wilton-ripple-like periodic trains in the far field.

[31]

Laget

, Dias

. Numerical computation of capillary-gravity interfacial solitary waves
Journal of Fluid Mechanics, 1997,349:221-251

DOI URL [本文引用: 1]

[32]

Kataoka

. The stability of finite-amplitude interfacial solitary waves
Fluid Dynamics Research, 2006,38(12):831-867

DOI URL [本文引用: 1]

Abstract

The linear stability of finite-amplitude interfacial gravity solitary waves propagating in a two-layer fluid is investigated analytically focusing on the occurrence of an exchange of stability. We make an asymptotic analysis for small growth rates of infinitesimal disturbances, and explicitly obtain their growth rates near an exchange of stability. The result indicates that an exchange of stability occurs at every stationary value of the total energy of the solitary waves. It also gives us information whether the number of growing modes increases or decreases after experiencing the exchange of stability. We apply these analytical results to specific interfacial solitary waves, and find various features on their stability that are not seen in the case of surface solitary waves.

[33]

Vanden-Broeck

J-M

. Numerical calculation of gravity-capillary interfaical waves of finite amplitude
Physics of Fluids, 1980,23:1723-1726

[本文引用: 1]

[34]

Turner

REL

, Vanden-Broeck

J-M

. The limiting configuration of interfacial gravity waves
Physics of Fluids, 1986,29:372-375

[本文引用: 1]

[35]

Dias

, Vanden-Broeck

J-M

. On internal fronts
Journal of Fluid Mechanics, 2003,479:145-154

DOI URL PMID [本文引用: 1]

Epitaxial lateral overgrowth (ELO) over a free-standing dielectric mask is an unexplored territory in selective epitaxy growth (SEG) of semiconductors. By shrinking the dielectric mask dimension to the micron scale, the growth fronts from ELO are able to converge and coalesce, thus providing the freedom to engineer the interfacial structure between the epi-layer and dielectric mask. We demonstrate, herein, anomalous adatom diffusion and migration at the Ge/SiO₂ interface upon SEG on a Si (100) wafer. We find, depending on the oxide strip length, a polyhedral cavity or tunnel can form on the oxide layer. More importantly, we observe a thermally induced substantial internal surface reconfiguration process of Ge atoms that connects two tunnels and one cavity in order to form a single tunnel. Defect-free Ge above the oxide strips is obtained after coalescence. Our findings yield new insight into adatom migration in an enclosed space, and the cavity and tunnel show the first known three-dimensional geometric configuration in selective heteroepitaxial structures.

[36]

Sha

, Vanden-Broeck

. Two-layer flows past a semicircular obstruction
Physics of Fluids, 1993,5:2661-2668

[本文引用: 1]

[37]

Dias

, Vanden-Broeck

. Steady two-layer flows over an obstacle
Philosophical Transactions of the Royal Society A, 2002,360:2137-2154

DOI URL PMID [本文引用: 1]

Nonlinear waves in a forced channel flow of two contiguous homogeneous fluids of different densities are considered. Each fluid layer is of finite depth. The forcing is due to an obstruction lying on the bottom. The study is restricted to steady flows. First a weakly nonlinear analysis is performed. At leading order the problem reduces to a forced Korteweg-de Vries equation, except near a critical value of the ratio of layer depths which leads to the vanishing of the nonlinear term. The weakly nonlinear results obtained by integrating the forced Korteweg-de Vries equation are validated by comparison with numerical results obtained by solving the full governing equations. The numerical method is based on boundary integral equation techniques. Although the problem of two-layer flows over an obstacle is a classical problem, several branches of solutions which have never been computed before are obtained.

[38]

Dias

, Vanden-Broeck

. Two-layer hydraulic falls over an obstacle
European Journal of Mechanics B/Fluids, 2004,23:879-898

DOI URL [本文引用: 1]

[39]

Baker

. Generalized vortex methods for free-surface flows//Waves on Fluid Interfaces, Wisconsin-Madison: Academic Press, 1983: 53-81

[本文引用: 2]

[40]

Hou

, John

, Michael

. Removing the stiffness from interfacial flows with surface tension
Journal of Computational Physics, 1994,114(2):312-338

DOI URL [本文引用: 2]

[41]

Meiron

, Saffman

. Oerhanging interfacial gravity waves of large amplitude
Journal of Fluid Mechanics, 1983,129:213-218

DOI URL PMID [本文引用: 1]

Internal solitary waves are hump-shaped, large-amplitude waves that are physically analogous to surface waves except that they propagate within the fluid, along density steps that typically characterize the layered vertical structure of lakes, oceans and the atmosphere. As do surface waves, internal solitary waves may overturn and break, and the process is thought to provide a globally significant source of turbulent mixing and energy dissipation. Although commonly observed in geophysical fluids, the origins of internal solitary waves remain unclear. Here we report a rarely observed natural case of the birth of internal solitary waves from a frontally forced interfacial gravity current intruding into a two-layer and vertically sheared background environment. The results of the analysis carried out suggest that fronts may represent additional and unexpected sources of internal solitary waves in regions of lakes, oceans and atmospheres that are dynamically similar to the situation examined here in the Saguenay Fjord, Canada.

[42]

Grimshaw

RHJ

, Pullin

. Extreme interfacial waves
Physics of Fluids, 1986,29:2802-2807

[43]

Akers

, Ambrose

, Wright

. Traveling waves from the arclength parameterization: Vortex sheets with surface tension
Interfaces and Free Boundaries, 2013,15:359-380

DOI URL [本文引用: 1]

We study traveling waves for the vortex sheet with surface tension. We use the angle-arclength description of the interface rather than Cartesian coordinates, and we utilize an arclength parameterization as well. In this setting, we make a new formulation of the traveling wave ansatz. For this problem, it should be possible for traveling waves to overturn, and notably, our formulation does allow for waves with multi-valued height. We prove that there exist traveling vortex sheets with surface tension bifurcating from equilibrium. We compute these waves by means of a quasi-Newton iteration in Fourier space; we find continua of traveling waves bifurcating from equilibrium and extending to include overturning waves, for a variety of values of the mean vortex sheet strength.

[44]

Wilkening

. Breakdown of self-similarity at the crests of large amplitude standing water waves
Physical Review Letters, 2011,107:184501

DOI URL PMID [本文引用: 1]

We study the limiting behavior of large-amplitude standing waves on deep water using high-resolution numerical simulations in double and quadruple precision. While periodic traveling waves approach Stokes's sharply crested extreme wave in an asymptotically self-similar manner, we find that standing waves behave differently. Instead of sharpening to a corner or cusp as previously conjectured, the crest tip develops a variety of oscillatory structures. This causes the bifurcation curve that parametrizes these waves to fragment into disjoint branches corresponding to the different oscillation patterns that occur. In many cases, a vertical jet of fluid pushes these structures upward, leading to wave profiles commonly seen in wave tank experiments. Thus, we observe a rich array of dynamic behavior at small length scales in a regime previously thought to be self-similar.

[45]

Ambrose

, Wilkening

. Computation of symmetric, time-periodic solutions of the vortex sheet with surface tension
Proceedings of the National Academy of Sciences of the United States of America, 2010,108(8):3361-3366

DOI URL PMID [本文引用: 1]

A numerical method is introduced for the computation of time-periodic vortex sheets with surface tension separating two immiscible, irrotational, two-dimensional ideal fluids of equal density. The approach is based on minimizing a nonlinear functional of the initial conditions and supposed period that is positive unless the solution is periodic, in which case it is zero. An adjoint-based optimal control technique is used to efficiently compute the gradient of this functional. Special care is required to handle singular integrals in the adjoint formulation. Starting with a solution of the linearized problem about the flat rest state, a family of smooth, symmetric breathers is found that, at quarter-period time intervals, alternately pass through a flat state of maximal kinetic energy, and a rest state in which all the energy is stored as potential energy in the interface. In some cases, the interface overturns before returning to the initial, flat configuration. It is found that the bifurcation diagram describing these solutions contains several disjoint curves separated by near-bifurcation events.

[46]

Wang

, Guan

. Mass transport by fully nonlinear internal waves in two-layer fluids
Preprint, 2019

[本文引用: 1]

[47]

Kodaira

, Waseda

, Miyata

, et al. Internal solitary waves in a two-fluid system with a free surface
Journal of Fluid Mechanics, 2016,804:201-223

DOI URL [本文引用: 3]

[48]

Forgia

G. la

, Sciortino

. The role of the free surface on interfacial solitary waves
Physics of Fluids, 2019,31:106601

DOI URL [本文引用: 2]

[49]

Craig

, Guyenne

, Kalisch

. Hamiltonian long wave expansions for free surfaces and interfaces
Communication on Pure and Applied Mathematics, 2005,58:1587-1641

DOI URL [本文引用: 1]

[50]

Haut

, Ablowitz

. A reformulation and applications of interfacial fluids with a free surface
Journal of Fluid Mechanics, 2009,631:375-396

DOI URL [本文引用: 1]

[51]

Barros

, Gavrilyuk

, Teshukov

. Dispersive nonlinear waves in two-layer flows with free surface. i. Model derivation and general properties
Studies in Applied Mathematics, 2007,119(3):191-211

DOI URL [本文引用: 1]

[52]

Fochesato

, Dias

, Grimshaw

. Generalized solitary waves and fronts in coupled korteweg-de vries systems
Physica D, 2005,210:96-117

DOI URL [本文引用: 1]

[53]

Dias

, Il’ichev

. Interfacial waves with free-surface boundary conditions: An approach via a model equation
Physica D, 2001,150:278-300

DOI URL [本文引用: 1]

[54]

Moni

, King

. Guided and unguided interfacial solitary waves
Quarterly Journal of Mechanics and Applied Mathematics, 1995,48:21-38

DOI URL [本文引用: 1]

[55]

Michallet

, Dias

. Numerical study of generalized interfacial solitary waves
Physics of Fluids, 1999,11(6):1502-1511

DOI URL PMID [本文引用: 1]

P$\breve{a}$r$\breve{a}$u

, Dias

. Interfacial periodic waves of permanent form with free-surface boundary conditions
Journal of Fluid Mechanics, 2001,437:325-336

DOI URL [本文引用: 1]

[57]

Wang

, P$\breve{a}$r$\breve{a}$u

, Milewski

, et al. Numerical study of interfacial solitary waves propagating under an elastic sheet
Proceedings of the Royal Society A, 2014,470(2168):20140111

DOI URL PMID [本文引用: 1]

Rusas

P-O

, Grue

. Solitary waves and conjugate flows in a three-layer fluid
European Journal of Mechanics B/Fluids, 2002,21:185-206

DOI URL [本文引用: 2]

[59]

Fructus

, Grue

. Fully nonlinear solitary waves in a layered stratified fluid
Journal of Fluid Mechanics, 2004,505:323-347

DOI URL [本文引用: 2]

[60]

Vanden-Broeck

, Turner

REL

. Long periodic internal waves
Physics of Fluids, 1992,4:1929-1935

DOI URL PMID [本文引用: 1]

Hypokalemic periodic paralysis (HOKPP) is a rare neuromuscular disorder caused by altered transport of cellular potassium that leads to significant muscle weakness of the extremities. Paralytic attacks are induced by a drop in the serum potassium level and they have been associated with specific triggers. This case describes a 21-year-old male who has had recurrent presentations of acute paralytic attacks following vigorous physical activity. At presentation, this patient exhibited flaccid paralysis of all skeletal muscles below the neck, but was alert and oriented with stable vital signs. The patient was found to have a potassium level of 2.1 mmol/L and an EKG demonstrating U waves (characteristic of hypokalemia). The patient was treated with potassium supplementation with resolution of symptoms. The mainstay of prevention of long term permanent muscle weakness is avoidance of triggers that can lead to hypokalemia. Through education on disease process and lifestyle modifications, we were able to end the cycle of recurrent hospital readmissions and the subsequent financial burden this generated for the patient and his family.

[61]

Sha

, Vanden-Broeck

. Internal solitary waves with stratification in density
Journal of the Australian Mathematical Society Series B, 1997,38:563-580

DOI URL [本文引用: 1]

[62]

Carr

, Davies

, Hoebers

. Experiments on the structure andstability of mode-2 internal solitary-like waves propagating on an offset pycnocline
Physics of Fluids, 2015,27:046602

DOI URL [本文引用: 3]

[63]

Deepwell

, Stastna

, Carr

, et al. Interaction of a mode-2 internal solitary wave with narrow isolated topography
Physics of Fluids, 2017,29:076601

DOI URL [本文引用: 2]

[64]

Carr

, Stastna

, Davies

, et al. Shoaling mode-2 internal solitary-like waves
Journal of Fluid Mechanics, 2019,879:604-632

DOI URL [本文引用: 1]

[65]

Ambrose

. Well-posedness of vortex sheets with surface tension
SIAM Journal of Mathematical Analysis, 2003,35(1):211-244

DOI URL [本文引用: 1]

[66]

Benney

. Long non-linear waves in fluid flows
Journal of Mathematical Physics, 1966,45:52-63

[本文引用: 1]

[67]

Lee

, Beardsley

. The generation of long nonlinear internal waves in a weakly stratified shear flow
Journal of Geophysical Research, 1974,79:453-462

DOI URL [本文引用: 1]

[68]

Dubreil-Jacotin

. Sur la d$\acute{e}$termination rigoureuse des ondes permanentes p$\acute{e}$riodiques d’ampleur finie
[PhD Thesis]. Gauthier-Villars, 1934

[本文引用: 1]

[69]

Long

. Some aspects of the flow of stratified fluds I. A theoretical investigation
Tellus, 1953,5:42-58

DOI URL PMID [本文引用: 1]

Theoretical investigation is performed to explore the novel aspects of nonlinear thermal radiation and non-uniform heat source/sink for chemically reactive flow of ferromagnetic Maxwell liquid over a permeable stretching sheet. Buongiorno model is employed to include Brownian motion and thermophoresis effects. The novelty of the existing study is to account the effect of binary chemical reaction, viscous dissipation, thermal and solutal stratification for ferromagnetic Maxwell fluid. Governing system of nonlinear partial differential equations is transformed into a system of nonlinear ordinary differential equations with the help of apposite similarity transformations. The acquired resulting nonlinear ODEs are solved numerically with the assistance built-in-shooting method (bvp4c). Effects of emanating variables are examined through graphs and tables. It is evident that heat transfer rate enhances with thermal radiation. It is analyzed that temperature upsurges for greater estimations of thermal radiation

(N 1 ?)

, ferromagnetic

(β ? 2)

and thermophoresis

(N ? t)

parameters however it declines for Prandtl number (Pr) and thermal stratified parameter (S?). Space and temperature dependent heat sinks are more appropriate for cooling purposes.

[70]

Stastna

, Lamb

. Large fully nonlinear internal solitary waves: The effect of background current
Physics of Fluids, 2002,14(9):2987-2999

DOI URL [本文引用: 1]

[71]

Mowbray

, Rarity

BSH

. A theoretical and experimental investigation of the phase configuration of internal waves of small amplitude in a density stratified liquid
Journal of Fluid Mechanics, 1967,56(1):1-16

DOI URL [本文引用: 1]

[72]

Lighthill

. Waves in Fluids. Cambridge University Press, 1978

[本文引用: 1]

[73]

Haynes

, Marks

, Mclntyre

, et al. On the “downward control” of extratropical diabatic circulations by eddy-induced mean zonal forces
Journal of the Atmospheric Sciences, 1991,48:651-678

DOI URL [本文引用: 1]

[74]

Fovell

, Durran

, Holton

. Numerical simulations of convectively generated stratospheric gravity waves
Journal of the Atmospheric Sciences, 1992,49(16):1427-1442

DOI URL [本文引用: 3]

[75]

Alexander

, Holton

, Durran

. The gravity wave response above deep convection in a squall line simulation
Journal of the Atmospheric Sciences, 1995,52:2212-2226

DOI URL [本文引用: 1]

[76]

. A high-order numerical scheme for St. Andrew’s cross in stratified fluids
Zhejiang University, 2018

[本文引用: 4]

[77]

Thomas

, Stevenson

. A similarity solution for viscous internal waves
Journal of Fluid Mechanics, 1972,54(3):495-506

DOI URL [本文引用: 1]

[78]

Gordon

, Klement

, Stevenson

. A viscous internal wave in a stratified fluid whose buoyancy frequency varies with altitude
Journal of Fluid Mechanics, 1975,69(3):615-624

DOI URL [本文引用: 1]

[79]

Hurley

, Keady

. The generation of internal waves by vibrating elliptic cylinders. Part 2. Approximate viscous solution
Journal of Fluid Mechanics, 2000,351:119-138

DOI URL [本文引用: 1]

[80]

Sutherland

, Linden

. Internal wave excitation by a vertically oscillating elliptical cylinder
Physics of Fluids, 2002,14(2):721-731

DOI URL [本文引用: 1]

[81]

Tabaei

, Akylas

. Nonlinear internal gravity wave beams
Journal of Fluid Mechanics, 2003,482:141-161

DOI URL PMID [本文引用: 1]

Horizontal ducting of sound between short-wavelength nonlinear internal gravity waves in coastal environments has been reported in many theoretical and experimental studies. Important consequences arising at the open end of an internal wave duct (the termination) are examined in this paper with three-dimensional normal mode theory and parabolic approximation modeling. For an acoustic source located in such a duct and sufficiently far from the termination, some of the propagating sound may exit the duct by penetrating the waves at high grazing angles, but a fair amount of the sound energy is still trapped in the duct and propagates toward the termination. Analysis here shows that the across-duct sound energy distribution at the termination is unique for each acoustic vertical mode, and as a result the sound radiating from the termination of the duct forms horizontal beams that are different for each mode. In addition to narrowband analysis, a broadband simulation is made for water depths of order 80 m and propagation distances of 24 km. Situations occur with one or more modes absent in the radiated field and with mode multipath in the impulse response. These are both consistent with field observations.

[82]

Tabaei

, Akylas

, Lamb

. Nonlinear effects in reflecting and colliding internal wave beams
Journal of Fluid Mechanics, 2005,526:217-243

DOI URL [本文引用: 1]

[83]

Diamessis

, Wunsch

, Delwiche

, et al. Nonlinear generation of harmonics through the interaction of an internal wave beam with a model oceanic pycnocline
Dynamics of Atmospheres and Oceans, 2014,66(2):110-137

DOI URL [本文引用: 1]

[84]

Javam

, Imberger

, Armfield

. Numerical study of internal wave reflection from sloping boundaries
Journal of Fluid Mechanics, 1999,396:183-201

DOI URL [本文引用: 1]

[85]

Javam

, Imberger

, Armfield

. Numerical study of internal wave-wave interactions in a stratified fluid
Journal of Fluid Mechanics, 2000,415:89-116

DOI URL

[86]

Slinn

, Riley

. A model for the simulation of turbulent boundary layers in an incompressible stratified flow
Journal of Computational Physics, 1998,144:550-602

DOI URL [本文引用: 1]

[87]

Liu

. A numerical and analytic study of internal waves in stratified fluids
[PhD Thesis]. University of Manchester, 1989

[本文引用: 1]

[88]

Diamessis

, Domaradzki

, Hesthaven

. A spectral multidomain penalty method model for the simulation of high Reynolds number localized stratified turbulence
Journal of Computational Physics, 2005,202:298-322

DOI URL [本文引用: 1]

[89]

Pal

, Stadler

MBD

, Sarkar

. The spatial evolution of fluctuations in a self-propelled wake compared to a patch of turbulence
Physics of Fluids, 2013,25(9):317-338

[本文引用: 1]

[90]

Stadler

MBD

, Sarkar

. Simulation of a propelled wake with moderate excess momentum in a stratified fluid
Journal of Fluid Mechanics, 2012,692(2):28-52

DOI URL

[91]

Abdilghanie

, Diamessis

. The internal gravity wave field emitted by a stably stratified turbulent wake
Journal of Fluid Mechanics, 2013,720(4):104-139

DOI URL [本文引用: 2]

[92]

Gilreath

, Brandt

. Experiments on the generation of internal waves in a stratified fluid
AIAA Journal, 1983,23(5):693-700

DOI URL [本文引用: 1]

[93]

Hopfinger

, Flor

, Chomaz

, Bonneton

. Internal waves generated by a moving sphere and its wake in a stratified fluid
Experiments in Fluids, 1991,11(4):255-261

DOI URL

[94]

Bonneton

, Chomaz

, Hopfinger

. Internal waves produced by the turbulent wake of a sphere moving horizontally in a stratified fluid
Journal of Fluid Mechanics, 1993,254:23-40

DOI URL

[95]

Meunier

. Stratified wake of a tilted cylinder. Part 2. lee internal waves
Journal of Fluid Mechanics, 2012,699(5):198-215

DOI URL [本文引用: 1]

[96]

Spedding

, Browand

, Bell

, Chen

. Internal waves from intermediate, or late-wake vortices. Stratified Flows I
//Proceedings of the 5th International Symposium on Stratified Flows, 2000, 113-118

[本文引用: 1]

[97]

Spedding

. The evolution of initially turbulent bluff-body wakes at high internal froude number
Journal of Fluid Mechanics, 1997,337:283-301

DOI URL [本文引用: 1]

[98]

Kops

SMDB

, Riley

, Winters

. Dynamics of turbulence strongly influenced by buoyancy
Physics of Fluids, 2003,15(7):2047-2059

DOI URL

[99]

Diamessis

, Spedding

, Domaradzki

. Similarity scaling and vorticity structure in high-Reynolds-number stably stratified turbulent wakes
Journal of Fluid Mechanics, 2011,671(3):52-95

DOI URL [本文引用: 1]

[100]

Taylor

, Sarkar

. Internal gravity waves generated by a turbulent bottom ekman layer
Journal of Fluid Mechanics, 2007,590:331-354

DOI URL [本文引用: 1]

[101]

Linden

. Internal wave excitation from stratified flow over a thin barrier
Journal of Fluid Mechanics, 1998,377:223-252

DOI URL [本文引用: 1]

[102]

Donato

, Peregrine

, Stocker

. The focusing of surface waves by internal waves
Journal of Fluid Mechanics, 1999,384:27-58

DOI URL PMID [本文引用: 1]

Acoustic fields have been widely used for manipulation of particles and cells within microfluidic systems. In this Letter, we explore a novel acoustofluidic phenomenon for particle patterning and focusing, where a periodic acoustic pressure field is produced parallel to internal channel boundaries with the imposition of either a traveling or standing surface acoustic wave (SAW). This effect results from the propagation and intersection of edge waves from the channel walls according to the Huygens-Fresnel principle and classical wave fronts from the substrate-fluid interface. We demonstrate versatile control over this effect to produce both one- and two-dimensional acoustic patterning from one-dimensional SAW fields and its utility for continuous particle focusing. Uniquely, this channel-guided acoustic focusing permits the generation of robust acoustic fields without channel resonance conditions and particle focusing positions that are difficult or impossible to produce otherwise.

[103]

Yuan

, Grimshaw

, Johnson

. The evolution of second mode internal solitary waves over variable topography
Journal of Fluid Mechanics, 2018,836:238-259

DOI URL [本文引用: 1]

[104]

Yuan

, Grimshaw

, Johnson

, et al. Topographic effect on oblique internal wave-wave interactions
Journal of Fluid Mechanics, 2018,856:36-60

DOI URL [本文引用: 1]

[105]

Yuan

, Grimshaw

, Johnson

, et al. The propagation of internal solitary waves over variable topography in a horizontally two-dimensional framework
Journal of Physical Oceanography, 2018,48:283-300

DOI URL [本文引用: 1]

[106]

Tunaley

JKE

. Simulation of internal wave wakes and comparison with observations
ESA Living Planet Symposium, 2013

[本文引用: 2]