鲍志勇
, 金彪


中国科学院大学物理科学学院, 北京 100090
摘要: 用Matsubara格林函数方法研究
s波超导/铁磁/
p波超导结构的约瑟夫森效应.研究中考虑了
p波超导中的3种配对形式.发现约瑟夫森电流的最低阶项是cos
φ,讨论这个反常项(cos
φ)随铁磁强度和铁磁宽度的变化.在这个系统中发现0-π转变,考虑了该系统在自旋电子学中的应用.
关键词: 约瑟夫森电流
p波超导低阶反常项
Superconductor/ferromagnet(SC/FM) hybrid structures have been studied for long. In these systems, the competition between the ferromagnetism and superconductivity plays a crucial role. A wide range of phenomena, such as the 0-π transition
[1], the odd-frequency pairing correlation
[2], and the coexistence of ferromagnetism and superconductivity in one material
[3-4], have been found. There have
been numerous studies on SC/FM/SC Josephson junctions, e.g., the spin-singlet SC/FM/spin-singlet SC junctions
[5-6] and the spin-triplet-SC/FM/spin-triplet-SC junctions
[7-9]. The Josephson effects in these junctions with unconventional superconductors are strongly influenced by the Andreev bound states
[10] since they dominate the tunneling behavior at low temperature
[11, 12].
In the spin-singlet-SC/spin-triplet-SC Josephsonjunctions
[13-15], the lowest usual harmonic term (
∝sin
φ) in the current-phase relation, which originates from tunneling processes involving only a single Cooper pair, vanishes due to the opposite parity of the pairing states. In these junctions, the leading contribution to the junction current comes from the coherent tunneling of even numbers Cooper pairs, and therefore the sin
2φ term is the leading harmonic
[16-19].
However, in the Josephson junctions with ferromagnet inter layer, the spin singlet-triplet conversion will occur.Thus the lowest harmonic terms are expected
[20]. The spin-triplet-SC/FM/spin-triple-SC junctions have been investigated extensively in the last decade
[21]. It is found that the 0-π transition can be produced by tuning the orientation, changing the magnitude of magnetic moment, or varying the width of the ferromagnet layer. The spin-singlet-SC/FM/spin-triplet-SC junctions have been studied by several group
[13, 22]. Recently, Brydon etal.
[23]have discussed the conditions under which the single-Cooper-pair tunneling current
∝cos
φ is possible. However, theoretical study on the 0-π transition in the spin-singlet-SC/FM/spin-triplet-SC junction is still lacking. In this work we will investigate how this single-Cooper-pair tunneling current is dependent on the strength of magnetization and the width of the central ferromagnet layer, an issue closely related to the investigation of 0-π transition in the spin-singlet-SC/FM/spin-triplet-SC junction. The Josephson current is calculated by using the Matsubara Green function formalism by Furusaki and Tsukada
[24-26].
1 Model and formalismLet us consider a quasi two-dimensional double tunneling Josephson junction consisting of a clean ferromagnet layer with width L sandwiched between an
s-wave SC (left) and a
p-wave SC (right) as shown schematically in
Fig. 1.
Fig. 1
We adopt the Stoner model for central ferromagnet where the direction of magnetization (M)is specified by polar angle
θ and azimuthal angle
φ,
| $M=M(sin\theta cos\varphi , sin\theta sin\varphi , cos\theta ).$ | (1) |
The tunneling barriers located perpendicular to the
x-axis are modeled by delta-type potentials
V(x)=V1δ(x)+V2δ(x-L). The junction is described by the Bogoliubove-de Gennes (BdG) equation
[27].The BdG equations in momentum space for quasiparticle states with energy
E can be written as
| $\left( \begin{matrix} {{\widehat{h}}_{F}}\left( k \right) & 0 \\ 0 & -{{{\hat{h}}}^{T}}{{\left( -k \right)}_{F}} \\\end{matrix} \right)\text{ }{{\Psi }_{F}}\left( k \right)=E{{\Psi }_{F}}(k)$ | (2) |
for ferromagnet, where
${{\widehat{h}}_{F}}\left( k \right)=(-\frac{{{h}^{2}}~{{\nabla }^{2}}}{2m}-\mu ){{{\hat{\sigma }}}_{0}}-M\cdot \hat{\sigma }$, and
| $\left( \begin{matrix} \widehat{h}\left( k \right) & \hat{\Delta }\left( k \right) \\ \hat{\Delta }{{\left( k \right)}^{\dagger }} & -{{{\hat{h}}}^{T}}\left( -k \right) \\\end{matrix} \right)\text{ }~{{\Psi }_{s, p}}\left( k \right)=E~{{\Psi }_{s, p}}(k)$ | (3) |
for superconductors, where
$\widehat{h}\left( k \right)=(-\frac{{{h}^{2}}~{{\nabla }^{2}}}{2m}-\mu ){{{\hat{\sigma }}}_{0}}$ and
| $\hat{\Delta }\left( k \right)=~\left\{ \begin{matrix} i{{\Delta }_{s}}{{\sigma }_{2}}{{e}^{i}}{{\varphi }_{s}}, & s-wave, \\ i{{\Delta }_{p}}d\left( k \right)\cdot \hat{\sigma }{{{\hat{\sigma }}}_{2}}{{e}^{i}}{{\varphi }_{p}}, & p-wave. \\\end{matrix} \right.$ | (4) |
In the above eguations
$\hat{\sigma }=({{{\hat{\sigma }}}_{1, }}{{{\hat{\sigma }}}_{2, }}{{{\hat{\sigma }}}_{2}})$ denotes the Pauli vector matrix,
${{{\hat{\sigma }}}_{0}}$ is the unit matrix, d(k) is the d vector of
p-wave SC, and
φs and
φp indicate the overall phase of the
s-wave and
p-wave order parameters, respectively. The temperature dependence of the gap amplitude
Δs, p is determined by the BCS-like gap equation. For the sake of simplicity, the effective masses and chemical potentials in all the three regions of the junction are assumed to be equal.
In this work we will confine ourselves to a case where the direction of the d vector is independent of wavevector k, namely d(k)=
f(k)
${\hat{z}}$, and consider the following three orbital pairing states
| $f\left( k \right)=\left\{ \begin{matrix} {{k}_{x}}/{{k}_{F}}, \\ {{k}_{y}}/{{k}_{F}}, \\ \left( {{k}_{x}}+i{{k}_{y}} \right)/{{k}_{F}}, \\\end{matrix} \right.\begin{matrix} {{p}_{x}}-wave, \\ {{p}_{y}}-wave, \\ \text{ }\left( {{p}_{x}}+i{{p}_{y}} \right)-wave. \\\end{matrix}$ | (5) |
It is noted that the condition for the formation of zero-energy Andreev bound state(ZABS)
[19] at the interface given by
| $f\left( {{k}_{x}},{{k}_{y}} \right)f\left( -{{k}_{x}},{{k}_{y}} \right)<0$ | (6) |
is satisfied for
(px+ipy)-wave and
px-wave states. In addition, for both the
s-wave-SC/FM/
(px+ipy)-wave-SC (
s/FM/(px+ipy)) junction and the
s/FM/px junctions the orbital pairing states of the superconductors have the same parity with respect to the interface momentum, and therefore a Josephson current with distinct phase relation
J∝cos
φ (φ=φs-φp) may appear especially when M‖d
[23].
The wave function of our
s/FM/p Josephson junction can be obtained by solving the BdG equation. We have
| $\begin{align} & \Psi \left( r \right)={{e}^{i{{k}_{y}}y}}[{{\Psi }_{s}}\left( x \right)\Theta \left( -x \right)+{{\Psi }_{F}}\left( x \right)\Theta \left( x \right)\Theta \left( L-x \right)+ \\ & {{\Psi }_{p}}\left( x \right)\Theta \left( x-L \right)], \\ \end{align}$ | (7) |
with
| $\begin{align} & {{\Psi }_{s}}\left( x \right)=\left( \begin{matrix} {{{\hat{u}}}^{e}}_{+} \\ {{{\hat{v}}}^{e}}_{+} \\\end{matrix} \right){{e}^{i}}{{k}_{x}}x\hat{\alpha }+\left( \begin{matrix} {{{\hat{u}}}_{-}}^{h} \\ {{{\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{v}}}_{-}}^{h} \\\end{matrix} \right){{e}^{-}}i{{k}_{x}}x\hat{\beta }+ \\ & {{\left( \begin{matrix} {{{\hat{u}}}^{e}}_{\text{-}} \\ {{{\hat{v}}}^{e}}_{\text{-}} \\\end{matrix} \right)}^{s}}{{e}^{^{-}i{{k}_{x}}x}}\hat{A}+{{\left( \begin{matrix} {{{\hat{u}}}_{+}}^{h} \\ {{{\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{v}}}_{+}}^{h} \\\end{matrix} \right)}^{s}}{{e}^{i{{k}_{x}}x}}\hat{B}, \\ & {{\Psi }_{p}}\left( x \right)={{\left( \begin{matrix} {{{\hat{u}}}^{e}}_{+} \\ {{{\hat{v}}}^{e}}_{+} \\\end{matrix} \right)}^{p}}{{e}^{i}}{{p}_{x}}x\hat{C}+{{\left( \begin{matrix} {{{\hat{u}}}_{-}}^{h} \\ {{{\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{v}}}_{-}}^{h} \\\end{matrix} \right)}^{p}}{{e}^{-}}i{{p}_{x}}x\hat{D}, \\ & {{\Psi }_{F}}=\left( \begin{matrix} n\cdot \hat{\sigma } \\ 0 \\\end{matrix} \right){{e}^{i{{{\hat{q}}}_{\sigma x}}x}}{{{\hat{e}}}_{l}}+\left( \begin{matrix} 0 \\ n\cdot {{{\hat{\sigma }}}^{*}} \\\end{matrix} \right){{e}^{i{{{\hat{q}}}_{\sigma x}}x}}{{{\hat{f}}}_{l}}+ \\ & \left( \begin{matrix} n\cdot \hat{\sigma } \\ 0 \\\end{matrix} \right){{e}^{-i{{{\hat{q}}}_{\sigma x}}x}}{{{\hat{g}}}_{l}}+\left( \begin{matrix} 0 \\ n\cdot {{{\hat{\sigma }}}^{*}} \\\end{matrix} \right){{e}^{i{{{\hat{q}}}_{\sigma x}}x}}+{{{\hat{h}}}_{l}}, \\ \end{align}$ | (8) |
where
$n=(sin\frac{\theta }{2}cos\varphi ,sin\frac{\theta }{2}sin\varphi ,cos\frac{\theta }{2})$,
| $\psi _{\pm }^{e}=\left( \begin{matrix} \hat{u}_{\pm }^{e} \\ \hat{v}_{\pm }^{e} \\\end{matrix} \right)=\left( \begin{matrix} {{u}_{\pm }}{{{\hat{\sigma }}}_{0}} \\ {{v}_{\pm }}\frac{\hat{\Delta }_{\pm }^{\dagger }}{\left| \hat{\Delta }_{\pm }^{\dagger } \right|} \\\end{matrix} \right),$ | (9) |
| $\psi _{\pm }^{h}=\left( \begin{matrix} \hat{u}_{\pm }^{h} \\ \hat{v}_{\pm }^{h} \\\end{matrix} \right)=\left( \begin{matrix} {{v}_{\pm }}\frac{{{{\hat{\Delta }}}_{\pm }}}{\left| {{{\hat{\Delta }}}_{\pm }} \right|} \\ {{u}_{\pm }}{{{\hat{\sigma }}}_{0}} \\\end{matrix} \right),$ | (10) |
| ${{u}_{\pm }}=\sqrt{\frac{1}{2}\left\{ 1+\frac{\Omega \pm }{{{\omega }_{n}}} \right\}},{{v}_{\pm }}=\sqrt{\frac{1}{2}\left\{ 1-\frac{\Omega \pm }{{{\omega }_{n}}} \right\}},$ | (11) |
with
| $\begin{align} & {{\Omega }_{\pm }}=\sqrt{{{\omega }_{n}}^{2}+{{\left| {{{\hat{\Delta }}}_{\pm }}\left( k \right) \right|}^{2}}}, \\ & {{\omega }_{n}}=(2n+1)\pi {{k}_{B}}T, \\ & {{{\hat{\Delta }}}_{\pm }}\left( k \right)=\hat{\Delta }\left( \pm {{k}_{x}},{{k}_{y}} \right), \\ & {{{\hat{q}}}_{\sigma }}=\left( \begin{matrix} {{q}_{1}} & 0 \\ 0 & {{q}_{2}} \\\end{matrix} \right). \\ \end{align}$ | (12) |
In the above expressions, the
x-components of wavevectors for
s-wave SC,
p-wave SC, and FM are respectively given by
| $\begin{align} & {{k}_{x}}\approx {{k}_{F}}cos{{\theta }_{k}}, \\ & {{p}_{x}}\approx {{k}_{F}}cos{{\theta }_{s}}, \\ & {{q}_{1,2x}}\approx {{k}_{F}}\sqrt{1-\left( + \right)\eta }cos{{\theta }_{1,2}} \\ \end{align}$ | (13) |
with
η=2m|M|/${\rlap{--} h}$2kF2. Due to the translational invariance along the
y-axis, the
y-component of wavevector is conserved during scattering, which yields
| $sin{{\theta }_{k}}=\sqrt{1-\left( + \right)\eta }sin{{\theta }_{1,2}}=sin{{\theta }_{s}}.$ | (14) |
Therefore for injection angles
| ${{\theta }_{k}}>{{\theta }_{c}}=arccos\eta $ | (15) |
q1x is imaginary, and the wave for minority-spin quasiparticle decays exponentially into the ferromagnet region. There are two columns in our wave function and they represent the spin-up channel and spin-down channel, respectively. The coeffi-cients of incoming, reflecting, and outgoing waves
$\hat{\alpha },\hat{\beta },\hat{A},\hat{B},\hat{C},\hat{A},{{{\hat{e}}}_{l}},{{{\hat{f}}}_{l}},{{{\hat{g}}}_{l}}and{{{\hat{h}}}_{l}}$ are all 2×2 matrices. These matrices should be determined by the boundary conditions:
| $\begin{align} & \psi \left( x={{0}^{+}} \right)=\psi \left( x={{0}^{-}} \right), \\ & \psi \left( x={{L}^{+}} \right)=\psi \left( x={{L}^{-}} \right), \\ & \frac{d}{dx}\psi \left( x \right){{|}_{x=0+}}-\frac{d}{dx}\psi \left( x \right){{|}_{x=0-}}=2m{{V}_{1}}\psi \left( x=0 \right), \\ & \frac{d}{dx}\psi \left( x \right){{|}_{L=0+}}-\frac{d}{dx}\psi \left( x \right){{|}_{L=0-}}=2m{{V}_{2}}\psi \left( x=L \right). \\ \end{align}$ | (16) |
The Andreev reflection coefficients are defined by the off-diagonal elements of the matrix relation
[28]| $\left( \begin{matrix} {\hat{A}} \\ {\hat{B}} \\\end{matrix} \right)=\left( \begin{matrix} {{{\hat{r}}}_{ee}} & {{{\hat{r}}}_{eh}} \\ {{{\hat{r}}}_{he}} & {{{\hat{r}}}_{hh}} \\\end{matrix} \right)\left( \begin{matrix} {\hat{\alpha }} \\ {\hat{\beta }} \\\end{matrix} \right).$ | (17) |
According to Green function method by Furusaki and Tsukada, the Josephson current can be expressed in terms of the Andreev coefficients
| $J\left( \varphi \right)=\frac{e}{2h}\sum\limits_{{{\omega }_{n}}}{T\sum\limits_{{{k}_{y}}}{\frac{1}{2{{\Omega }_{s}}}Tr[{{{\hat{\Delta }}}_{s}}{{{\hat{r}}}_{he}}-\hat{\Delta }_{s}^{\dagger }{{{\hat{r}}}_{eh}}]}}.$ | (18) |
When
θ=0, the Andreev reflection coefficients can be calculated analytically, and we have
| ${{\hat r}_{eh}} = {e^{i{\varphi _s}}}\left( {\begin{array}{*{20}{c}} 0&{\frac{{{\xi _ - }}}{{{\Xi _ - }}}} \\ {\frac{{{\xi _ + }}}{{{\Xi _ + }}}}&0 \end{array}} \right),$ | (19) |
| ${{\hat r}_{eh}} = {e^{^ - i{\varphi _s}}}\left( {\begin{array}{*{20}{c}} 0&{\frac{{{{\tilde \xi }_ + }}}{{{\Xi _ + }}}} \\ {\frac{{{\xi _\_}}}{{{\Xi _\_}}}}&0 \end{array}} \right),$ | (20) |
where
| $\begin{gathered} {\Xi _ \pm } = 2i\tilde k{g_2}\left( {{u_p}^2{u_s}^2 - {{\tilde v}_p}^2{v_s}^2} \right) + 8i{{\tilde k}^2}{{\tilde q}_1}{{\tilde q}_2}{u_s}{v_s}{u_p}{{\tilde v}_p}sin\varphi \pm \hfill \\ [{\zeta _1}{\zeta _2}\left( {{u_p}^2 + {{\tilde v}_p}^2} \right)\left( {{u_s}^2 - {v_s}^2} \right) + 2{g_1}{{\tilde k}^2}\left( {{u_p}^2{u_s}^2 + {v_p}^2{v_s}^2} \right)] \hfill \\ {\xi _ \pm } = 2{g_1}{{\tilde k}^2}{u_s}{v_s}({u_p}^2 - {{\tilde v}_p}^2) \pm \hfill \\ [2i{g_2}\tilde k{u_s}{v_s}\left( {{u_p}^2 + {{\tilde v}_p}^2} \right) - 4{{\tilde k}^2}{{\tilde q}_1}{{\tilde q}_2}{u_p}{{\tilde v}_p}f], \hfill \\ {{\tilde \xi }_ \pm } = 2{g_1}{{\tilde k}^2}{u_s}{v_s}\left( {{u_p}^2 - {{\tilde v}_p}^2} \right) \pm \hfill \\ [2i{g_2}\tilde k{u_s}{v_s}\left( {{u_p}^2 + {{\tilde v}_p}^2} \right) - 4{{\tilde k}^2}{{\tilde q}_1}{{\tilde q}_2}{u_s}{v_s}{u_p}{{\tilde v}_p}{f^*}], \hfill \\ \end{gathered} $ | (21) |
with
| $\begin{align} & {{g}_{1}}=2{{{\tilde{q}}}_{1}}{{{\tilde{q}}}_{2}}cos({{q}_{1}}L)cos\left( {{q}_{2}}L \right)+ \\ & ({{{\tilde{q}}}_{1}}^{2}+{{{\tilde{q}}}_{2}}^{2})sin({{q}_{1}}L)sin\left( {{q}_{2}}L \right), \\ & {{g}_{2}}=\left( {{Z}^{2}}+{{{\tilde{k}}}^{2}} \right){{\chi }_{1}}+{{{\tilde{q}}}_{1}}{{{\tilde{q}}}_{2}}{{\chi }_{2}}+ \\ & Z({{{\tilde{q}}}_{1}}^{2}-{{{\tilde{q}}}_{2}}^{2})sin({{q}_{1}}L)sin\left( {{q}_{2}}L \right), \\ & {{\chi }_{1}}={{{\tilde{q}}}_{2}}sin({{q}_{2}}L)cos\left( {{q}_{1}}L \right)-{{{\tilde{q}}}_{1}}sin({{q}_{1}}L)cos\left( {{q}_{2}}L \right), \\ & {{\chi }_{2}}={{{\tilde{q}}}_{1}}sin({{q}_{2}}L)cos\left( {{q}_{1}}L \right)-{{{\tilde{q}}}_{2}}sin({{q}_{1}}L)cos\left( {{q}_{2}}L \right), \\ & f={{e}^{-i\varphi }}{{u}_{s}}^{2}-{{e}^{i\varphi }}{{v}_{s}}^{2},{{{\tilde{v}}}_{p}}={{v}_{p}}f\left( k \right), \\ & {{\zeta }_{\sigma }}=({{Z}^{2}}+{{{\tilde{k}}}^{2}}-{{{\tilde{q}}}^{2}}_{\sigma })sin\left( {{q}_{\sigma }}L \right)+2{{{\tilde{q}}}_{\sigma }}Zcos\left( {{q}_{\sigma }}L \right), \\ & {{{\tilde{q}}}_{\sigma }}={{q}_{\sigma }}/{{{\tilde{k}}}_{F}},k=k/{{k}_{F}},Z=2mV/{{k}_{F}}. \\ \end{align}$ | (22) |
The current
J(φ) can in general be decomposed into a Fourier series,
| $I\left( \varphi \right)=\sum\limits_{n\ge 1}{[{{I}_{n}}sin\left( n\varphi \right)+{{J}_{n}}cos\left( n\varphi \right)]},$ | (23) |
where
In and
Jn are coefficient to be determined.The components with index
n correspond to the amplitudes of the
n th reflection processes of quasiparticles. The
Jn vanishs if the time-reversal symmetry is not broken. In the following we consider a symmetric junction (
V1=V2=V), and further assume
Δs=Δp for simplicity.
It is well-known
[29]that, in the spin space where the matrix in Eq. (2) is diagonal, the gap matrix in Eq. (3) for
p-wave becomes
| ${{{\hat{\Delta }}}^{F}}_{p}\left( k \right)=\left( \begin{matrix} sin\theta & cos\theta \\ cos\theta & -sin\theta \\\end{matrix} \right){{\Delta }_{p}}f(k){{e}^{i{{\varphi }_{p}}}},$ | (24) |
with
${{{\hat{\Delta }}}^{F}}_{s}\left( k \right)$ for
s-wave remaining unchanged. Consequently the Josephson current depends on the orientation of the magnetization only through the polar angle
θ. As can be seen, the spin state of the
p-wave SC depends strikingly on
θ. When
θ=0 the gap matrix for
p-wave is off-diagonal, and the SC is in an opposite-spin-pairing state (the same spin state as the
s-wave SC). When
${{{\hat{\Delta }}}^{F}}_{p}$ is diagonal and the SC is in an equal-spin-pairing state. For
0<θ<π, the SC is a superposition of these two spin states.
The Josephson current is normalized by the normal conductance of the junction at the limit
η=0,
J0=eπ
Δ0P/${\rlap{--} h}$, where the transmission probability
P of junction is calculated as
| $P=\frac{1}{2}\sum\limits_{{{k}_{y}}}{\sum\limits_{\sigma =1,2}{\frac{4{{{\tilde{k}}}^{2}}{{{\tilde{q}}}^{2}}_{\sigma }}{{{\zeta }_{\sigma }}^{2}+4{{{\tilde{k}}}^{2}}{{{\tilde{q}}}^{2}}_{\sigma }}}}.$ | (25) |
2 Results and discussionsIn this section we present results for the Josephson current in an
s/
FM/
p junction. For the
p-wave SC we choose the three different superconducting states listed in Eq. (5). We first discuss the dependence of current-phase relation on the polar angle
θ. The parameters are fixed at
T=0.01Tc,
η=0.4,
Z=5.0, and
kFL=3.5. In
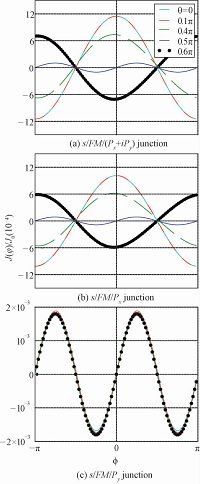
Fig. 2(a) we plot the Josephson current as a function of
φ for the
s/FM/(px+ipy) junction with
θ=0, 0.1π, 0.4π, 0.5π, and 0.6π. It is noted first of all that at
φ=±π
/2 the Josephson current vanishes in all the cases, implying the absence of the lowest usual sinusoidal term
∝sin
φ. At
θ=0 the current is dominated by the lowest unusual harmonic contribution
∝cos
φ.
J∝sin
2φ at
θ=π
/2. In addition, detailed calculations show that
J satisfies the relation
J(φ, θ)=-J(-φ, π
-θ). Therefore we can predict that the dominant term in the Josephson current for
s/
FM/
(px+ipy) junction is
| $J\left( \varphi \right)={{{\tilde{J}}}_{1}}cos\theta cos\varphi +{{I}_{2}}sin\left( 2\varphi \right).$ | (26) |
Note that the contribution from the first term above vanishes identically when
η=0.
Fig. 2
In
Fig. 2(b) we show the results for
s/FM/px junction.The results for this junction are very similar to those for the
s/
FM/
(px+ipy) junction. Therefore the results can also be summarized by Eq. (26). We now analyge the results for
s/FM/py junction, which are shown in
Fig. 2(c).The term proportional to sin
φ is absent in this case too. In contrast to the
(px+ipy) and
px cases the dominant contribution to Josephson current is
∝sin
2φ. It is also noted that the current shows very weak dependence upon the angle
θ and its amplitude is significantly reduced.
The results in
Fig. 2 clearly indicate that the current-phase relation in a
s/FM/p Josephson junction depends strongly on the detailed properties of
p-wave Cooper pair wave function including both the spin and orbital parts. In particular, the unusual
∝cos
φ term may appear when the spin is in the opposite-spin-pairing state, and simultaneously the orbital function
f(k) has the same parity with respect to
kx. At
θ=π
/2, the dominant contribution to Josephson current is
∝sin
2φ for our three
p-wave states. However, the amplitudes of the Josephson current in the
px+ipy and
px cases are largely enhanced in comparison to that in the
py case (see
Fig. 2), indicating the importance of resonant tunneling through the ZABS at low temperature (the low-temperature anomaly). These results are consistent with those obtained by Brydon et al.
[23]in the calculation of a two-dimensional microscopic lattice model.We have discussed the current-phase relation for fixed values of
η and
kFL, where it is found in particular that single-Cooper-pair tunneling current (
∝cos
φ) is possible when certain conditions are fulfilled, e.g., M·d≠0. Next we will consider the
s/FM/(px+ipy) junction and focus our attention to how the contribution from the cos
φ term is affected by the magnitude of magnetization M.We explicitly calculate the
η dependence of the coefficient
J1 in Eq. (26). The coefficient
J1 is approximately egual to the value of the total Josephson current at
φ=0 and
θ=0 (
${{{\tilde{J}}}_{1}}$≈J(φ=0, θ=0)).The dominant correction to this approximation stems from the
J3 term since the cos
2φ term is absent for our junction.
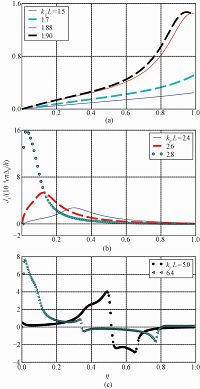
Plotted in
Fig. 3 are the
η dependence of
${{{\tilde{J}}}_{1}}$ for several values of
kFL at
T=0.01Tc and
Z=5.0. When
kFL is small,
${{{\tilde{J}}}_{1}}$ shows a linear dependence in all cases. Remarkably, this linear dependence persists even when the magnetization reaches the half metallic limit
η→1 for
kFL<1.5, and
${{{\tilde{J}}}_{1}}$ increases monotonically up to
η≈0.9 for
kFL<1.9 (see
Fig. 3(a)). For lager values of
kFL,
${{{\tilde{J}}}_{1}}$ exhibits distinct one-peak structure. The position of the peak shifts leftward and the peak value increases drastically with
kFL for
1.9<η≤3 (see
Fig. 3(b)). It is also noted that, in contrast to the cases in
Fig. 3(a),
${{{\tilde{J}}}_{1}}$ is suppressed more seriously when
kFL is lager in this region. Depicted in
Fig. 3 (c) are the results for
kFL=5.0, 6.4. The striking feature we can see here is that,
${{{\tilde{J}}}_{1}}$ changes its sign at some points. In the case of
kFL=5.0, the sign of
${{{\tilde{J}}}_{1}}$ is inverted at
η≈0.52. We predict that a 0-π transition takes place around this point.
Fig. 3
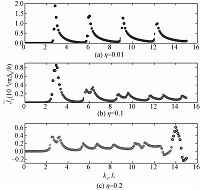
In our junction the quantum interference effect can arise due to the different wavevectors of quasiparticles within the ferromagnet, leading to an oscillatory variation of
${{{\tilde{J}}}_{1}}$ as a function of the thickness
kFL. We show in
Fig. 4 the
kFL dependence of
${{{\tilde{J}}}_{1}}$ for
η=0.01, 0.1, and 0.2 at
T=0.01Tc and
Z=5.0. One can see that, the increment in
η tends to depress the amplitude and complicats the structure of oscillation. Finally, we discuss a possible spintronic application of our
s/FM/(px+ipy) junction. Note that the chiral
(px+ipy)-wave state is currently considered to be possible candidate of pairing state of Sr
2RuO
4[30]. As discussed above, due to the interplay between ferromagnetism and superconductivity, the Josephson current in
s/FM/(px+ipy) junction depends sensitively on
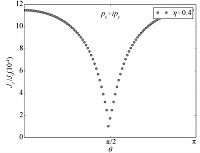
θ. Shown in
Fig. 5 is the variation of the critical Josephson current
Jc with the angle
θ for fixed parameters
η=0.4,
Z=5.0,
kFL=3.5, and
T=0.01Tc.The critical Josephson current is defined as
Jc≡max
(|J(φ)|).
Jc reaches its maximum at
θ=0 while its minimum at
θ=π/2. The ratio
(Jc(θ=0)-Jc(θ=0))/Jc(θ=0) may become very large for reasonably chosen system parameters. Therefore it may be possible to obtain a huge TMR-like effect by tuning the direction of the magnetic moment using weak external magnetic field.
Fig. 4
Fig. 5
3 conclusionsIn this work, we have studied the Josephson effect in
s/FM/p junction based on the BdG equation using the Matsubara Green function formalized by Furusaki and Tsukada. Three typical
p-wave pairing states are considered for the sake of comparison. Our results concerning the contribution to Josephson current from the lowest harmonic term (
∝cos
φ) are consistent with the previous studies using the lattice model. We have further calculated the dependence of the cos
φ term on the strength of magnetic moment as well as on the width of the ferromagnet layer. It is found, in particular, that the 0-π transition may occur in
s/FM/(px+i
py) and
s/FM/px junctions in certain range of system parameters. In addition, a possible spintronic application scheme has been discussed. Further systematic investigations about the dependence of the 0-π transition on parameters
θ,
η,
kFL and
Z and temperature
T would be interesting.
References| [1] | Ryazanov V V, Oboznov V A, Rusanov A Yu, et al. Coupling of two superconductors through a ferromagnet: evidence for a π junction[J].Physical review letter, 2001, 86:2427.DOI:10.1103/PhysRevLett.86.2427 |
| [2] | Begeret F S, Volkov A F, Efetov K B. Odd triplet superconductivity and related phenomena in superconductor-ferromagnet structures[J].Reviews of Modern Physics, 2005, 77:1321.DOI:10.1103/RevModPhys.77.1321 |
| [3] | Saxena S S, Agarwal P, Ahilan1K, et al. Superconductivity on the border of itinerant-electron ferromagnetism in UGe2[J].Nature, 2000, 406:587.DOI:10.1038/35020500 |
| [4] | Aoki D, Huxley A D, Ressouche E, et al. Coexistence of superconductivity and ferromagnetism in URhGe[J].Nature, 2001, 413:613.DOI:10.1038/35098048 |
| [5] | Buzdin A I, Bulaevskii L N, Panyukov S V. Critical-currenots cillations as a function of the exchange field and thickness of the ferromagnetic metal(F) in an S-F-S Josephson Junction[J].JETP Lett, 1982, 35:178. |
| [6] | Radovic Z, Lazarides N, Flytzanis N. Josephson effect in double-barrier superconductor-ferromagnet junctions[J].Physical Review B, 2003, 68:014501. |
| [7] | Kastening B, Morr D K, Manske D, et al. Novel Josephson Effect in Triplet-Superconductor-Ferromagnet-Triplet-Superconductor Junctions[J].Physical review letter, 2006, 96:047009.DOI:10.1103/PhysRevLett.96.047009 |
| [8] | Linder J, Gr?nsleth M S, Sudb? A. Tunneling currents in ferromagnetic systems with multiple broken symmetries[J].Physical Review B, 2007, 75:024508.DOI:10.1103/PhysRevB.75.024508 |
| [9] | Brydon P M R, Kastening B, Morr D K, et al. Interplay of ferromagnetism and triplet superconductivity in a Josephson junction[J].Physical Review B, 2008, 77:104504.DOI:10.1103/PhysRevB.77.104504 |
| [10] | Andreev A F. Thermal conductivity of the intermediate state of superconductors[J].Sov Phys JETP, 1964, 19:1228. |
| [11] | Tanaka Y, Kashiwaya S. Theory of the Josephson effect in d-wave superconductors[J].Physical Review B, 1996, 53:11957.DOI:10.1103/PhysRevB.53.R11957 |
| [12] | Barash Y, Burkhardt H, Rainer D. Low-temperature anomaly in the josephson critical current of junctions in d-wave superconductors[J].Physical Review Letter, 1996, 77:4070.DOI:10.1103/PhysRevLett.77.4070 |
| [13] | Kwon H J, Sengupta K, Yakovenko V M. Fractional ac Josephson effect in p-and d-wave superconductors[J].European Physical Journal B, 2004, 37:349. |
| [14] | Asano Y, Tanaka Y, Sigrist M, et al. Josephson interferometer in a ring topology as a proof of the symmetry of Sr2RuO4[J].Physical Review B, 2006, 71:214501. |
| [15] | Yokoyama T, Tanaka Y, Golubov A A. Theory of the Josephson effect in unconventional superconducting junctions with diffusive barriers[J].Physical Review B, 2007, 75:094514.DOI:10.1103/PhysRevB.75.094514 |
| [16] | Millis A, Rainer D, Sauls J A. Quasiclassical theory of superconductivity near magnetically active interfaces[J].Physical Review B, 1988, 38:4504. |
| [17] | Yip S. Weak link between conventional and unconve-ntional superconductors[J].Journal of Low Temperature Physics, 1993, 91:203.DOI:10.1007/BF00120849 |
| [18] | ] Sigrist M, Ueda K. Phenomenological theory of unconventional superconductivity[J].Reviews of Modern Physics, 1991, 63:239.DOI:10.1103/RevModPhys.63.239 |
| [19] | Asano Y, Tanaka Y, Sigrist M, et al. Josephson current in s-wave-superconductor/Sr2RuO4 junctions[J].Physical Review B, 2003, 67:184505.DOI:10.1103/PhysRevB.67.184505 |
| [20] | Eschrig M, Kopu J, Cuevas J C, et al. Theory of half-metal/superconductor heterostructures[J].Physical Review Letter, 2003, 90:137003.DOI:10.1103/PhysRevLett.90.137003 |
| [21] | Bujnowski B, Timm C, Brydon P M R. The Josephson effect between triplet superconductors through a finite ferromagnetic barrier[J].J Phys: Condens Matter, 2012, 24:045701.DOI:10.1088/0953-8984/24/4/045701 |
| [22] | Tanaka Y, Kashiwaya S. Josephson effect in unconventional-superconductor/ferromagnet/unconventional-superconductor junctions[J].Journal of the Physical Society of Japan, 1999, 68:3485.DOI:10.1143/JPSJ.68.3485 |
| [23] | Brydon P M R, Chen W, Asano Y, et al. Charge and spin supercurrents in triplet superconductor-ferromagnet-singlet superconductor Josephson junctions[J].Physical Review B, 2013, 88:054509.DOI:10.1103/PhysRevB.88.054509 |
| [24] | Furusaki A, Tsukada M. Dc Josephson effect and Andreev reflection[J].Solid State Communications, 1991, 78:299.DOI:10.1016/0038-1098(91)90201-6 |
| [25] | Asano Y. Direct-current Josephson effect in SNS junctions of anisotropic superconductors[J].Physical Review B, 2001, 64:224515.DOI:10.1103/PhysRevB.64.224515 |
| [26] | Brydon P R. Spontaneous spin current due to triplet superconductor-ferromagnet interfaces[J].Physical Review B, 2009, 80:224520.DOI:10.1103/PhysRevB.80.224520 |
| [27] | de Gennes P G. Superconductivity of metals and alloys[M].New York: Addison-Wesley, 1989: 137-145. |
| [28] | Asano Y. Josephson spin current in triplet superconductor junctions[J].Physical Review B, 2006, 74:220501.DOI:10.1103/PhysRevB.74.220501 |
| [29] | Yinhan Z, Qiang C, Biao J. Tunneling conductance in ferromagnet/Sr2RuO4 junction: Detection of the d-vector direction[J].Physica C, 2010, 470:502.DOI:10.1016/j.physc.2010.04.008 |
| [30] | Mackenzie A P, Maeno Y. The superconductivity of Sr2RuO4 and the physics of spin-triplet pairing[J].Reviews of Modern Physics, 2003, 75:657.DOI:10.1103/RevModPhys.75.657 |